Interpreting the Chain Rule Graphically

Dr. Trefor Bazett
26 Aug 201705:14
EducationalLearning
32 Likes 10 Comments

TLDRThe video script explains the concept of the chain rule in calculus through a graphical illustration. It uses two functions, a parabola (f(x)) and a horizontally compressed version of it (H(x) = f(2x)). The script demonstrates algebraically how to compute the derivative of H(x) using the chain rule, which involves multiplying the derivative of the outer function (f') evaluated at the inner function (2x) by the derivative of the inner function itself (2). The explanation highlights how the chain rule accounts for the horizontal compression by adjusting the derivative accordingly. The script also discusses the points where the functions have horizontal tangent lines (zero derivatives) and verifies the formula by plugging in specific values. The example uses simple functions to illustrate the concept, but the principle applies to more complex functions as well, emphasizing the product of the outer function's derivative and the inner function's derivative as the key to finding the derivative of a composite function.

Takeaways
  • 📐 The chain rule is demonstrated graphically using two functions: a parabola f(x) and a horizontally compressed version, H(x), which is f(2x).
  • 🔄 H(x) is a composition of f(x) with an inside function G(x), where G(x) is simply 2x.
  • 🧮 To find the derivative of H(x) (H'(x)), apply the chain rule by first taking the derivative of the outer function (f') evaluated at the inside function (2x), and then multiplying by the derivative of the inside function (2).
  • 📍 The derivative of H at a point is found by evaluating the derivative of f at twice that point and multiplying by 2, reflecting the horizontal compression.
  • 🔵 The chain rule formula H'(x) = f'(2x) * 2 shows a horizontal compression factor from the inside function and an additional multiplication by 2.
  • 🔑 The points where the horizontal tangent lines (zero derivatives) occur for f(x) and H(x) are different, with H(x) having a zero derivative at x = 1/2, while f(x) has it at x = 1.
  • 🈚️ At x = 1/2, H'(x) is calculated to be zero, verifying the chain rule formula since f'(1) is zero and the multiplication by 2 does not affect the result.
  • ➗ The concept of the chain rule remains applicable even if the functions f(x) and G(x) are more complex, as the principle of multiplying the derivatives of the outer and inner functions is consistent.
  • ↔️ The derivative of the composition function shifts with the inside function's value: f'(2x) reflects the effect of the G(x) = 2x transformation.
  • ✖️ The multiplication by 2 in the chain rule not only adjusts the value of the derivative but also reflects the change in the slope due to the horizontal compression.
  • 📉 At points where the derivative of f(x) is zero, the derivative of H(x) will also be zero, as demonstrated with the specific value of x = 1/2.
  • 📈 Conversely, at points where the derivative of f(x) is non-zero, the derivative of H(x) will be the product of the derivative of f at the transformed point (2x) and the derivative of the inside function (2).
Q & A

  • What is the main topic of the transcript?

    -The main topic of the transcript is the explanation of the chain rule in calculus, using a graphical illustration involving two functions, a parabola and a horizontally compressed version of it through composition.

  • What is the first function mentioned in the transcript?

    -The first function mentioned is a parabola represented by f(x).

  • How does the second function, H(x), relate to the first function, f(x)?

    -The second function, H(x), is a composition of f(x) with another function G(x), where G(x) is defined as 2x, which horizontally compresses the graph of f(x).

  • What is the role of the inside function G in the composition?

    -The inside function G(x) = 2x is used to horizontally compress the graph of f(x), effectively changing the x-values that f(x) operates on.

  • How does the chain rule apply to finding the derivative of H(x)?

    -The chain rule states that the derivative of H(x), denoted as H'(x), is found by multiplying the derivative of the outer function f' evaluated at the inside function (2x), by the derivative of the inside function (2).

  • What is the algebraic expression for the derivative of H(x) using the chain rule?

    -The derivative of H(x), H'(x), is f'(2x) * 2.

  • What happens to the derivative of H(x) when the derivative of the inside function is zero?

    -When the derivative of the inside function (G'(x) which is 2) is multiplied by the derivative of the outer function evaluated at a point where its derivative is zero, the result is zero.

  • At what x-value does the derivative of the inside function G(x) become zero?

    -The derivative of G(x) = 2x is always 2 and never becomes zero, since G(x) is a linear function with a constant rate of change.

  • What is the significance of the points where the functions have horizontal tangent lines?

    -The points where the functions have horizontal tangent lines are significant because at these points the derivative of the function is zero, indicating an inflection point or a peak/valley in the function's graph.

  • How does the chain rule reflect the effect of the inside function's derivative on the derivative of the composition?

    -The chain rule reflects this by multiplying the derivative of the outer function evaluated at the inside function by the derivative of the inside function. This shows how the rate of change of the inside function modulates the rate of change of the outer function.

  • In the example given, how does the slope of the tangent line change when evaluating the derivative of H(x) at different points?

    -The slope of the tangent line changes by a factor that is the product of the slope of the outer function's tangent line at the transformed point (2x) and the derivative of the inside function (2 in this case).

  • What is the key takeaway from the transcript regarding the application of the chain rule?

    -The key takeaway is that to find the derivative of a composition of functions, one must evaluate the derivative of the outer function at the point transformed by the inside function and multiply by the derivative of the inside function.

Outlines
00:00
📊 Understanding the Chain Rule with Graphical Illustrations

This section provides a visual and algebraic explanation of the chain rule using two functions: a parabola f(x) and its horizontal compression h(x) = f(2x), referred to as F of 2x. The inner function g(x) equals 2x, making the composite function h(x) = F(g(x)). The chain rule is applied to find the derivative h'(x), which involves multiplying the derivative of the outer function f'(2x) by the derivative of the inner function g'(x), which is 2. The explanation is illustrated by examining specific points on the parabola where the derivative is zero, demonstrating how the chain rule correctly predicts these derivatives through a series of graphical and numerical examples.

05:01
🔗 Chain Rule Application: Modulating Factors in Derivatives

The second paragraph highlights how the chain rule integrates a 'modulating factor'—in this case, the derivative of the inner function, which is 2. This modulating factor adjusts the derivative of the outer function, resulting in the derivative of the composite function. The relationship shows that while the derivative of the original function f(x) is evaluated at a shifted point (due to the inner function g(x) = 2x), it is also scaled by the derivative of g(x), illustrating the dual influence of shifting and scaling in the application of the chain rule.

Mindmap
Keywords
💡Chain Rule
The Chain Rule is a fundamental principle in calculus for finding the derivative of a composite function. It states that the derivative of a function composed of two functions is the derivative of the outer function evaluated at the inner function, multiplied by the derivative of the inner function. In the video, the Chain Rule is used to find the derivative of H(x) = F(2x), illustrating how the rule accounts for the horizontal compression factor introduced by the inner function G(x) = 2x.
💡Derivative
A derivative in calculus represents the rate at which a function changes with respect to a change in its variable. It is a measure of the sensitivity to change. In the context of the video, the derivative of H(x) is calculated using the Chain Rule, showing how the derivative of the outer function F and the inner function G(x) = 2x interact.
💡Parabola
A parabola is a U-shaped curve that is often the graph of a quadratic equation. In the video, the function f(x) is described as a parabola, and its transformation under the composition with the function G(x) = 2x is discussed. The transformation results in a horizontally compressed parabola, F(2x), which is a key visual element in the explanation of the Chain Rule.
💡Composition of Functions
The composition of functions involves applying one function to the result of another. In the video, the function H(x) is a composition of the functions F and G, where H(x) = F(G(x)). The concept is central to the application of the Chain Rule, as it involves finding the derivative of H(x) in terms of x.
💡Horizontal Compression
Horizontal compression in the context of functions refers to the process of scaling the x-values of a graph, effectively squishing or stretching the graph horizontally without altering its y-values. In the video, the function F(2x) represents a horizontally compressed version of f(x), which is a direct result of the composition with the function G(x) = 2x.
💡Tangent Line
A tangent line to a curve at a given point is a straight line that 'just touches' the curve at that point. It represents the instantaneous rate of change of the function at that point. The video discusses the tangent lines of the functions f(x) and H(x) at points where their derivatives are zero, which are key to understanding the behavior of these functions at those points.
💡Zero Derivative
A zero derivative indicates a point on a function where the slope of the tangent line is horizontal, meaning there is no change in the function's value at that point. In the script, the points where the derivatives of f(x) and H(x) are zero are identified, which are crucial for understanding the application of the Chain Rule.
💡Graphical Illustration
Graphical illustration involves using visual representations such as graphs or diagrams to explain a concept or data. The video uses graphical illustrations to demonstrate how the Chain Rule works by showing the transformation of the parabola f(x) into F(2x) and how this transformation affects the derivative.
💡Algebraic Computation
Algebraic computation refers to the process of performing mathematical operations using symbols and algebraic rules. In the video, the Chain Rule is applied algebraically to find the derivative of H(x), showing the step-by-step process of how the derivative is calculated.
💡Inside Function
In the context of composite functions, the inside function is the one that is applied first, and its result is then used as the input for the outside function. In the video, G(x) = 2x is the inside function, which is composed with the outside function F to create H(x) = F(2x).
💡Outside Function
The outside function in a composite function is the one that is applied second, after the inside function has been applied. In the video, F is the outside function that is composed with the inside function G(x) = 2x to form the composite function H(x).
Highlights

Introduction to the chain rule in calculus using graphical examples.

Illustration of function composition through the example of a parabola F(x) and its horizontal compression F(2x).

Explanation of how F(2x) compresses the function F(x) into a narrower parabola.

Introduction of the inner function G, defined as G(x) = 2x, to explain function composition.

Application of the chain rule to compute the derivative of the composition H(x) = F(G(x)).

Detailing the process: Differentiate the outside function F at the inner function G(x), and multiply by the derivative of G(x).

Demonstration that the derivative of the inner function G(x) = 2x is simply 2.

Explanation of the derivative of H(x) incorporating a horizontal compression factor from the inner function G(x).

Analyzing points where the functions F and G have horizontal tangent lines or zero derivatives.

Verification of the chain rule by plugging specific values and checking the outcomes.

Observation that H'(0.5) equals zero, confirming the accuracy of the chain rule application.

Exploration of how the derivative of H varies from the derivative of F due to the influence of G.

Explanation of the derivative's behavior at different points, emphasizing the shift and scaling effects of G(x).

Comparative analysis of tangent lines and slopes of F at different points to illustrate the impact of the chain rule.

Generalization of the concept to potentially complicated functions F and G, maintaining the core principles of the chain rule.

Transcripts

Browse More Related Video

Chain rule | Derivative rules | AP Calculus AB | Khan Academy

The Chain Rule

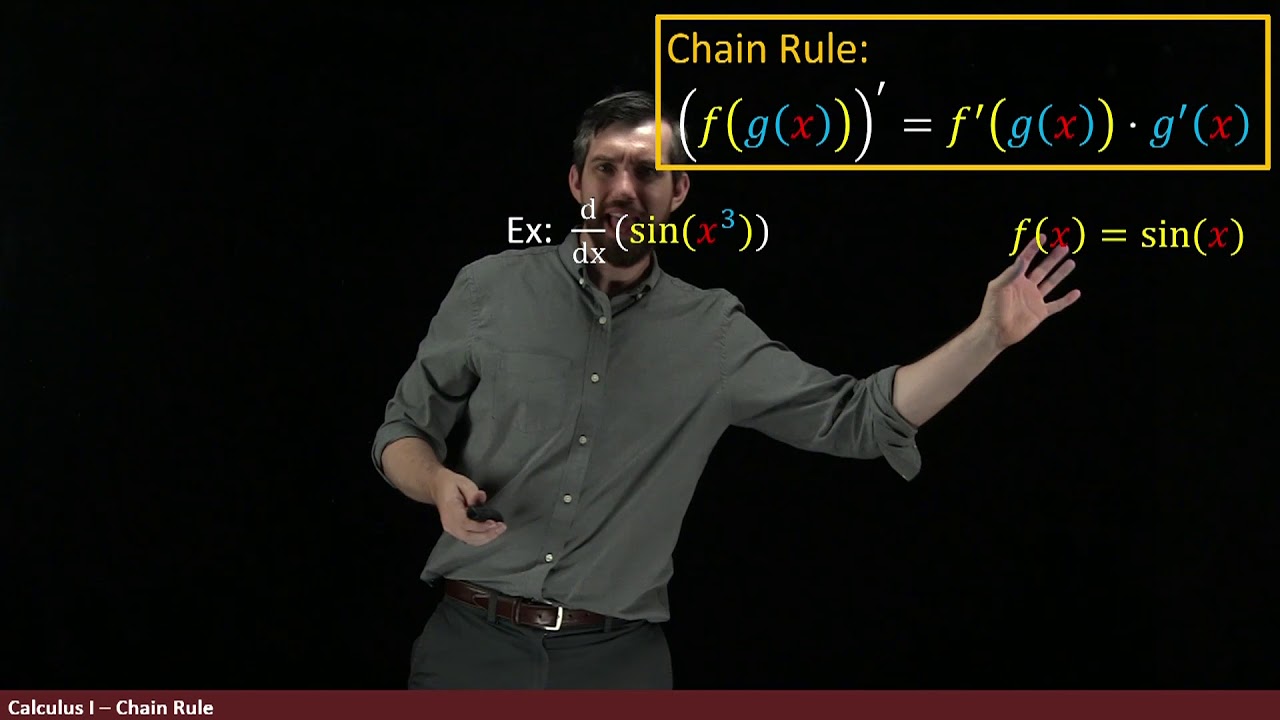
Chain Rule: the Derivative of a Composition

Exponential functions differentiation | Advanced derivatives | AP Calculus AB | Khan Academy

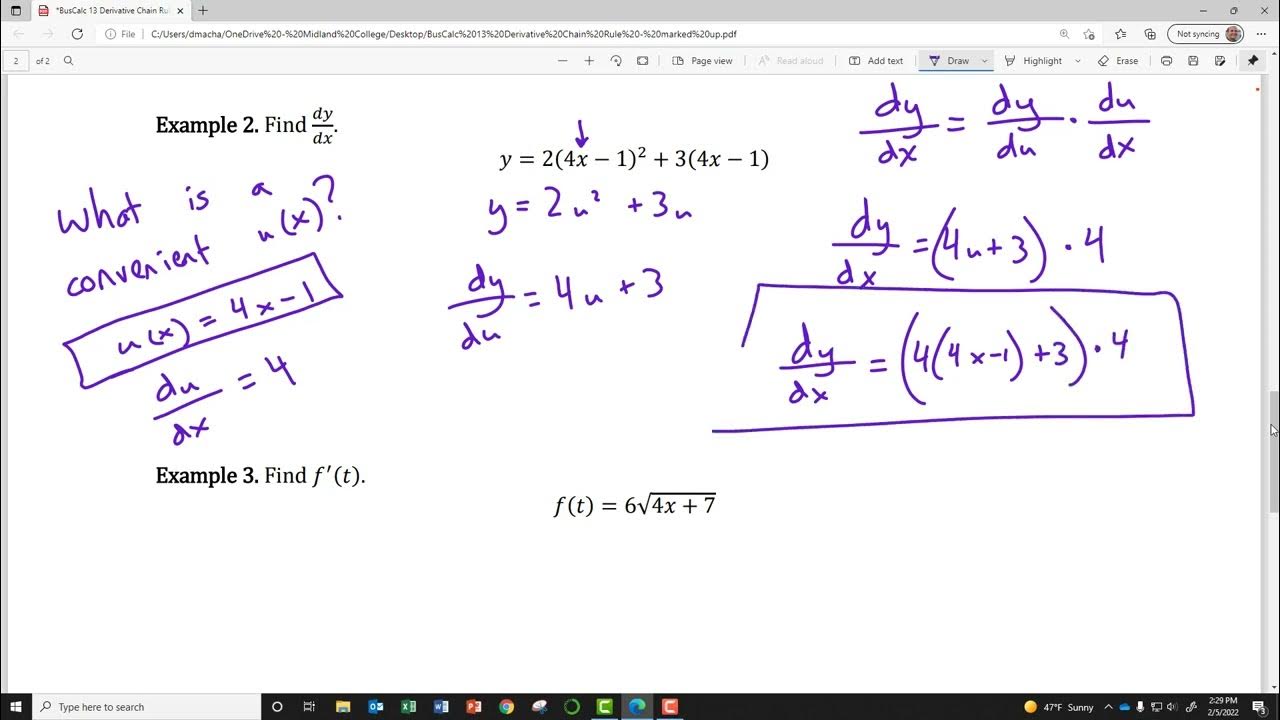
BusCalc 13 Derivative Chain Rule

Worked example: Derivative of cos_(x) using the chain rule | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Chain RuleDerivativesParabolic FunctionHorizontal CompressionAlgebraic GeometryGraphical IllustrationMathematical ConceptsFunction CompositionTangent LinesSlope AnalysisEducational Content