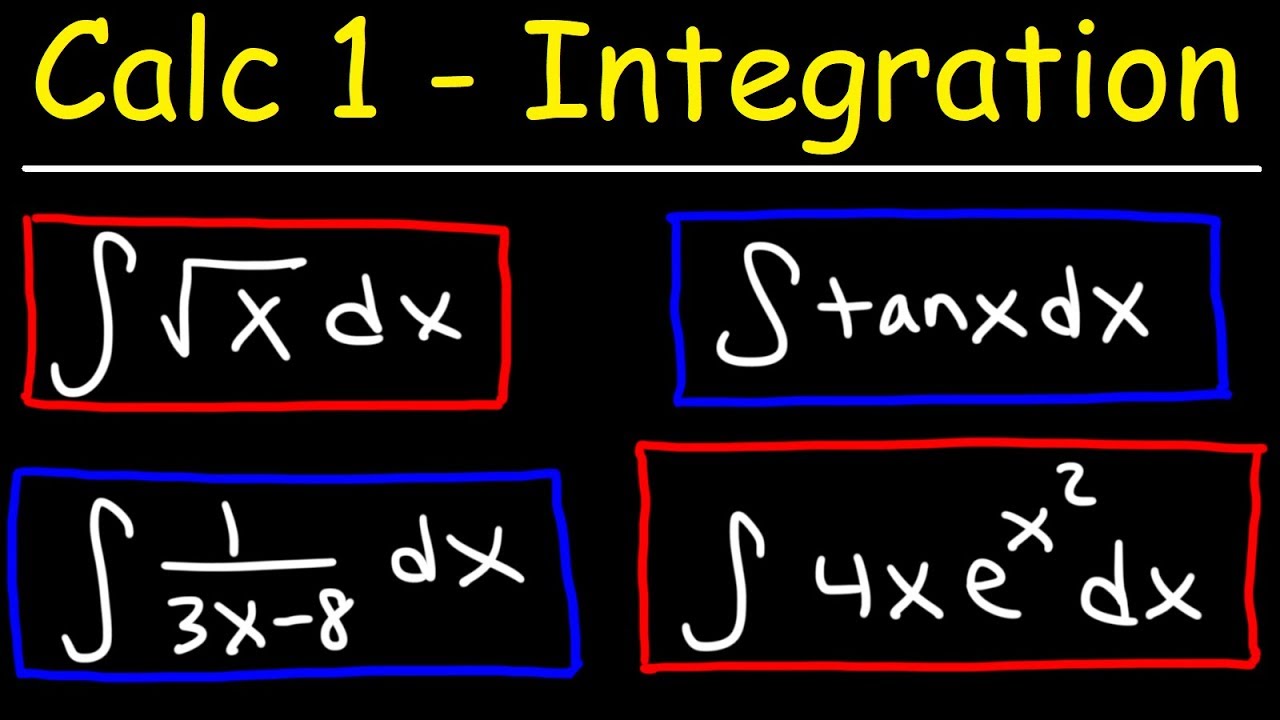
U-Substitution

The video begins with an introduction to U-substitution, a technique used for anti-differentiating composite functions. The presenter aims to expand the viewer's ability to reverse engineer the chain rule for anti-derivatives. The chain rule is reviewed, emphasizing the process of taking the derivative of the outer function and multiplying by the derivative of the inner function. The video then outlines a structured approach to U-substitution, starting with setting U equal to the inner function, taking its derivative, and manipulating the integral to isolate terms involving U's derivative. The process is illustrated with an example involving the anti-derivative of a composite function, leading to the discovery of the anti-derivative through U-substitution.

This section delves into 'perfect match' U-substitution scenarios where the derivative of the inner function directly corresponds to the outer function's derivative. The presenter demonstrates the process with an example involving the anti-derivative of (x^2 + 1)^4 multiplied by 2x. The steps include setting U to the inner function, deriving U with respect to x, and adjusting the integral to match terms that can be replaced by dU. The anti-derivative is then found by integrating with respect to U and substituting back to the original variable. The presenter also provides practice problems for viewers to apply the technique on their own.

The video continues with cases where the U-substitution is not an immediate perfect match, requiring some algebraic manipulation to fit the U-substitution criteria. The presenter shows how to adjust the integral by dividing through by a constant to make the match perfect, then proceeds with the substitution. An example is given where the integral involves x times (x^2 + 1) to the power of 4, which requires rewriting and dividing by two to match the derivative of U. The anti-derivative is then found in terms of U and substituted back to the original variable, x. The presenter emphasizes the importance of practicing these techniques for mastery.

In this part, the presenter discusses U-substitution when the inner function of the composition is linear. The video explains that anti-differentiating functions like the cosine of a linear function can be approached with U-substitution, even if it's not a perfect match. The example given involves anti-differentiating the cosine of 5x, where U is set to 5x, and the integral is adjusted by solving for dx in terms of du. The presenter shows that after substitution, the integral simplifies to a standard form that can be easily anti-differentiated, and the result is then expressed in terms of the original variable.

The focus shifts to definite integrals and how U-substitution can be applied to evaluate them. The presenter illustrates this with an example where the integral involves 1/(U'/U), with U being the denominator. The bounds of integration are converted from x values to U values, and the integral is then anti-differentiated with respect to U. The final step involves evaluating the anti-derivative at the new bounds and simplifying the result using logarithmic properties. The presenter advises against applying complex log properties to match multiple-choice answers in exams.

The video concludes with a set of practice problems for viewers to apply what they've learned about U-substitution in various scenarios, including definite integrals. The presenter provides guidance on how to approach these problems and the importance of practicing to gain confidence and experience. The video also touches on problem types involving graphs or tables where U-substitution is required, and the presenter demonstrates how to work through these, emphasizing the need to convert bounds and integrals entirely to U for consistency and accuracy.