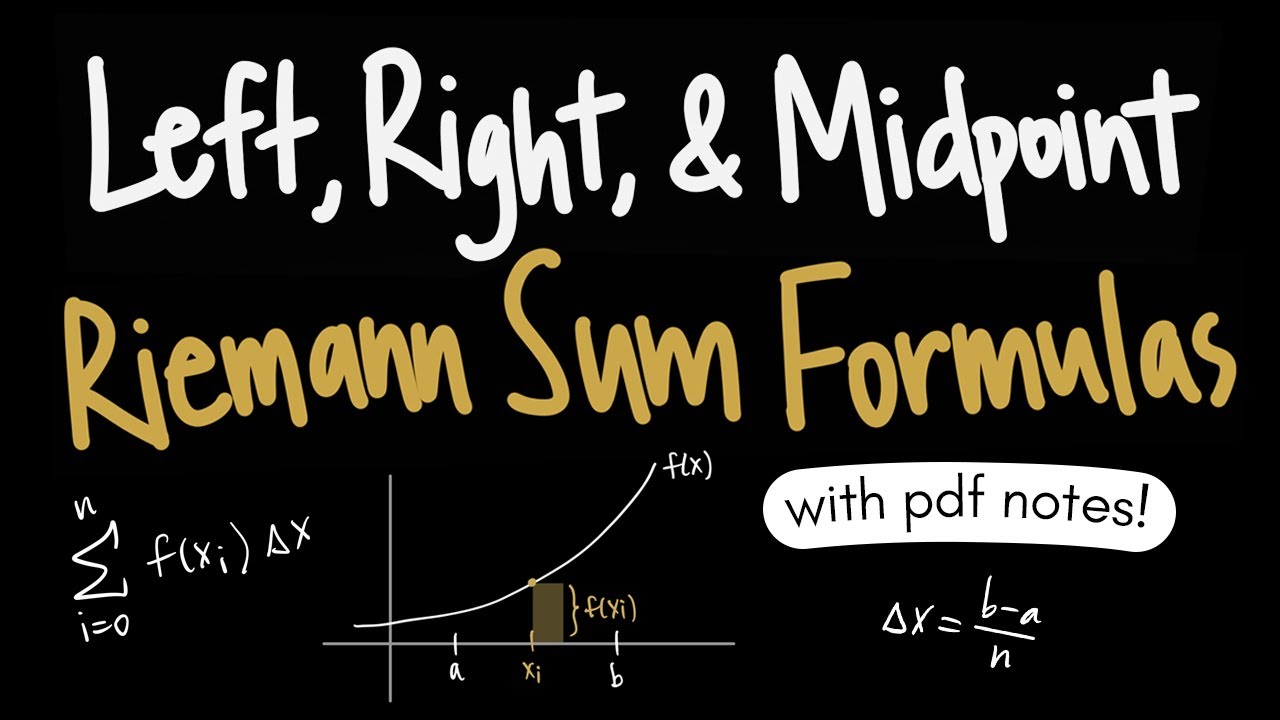
Riemann and Trapezoidal Sums from Tables of Values
TLDRThe video tutorial guides viewers through the process of approximating definite integrals using various Riemann sums and the trapezoidal rule, based on tables of data. The presenter begins with a left Riemann sum to approximate the integral of velocity over time, emphasizing the importance of acknowledging the approximation with 'approximately equal to.' Next, a right Riemann sum is used to approximate a different integral, highlighting the use of right endpoints for this method. The video then covers the trapezoidal sum for calculating the average value of a temperature function, explaining the formula for the area of a trapezoid and the process of summing these areas. Finally, the midpoint Riemann sum is explored for approximating the volume of a series of cylinders, demonstrating how to find midpoints and square the function values. The presenter provides step-by-step calculations, including simplifying the results and discussing units, to ensure a clear understanding of each method.
Takeaways
- 📊 **Left Riemann Sum**: Approximate the integral by multiplying the width of the interval by the value of the function at the left endpoint of the interval.
- 📈 **Approximation Significance**: Always use the approximation sign (≈) to indicate that the integral is being estimated, not calculated exactly.
- 🔢 **Interval Width and Endpoints**: Identify the width of each interval and the corresponding function value at the chosen endpoint (left or right) for Riemann sums.
- 🔠 **Right Riemann Sum**: For a right Riemann sum, use the function value at the right endpoint of the interval to calculate the sum.
- 🎛️ **Units in Calculations**: Ensure that the units of the integral match the units of the function being integrated.
- 📐 **Trapezoidal Sum**: Use the average of the function values at the endpoints of each interval and multiply by half the interval width to find the area of each trapezoid.
- 🔄 **Summing Trapezoids**: Add the areas of all trapezoids to approximate the integral over the given range.
- 🧮 **Midpoint Riemann Sum**: For a midpoint sum, find the midpoint of each interval and use the function value at that point, squaring it if necessary.
- 📋 **Table Marking**: Mark up tables carefully, ensuring to read the entire question before making assumptions about what values are needed.
- 📏 **Constant Factors**: Remember to include any constant factors, such as π in the given example, when approximating integrals.
- 🚫 **Avoid Generalizations**: Do not rely on memorized formulas for trapezoidal sums as each interval's height may differ, requiring individual calculation.
- 🔍 **Attention to Detail**: Be cautious of skipping columns if the midpoint does not align with the table's data points.
Q & A
What is the main topic of the video?
-The video is about finding Riemann sums and trapezoidal sums from tables of data to approximate definite integrals.
What is the first method used in the video to approximate the integral from 0 to 14 of V(T) dT?
-The first method used is the left Riemann sum, which uses the left endpoint of each interval to approximate the integral.
How is the width of the interval determined in the left Riemann sum method?
-The width of the interval is determined by the difference between the T values of consecutive data points in the table.
What is the approximate value of the definite integral from 0 to 14 of V(T) dT using the left Riemann sum method?
-The approximate value of the integral is 980 meters.
What is the second method used in the video to approximate the integral from 4 to 25 of V(T) dT?
-The second method used is the right Riemann sum, which uses the right endpoint of each interval to approximate the integral.
How does the right Riemann sum method differ from the left Riemann sum method?
-The right Riemann sum method uses the right endpoint of each interval, whereas the left Riemann sum method uses the left endpoint.
What is the approximate value of the definite integral from 4 to 25 of V(T) dT using the right Riemann sum method?
-The approximate value of the integral is -550 meters.
What is the purpose of the trapezoidal sum method?
-The trapezoidal sum method is used to approximate the average value of a function over an interval by summing the areas of trapezoids formed between the function's graph and the x-axis.
How is the area of a trapezoid calculated in the trapezoidal sum method?
-The area of a trapezoid is calculated as half the height times the sum of the two bases (the function values at the endpoints of the interval).
What is the approximate average value of the temperature function from 0 to 15 using the trapezoidal sum method?
-The approximate average value is 298 degrees Fahrenheit.
What is the fourth method discussed in the video for approximating definite integrals?
-The fourth method discussed is the midpoint Riemann sum, which uses the function value at the midpoint of each interval.
How is the midpoint Riemann sum method applied in the video to approximate the integral of R(H) squared from 0 to 30?
-The method involves finding the midpoint of each interval, calculating the function value at that midpoint, squaring it, and then summing up these values after multiplying by π to approximate the volume of a series of cylindrical sections.
Outlines
📊 Left Riemann Sums and Approximating Definite Integrals
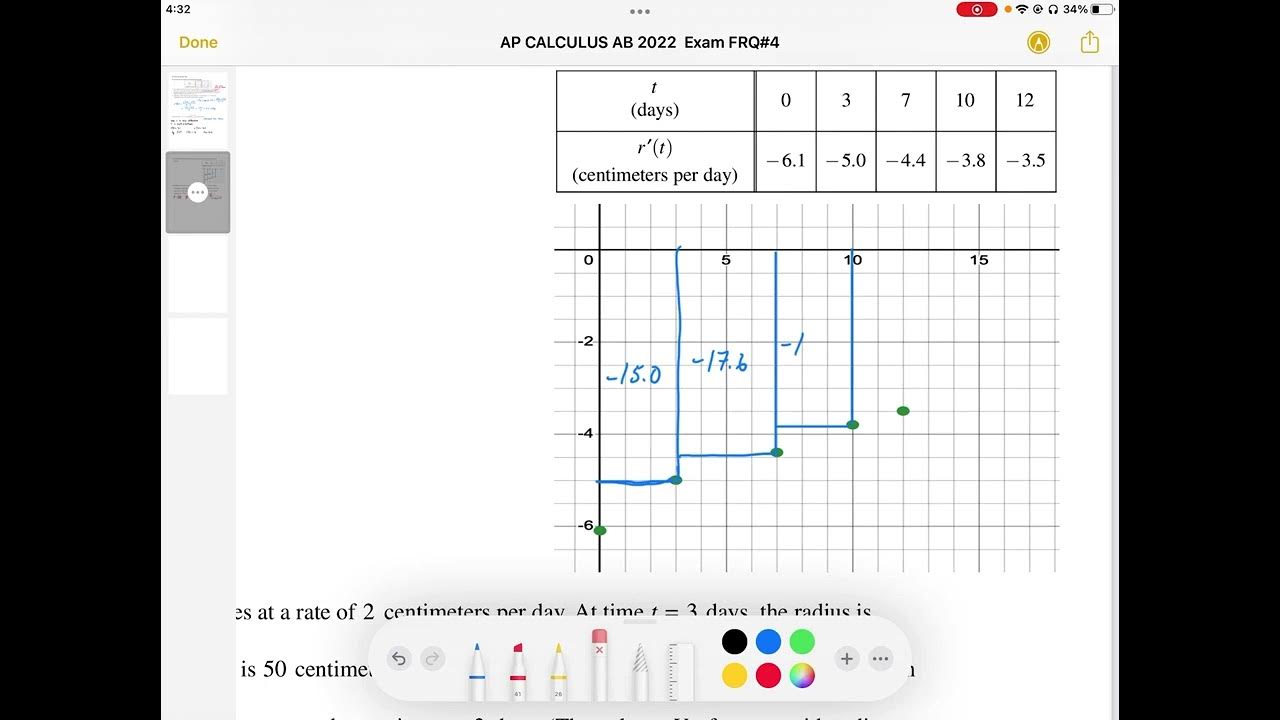
The first paragraph introduces the concept of using left Riemann sums to approximate definite integrals from a table of data. The video demonstrates how to calculate the integral from 0 to 14 of V(T) with DT, where T is time in minutes and V(T) is velocity in meters per minute. The process involves multiplying the width of each interval by the function value at the left endpoint of the interval and summing these products. The integral is then approximated to be 980 meters. The importance of acknowledging the approximation with 'approximately equal to' is emphasized, and the units of the integral are discussed.
📈 Right Riemann Sums and Definite Integral Approximation
The second paragraph explains the method of right Riemann sums for approximating definite integrals. It walks through the process of calculating the integral from 4 to 25 of V(T) DT, using the right endpoint values of the function within each interval. The width of each interval and the corresponding right endpoint function values are identified, and these are summed to approximate the integral, which is found to be approximately negative 550 meters. The concept of units is also discussed, noting that the result should be in meters, as the integral involves meters per minute over minutes.
🌡️ Trapezoidal Sums for Average Temperature Approximation
The third paragraph covers the use of trapezoidal sums to approximate the average value of a temperature function over an interval. The average value is defined as the integral of the temperature function H(T) from 0 to 15 divided by the interval length, 15. The process involves calculating the area of each trapezoid formed by the data points and summing these areas. The video emphasizes the importance of factoring out constants, such as 1/15, and not relying on generalizations when dealing with different heights in the trapezoids. The final approximation of the average temperature is given as approximately 298 degrees Fahrenheit.
🔢 Midpoint Riemann Sums for Volume Calculations
The final paragraph discusses the application of midpoint Riemann sums to approximate the integral that represents the volume of a shape, specifically the volume of cylinders stacked together. The integral to be approximated is π times the integral from 0 to 30 of R(H) squared dH, where R(H) is a function measured in inches. The video explains how to find the midpoint of each interval, use the corresponding height (R value), square it, and then sum these values. The final approximation is π times the integral from zero to thirty R(H) squared dH, which is approximately 7,868.433 cubic inches. The process is akin to summing the volumes of individual cylinders, each with a specific height and squared radius.
Mindmap
Keywords
💡Riemann sums
💡Trapezoidal sum
💡Midpoint Riemann sum
💡Definite integral
💡Table of data
💡Left endpoint
💡Right endpoint
💡Approximation
💡Units
💡Volume calculation
💡Cylinder
Highlights
The video demonstrates how to find Riemann sums and trapezoidal sums from tables of data.
Approximation of the integral from 0 to 14 of V(T) dT using a left Riemann sum is explained.
The importance of acknowledging approximation with the symbol '≈' is emphasized.
The process of calculating the width of the interval and using the left endpoint value for a left Riemann sum is shown.
The final approximate integral value is 980 meters, obtained using a calculator.
A right Riemann sum is used to approximate the definite integral from 4 to 25 of V(T) dT.
The right endpoint value is used for each interval in the right Riemann sum calculation.
The approximate integral value for the right Riemann sum is given as -550 meters.
A trapezoidal sum is introduced to approximate the average temperature on the interval from 0 to 15.
The formula for the area of a trapezoid (1/2 * height * sum of bases) is used in the trapezoidal sum.
The average value of the temperature function is found to be approximately 298 degrees Fahrenheit.
The midpoint Riemann sum is used to approximate π times the integral from 0 to 30 of R(H)^2 dH.
The midpoint of each interval and the corresponding height (R value) are used in the midpoint Riemann sum.
The integral represents a volume calculation, which is analogous to summing the volumes of cylinders.
The final approximate value of π times the integral is 7,868.433 cubic inches.
The video provides a step-by-step guide on how to handle different types of sums with tables in a mathematical context.
The importance of including constant multiples, such as π, in the approximation is highlighted.
Practical tips for simplifying calculations and avoiding common mistakes are offered throughout the video.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: