Rate constant k from half-life example | Kinetics | Chemistry | Khan Academy
TLDRThis educational video script explains how to determine the rate constant 'K' for a first-order chemical reaction using the decomposition of hydrogen peroxide as an example. It introduces two methods: a graphical approach using the integrated rate law and a more direct method leveraging the constant half-life of first-order reactions. The script emphasizes calculating 'K' from the half-life, demonstrating how to use the relationship between 'K' and half-life to find the rate constant without graphing, which is especially useful for exams or when a graphing calculator is unavailable.
Takeaways
- 🧪 The video explains how to find the rate constant (K) for a first-order reaction using provided data.
- 📊 One method to find K is to graph the natural log of concentration over time versus time and use the slope to determine K.
- 📝 Another method involves using the half-life of the reaction, which is constant for first-order reactions.
- 🔢 The half-life (t 1/2) is related to the rate constant by the equation t 1/2 = 0.693 / K.
- 🧩 To find K without graphing, identify the half-life from the data where the concentration halves.
- 🕒 In the given example, the half-life is consistently 2.16 x 10^4 seconds.
- ⚙️ By rearranging the half-life equation, K can be calculated as K = 0.693 / half-life.
- 📉 When calculating, K is found to be 3.21 x 10^-5 s^-1.
- 🧪 The data in the example is very clean and idealized; real experimental data might not look as perfect.
- 🔍 Recognizing a first-order reaction can be done by checking for a constant half-life in the data.
Q & A
What is the main objective of the video?
-The main objective of the video is to demonstrate how to find the rate constant K for a first-order reaction using concentration over time data.
What is the chemical reaction being analyzed in the video?
-The chemical reaction being analyzed is the decomposition of hydrogen peroxide (H2O2) into water (H2O) and oxygen (O2) at 40 degrees Celsius.
What is one method mentioned for determining the rate constant K graphically?
-One method for determining the rate constant K graphically is to plot the natural log of the concentration over time versus time and use the integrated rate law for a first-order reaction.
What is the integrated rate law equation for a first-order reaction used in the video?
-The integrated rate law equation for a first-order reaction used in the video is: ln([A]) = ln([A0]) - Kt, where [A] is the concentration at time t, [A0] is the initial concentration, and K is the rate constant.
Why might solving for K using the half-life be advantageous, especially during exams?
-Solving for K using the half-life is advantageous because it avoids the need for graphing calculators and can be done using a simple equation if the half-life is known.
What is the relationship between the half-life and the rate constant K for a first-order reaction?
-The relationship between the half-life (t 1/2) and the rate constant K for a first-order reaction is given by the equation: t 1/2 = 0.693 / K.
How was the half-life determined from the data points in the video?
-The half-life was determined by observing that the concentration of hydrogen peroxide halved consistently over a time period of 2.16 x 10^4 seconds across multiple data points.
What is the calculated rate constant K from the given data in the video?
-The calculated rate constant K from the given data is 3.21 x 10^-5 s^-1.
What units are used for the rate constant K in a first-order reaction?
-The units for the rate constant K in a first-order reaction are s^-1 (inverse seconds).
Why is it important to verify the constancy of the half-life when determining if a reaction is first-order?
-It is important to verify the constancy of the half-life because a constant half-life is a characteristic feature of a first-order reaction, confirming that the reaction follows first-order kinetics.
Outlines
🧪 Determining the Rate Constant for a First Order Reaction
This paragraph introduces the topic of finding the rate constant (K) for a first order chemical reaction using a given data set of hydrogen peroxide (H2O2) concentration over time. The reaction involves the decomposition of hydrogen peroxide into water and oxygen at 40 degrees Celsius. Two methods are suggested for solving for K: a graphical approach using the natural log of concentration versus time, and a more direct method leveraging the relationship between the half-life of the reaction and the rate constant. The integrated rate law for a first order reaction is also mentioned, which states that the natural log of the concentration at any time is equal to the natural log of the initial concentration minus K times time (Kt).
📈 Utilizing Half-Life to Calculate the Rate Constant
The second paragraph focuses on using the half-life of a first order reaction to calculate the rate constant without graphing. It explains that the half-life (t 1/2) is constant and can be used in the equation t 1/2 = ln(2) / K to solve for K. The natural log of two is approximately 0.693, which can be used to find K by dividing it by the half-life. The example data provided shows a consistent half-life of 2.16 x 10^4 seconds across different data points, confirming the reaction's first order nature. By substituting the half-life into the equation, the rate constant K is calculated to be 3.21 x 10^-5 with units of 1/s. The paragraph concludes by emphasizing the importance of recognizing a first order reaction through its constant half-life, whether from a graph or data table.
Mindmap
Keywords
💡First Order Reaction
💡Rate Constant (K)
💡Integrated Rate Law
💡Natural Logarithm
💡Half-Life (t 1/2)
💡Concentration
💡Decomposition Reaction
💡Graphing
💡Data Points
💡Time
💡Units
Highlights
The video discusses how to find the rate constant K for a first order reaction.
A table of data for hydrogen peroxide concentration over time is provided.
The reaction being studied is the decomposition of hydrogen peroxide to water and oxygen at 40 degrees Celsius.
Two common methods for solving for K are introduced: graphically using the integrated rate law and by using the half-life equation.
For a first order reaction, the natural log of concentration over time versus time graph is recommended.
The integrated rate law for a first order reaction is explained, where ln(concentration) = ln(initial concentration) - Kt.
The slope of the graph gives the value of -K, which can be used to find the rate constant.
An alternative method using the half-life of the reaction is suggested for easier calculation.
The half-life t1/2 is constant for a first order reaction and is related to the rate constant K.
The equation t1/2 = ln(2) / K is used to solve for K without graphing.
The natural log of two is approximately 0.693, which simplifies the half-life equation.
The half-life can be determined from the data table by observing when the concentration is halved.
The data points consistently show a half-life of 2.16 times 10 to the power of four seconds.
The example data is acknowledged as being idealized and cleaner than typical experimental results.
The rate constant K is calculated by rearranging the half-life equation to K = 0.693 / half-life.
The calculated rate constant K is 3.21 times 10 to the minus five per second.
The importance of recognizing a first order reaction by its constant half-life is emphasized.
The video concludes with a reminder that experimental data may not always be as clean as the example provided.
Transcripts
Browse More Related Video
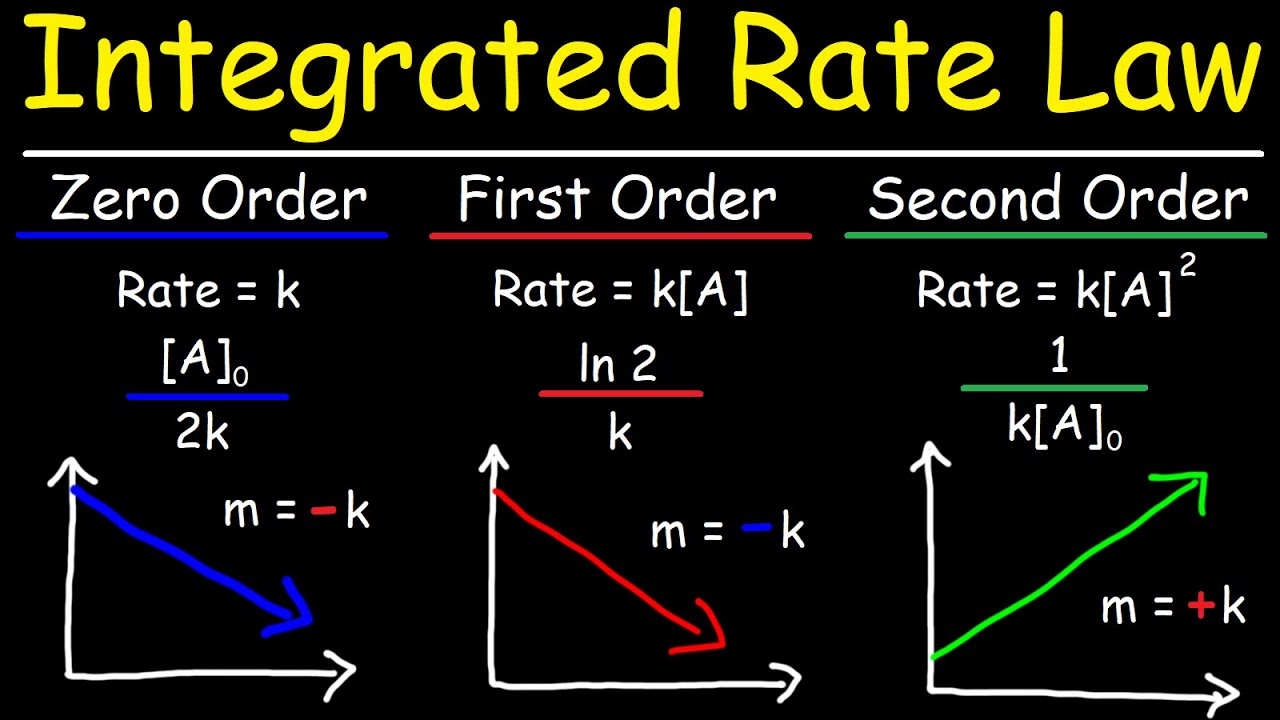
Integrated Rate Laws - Zero, First, & Second Order Reactions - Chemical Kinetics

AP Chemistry Unit 5 Part 1 Review: Reaction Kinetics
Units of the rate constant | Kinetics | AP Chemistry | Khan Academy

2 | FRQ (Short) | Practice Sessions | AP Chemistry

12.32 | Describe how graphical methods can be used to determine the order of a reaction and its rate
12.33 | Use the data provided to graphically determine the order and rate constant of the following
5.0 / 5 (0 votes)
Thanks for rating: