Raoult's Law - How To Calculate The Vapor Pressure of a Solution
TLDRThis video explains how Raoult's Law is used to calculate the vapor pressure of a solution, demonstrating with examples involving glucose and water, and calcium chloride. It covers the calculation of mole fractions and vapor pressures, highlighting the effect of non-volatile solutes on the vapor pressure of the solvent.
Takeaways
- 🌡️ Raoult's Law is used to calculate the vapor pressure of a solution, which is influenced by the presence of a non-volatile solute.
- 💧 The vapor pressure of a solution is the product of the mole fraction of the solvent and the vapor pressure of the pure solvent.
- 🍬 Adding a non-volatile solute to a solvent, like salt to water, decreases the vapor pressure of the resulting solution.
- 🧪 To calculate the vapor pressure of a solution, first determine the moles of the solvent and the solute.
- 🔍 The mole fraction of the solvent is calculated by dividing the moles of the solvent by the total moles in the solution.
- 🍇 In the example with glucose and water, the mole fraction of water is used to find the vapor pressure of the solution, which is slightly less than that of pure water.
- 🧂 When dealing with ionic compounds, calculate the total moles of ions in the solution, not just the moles of the compound.
- 🌡️ The vapor pressure of the solution is dependent on the total number of solute particles, not their identity.
- 🧪 For the example with calcium chloride, the vapor pressure of the solution is calculated using the mole fraction of water and the vapor pressure of pure water.
- 🍬 To find the mass of glucose needed to achieve a specific vapor pressure, use Raoult's Law and solve for the unknown mole fraction and moles of glucose.
Q & A
What is Raoult's Law?
-Raoult's Law states that the vapor pressure of a solution is equal to the product of the mole fraction of the solvent and the vapor pressure of the pure solvent. It implies that adding a non-volatile solute to a solvent will decrease the vapor pressure of the solution.
How does the vapor pressure of a solution compare to that of the pure solvent according to Raoult's Law?
-According to Raoult's Law, the vapor pressure of a solution will be less than the vapor pressure of the pure solvent when a non-volatile solute is added.
What is the vapor pressure of pure water at 25 degrees Celsius mentioned in the script?
-The vapor pressure of pure water at 25 degrees Celsius is 23.8 torr.
How is the mole fraction of the solvent calculated in Raoult's Law?
-The mole fraction of the solvent is calculated by dividing the moles of the solvent by the total moles in the solution, which includes both the moles of the solvent and the moles of the solute.
What is the molar mass of water and how many moles of water are in 500 milliliters?
-The molar mass of water is approximately 18.016 grams per mole. In 500 milliliters of water, there are 27.753 moles (calculated by dividing 500 grams by 18.016 grams per mole).
What is the chemical formula of glucose and what is its molar mass?
-The chemical formula of glucose is C6H12O6. Its molar mass is 180.156 grams per mole.
How many moles of glucose are in 30 grams?
-There are 0.1665 moles of glucose in 30 grams (calculated by dividing 30 grams by 180.156 grams per mole).
What is the mole fraction of water in a solution with glucose and water, and how is it calculated?
-The mole fraction of water is 0.994. It is calculated by dividing the moles of water (27.753) by the total moles in the solution (27.753 + 0.1665).
What is the vapor pressure of a solution with glucose dissolved in water, and how is it calculated?
-The vapor pressure of the solution is 23.66 torr. It is calculated by multiplying the mole fraction of water (0.994) by the vapor pressure of pure water (23.8 torr).
How does the presence of an ionic solute like calcium chloride affect the vapor pressure of a solution?
-The vapor pressure of a solution with an ionic solute is dependent on the total number of solute particles (ions) in the solution, not just the identity of the solute particles. This affects the calculation of the mole fraction and thus the vapor pressure.
How many grams of glucose need to be added to 250 grams of water at 40 degrees Celsius to achieve a vapor pressure of 54 torr?
-60.7 grams of glucose need to be added to 250 grams of water at 40 degrees Celsius to achieve a vapor pressure of 54 torr.
Outlines
🌡️ Raoult's Law and Vapor Pressure Calculation
This paragraph introduces Raoult's law, which is used to calculate the vapor pressure of a solution. The equation states that the vapor pressure of a solution is the product of the mole fraction of the solvent and the vapor pressure of the solvent. The law implies that adding a non-volatile solute to a solvent will decrease the vapor pressure of the solution. An example is provided where the vapor pressure of a glucose-water solution is calculated, demonstrating how the vapor pressure is less than that of pure water. The process involves calculating the moles of water and glucose, determining the mole fraction of water, and then using Raoult's law to find the vapor pressure of the solution.
🧪 Vapor Pressure with Ionic Solute
This paragraph extends the discussion on Raoult's law to include solutions with ionic solutes, such as calcium chloride in water. The key difference highlighted is that the total moles of ions in the solution must be considered, not just the moles of the ionic compound itself. The example calculates the moles of water and calcium chloride, converts the moles of calcium chloride to the moles of ions, and then calculates the mole fraction of water. Using Raoult's law, the vapor pressure of the solution is determined, showing how it is affected by the presence of ionic solute particles.
🔍 Determining Glucose Mass for Desired Vapor Pressure
The final paragraph focuses on using Raoult's law to determine the amount of glucose needed to achieve a specific vapor pressure in a water solution. Given the vapor pressure of water at 40 degrees Celsius and the desired vapor pressure of the solution, the mole fraction of water is calculated. This leads to determining the moles of water and subsequently the moles of glucose required. The process involves algebraic manipulation to solve for the moles of glucose and then converting these moles to grams, ultimately providing the mass of glucose needed to create a solution with the desired vapor pressure.
Mindmap
Keywords
💡Raoult's Law
💡Vapor Pressure
💡Mole Fraction
💡Solvent
💡Solute
💡Glucose
💡Calcium Chloride
💡Molar Mass
💡Ionic Compound
💡Vapor Pressure of Pure Water
Highlights
Introduction to Raoult's Law and its use in calculating vapor pressure of a solution.
Raoult's Law states that vapor pressure of a solution is less than that of the pure solvent when a non-volatile solute is added.
Example given: Vapor pressure of salt water solution is less than that of pure water.
Calculation of vapor pressure involves finding the mole fraction of the solvent.
Mole fraction is calculated as moles of solvent divided by total moles in the solution.
Example calculation provided for a solution of water and glucose.
Vapor pressure of the solution is calculated as the product of the mole fraction of water and the vapor pressure of pure water.
Result for the example shows the vapor pressure of the solution is slightly lower than that of pure water.
Introduction of a second example involving calcium chloride dissolved in water.
Explanation of how to calculate moles of water and moles of calcium chloride.
Emphasis on calculating total moles of ions when dealing with ionic compounds.
Vapor pressure of the solution is dependent on the total number of solute particles.
Calculation of mole fraction and vapor pressure for the calcium chloride solution.
Result for the calcium chloride solution shows a lower vapor pressure compared to pure water.
Introduction of a third example involving glucose added to water to achieve a specific vapor pressure.
Use of Raoult's Law to find the mass of glucose needed to achieve a desired vapor pressure.
Detailed calculation steps provided for determining the moles of glucose and its mass.
Final result shows the amount of glucose needed to create a solution with a specific vapor pressure at a given temperature.
Transcripts
Browse More Related Video

Boiling point elevation and freezing point depression | Chemistry | Khan Academy

Vapor pressure | States of matter and intermolecular forces | Chemistry | Khan Academy

reading water tables

2.4 Reference Table H (Vapor Pressure and Temperature)
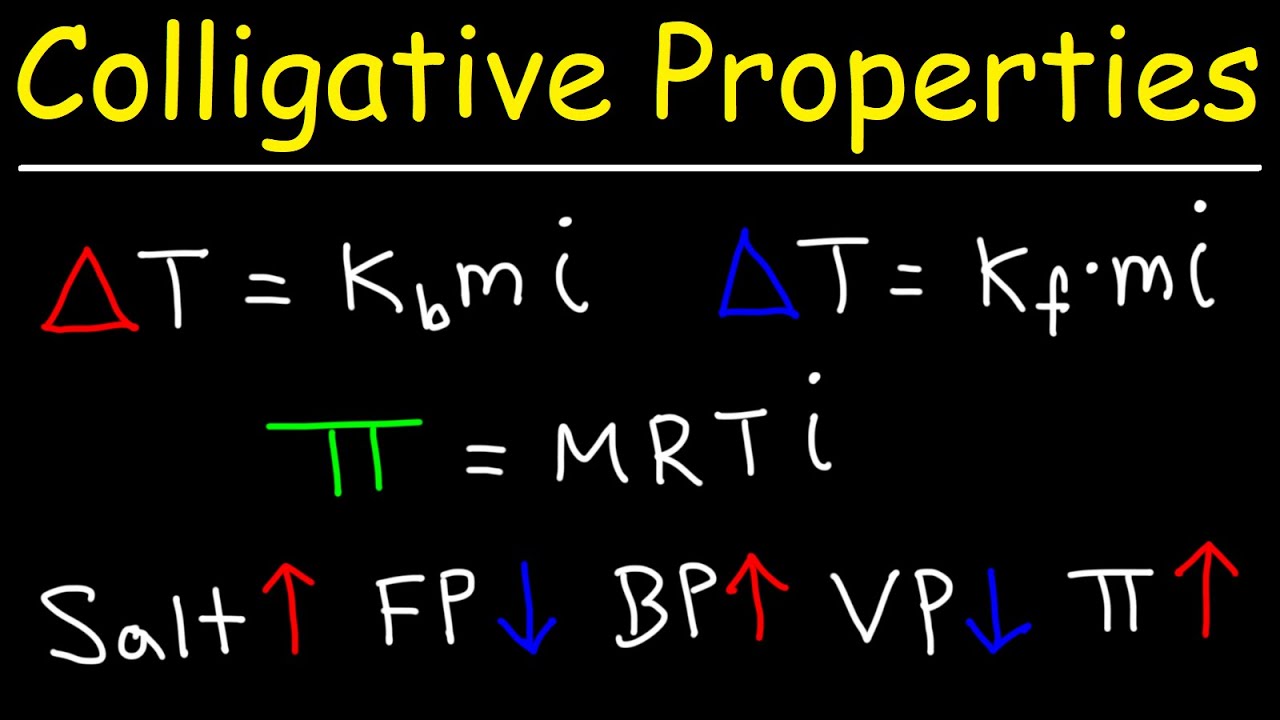
Colligative Properties - Boiling Point Elevation, Freezing Point Depression & Osmotic Pressure
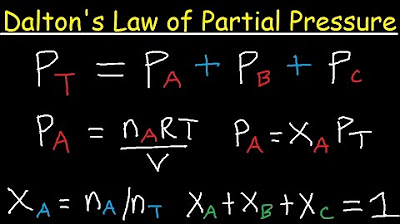
Dalton's Law of Partial Pressure Problems & Examples - Chemistry
5.0 / 5 (0 votes)
Thanks for rating: