FIND THE WORK REQUIRED TO PUMP THE WATER OUT OF THE TANK

Jake's Math Lessons
9 Apr 202115:06
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, Jake presents a calculus-based problem involving the work required to pump water out of a triangular prism tank. He explains the process of breaking down the tank into layers and using similar triangles to derive an equation for the volume of each layer. Jake then demonstrates how to calculate the force on each layer using the weight of water and integrates this to find the total work needed to empty the tank. The video highlights the difference in calculations between metric and imperial units, providing a practical example of applying calculus to real-world physics problems.

Takeaways
  • 📚 The video is a math tutorial by Jake, focused on calculating the work required to pump water out of a tank.
  • 💡 The problem is approached by considering the tank as a series of layers, each represented by a triangular prism.
  • 📐 The tutorial uses similar triangles to determine the dimensions of each layer of water as you go down the tank.
  • 🔢 A variable 'x*' is introduced to represent the vertical distance from the spout to the layer of water being considered.
  • 📉 The width of each layer is calculated using the ratio of similar triangles, resulting in an equation for the width in terms of 'x*'.
  • 📏 The volume of each layer is determined by considering it as a thin rectangular prism with a constant width of 10 feet.
  • 🌊 The weight of water per cubic foot (62.5 pounds) is used to calculate the force acting on each layer, skipping the need to calculate mass.
  • ⚙️ Work is defined as the force times the distance, and the formula for work is applied to find the work required to lift each layer.
  • 📈 An integral is set up to sum the work required for all layers, with the bounds from 0 to 6 feet representing the depth of the tank.
  • 📝 The integral is simplified and solved to find the total work required to pump all the water out of the tank.
  • 👍 The video concludes with an encouragement to subscribe for more math help, emphasizing the usefulness of the study guide mentioned.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is to demonstrate how to calculate the work required to pump water out of a tank through a spout, using imperial units.

  • What is the significance of using 'x_i*' in the video?

    -The 'x_i*' variable represents the vertical distance from the layer of water being considered to the spout, which is essential for calculating the work required to pump that layer of water.

  • How does the video approach the problem of calculating the work required to pump water out of the tank?

    -The video breaks down the problem by considering the tank as a series of layers, each represented as a rectangular prism, and then calculates the volume and force for each layer before integrating to find the total work.

  • What is the role of similar triangles in this problem?

    -Similar triangles are used to establish a relationship between the dimensions of the smaller triangle representing a layer of water and the larger triangle representing the tank, which helps in determining the width of each layer.

  • Why does the video mention a previous video about a spherical tank?

    -The video mentions a previous video to highlight the difference in calculations when using imperial units (as in the current video) versus the metric system used in the spherical tank example.

  • What is the purpose of the study guide mentioned in the video?

    -The study guide is a resource that includes formulas and explanations to help students study for calculus exams and complete homework more efficiently.

  • How does the video simplify the calculation of the volume of each layer of water?

    -The video simplifies the calculation by using the derived equation for the width of each layer, the constant width of 10 feet, and the concept of 'delta x' to represent the thickness of each layer.

  • Why does the video use 62.5 pounds per cubic foot to calculate the force acting on each layer?

    -The value of 62.5 pounds per cubic foot represents the weight of water, which accounts for both the mass of the water and the force of gravity, simplifying the calculation in the imperial system.

  • What is the formula for work used in the video?

    -The formula for work used in the video is 'work = force times distance', which is applied to calculate the work required to lift each layer of water to the spout.

  • How does the video determine the bounds for the integral to calculate the total work?

    -The video determines the bounds for the integral by considering the range of 'x' values from 0 (top layer of water) to 6 feet (bottom layer), representing the full depth of the tank.

  • What is the final step in the video to find the total work required to pump all the water out of the tank?

    -The final step is to integrate the derived equation for the work required to lift each layer of water over the bounds of 0 to 6 feet, which gives the total work in feet-pounds.

Outlines
00:00
📚 Introduction to Calculus 2 Work Problem

Jake introduces a calculus 2 work problem involving a water tank, aiming to calculate the work required to pump water out of a spout. He contrasts this with a previous problem involving a spherical tank, highlighting the difference in units between the metric and imperial systems. Jake also promotes his calculus 2 study guide for further learning resources.

05:01
📐 Setting Up the Problem with Similar Triangles

The explanation delves into the geometric approach to the problem, using similar triangles to determine the volume of the water in each layer of the tank. Jake introduces a variable 'x_i star' to represent the distance from the water layer to the spout and sets up a ratio to solve for the width 'y' of the water layer, leading to an equation for the volume of the water in terms of 'x_i star'.

10:01
🔢 Calculating Force and Work in the Imperial System

Jake explains the simplification of the problem due to the use of the imperial system, where the weight of water (62.5 pounds per cubic foot) already accounts for both mass and the force of gravity. He derives the equation for the force acting on each layer of water and then multiplies it by the distance 'x_i star' to find the work required to lift each layer. The process involves setting up an integral to sum the work for all layers from the top to the bottom of the tank.

15:02
📉 Integrating to Find Total Work Required

The final step involves integrating the derived equation to find the total work required to pump all the water out of the tank. Jake simplifies the integral and sets the bounds from 0 to 6 feet, corresponding to the depth of the tank. He demonstrates the integration process using the power rule and evaluates the integral to find the total work in foot-pounds, concluding the video with a call to action to subscribe for more calculus help.

👋 Closing Remarks and Subscription Invitation

In the closing paragraph, Jake thanks viewers for watching and encourages them to subscribe to his channel for more educational content on calculus. He expresses his hope that the viewers will find his videos helpful for their studies and achieve good grades.

Mindmap
Keywords
💡Work
In the context of this video, 'work' refers to the physical effort or energy required to move an object, in this case, water, against gravity. It is a central theme of the video, as the host explains how to calculate the work needed to pump water out of a tank. The script uses the term in the formula 'work = force x distance', illustrating the calculation process with the given dimensions and conditions of the tank.
💡Spout
The 'spout' is the outlet from which the water is being pumped out of the tank. It is a key component in the problem as the distance from the water layer to the spout determines the amount of work required. The script mentions the spout multiple times, emphasizing its role in calculating the vertical distance that each layer of water must be pumped.
💡Tank
The 'tank' is the container that is full of water in the problem presented. It is described as having a triangular prism shape with specific dimensions. The tank's geometry is essential for understanding the distribution of water layers and calculating the work required to pump the water out through the spout.
💡Imperial Units
The 'Imperial Units' refers to the system of measurement using feet and pounds, as opposed to the metric system which uses meters and kilograms. The video highlights the difference in calculations between these two systems, especially when dealing with the weight of water and the force of gravity, which is directly related to the work calculation.
💡Volume
In the script, 'volume' is used to describe the space occupied by a layer of water in the tank. The host explains how to calculate the volume of an individual layer, which is necessary to determine the amount of work required to pump that layer out. The volume calculation is integral to finding the force acting on the water due to gravity.
💡Similar Triangles
The concept of 'similar triangles' is used to relate the dimensions of different layers of water in the tank. By comparing the ratios of sides of these triangles, the host derives an equation for the width of each water layer as a function of its depth, which is crucial for calculating the volume and, subsequently, the work required.
💡Force
'Force' in this video is the product of mass and acceleration (as per Newton's second law), and it is used to calculate the work needed to move the water. The script explains that in the Imperial system, the weight of water (which includes both mass and the acceleration due to gravity) is used directly to find the force exerted on each layer of water.
💡Integral
An 'integral' in the context of the video is a mathematical operation used to sum up the work required for each infinitely thin layer of water in the tank. The host sets up an integral to find the total work by integrating the work equation over the range of depths from the top to the bottom of the tank.
💡Delta x
'Delta x' represents a small change in the independent variable, in this case, the depth at which a layer of water sits in the tank. It is used to describe the thickness of each layer when calculating the volume and, by extension, the work required to pump that layer out.
💡Rectangular Prism
A 'rectangular prism' is the shape that the host imagines each layer of water to be in order to calculate its volume. The script describes how each layer is treated as a thin rectangular prism with a length, width, and height, which are all functions of the depth within the tank.
💡Study Guide
The 'study guide' mentioned in the script is a resource that the host has created to help students with their calculus studies. It includes formulas and explanations that are relevant to the problem-solving process shown in the video, such as the formula for work.
Highlights

Introduction to a math problem involving calculating the work required to pump water out of a tank.

Comparison with a previous problem involving a spherical tank, emphasizing the difference in units between imperial and metric systems.

Mention of a calculus 2 study guide available for download, aimed at assisting with tests and homework.

Explanation of breaking down the tank into layers to calculate the volume of each layer of water.

Introduction of the variable 'x_i*' to represent the distance from the layer of water to the spout.

Use of similar triangles to determine the width of each water layer as it changes with depth.

Derivation of an equation for the width of the water layer dependent on 'x_i*'.

Calculation of the volume of the water layer using the derived width and fixed dimensions of the tank.

Transition from volume to force, leveraging the weight of water in the imperial system.

Explanation of how the weight of water in pounds per cubic foot accounts for both mass and gravity.

Conversion of the force acting on a water layer into work required to lift it using the formula 'work = force * distance'.

Setting up an integral to sum the work required for each layer of water to find the total work to pump all water out.

Integration of the work equation over the bounds of 0 to 6 feet to find the total work.

Simplification of the integral and application of the power rule to perform the integration.

Evaluation of the integral from 0 to 6 to find the total work in feet-pounds.

Conclusion of the video with an invitation to subscribe for more calculus problem-solving videos.

Transcripts

Browse More Related Video

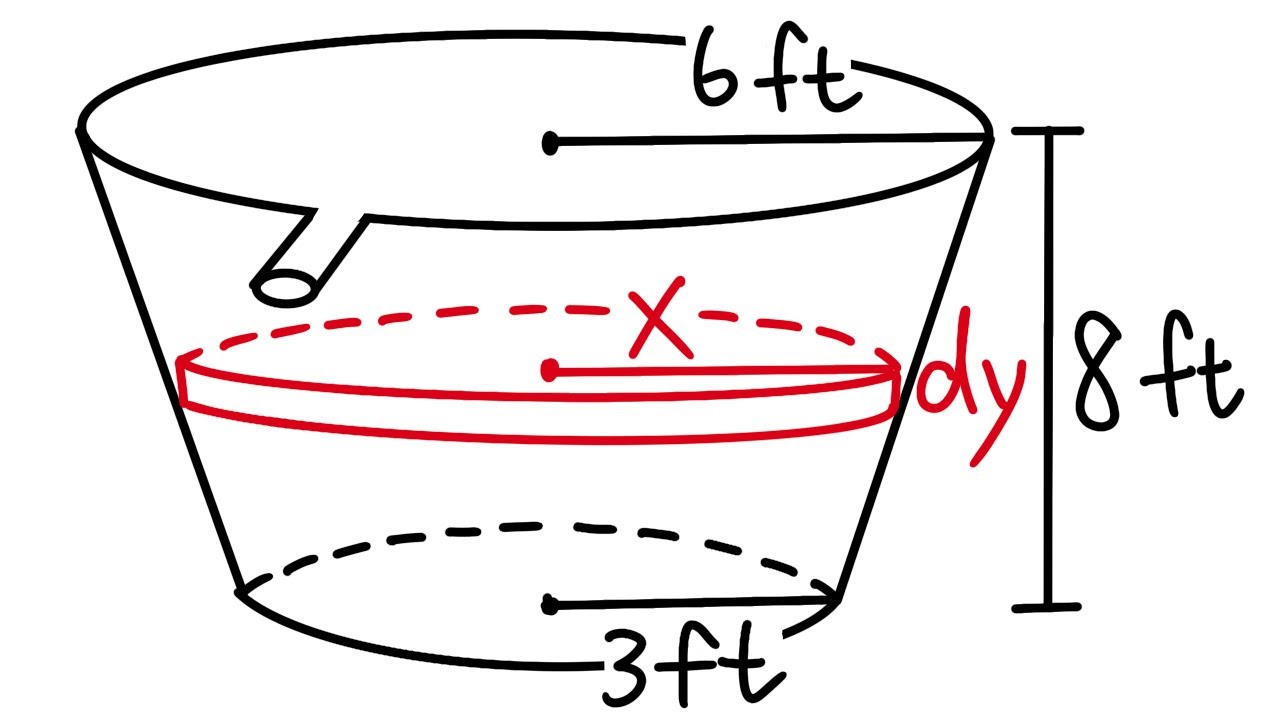
Pumping water out of a bucket, calculating work, calculus 2 tutorial

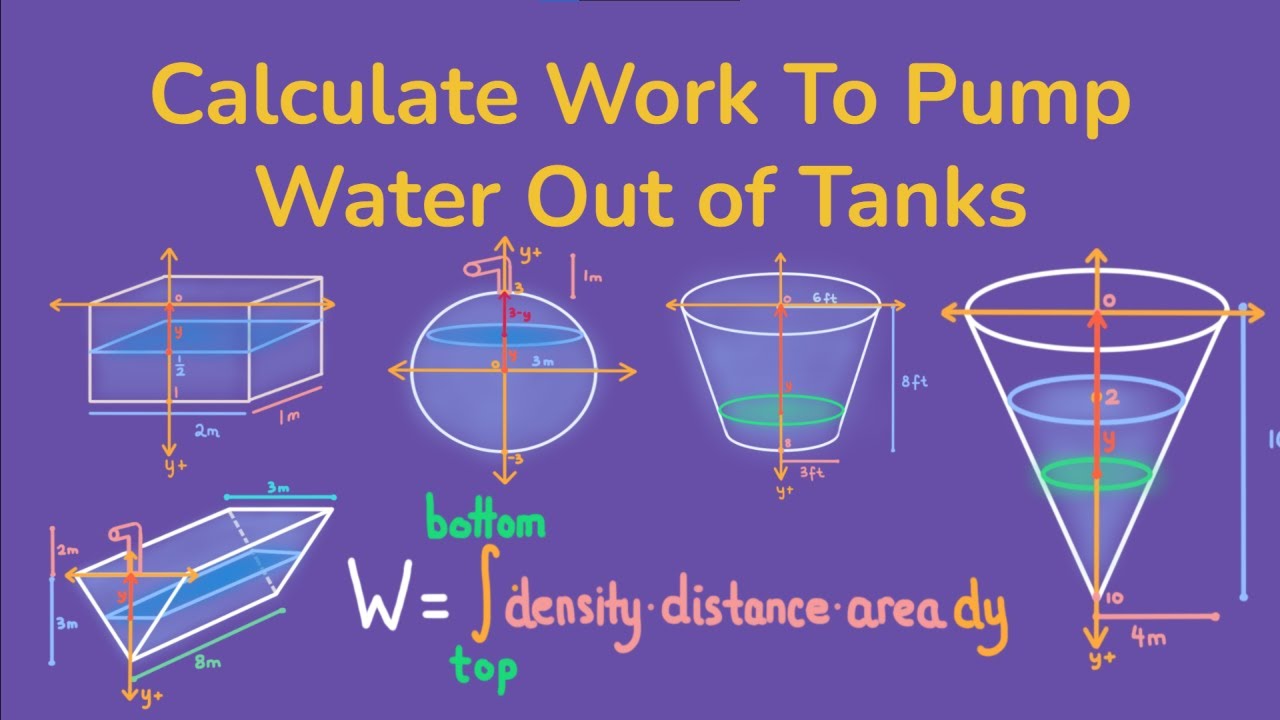
Calculate Work to Pump Liquid Out of Tanks - Calculus 2

Calculate Work to Pump Water Out of Rectangular Tank

Calculating the Work Required to Drain a Tank

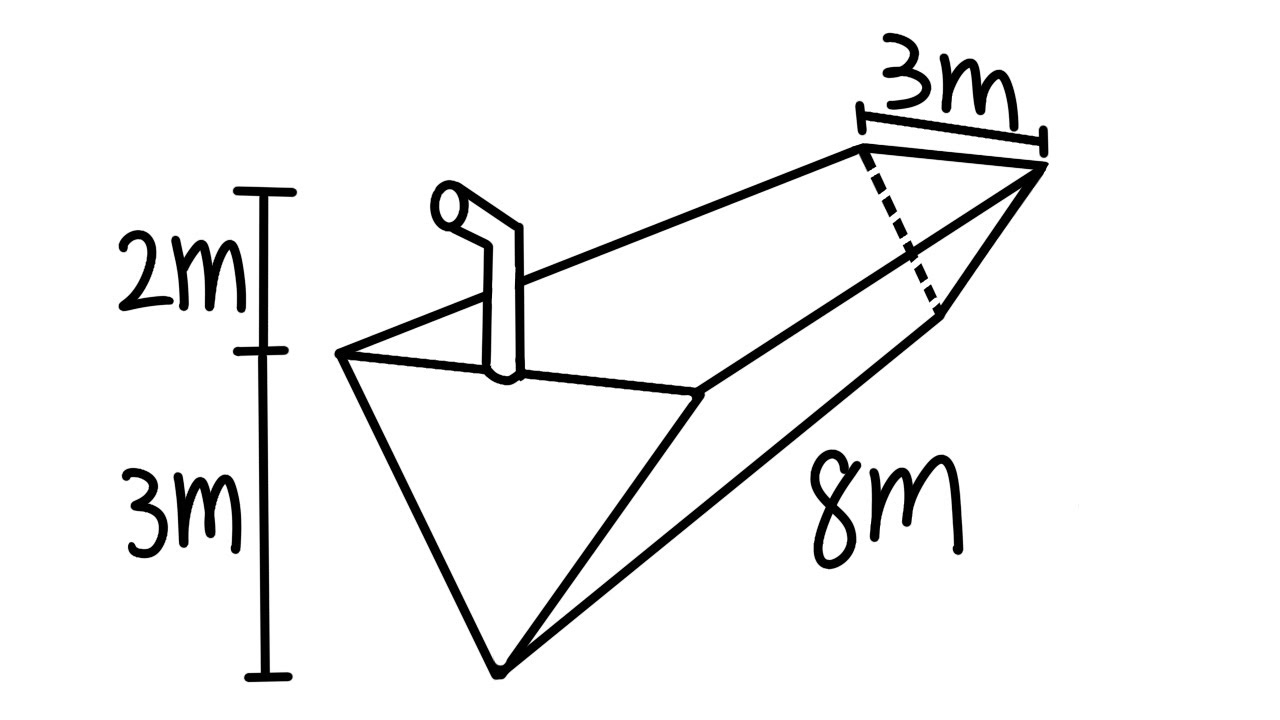
Calculating Work, pumping water out of a tank, calculus 2 tutorial, application of integration

calculating work by using integral, pumping water out of a tank, calculus 2 tutorial

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Calculus TutorialWork CalculationPhysics ProblemImperial UnitsFluid DynamicsLayered AnalysisVolume EquationForce of WaterIntegral CalculationEducational ContentStudy Guide