Math 195 Lecture 1 - The Real Numbers, Fractions, LCD, and Interval Notation
TLDRThis lecture introduces fundamental concepts in mathematics, focusing on the hierarchy of number sets including natural, integer, rational, irrational, and real numbers. It defines their properties and relationships, emphasizing the importance of the real numbers in calculus. The instructor discusses set notation, interval notation, and the arithmetic of fractions, stressing the need for efficiency in mathematical operations. The lecture aims to perfect the observation skills and mathematical foundations of students, preparing them for more advanced topics.
Takeaways
- 📚 The lecture covers foundational mathematical concepts, emphasizing the importance of understanding and perfecting basic skills for future learning in calculus and precalculus.
- 🔢 It introduces the hierarchy of numbers, starting with natural numbers (positive integers), followed by integers, rational numbers (fractions), and real numbers, which include both rational and irrational numbers.
- 📉 The real numbers are described as filling in all the gaps on the number line, in contrast to rational numbers which have gaps, such as the number represented by the square root of 2.
- 📝 The script explains mathematical properties such as commutative, associative, and distributive properties of real numbers, which are fundamental axioms in mathematics.
- 🔍 The importance of paying attention to detail is stressed, as even familiar topics may have nuances that students have not fully grasped.
- 📈 The concept of additive and multiplicative identities is discussed, along with the existence of negatives and reciprocals, which are essential for understanding operations on numbers.
- ➗ The process of simplifying complex fractions and the least common denominator (LCD) is introduced as a method to simplify expressions, highlighting the efficiency of using the LCD over traditional methods.
- 🔢 The script provides examples of how to multiply and divide fractions, as well as how to add them together, either by finding a common denominator or by using the LCD.
- 📐 The lecturer uses geometric interpretations, such as area of rectangles, to explain the distributive property and its acceptance as a fundamental truth in mathematics.
- 📋 Terminology related to sets, such as roster method, set builder notation, union, intersection, and interval notation, is defined, which is crucial for set theory in mathematics.
- 📘 The importance of efficiency in mathematical computations is highlighted, encouraging students to learn and practice the most efficient methods to save time and reduce errors.
Q & A
What are the basic sets of numbers discussed in the script?
-The basic sets of numbers discussed are natural numbers (positive whole numbers), integers (whole numbers including negative and zero), rational numbers (fractions of integers where the denominator is not zero), and real numbers (all numbers on the number line, including both rational and irrational numbers).
What is the difference between natural numbers and integers?
-Natural numbers are all the positive whole numbers (e.g., 1, 2, 3, ...), while integers include natural numbers, negative natural numbers, and zero.
What are the properties of real numbers mentioned in the script?
-The properties of real numbers mentioned include commutative properties (order does not matter in addition and multiplication), associative properties (allows for shifting parentheses in addition and multiplication), and the distributive property (allows for distribution of multiplication over addition).
What is an axiom in mathematics?
-An axiom is a mathematical statement that is accepted without proof, essentially a foundational assumption upon which other mathematical truths are built.
How are rational numbers defined in the script?
-Rational numbers are defined as fractions where both the numerator and the denominator are integers, and the denominator is not zero.
What is the additive identity in real numbers?
-The additive identity in real numbers is 0, because adding zero to any number does not change the value of that number.
What is the multiplicative identity in real numbers?
-The multiplicative identity in real numbers is 1, because multiplying any number by one does not change the value of that number.
What is the definition of a reciprocal in the context of real numbers?
-A reciprocal of a real number 'a' (where 'a' is not zero) is 1/a, which when multiplied by 'a' gives the multiplicative identity, 1.
What is the concept of the least common denominator (LCD) in fractions?
-The least common denominator (LCD) is the smallest positive number that all the denominators of a set of fractions can divide into evenly (resulting in whole numbers).
How is the least common denominator (LCD) found for two numbers?
-To find the LCD for two numbers, one should find the prime factorization of each number and then take the product of all the prime factors raised to their highest powers as they appear in either factorization.
What are the two main notations for representing sets?
-The two main notations for representing sets are the roster method, where you list the elements of the set, and the set builder notation, where you describe a property that the elements of the set must satisfy.
What is the difference between union and intersection of sets?
-The union of two sets includes all elements that are in either set, while the intersection includes only the elements that are common to both sets.
What is interval notation and why is it important?
-Interval notation is a way to express a range of numbers on the number line. It is important because it allows for clear and concise communication of sets of numbers that meet certain criteria, such as being between two values or greater than/less than a certain value.
What is the empty set and why is it significant in mathematics?
-The empty set is a set with no elements. It is significant because it represents a situation where no elements fulfill a given property, and it is a fundamental concept in set theory.
Outlines
📚 Introduction to Mathematical Concepts
The instructor begins by emphasizing the importance of paying attention in class, even to familiar topics, to refine observational skills that will be crucial later. The lecture delves into the foundation of real numbers, starting with natural numbers (positive integers), denoted in boldface as ℕ. It then covers integers (whole numbers including negative and zero), denoted as ℤ, and rational numbers, which are fractions of integers where the denominator is non-zero, denoted as ℚ. The real numbers, which fill in the gaps between rational numbers, are introduced with the notation ℝ, and the instructor mentions the complexity of defining them precisely. The concept of irrational numbers, which cannot be expressed as fractions but exist on the number line, is also briefly introduced.
🔍 Exploring the Properties of Real Numbers
This paragraph discusses the properties of real numbers, such as commutative and associative properties, which dictate the order of operations does not affect the outcome for addition and multiplication. The distributive property is also highlighted, which allows for the distribution of multiplication over addition. These properties are fundamental axioms, or statements accepted without proof, that all derived mathematical principles must adhere to. The instructor uses the analogy of area calculation to explain the intuitive nature of these properties, emphasizing their importance in understanding real numbers and their operations.
📘 Terminology and Basic Operations of Real Numbers
The instructor introduces key mathematical terminology related to real numbers, such as additive and multiplicative identities, which are numbers that do not change the value of another number when added or multiplied, respectively. The concept of negatives and the additive inverse is explained, along with the idea of moving units on the number line to represent addition and subtraction. The instructor also clarifies that zero is neither positive nor negative but is considered non-negative. The properties of negative numbers, such as their equidistant position from zero on the number line, are also discussed.
📙 Multiplication and Fractions in Real Numbers
The paragraph focuses on the multiplication of real numbers, introducing the concept of multiplicative identity and the reciprocal of a number. It explains that the reciprocal of a non-zero number is the number that, when multiplied by the original, yields the multiplicative identity. The instructor also covers the basic operations with fractions, including how to add, multiply, and divide them. The importance of never dividing by zero is emphasized, and the process of finding a common denominator for adding fractions is discussed. The concept of the least common denominator (LCD) is introduced as a way to simplify the process of adding fractions.
📒 Techniques for Simplifying Complex Fractions
The instructor discusses methods for simplifying complex fractions, which are fractions with other fractions in their numerator and/or denominator. Two primary methods are presented: one involves finding a common denominator and combining the fractions by definition, while the other uses the least common denominator (LCD) to simplify the complex fraction more efficiently. The benefits of using the LCD are highlighted, as it can reduce the number of steps required to simplify an expression, thus minimizing the potential for errors.
📕 Factoring and Efficient Mathematical Practices
This paragraph emphasizes the importance of factoring in mathematics, especially for simplifying expressions and solving equations. The instructor demonstrates the trial and error method for factoring quadratic expressions and contrasts it with the AC method, which will be covered later. The discussion highlights the value of efficiency in mathematical problem-solving, encouraging students to practice different methods to find the most efficient one for their needs. The instructor also stresses the importance of practicing to improve mental manipulation of numbers and expressions, especially in the absence of calculators.
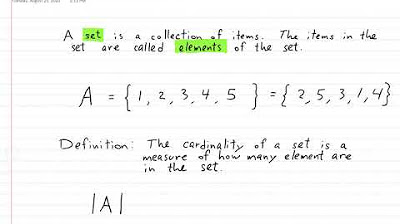
📔 Sets and Notation in Mathematics
The instructor introduces the concept of sets and the two main notations used to represent them: the roster method, which lists the elements of a set, and the set builder notation, which describes the properties that elements of a set must satisfy. The use of ellipses to denote continuation of a pattern and the symbols for membership and non-membership in a set are explained. Additionally, the paragraph covers the union and intersection of sets, explaining how to combine and find common elements between two sets, respectively.
📓 Interval Notation and Inequalities
This paragraph delves into interval notation, which is used to express the range of values that a variable can take, such as being greater than, less than, or between two numbers. The instructor explains the different types of intervals and their corresponding notations, including those that include or exclude the endpoints. The use of number lines to visually represent these intervals is also discussed, providing a pictorial understanding of the concepts introduced.
📝 Conclusion and Preview of Future Topics
In the concluding paragraph, the instructor reminds students that they are starting from the very beginning, ensuring that everyone has a solid foundation in the material. The instructor also previews upcoming topics, such as the handling of intervals and their intersections and unions, which will be covered in future lectures. The paragraph ends with a note that there is no homework assigned yet, but it will be given once the current topic is completed.
Mindmap
Keywords
💡Natural Numbers
💡Integers
💡Rational Numbers
💡Real Numbers
💡Irrational Numbers
💡Commutative Property
💡Associative Property
💡Distributive Property
💡Additive Identity
💡Multiplicative Identity
💡Fractions
💡Least Common Denominator (LCD)
💡Set Theory
💡Interval Notation
Highlights
Introduction to the syllabus and the importance of paying attention to refine observational skills.
Explanation of the hierarchy of numbers starting with natural numbers, integers, rational numbers, and real numbers.
Clarification of the definition of natural numbers and the distinction between different sources' definitions.
Description of integers, including the inclusion of negative natural numbers and zero.
Introduction to rational numbers as fractions with integer numerators and non-zero integer denominators.
Discussion on the difficulty of defining real numbers and their representation on the number line.
Differentiation between rational and irrational numbers, with examples of irrational numbers like π and e.
Complex numbers are briefly mentioned, emphasizing their irrelevance to the class.
Properties of real numbers, including commutative, associative, and distributive properties, are explained.
The concept of axioms in mathematics and their role as foundational statements without proof.
Terminology related to equations, such as expanding and factoring, and their importance in problem-solving.
Explanation of additive and multiplicative identities and their roles in mathematics.
Properties of negatives, including the negative of a number being equidistant from zero.
Guidance on adding and subtracting fractions, including finding a common denominator.
Multiplication and division of fractions, with the introduction of the least common denominator (LCD).
The process of simplifying complex fractions by using the LCD method.
Efficiency in mathematical problem-solving and the importance of choosing the most efficient method.
Introduction to set theory, including the definition of sets and the use of roster and set builder notation.
Explanation of union and intersection of sets, and the concept of the empty set.
Introduction to interval notation and its importance in expressing mathematical ideas pictorially.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: