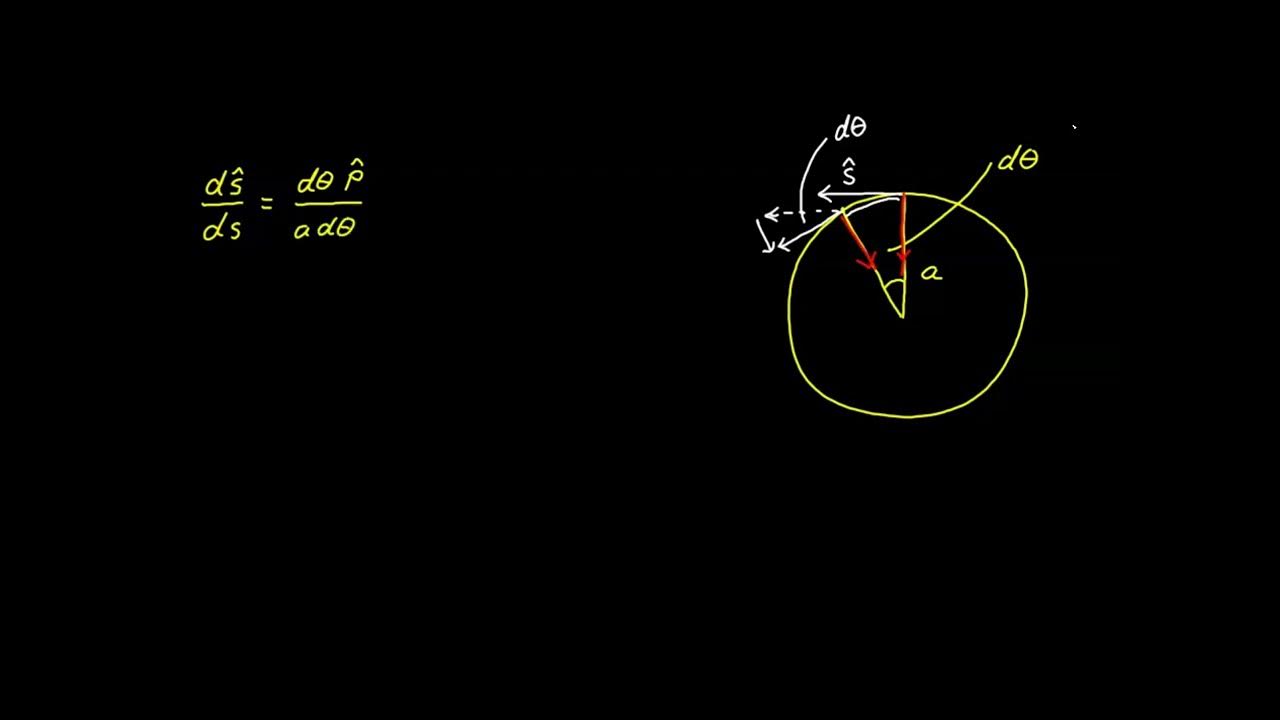Curvature of a helix, part 2
TLDRThis video script explores the concept of curvature in the context of a three-dimensional helix. The presenter explains how to calculate curvature by finding the circle that best fits the curve, akin to tracing a path with a spaceship. The focus is on deriving the unit tangent vector function and its derivative with respect to arc length. The process simplifies the tangent vector equation and calculates its derivative, leading to the curvature formula. The final curvature value indicates a slightly less curved path than a circle with a radius of one, which is intuitive given the helix's structure.
Takeaways
- 📚 The video discusses the concept of curvature for a three-dimensional curve, specifically a helix, and how to calculate it.
- 🚀 The curvature is likened to the circle that most closely follows the path of the curve, akin to the trajectory of a spaceship turning in space.
- 🔍 Curvature is calculated by finding the derivative of the unit tangent vector with respect to arc length, which is a measure of how much the curve deviates from being a straight line.
- 📐 The unit tangent vector function is given for the curve, which provides the direction of the curve at any point.
- 🔄 To find the curvature, the derivative of the unit tangent vector with respect to the parameter T is taken and then divided by the derivative of the parameterization function with respect to T, which corrects for arc length.
- 📝 The script simplifies the unit tangent vector function by adjusting the fractions to make the derivative calculation more straightforward.
- 🔢 The derivative of each component of the tangent vector function is calculated, resulting in a new vector.
- 🌀 The magnitude of the derivative vector is found by taking the square root of the sum of the squares of its components, simplified using trigonometric identities.
- 🔗 The curvature formula is applied by plugging in the magnitude of the derivative and the magnitude of the tangent vector function, leading to the curvature value.
- 🔑 The final curvature value is derived, indicating that the helix curves slightly less than a circle with a radius of one, which is consistent with the visual representation of the helix.
- 🎓 The video concludes by emphasizing the importance of understanding the process of finding curvature, including the concept of dt/ds and the unit arc length.
Q & A
What is the main topic discussed in the script?
-The main topic discussed in the script is the calculation of curvature for a three-dimensional helix curve.
What is the analogy used to explain the concept of curvature?
-The analogy used is imagining a circle that most closely hugs the curve, or considering the path a spaceship would trace out if its instruments locked up during a turn.
What is the definition of curvature in the context of the script?
-Curvature is defined as the reciprocal of the radius of the circle that most closely follows the curve, or the magnitude of the derivative of the unit tangent vector with respect to arclength.
What is the unit tangent vector function mentioned in the script?
-The unit tangent vector function is a mathematical function that, for any given parameter value T, provides a unit length vector that is tangent to the curve at that point.
Why is it necessary to take the derivative of the unit tangent vector with respect to arclength?
-Taking the derivative of the unit tangent vector with respect to arclength is necessary to find the curvature of the curve, as it provides information about how the direction of the tangent vector changes along the curve.
What is the process to correct the derivative of the parameterization function to correspond to unit length?
-The process involves dividing the derivative of the parameterization function by the magnitude of the derivative of the parameterization function with respect to T, which is the arclength.
How is the derivative of the tangent vector function calculated in the script?
-The derivative of each component of the tangent vector function is taken, resulting in a new vector where the components are the derivatives of the sine and cosine functions, divided by a constant.
What is the significance of the magnitude of the derivative of the tangent vector function?
-The magnitude of the derivative of the tangent vector function is used to calculate the curvature of the curve, as it represents the rate of change of the tangent vector's direction with respect to arclength.
How is the final curvature value obtained in the script?
-The final curvature value is obtained by taking the square root of the ratio of the magnitude of the derivative of the tangent vector function to the square of the magnitude of the derivative of the parameterization function.
What does the curvature value signify in the context of the helix?
-The curvature value signifies how tightly the helix is wound around its axis. A value less than one indicates that the helix is less curved than a circle with a radius of one.
Why is the curvature of the helix less than that of a circle with radius one?
-The curvature of the helix is less than that of a circle with radius one because the helix has an additional z-component, making it slightly straighter than a circle, thus reducing the curvature.
Outlines
📚 Calculating Curvature of a 3D Helix
This paragraph delves into the mathematical concept of curvature, specifically for a three-dimensional helix. The voiceover explains the process of finding the curvature by visualizing the circle that most closely fits the curve, akin to the path a spaceship would trace while turning. The focus then shifts to the unit tangent vector function, which at any given point T on the curve, provides a unit length vector tangent to the curve. The ultimate goal is to find the derivative of this unit tangent vector with respect to arc length, which involves taking the derivative with respect to the parameter T, and then normalizing by the derivative of the parameterization function with respect to T, which represents arc length. The explanation simplifies the unit tangent vector function and proceeds to calculate its derivative, emphasizing the importance of the magnitude of this derivative in determining curvature. The process concludes with finding the magnitude of the derivative of the tangent vector function, resulting in a simplified expression for curvature, which is approximately the square root of 25 over 26.
🔍 Finalizing the Curvature Calculation for a Helix
Building upon the previous explanation, this paragraph continues the discussion on calculating the curvature of a helix. It revisits the magnitude of the derivative of the tangent vector function, which was previously determined to be the square root of 26 divided by 5. The voiceover corrects a potential misconception about the cancellation of terms, clarifying that the terms 25 over 26 and 26 over 25 are reciprocal, not identical. The final step involves dividing the square root of 25 over 26 by the square root of 26 over 25, leading to the curvature value of 25 over 26. This result indicates a curvature slightly less than that of a circle with a radius of one, which makes intuitive sense given the helical shape's deviation from a perfect circle. The paragraph concludes by relating this mathematical finding back to the visual representation of the helix and hints at a future example that will utilize a direct formula for more complex scenarios.
Mindmap
Keywords
💡Parametric function
💡Curvature
💡Unit tangent vector
💡Arclength
💡Derivative
💡Magnitude
💡Parameterization
💡Sine and Cosine
💡Square root
💡Radius of curvature
Highlights
Exploration of a parametric function for a 3D curve, specifically a helix.
Introduction to the concept of curvature in 3D space, like imagining a spaceship tracing out a circle.
Explanation of the unit tangent vector function for a curve at any given value T.
The goal of finding curvature is to determine the derivative of the unit tangent vector with respect to arclength.
Clarification on the importance of the parameter T and its relation to arclength in the curvature formula.
Simplification of the unit tangent vector function by adjusting fractions for easier understanding.
Derivation of the tangent vector function, changing sine to cosine and vice versa, with adjustments for constants.
Calculation of the magnitude of the derivative of the tangent vector function.
Factoring out common terms to simplify the expression for the magnitude of the derivative.
Final expression for curvature derived as the square root of 25 divided by 26.
Interpretation of the curvature value, indicating a curve less than a circle with radius one.
Visual explanation of how the helix's curvature is affected by its z-component.
Discussion on the relationship between curvature and the radius of curvature in the context of a helix.
Introduction to the next example which will involve using the curvature formula for more complex scenarios.
Promise of a future video that will demonstrate the use of the curvature formula in a different context.
Emphasis on the practical application of finding dt/ds and the unit arclength in curvature calculations.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: