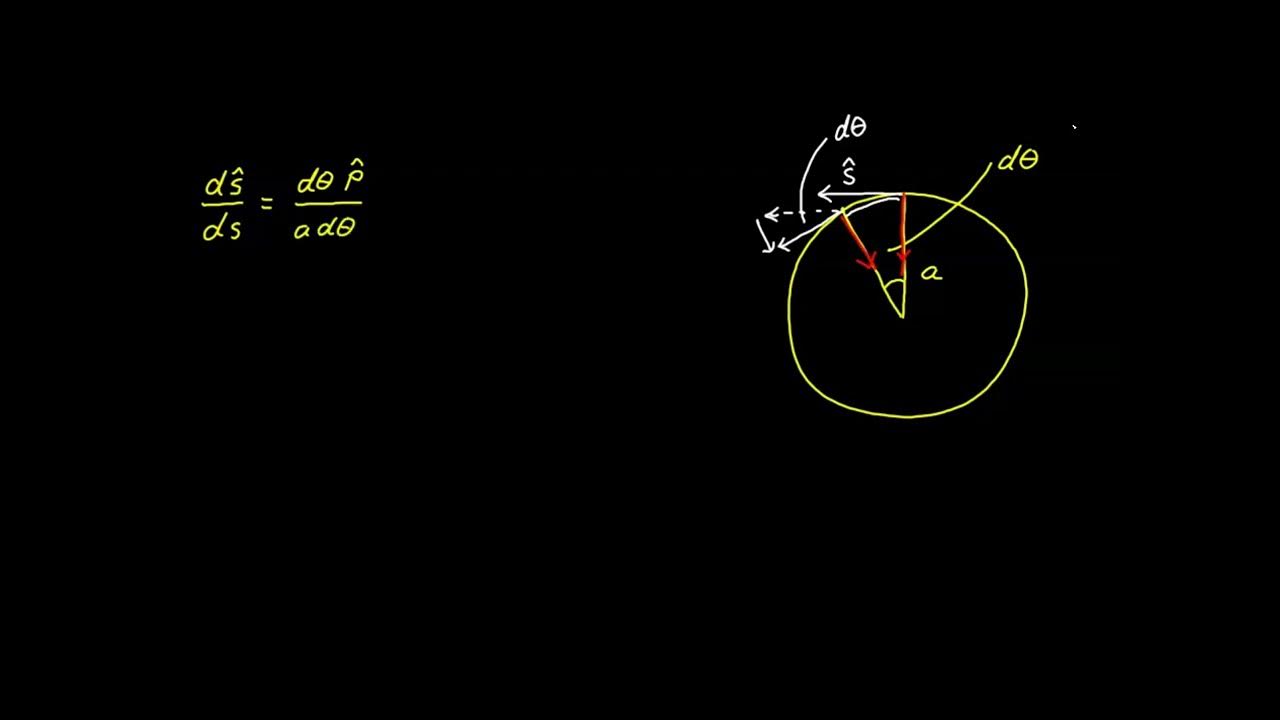Curvature of a helix, part 1
TLDRThe video script explores the concept of curvature in the context of a three-dimensional helix, a spiral curve resembling a circular path with an increasing z-component. It explains the visualization of a helix and introduces the idea of curvature as the radius of a circle traced by a spaceship steering wheel locked in place. The script delves into the mathematical process of finding the unit tangent vector by differentiating the parametric equation of the helix and normalizing it. The magnitude of the derivative is calculated, simplifying due to the properties of sine and cosine, leading to a constant magnitude. The unit tangent vector is then derived, setting the stage for further exploration in subsequent content.
Takeaways
- 📚 The script discusses the computation of curvature for a three-dimensional parametric curve, specifically a helix.
- 🌀 The helix is described with parametric equations involving cosine and sine for the x and y components, and a linear function for the z component.
- 🎨 A visual representation of the helix is provided, emphasizing its circular appearance from the xy-plane perspective and its spiral nature in 3D space.
- 🚀 The concept of curvature is introduced as the radius of the circle that a spaceship would trace if it were to lock in a certain position on the helix.
- 🔍 The curvature, or kappa, is calculated as the reciprocal of the radius of this circle, representing the rate of change in direction of the curve.
- 🔄 The unit tangent vector function is introduced as a method to determine the direction of travel along the helix at any given point.
- 📉 The process of finding the unit tangent vector involves taking the derivative of the parameterization and then normalizing it by its magnitude.
- 📐 The magnitude of the derivative of the parameterization is calculated, resulting in a constant value, simplifying the computation of the unit tangent vector.
- 📈 The unit tangent vector function is derived by dividing the derivative of the parameterization by its magnitude, yielding a simplified expression.
- 🔬 The script suggests that the magnitude's constancy simplifies the computation, as it does not vary with the parameter t, allowing for a straightforward unit tangent vector function.
- 📝 The script concludes with the derivation of the unit tangent vector function and indicates that further steps in the curvature calculation will be covered in a subsequent video.
Q & A
What is the parametric equation of the three-dimensional curve discussed in the script?
-The parametric equation of the curve is given by x = cos(t), y = sin(t), and z = t/5, which represents a helix.
What does the shape of the curve look like when viewed from the xy-plane perspective?
-From the xy-plane perspective, the curve appears to draw a circle due to the cosine and sine components of the x and y coordinates.
How does the z-component of the helix differ from the xy-plane components?
-The z-component, which is t/5, increases as the parameter t increases, giving the helix a spiral staircase-like appearance in the third dimension.
What is the concept of curvature in the context of the helix discussed in the script?
-Curvature in this context refers to the radius of the circle that a spaceship would trace out if it were to turn along the helix and then lock its steering, creating a giant circle in space.
Why is the unit tangent vector function important when computing curvature?
-The unit tangent vector function is important because it gives the direction of travel at every point on the curve, which is essential for determining the rate and nature of the curve's turning.
How is the unit tangent vector function derived from the parametric equation of the curve?
-The unit tangent vector function is derived by taking the derivative of the parametric equation, which gives the tangent vector, and then dividing by its magnitude to normalize it.
What is the derivative of the parametric function for the helix?
-The derivative of the parametric function is given by S'(t) = (-sin(t), cos(t), 1/5).
How is the magnitude of the derivative of the parametric function calculated?
-The magnitude is calculated by taking the square root of the sum of the squares of each component, which simplifies to √(1 + 1/25) due to the Pythagorean identity.
What simplification occurs when calculating the magnitude of the derivative due to the properties of sine and cosine?
-The simplification comes from the fact that sine squared plus cosine squared equals one, which reduces the magnitude calculation to √(1 + 1/25).
What is the final expression for the magnitude of the derivative of the helix's parametric function?
-The final expression for the magnitude is √(26/25), which can also be written as √(26)/5.
How is the unit tangent vector function related to the magnitude of the derivative?
-The unit tangent vector function is obtained by dividing each component of the derivative by the magnitude, resulting in a vector with a length of one.
Outlines
🌀 Introduction to Helix and Curvature
This paragraph introduces the concept of computing the curvature of a three-dimensional parametric curve, specifically a helix. The helix is described with its parametric equations involving cosine and sine functions for the x and y components, resembling a circle, and a linearly increasing z component, creating a spiral effect. The paragraph also explains the geometric interpretation of curvature as the radius of the circle that a moving object would trace if it were to turn while being constrained to the curve. The importance of finding the unit tangent vector function is highlighted as the first step in curvature computation.
📐 Deriving the Unit Tangent Vector for a Helix
The second paragraph delves into the process of finding the unit tangent vector for the helix. It begins by taking the derivative of the parametric equations to obtain the tangent vector. The magnitude of this tangent vector is calculated, simplifying to a constant value due to the properties of sine and cosine functions. The unit tangent vector is then found by dividing the tangent vector by its magnitude. The paragraph emphasizes the simplicity of the resulting unit tangent vector due to the constant magnitude, which does not depend on the parameter t, and sets the stage for further exploration in the subsequent video.
Mindmap
Keywords
💡Curvature
💡Helix
💡Parametric Curve
💡Unit Tangent Vector
💡Derivative
💡Magnitude
💡Arc Length
💡Spaceship
💡Sine and Cosine Functions
💡Normalization
Highlights
The video discusses computing the curvature of a three-dimensional parametric curve, specifically a helix.
The helix is defined with x and y components as cosine and sine of t, and a z component of t/5.
The helix visually appears as a circle from the xy-plane perspective but rises like a spiral staircase in the z direction.
Curvature represents the radius of the circle a spaceship would trace if steering locked while driving along the helix.
The curvature is calculated as the reciprocal of the radius of the traced circle.
The unit tangent vector function is introduced, giving the direction of travel at every point on the helix.
The unit tangent vector is found by differentiating the parameterization and dividing by its magnitude.
The derivative of the parameterization function S'(t) is calculated for each component.
The magnitude of S'(t) simplifies to √(26/25) due to the properties of sine and cosine squared.
The unit tangent vector function is obtained by dividing the derivative by its constant magnitude.
The final unit tangent vector components are -sin(t)/√(26/5), cos(t)/√(26/5), and 1/5.
The magnitude of the derivative is a constant, simplifying the unit tangent vector calculation.
The video concludes with the derivation of the unit tangent vector, with further analysis to be continued in the next video.
The helix serves as a practical example for understanding curvature in three-dimensional space.
The video provides a clear visual representation of the helix and its curvature concept.
The mathematical process of finding the unit tangent vector is explained in a step-by-step manner.
The video emphasizes the importance of understanding the geometric interpretation of curvature.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: