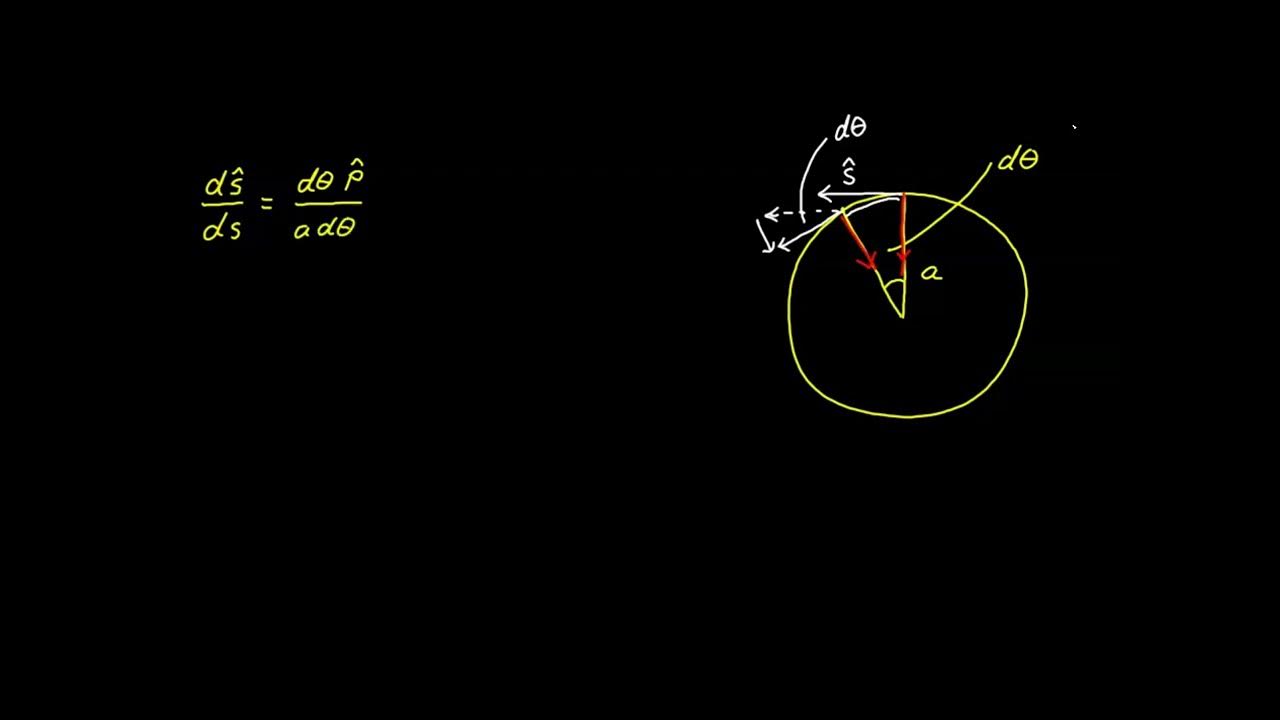Curvature formula, part 3
TLDRThis script explains the concept of the unit tangent vector function in calculus, particularly in the context of parameterized curves. It uses the example of a circle with radius R to illustrate the derivation of the unit tangent vector and its magnitude, which simplifies to R in the case of a circle. The script further delves into the more abstract case, where the simplification does not occur, and introduces the concept of curvature as the derivative of the unit tangent vector with respect to arc length. The formula for curvature is derived and explained, with a promise to explore its intuition and application to non-circular curves in the next video.
Takeaways
- 📚 The video discusses the concept of finding the unit tangent vector function given a parameterization of a curve.
- 🔍 The example provided is a circle with a radius R, but the process is also explained in more abstract terms for general understanding.
- 📐 The unit tangent vector is derived from the derivative of the parameterization and then normalized to ensure it's a unit vector.
- 🔢 For the circle example, the simplification process leads to a unit tangent vector function with magnitude R.
- 🌐 In general, the magnitude of the derivative of the parameterization involves a square root of the sum of squared derivatives, which doesn't always simplify.
- 🤔 The video emphasizes that simplification, as seen in the circle example, is not guaranteed and depends on the specific function.
- 🔍 The next goal after finding the unit tangent vector is to find its derivative with respect to arc length, which is related to curvature.
- 📉 To find curvature, the derivative of the unit tangent vector with respect to the parameter is taken and then divided by the derivative of the arc length function with respect to the parameter.
- 🔄 The process involves understanding how the unit tangent vector changes with respect to a small change in the parameter and correcting for the actual change in arc length.
- 🌀 For the circle, the magnitude of the derivative of the unit tangent vector is shown to be R, which is equal to the radius and thus the curvature is 1/R.
- 📝 The general formula for curvature is derived and involves a complex expression involving first and second derivatives of the parameterization components.
- 🚀 The video concludes with a promise to provide an intuitive explanation for the curvature formula in the next video and to apply it to a non-circular example.
Q & A
What is the purpose of finding the unit tangent vector function in the context of the video?
-The unit tangent vector function is used to determine the direction of the tangent to a curve at any given point, which is essential for calculating properties such as curvature.
How is the unit tangent vector derived from a parameterized curve?
-The unit tangent vector is derived by taking the derivative of the parameterized curve to get the tangent vector, and then normalizing this vector to have a magnitude of one.
What is the significance of normalizing the tangent vector?
-Normalizing the tangent vector ensures that its magnitude is one, which is necessary for the vector to represent direction without any scaling by length.
Why might the magnitude of the derivative not simplify as it did in the case of a circle?
-The magnitude of the derivative may not simplify because it involves the square root of the sum of the squares of the derivatives of the x and y components, which does not always result in a simple expression.
What is the relationship between the unit tangent vector and the curvature of a curve?
-The curvature of a curve is related to the rate of change of the unit tangent vector with respect to arc length. It measures how sharply the curve is bending at a particular point.
How is the derivative of the unit tangent vector with respect to arc length found?
-The derivative of the unit tangent vector with respect to arc length is found by taking the derivative with respect to the parameter, and then dividing by the derivative of the arc length function with respect to the parameter.
What does the magnitude of the derivative of the unit tangent vector represent in the context of curvature?
-The magnitude of the derivative of the unit tangent vector represents the curvature of the curve at a point. It is a measure of how much the tangent vector is changing as one moves along the curve.
Why is the curvature of a circle equal to 1/R, where R is the radius of the circle?
-For a circle, the curvature is equal to 1/R because the circle itself is the curve that most closely hugs the curve at any point, making its radius the measure of how sharply it bends.
What is the general formula for curvature in terms of the derivatives of the parameterized curve?
-The general formula for curvature involves the derivative of the first component of the tangent vector function multiplied by the second derivative of the second component, minus the first derivative of the second component multiplied by the second derivative of the first component, all divided by the three-halves power of the sum of the squares of the first and second derivatives.
Why is it important to understand different ways of thinking about curvature?
-Understanding different ways of thinking about curvature helps in gaining a deeper intuition about the concept and allows for the application of the most suitable method depending on the specific curve being analyzed.
What will be the focus of the next video according to the script?
-The next video will focus on providing an intuition for why the curvature formula makes sense and will include an example of computing the curvature of a curve that is not a circle.
Outlines
📐 Deriving the Unit Tangent Vector
This section discusses finding the unit tangent vector function from a given parameterization, using a circle with radius R as an example. It explains the process of normalizing the tangent vector by dividing it by its magnitude, and highlights that this simplification is specific to the circle. The general formula for the magnitude of the derivative vector is provided, emphasizing the complexity involved in non-circular cases.
📏 Calculating Curvature for a Circle
The focus shifts to finding the curvature by taking the derivative of the unit tangent vector with respect to arc length, s. The explanation includes a detailed breakdown of the process, showing how to derive and normalize the tangent vector function in the specific case of a circle. It concludes with the formula for the magnitude of this derivative, emphasizing its simplicity and elegance in the circle example.
Mindmap
Keywords
💡Unit Tangent Vector
💡Parameterization
💡Derivative
💡Magnitude
💡Normalization
💡Curvature
💡Arc Length
💡Chain Rule
💡Differentiation
💡Radius
💡Simplification
Highlights
Exploring the concept of a unit tangent vector function derived from a parameterization.
Demonstrating the process of finding the unit tangent vector for a circle with radius R.
Abstracting the unit tangent vector concept for a general case.
Normalization of the tangent vector to create the unit tangent vector.
Differentiating between the simplified unit tangent vector of a circle and a more complex general case.
Calculating the magnitude of the derivative to find the unit tangent vector.
The importance of dividing by the magnitude of the tangent vector to achieve unit length.
Understanding the x-component and y-component of the derivative in the context of a unit tangent vector.
The unique simplification that occurs with the unit tangent vector of a circle.
Transitioning to finding the derivative of the unit tangent vector with respect to arc length.
The significance of ds/dt in understanding the change in the unit tangent vector along the curve.
The poetic connection between the magnitude of the derivative and the circle's radius.
Deriving the curvature function for a circle, which is constant and equal to 1/R.
The general formula for curvature involving the derivatives of the tangent vector function.
The complexity of calculating curvature for non-circular curves.
Providing an intuitive understanding of the curvature formula and its components.
Announcing a future video that will delve into the intuition behind the curvature formula.
The three different ways to conceptualize curvature: the closest circle, the change in the unit tangent vector, and the magnitude of its derivative.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: