Introduction to Two Way ANOVA (Factorial Analysis)

statisticsfun
24 Mar 201308:00
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial introduces the concept of two-way ANOVA, also known as factorial analysis, which is used to determine the impact of multiple factors on data variation. The video is part of a playlist covering calculations, interpretation of results, and the use of SPSS and Microsoft Excel for analysis. The example given involves analyzing arithmetic test scores of boys and girls across different age groups to understand the effects of gender and age. The tutorial explains organizing data into a mean table to facilitate calculations and understanding of degrees of freedom, factors, and their interactions. Future videos in the playlist will demonstrate manual calculations, F-ratio computation, and result interpretation, aiming to test the significance of gender, age, and their interaction on student scores.

Takeaways
  • 📚 The tutorial introduces the concept of two-way ANOVA, also known as factorial ANOVA, which is used to analyze the impact of two factors on a variable.
  • 👦👧 The example given is an arithmetic test administered to boys and girls of different age groups (10, 11, and 12 years old) to determine the influence of gender and age on test scores.
  • 🔢 In a one-way ANOVA, the analysis focuses on the average test scores of different age groups, whereas a two-way ANOVA considers both gender and age as factors.
  • 📊 The script explains the process of creating a 'mean table' to organize data by gender and age groups, facilitating the calculation of degrees of freedom and understanding of factors.
  • 🔄 The two-way ANOVA involves reorganizing data in multiple ways to analyze the effects of each factor (gender and age) and their interaction.
  • 📈 The tutorial will cover calculations by hand, using SPSS, and Microsoft Excel for two-way ANOVA in the playlist.
  • 📝 The 'mean table' is essential for organizing data into rows and columns representing different gender and age groups, as well as overall averages.
  • 🔢 The script mentions calculating sums of squares for each factor (gender and age), their interaction, and within the error, which are crucial for the ANOVA analysis.
  • 📉 The total sum of squares is the sum of all individual sums of squares, including the factors, interaction, and error.
  • 📚 The playlist includes videos on calculating the F ratio, degrees of freedom, and interpreting the results of the two-way ANOVA.
  • 🚫 The null hypotheses to be tested are that gender, age, and their interaction have no significant effect on student scores.
  • 📌 The tutorial encourages sharing knowledge and subscribing for more content, indicating the importance of community engagement in learning.
Q & A

  • What is the main topic of the tutorial video?

    -The main topic of the tutorial video is the introduction to two-way ANOVA or factorial analysis.

  • What is the purpose of a two-way ANOVA?

    -The purpose of a two-way ANOVA is to determine the impact of two factors on the variation in a dataset, such as whether gender and age groups affect test scores.

  • How does a two-way ANOVA differ from a one-way ANOVA?

    -A two-way ANOVA is more complex than a one-way ANOVA as it considers the effects of two factors and their interaction, whereas a one-way ANOVA only considers the effects of one factor.

  • What are the two factors considered in the example given in the script?

    -The two factors considered in the example are gender and age groups (10, 11, and 12-year-olds).

  • What is the significance of organizing data in a table in a two-way ANOVA?

    -Organizing data in a table in a two-way ANOVA makes it easier to calculate degrees of freedom, understand the factors, and perform other necessary calculations.

  • What are the hypotheses tested in a two-way ANOVA?

    -The hypotheses tested in a two-way ANOVA are: 1) Gender has no significant effect on student scores, 2) Age has no significant effect on student scores, and 3) The interaction between gender and age has no significant effect on student scores.

  • What does the script mention about the total sum of squares in a two-way ANOVA?

    -The script mentions that the total sum of squares in a two-way ANOVA is the sum of the sum of squares of the first factor (gender), the second factor (age), their interaction, and the error.

  • What is the next step the tutorial will cover after introducing the two-way ANOVA?

    -The next step the tutorial will cover is showing how to make the calculations for a two-way ANOVA by hand.

  • How does the script suggest viewers engage with the content?

    -The script suggests viewers engage with the content by sharing knowledge, showing love on social media platforms, asking questions, and subscribing for more content.

  • What is the significance of the F ratio in the context of ANOVA?

    -The F ratio is used to determine whether to reject or fail to reject the null hypothesis in ANOVA. If the F ratio falls in the rejection region (red area), the null hypothesis is rejected; if it falls in the non-rejection region (green area), the null hypothesis is not rejected.

Outlines
00:00
📊 Introduction to Two-Way ANOVA

This paragraph introduces the concept of two-way ANOVA, also known as factorial ANOVA, as part of a larger playlist dedicated to explaining this statistical method in depth. The tutorial sets up a hypothetical scenario involving an arithmetic test given to different age groups of boys and girls to illustrate the use of two-way ANOVA. It contrasts this with one-way ANOVA, explaining that the two-way method involves analyzing the impact of two factors, gender and age, on test scores. The paragraph also outlines the process of organizing data into a mean table, which includes averages for each age and gender group, facilitating the calculation of degrees of freedom and understanding of the factors involved.

05:04
🔍 Deep Dive into Two-Way ANOVA Calculations

Building on the introduction, this paragraph delves into the specifics of conducting a two-way ANOVA. It discusses the process of reorganizing data to analyze the effects of each factor—age and gender—separately, as well as their interaction. The paragraph explains the calculation of sum of squares for each factor, the interaction between them, and the total sum of squares. It also touches on the upcoming content in the playlist, which will cover how to perform these calculations by hand, calculate the F ratio, determine degrees of freedom, and interpret the results. The ultimate goal is to test three hypotheses regarding the significance of gender, age, and their interaction on student scores, with the F ratio indicating the decision to reject or fail to reject the null hypotheses.

Mindmap
Keywords
💡Two-way ANOVA
Two-way ANOVA, also known as factorial ANOVA, is a statistical method used to analyze the differences between two or more levels of two independent variables on a dependent variable. In the video, it is used to determine if gender and age groups have an impact on arithmetic test scores. The script describes how this method involves analyzing the data in various ways to understand the effects of each factor and their interaction.
💡Factorial Analysis
Factorial Analysis refers to the process of examining the effects of multiple factors on a dependent variable. The video script uses the example of a two-way ANOVA to illustrate how factorial analysis can reveal the influence of gender and age on test scores, beyond what one-way ANOVA could show by looking at only one factor at a time.
💡Arithmetic Test
An Arithmetic Test is a type of assessment used to evaluate a person's mathematical skills in arithmetic. In the script, the arithmetic test is the dependent variable being analyzed to see how it varies with respect to gender and age groups, serving as the basis for the two-way ANOVA.
💡Gender
Gender is one of the independent variables in the study described in the video. It is used to categorize the participants into boys and girls to see if there is a significant difference in their arithmetic test scores. The script mentions that gender, along with age, is a factor in the two-way ANOVA.
💡Age Group
Age Group is another independent variable in the study, with the script mentioning three specific age groups: 10-year-olds, 11-year-olds, and 12-year-olds. The video aims to find out if there is a significant difference in test scores among these age groups.
💡Degrees of Freedom
Degrees of Freedom in statistics refer to the number of values in a data set that are free to vary. In the context of the video, degrees of freedom are important for conducting the two-way ANOVA, as they are used in the calculations to determine the significance of the results.
💡Sum of Squares
Sum of Squares is a statistical term used to quantify the variance or dispersion of data points in a dataset. In the script, the sum of squares is calculated for each factor (gender and age) and their interaction, as well as the total, to perform the two-way ANOVA analysis.
💡F Ratio
The F Ratio is a statistical measure used in ANOVA to determine whether the variance between groups is significantly greater than the variance within groups. The video script mentions that the F ratio will be calculated and used to test the null hypotheses related to gender, age, and their interaction.
💡Null Hypothesis
The Null Hypothesis is a statement of no effect or no difference that is tested in an experiment. In the video, the null hypotheses are that gender, age, and their interaction have no significant effect on student scores. The results of the two-way ANOVA will be used to accept or reject these hypotheses.
💡Interpreting Results
Interpreting Results involves making sense of the data obtained from an analysis. The video script indicates that part of the tutorial series will teach viewers how to interpret the results of a two-way ANOVA, including understanding the significance of the F ratio and its relation to the rejection or acceptance of the null hypotheses.
💡Playlist
A Playlist in the context of the video refers to a collection of related tutorial videos. The script mentions that the tutorial is part of a playlist that covers various aspects of two-way ANOVA, from calculations to result interpretation, and encourages viewers to follow the playlist for a comprehensive understanding.
Highlights

Introduction to the concept of two-way ANOVA or factorial analysis.

The tutorial is part of a playlist covering various aspects of two-way ANOVA from manual calculations to software interpretations.

A scenario is presented where an arithmetic test is given to boys and girls of different age groups to determine the impact on test score variation.

Two factors considered in the analysis are gender and age group.

Explanation of the difference between one-way and two-way ANOVA, highlighting the complexity of the latter.

Demonstration of how to calculate and compare averages in a one-way ANOVA setup.

Description of the process to reorganize data for a two-way ANOVA, including calculating the mean for different subgroups.

Creation of a 'mean table' to facilitate the calculation of degrees of freedom and other necessary calculations.

Differentiation between the first and second factors in a two-way ANOVA, with an example of gender and age.

Explanation of how a two-way ANOVA involves reorganizing data in multiple ways for analysis.

The process of calculating sum of squares for the first factor (age), then for the second factor (gender), and their interaction.

Total sum of squares calculation as part of the two-way ANOVA process.

Upcoming tutorial content on manual calculations for two-way ANOVA.

Future videos will cover calculating the F ratio, degrees of freedom, and interpreting results.

Null hypotheses to be tested: no significant effect of gender, age, or their interaction on student scores.

Guidance on how to share knowledge and engage with the content through social media and subscription.

Transcripts

Browse More Related Video

How To... Perform a One-Way ANOVA Test in SPSS

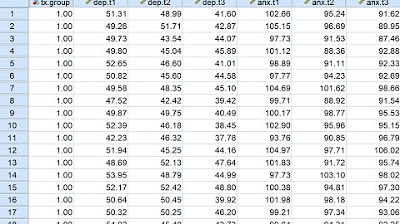
Two-way repeated measures ANOVA in SPSS: one-within, one-between (March 2020)

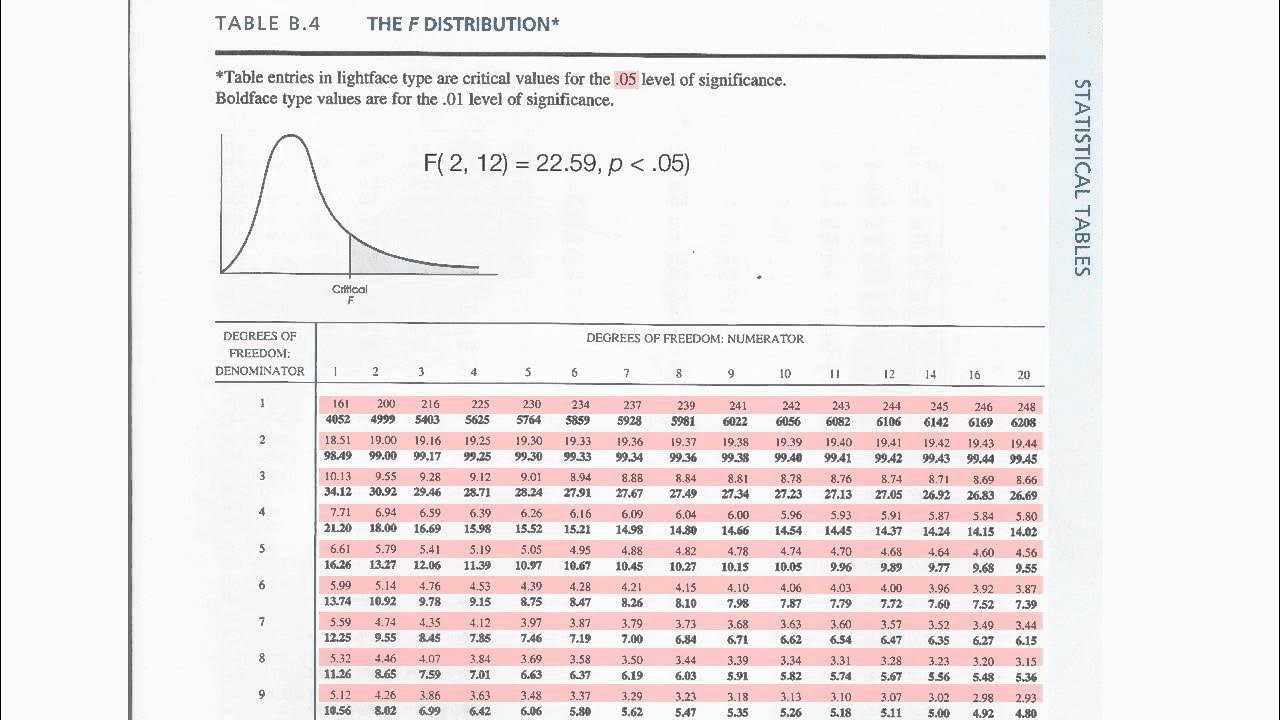
How to read F Distribution Table used in Analysis of Variance (ANOVA)

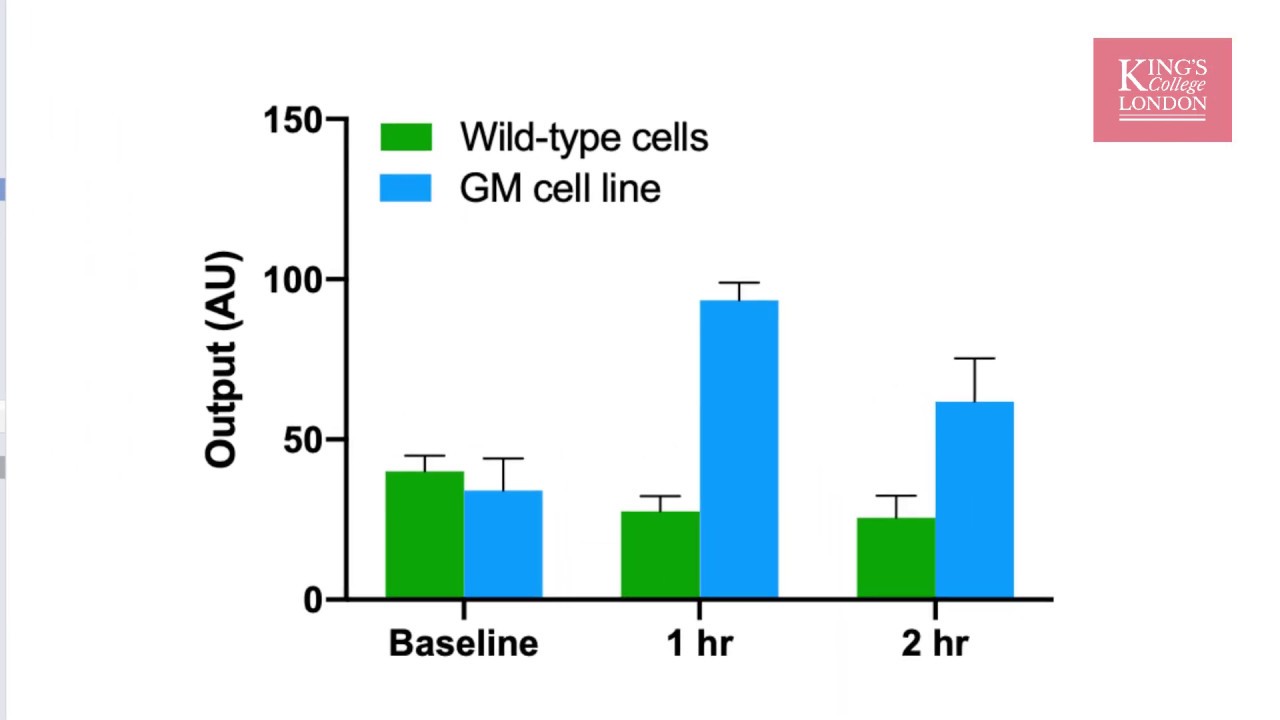
Two-way ANOVA in GraphPad Prism

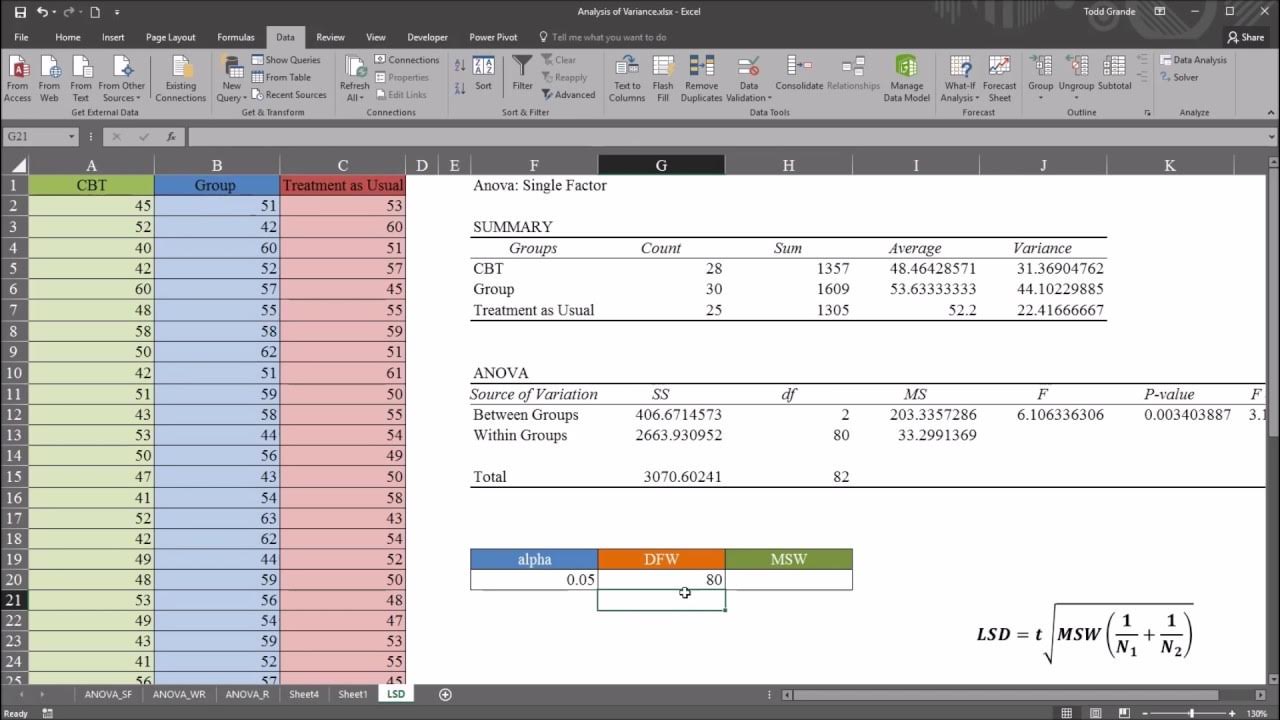
One-Way ANOVA with LSD (Least Significant Difference) Post Hoc Test in Excel

SPSS One-way ANOVA: Post Hoc - Duncan test

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Two-Way ANOVAFactorial AnalysisEducationalTutorial SeriesStatistical MethodsData InterpretationHand CalculationsSPSSExcelPlaylistGender & Age