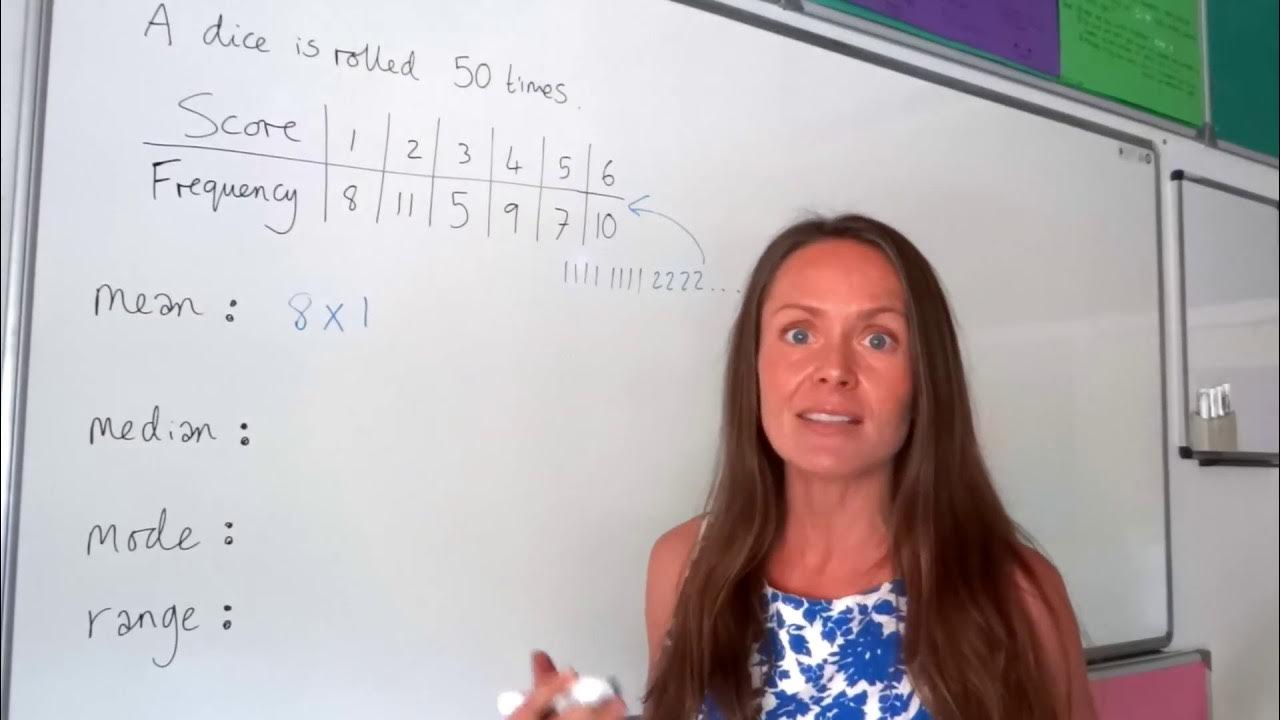Mean, Median, Mode, and Range using Legos

Math In Demand
18 May 202005:30
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video creatively uses Legos to illustrate the concepts of mean, median, mode, and range. The presenter organizes Legos by stud count, calculates the mean by dividing total studs by the number of pieces, determines the median by identifying the middle value in the ordered set, identifies the mode as the most frequent stud count, and calculates the range as the difference between the highest and lowest stud counts. The video also encourages viewers to suggest alternative items for teaching these statistical measures and invites them to subscribe for more math content.

Takeaways
  • 📚 The video demonstrates how to calculate mean, median, mode, and range using Legos as a visual aid.
  • 🔢 The mean is calculated by summing all the studs on the Legos and dividing by the number of Lego pieces.
  • 🎵 The script uses music to transition between different steps of the calculation process.
  • 🔄 The Legos are arranged from least to greatest by the number of studs to facilitate the calculation of median and range.
  • 🏁 The median is the middle value of the ordered Legos, found by removing Legos from the ends until the middle is reached.
  • 📈 The mode is the number that occurs most frequently among the Legos' stud counts.
  • 📊 The range is determined by subtracting the lowest stud count from the highest stud count.
  • 🤔 The video encourages viewers to think of other items that could be used to demonstrate mean, median, mode, and range.
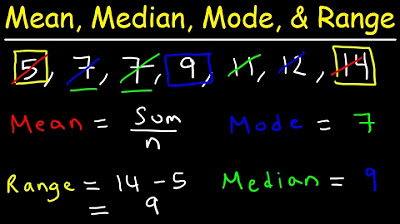
  • 📈 In the first example, the mean is 4, the median is 3, the mode is 2, and the range is 7.
  • 📊 In the second example, the mean is approximately 5.3, the median is 6, the mode is both 4 and 6, and the range is 9.
  • 🔔 The video ends with a call to action for viewers to subscribe for more math videos and to enable notifications.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is explaining the concepts of mean, median, mode, and range using Legos as an example.

  • How does the video demonstrate the process of finding the mean?

    -The video demonstrates finding the mean by adding up all the studs on the Legos and dividing by the total number of Lego pieces to get the average.

  • What is the median in the first example with 12 Lego pieces?

    -In the first example, the median is 3, which is the middle value after arranging the Legos in order from least to greatest.

  • How is the mode identified in the video?

    -The mode is identified by counting the number that occurs the most. In the first example, the mode is 2, as it occurs four times.

  • What is the range in the first example of the video?

    -The range in the first example is 7, which is the difference between the highest value (8) and the lowest value (1).

  • In the second problem, what is the mean of the Lego pieces?

    -In the second problem, the mean is approximately 5.3, obtained by dividing the total number of studs (111) by the number of Lego pieces (21).

  • What is the median in the second example with 21 Lego pieces?

    -In the second example, the median is 6, which is the middle value after arranging the Legos and removing the ones from the outsides.

  • What are the modes in the second example of the video?

    -In the second example, there are two modes: 4 and 6, as both occur six times.

  • What is the range in the second example of the video?

    -The range in the second example is 9, which is the difference between the highest value (10) and the lowest value (1).

  • What does the video encourage viewers to do at the end?

    -The video encourages viewers to think of other items that can be used to demonstrate mean, median, mode, and range, and to subscribe for more math videos and click the bell for notifications.

Outlines
00:00
📚 Introduction to Mean, Median, Mode, and Range with Legos

This paragraph introduces a video that teaches the concepts of mean, median, mode, and range using Legos as a visual aid. The presenter explains the process of arranging Legos by the number of studs, from least to greatest, and then counts these studs to find the total. The mean is calculated by dividing the total number of studs by the number of Lego pieces. The median is identified as the middle value in the ordered set, and the mode is the most frequently occurring number of studs. The range is determined by subtracting the lowest value from the highest. The video demonstrates these calculations with two sets of Legos, showing how to find the mean as 4 and 5.3, the median as 3 and 6, the mode as 2 and both 4 and 6, and the range as 7 and 9, respectively.

05:04
🤔 Exploring Alternatives to Legos for Statistical Concepts

The second paragraph extends an invitation for suggestions on other items that could be used to understand mean, median, mode, and range, besides Legos. It encourages viewers to think creatively about everyday objects that could serve as teaching tools for these statistical measures. The paragraph ends with a reminder for viewers to subscribe for more math-related content and to enable notifications for updates, promising to see them in the next video.

Mindmap
Keywords
💡Mean
Mean, also known as average, is a measure of central tendency that represents the sum of all values divided by the number of values. In the video, the mean is calculated by dividing the total number of studs on all Legos by the number of Lego pieces. For example, with 48 studs and 12 Legos, the mean is 4. This calculation helps to understand the average number of studs on a Lego piece in the collection.
💡Median
Median is another measure of central tendency that identifies the middle value of a data set when it is ordered from least to greatest. In the video, the median is found by removing Legos from the extremes until the central value is reached. For a set of 12 Legos, the median is 3, as it is the middle value between the two remaining Legos after removing the outer ones. This gives a central reference point for the distribution of studs.
💡Mode
Mode refers to the value that appears most frequently in a data set. In the context of the video, the mode is determined by identifying which number of studs occurs the most among the Legos. For instance, the number of studs that appears four times is two, making it the mode. This indicates the most common stud count among the Lego pieces.
💡Range
Range is the difference between the highest and lowest values in a data set, providing a measure of dispersion or spread. In the video, the range is calculated by subtracting the lowest stud count (1) from the highest (8), resulting in a range of 7. This shows the extent of variation in the number of studs across the Lego pieces.
💡Lego
Lego is a brand of construction toys that are used in the video as a practical and visual aid to demonstrate statistical concepts. The Legos are sorted by the number of studs on top, serving as a tangible example for explaining statistical measures such as mean, median, mode, and range.
💡Studs
Studs are the small protruding dots on the top of Lego pieces that allow them to interlock with each other. In the video, the number of studs is used as a variable to calculate statistical measures. The count of studs on each Lego is a key factor in determining the mean, median, mode, and range of the collection.
💡Ordered
Ordered, in the context of the video, refers to arranging the Legos from least to greatest based on the number of studs. This ordering is essential for finding the median and understanding the distribution of the data set. For example, the Legos are ordered to easily identify the middle value for calculating the median.
💡Measure of Central Tendency
Measures of central tendency are statistical measures that describe the center of a data set. The video discusses three such measures: mean, median, and mode. These measures provide different perspectives on the typical value within a data set of Lego studs.
💡Data Set
A data set in the video refers to the collection of Lego pieces with their respective number of studs. Each Lego and its stud count is a data point within the set, which is then used to calculate various statistical measures.
💡Decimal
Decimal, as mentioned in the video, is a numerical value that can represent a part of a whole, indicated by a decimal point. When calculating the mean with 111 studs and 21 Legos, the result is a decimal (5.3), which is then rounded to the nearest whole number for simplicity.
💡Contextualize
Contextualize, in the context of this task, means to provide explanations that are not only definitions but also show how the terms relate to the video's narrative. For example, explaining how the term 'mean' is used to find the average stud count on Legos in the video.
Highlights

Introduction to the educational video using Legos to illustrate statistical concepts.

Explanation of the process to determine the mean by counting studs on Legos and dividing the total by the number of pieces.

Demonstration of finding the mean, which is calculated to be 4 for the first set of Legos.

Description of how to find the median by ordering Legos and identifying the middle value.

Reveal that the median for the first set of Legos is 3, determined by the middle value between two Legos.

Introduction to the mode as the most frequently occurring number in a set, shown with the number 2 occurring four times.

Calculation of the range as the difference between the highest and lowest values, resulting in 7 for the first set.

Presentation of a second problem with a larger set of Legos to illustrate the statistical concepts.

Calculation of the mean for the second set of Legos, which rounds to approximately 5.3.

Identification of the median for the second set as 6, the single middle value after ordering the Legos.

Introduction of the concept of multiple modes, with both 4 and 6 occurring six times each in the second set.

Explanation of calculating the range for the second set, which is 9, the difference between the highest and lowest values.

Engagement with the audience by asking for suggestions of other items that could be used to teach mean, median, mode, and range.

Encouragement for viewers to subscribe for more math videos and to enable notifications for updates.

Anticipation of the next video in the series, creating a sense of continuity and engagement.

Transcripts

Browse More Related Video

Mean, Median, Mode, and Range | Math with Mr. J

Finding Mean, Median, and Mode | Math with Mr. J

Mean, Median and Mode - Measures of Central Tendency

Math Antics - Mean, Median and Mode

The Maths Prof: Frequency Tables (Mean, Median, Mode & Range)

Mean, Median, Mode, and Range - How To Find It!

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Mean CalculationMedian ValueMode FrequencyRange DifferenceLego MathEducational VideoMath ConceptsStatistical MeasuresLearning ToolsEngaging ContentMathematics Tutorial