math 119 Chapter 9 part 2

Brad Bolton

28 Jan 202133:17

EducationalLearning

32 Likes 10 Comments

TLDRThis video script covers the concept of independent and dependent samples in statistical analysis, focusing on their definitions and applications in hypothesis testing. The instructor explains how to determine if samples are independent or dependent, using examples from medical studies. The script also discusses inferences about two means, including the use of two-sample t-tests and confidence intervals for estimating differences between population means. Practical examples, such as the comparison of nicotine levels in filtered and non-filtered cigarettes, are provided to illustrate the statistical methods and their conclusions.

Takeaways

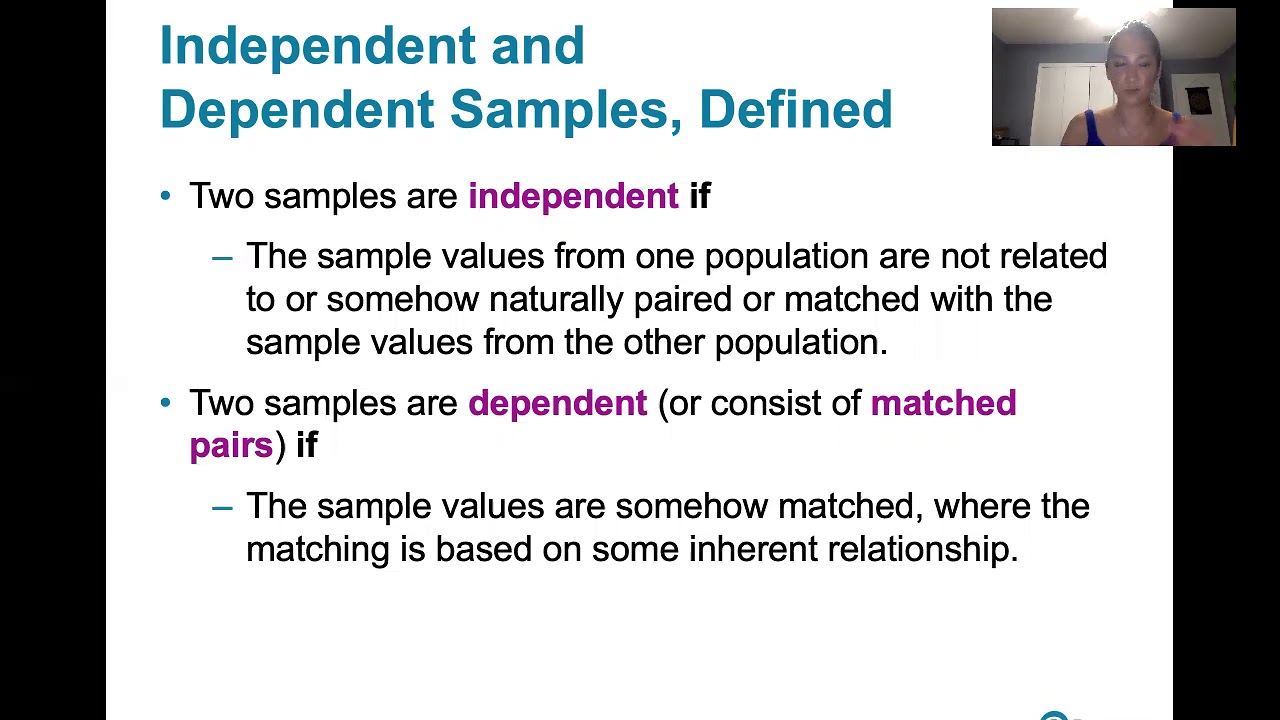
📚 The lecture discusses two types of samples: independent and dependent. Independent samples are not related, while dependent samples are matched or paired.
🔍 A quick way to identify dependent samples is when the same group is tested twice, such as in medical studies where blood pressure is measured before and after medication.
📈 The section on inferences about two means uses independent samples to test claims about differences between population means or to construct confidence intervals.
📝 Requirements for using the two-sample t-test include having independent random samples, and either both groups are larger than 30 or both come from normally distributed populations with unknown and not necessarily equal standard deviations.
🧮 The formula for the two-sample t-test is complex and typically done using a calculator, focusing on comparing the means of the two independent samples when the standard deviations are unknown.
💊 An example of a two-sample t-test is given involving a study on the effectiveness of paroxetine for treating bipolar depression, comparing the mean depression levels between a placebo and a drug group.
📉 The p-value obtained from the calculator is used to determine whether to reject or fail to reject the null hypothesis, with a p-value greater than the significance level indicating insufficient evidence to support the claim.
🚬 Another example involves comparing the mean nicotine levels of filtered versus non-filtered king-sized cigarettes, demonstrating the use of a two-sample t-test with a significant result.
🔑 The importance of understanding the difference between p-values and population proportions is emphasized, as 'p' is used in various contexts within statistics.
🌐 The script concludes with a discussion on confidence intervals for independent means, explaining that if zero is within the interval, it suggests no significant difference between the two means.
🔚 The final part of the script hints at upcoming topics, including inferences about two means with matched pairs, and notes that the course is nearing completion with a few more videos to be released.

Q & A

What are the two main types of samples discussed in the script?
-The two main types of samples discussed are independent samples and dependent (or matched pairs) samples. Independent samples are not related or paired with each other, while dependent samples are where the members of one sample can be used to determine members of the other sample.
How can you quickly recognize dependent samples in practical scenarios?
-Dependent samples can be quickly recognized in scenarios like medical studies where the same group of subjects is tested before and after an intervention, such as taking medication. For example, measuring blood pressure before and after administering medication to the same individuals indicates a matched pair design.
What are the conditions required for inferences about two means using independent samples?
-The conditions for inferences about two means using independent samples include that both samples are random samples, and either the group is larger than 30 or both samples come from normally distributed populations with unknown and not necessarily equal standard deviations.
Why is it safer to use unpooled data in a two-sample t-test when the standard deviations are unknown?
-Using unpooled data is considered safer because it does not assume that the standard deviations of the two populations are equal. Pooling data can lead to a loss of accuracy if the assumption of equal standard deviations is incorrect, whereas using unpooled data is a bit more conservative and less likely to result in inaccurate conclusions.
What is the significance of the p-value in hypothesis testing?
-The p-value indicates the probability of observing the test results under the null hypothesis. A small p-value suggests that it is unlikely the observed results occurred by chance under the null hypothesis, leading to the rejection of the null hypothesis. A p-value greater than the significance level (alpha) means the null hypothesis is not rejected.
How does the script differentiate between the use of 'p' for population proportion and 'p' for p-value?
-The script clarifies that 'p' can have multiple meanings in statistics, including population proportion, an estimate of the population proportion (p-hat), probability, and the p-value. It emphasizes that the p-value from a calculator is not the same as the population proportion.
What is the conclusion when the calculated p-value is greater than the significance level (alpha) in a hypothesis test?
-When the p-value is greater than the significance level (alpha), it means there is insufficient evidence to support the alternative hypothesis, and the null hypothesis is not rejected.
Can you explain the concept of a two-sample t-test and when it is used?
-A two-sample t-test is used to compare the means of two independent groups to determine if there is a significant difference between them. It is used when the standard deviations are unknown and the samples may not be from normally distributed populations or when the sample sizes are small.
What is the purpose of constructing a confidence interval for the difference between two means?
-The purpose of constructing a confidence interval for the difference between two means is to estimate the range within which the true difference between the population means is likely to fall. It provides an interval estimate rather than a point estimate, offering a measure of uncertainty around the difference.
How does a confidence interval help in understanding the results of a study comparing two groups?
-A confidence interval helps in understanding whether there is a significant difference between the two groups. If zero is not within the confidence interval for the difference between the means, it suggests that there is a significant difference between the two groups. If zero is within the interval, it indicates that there may not be a significant difference.
What is the implication of zero being within a confidence interval for the difference between two means?
-If zero is within the confidence interval for the difference between two means, it implies that there is a possibility that the two means are not significantly different from each other. This does not prove they are the same, but it does not provide enough evidence to claim a significant difference.
How does the script illustrate the application of hypothesis testing and confidence intervals in real-world scenarios?
-The script uses examples such as medical studies, cigarette nicotine content, age discrimination in promotions, and weight loss effectiveness of diets to illustrate how hypothesis testing and confidence intervals are applied to analyze data and draw conclusions in real-world scenarios.

Outlines

00:00

📚 Introduction to Independent and Dependent Samples

The script begins with an introduction to the concepts of independent and dependent samples in statistical analysis. It explains that independent samples are not related to each other, while dependent samples are related or matched, often used in medical studies where the same subjects are measured twice. The example of measuring blood pressure before and after medication is given to illustrate dependent samples. The script also discusses the statistical requirements for making inferences about two means, emphasizing that both samples should be random and either both should be larger than 30 or come from normally distributed populations with unknown standard deviations.

05:02

🔍 Two-Sample T-Test for Independent Samples

This paragraph delves into the specifics of the two-sample t-test for independent samples, which is used to test claims about the differences between two population means. The script outlines the prerequisites for using this test, including the random sampling and normal distribution of populations. It also explains the process of using a calculator to compute the test statistic, avoiding the complex formula by hand, and emphasizes the importance of identifying whether to use pooled or unpooled data based on the similarity of standard deviations. The paragraph concludes with an example of a study comparing the effectiveness of a medication using a two-sample t-test.

10:06

📉 Hypothesis Testing for Mean Differences

The script continues with a detailed explanation of hypothesis testing for mean differences between two groups. It describes the process of stating the null hypothesis (μ1 = μ2) and the alternative hypothesis (μ1 > μ2), setting the significance level (α), and computing the test statistic using a calculator. The example of a study comparing a placebo group to a drug group for depression levels is used to illustrate the process. The script also discusses the interpretation of the p-value and the decision to reject or fail to reject the null hypothesis based on the comparison of the p-value to the significance level.

15:06

🚭 Testing Claims About Cigarette Nicotine Levels

This paragraph presents a practical application of hypothesis testing to compare the mean nicotine levels between filtered and non-filtered king-sized cigarettes. The script guides through the process of stating the claim, setting up the null and alternative hypotheses, determining the significance level, and using a calculator to compute the test statistic and p-value. The extremely small p-value obtained leads to the rejection of the null hypothesis, supporting the claim that filtered cigarettes have a lower mean nicotine level than non-filtered ones.

20:10

📊 Confidence Intervals for Independent Means

The script introduces the concept of confidence intervals for estimating the difference between two independent means when the population standard deviations are unknown. It explains the formula for calculating the confidence interval and emphasizes the use of a calculator for this purpose. The script also discusses the interpretation of confidence intervals in the context of hypothesis testing, using an example of age discrimination in promotions. The inclusion or exclusion of zero in the confidence interval indicates whether there is a significant difference between the two means.

25:17

🍽 Comparing Diet Effectiveness Through Confidence Intervals

This paragraph demonstrates how to use confidence intervals to compare the effectiveness of two diets in terms of weight loss. The script outlines the process of calculating a 95% confidence interval for the difference in mean weight loss between the Weight Watchers and Atkins diets. The result shows that zero is not included in the confidence interval, indicating a significant difference in the average weight loss between the two diets.

30:23

📈 Conclusion of Chapter Nine and Course Update

The final paragraph wraps up the discussion on inferences about two means and provides an update on the course schedule. The script mentions that the next section will cover inferences about two means for matched pairs and indicates that there are a few more videos to be released before the end of the semester. It also informs the students about the upcoming exam and final course assessment, signaling that the course is nearing completion.

Mindmap

Keywords

💡Independent Samples

Independent samples refer to data collected from two separate groups where the selection of values from one group does not influence the selection from the other. This concept is crucial in the video's theme of statistical analysis, as it underpins the methodology for comparing two groups without bias. For instance, the script mentions that samples are independent if they are not related or paired, contrasting with dependent samples where members of one sample can determine members of the other.

💡Dependent Samples

Dependent samples, also known as matched pairs, are groups where the selection from one is related to or can be used to determine the selection from the other. This is a key concept in the video as it explains how certain studies, such as medical trials, use the same subjects at different times to measure changes. The script provides an example of blood pressure measurements taken before and after medication, illustrating dependent sampling.

💡Two-Sample T-Test

A two-sample t-test is a statistical method used to determine if there is a significant difference between the means of two independent groups. The video script discusses this concept extensively, explaining its use when the standard deviations of the groups are unknown. The t-test is central to the video's narrative on comparing group means and making inferences about population parameters.

💡Significance Level (Alpha)

The significance level, often denoted as alpha, is the probability of rejecting the null hypothesis when it is true. In the video, alpha is set at 0.05, which means there is a 5% chance of concluding a difference when there is none. This concept is integral to hypothesis testing and is used to determine the strength of evidence against the null hypothesis.

💡Null Hypothesis

The null hypothesis is a statement of no effect or no difference between groups and serves as a basis for statistical testing. In the video, the null hypothesis is often stated as the equality of means between two groups (mu1 = mu2). It is a fundamental concept in the video's discussion of hypothesis testing and is used to establish a benchmark for comparison against the alternative hypothesis.

💡Alternative Hypothesis

The alternative hypothesis is a statement that contradicts the null hypothesis, suggesting that there is an effect or a difference between groups. The video script uses the alternative hypothesis to frame research questions and to determine if there is sufficient evidence to reject the null hypothesis. For example, the script mentions an alternative hypothesis stating that the mean depression level of a placebo group is greater than that of a drug group.

💡Confidence Interval

A confidence interval provides a range of values within which the true population parameter is likely to fall with a certain level of confidence. The video discusses constructing confidence intervals for the difference between two means, which is a key method for estimating and interpreting the range of an effect. The script illustrates this with examples, such as comparing the mean age of promoted versus non-promoted workers.

💡P-Value

The p-value is the probability of observing the data (or something more extreme) if the null hypothesis were true. In the video, the p-value is used to assess the evidence against the null hypothesis. A high p-value (greater than alpha) indicates insufficient evidence to reject the null, while a low p-value suggests strong evidence against the null, as demonstrated in the cigarette nicotine example.

💡Standard Deviation

Standard deviation is a measure of the amount of variation or dispersion in a set of values. In the video, standard deviations of different groups are used in the calculation of the two-sample t-test and confidence intervals. The script mentions that standard deviations are often unknown and not assumed to be equal, which is a common scenario in statistical analysis.

💡Hypothesis Testing

Hypothesis testing is a process of making decisions about a population parameter based on a sample statistic. The video script explains the steps of hypothesis testing, including stating the null and alternative hypotheses, determining the significance level, calculating the test statistic, and making a conclusion based on the p-value. The concept is central to the video's educational content on statistical inference.

Highlights

Introduction to the second video for Chapter Nine focusing on two important types of samples: independent and dependent.

Definition of independent samples as those selected from one population not related to the other.

Dependent samples are those where members of one sample can determine members of the other, often used in medical studies.

Explanation of how to quickly recognize dependent versus independent samples in practical scenarios.

Requirements for inferences about two means using independent samples, including random sampling and normal distribution assumptions.

Clarification on the use of calculators for complex formulas in two-sample t-tests, emphasizing the importance of not assuming equal standard deviations.

Description of a study design to test the effectiveness of paroxetine for treating bipolar depression using a two-sample t-test.

Step-by-step guide on setting up a hypothesis test for two-sample means, including null and alternative hypotheses.

Use of a significance level in hypothesis testing and the importance of understanding the direction of the test (one-tailed or two-tailed).

Process of computing the test statistic using a calculator for a two-sample t-test and interpreting the results.

Interpretation of a p-value in the context of hypothesis testing and its comparison with the significance level.

Example of applying hypothesis testing to determine if a drug is effective in treating depression, using a placebo group for comparison.

Discussion on the implications of failing to reject the null hypothesis in the context of medical studies and funding.

Introduction to confidence intervals for estimating the difference between two independent means.

Explanation of how confidence intervals can indicate the presence or absence of a difference between two means.

Case study on age discrimination in promotions, using confidence intervals to analyze potential bias.

Application of confidence intervals to compare the effectiveness of two diets in a randomized trial.

Conclusion on the importance of understanding statistical methods for hypothesis testing and confidence intervals in practical research scenarios.

Transcripts

Browse More Related Video

9.2.0 Two Means, Independent Samples - Lesson Overview, Learning Outcomes, Key Concepts

Elementary Stats Lesson # 21

9.2.1 Two Means, Independent Samples - Independent and Dependent Samples

Math 119 Chapter 9 part 3

9.2.5 Two Means, Indep. Samples - Three Additional Cases for Hypothesis Tests, Confidence Intervals

SPSS (9): Mean Comparison Tests | T-tests, ANOVA & Post-Hoc tests

Related Tags

Statistics Research Methods Sample Types Dependent Samples Independent Samples Hypothesis Testing Confidence Intervals T-test Medical Studies Data Analysis Educational Content