9.2.0 Two Means, Independent Samples - Lesson Overview, Learning Outcomes, Key Concepts

Sasha Townsend - Tulsa
22 Nov 202005:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script offers an overview of Lesson 9.2, focusing on statistical inferences about two means using independent samples. It is based on the sixth edition of 'Essentials of Statistics' by Mario Triola, modified for the course. The lesson introduces key concepts, including testing claims about population means through p-value, critical value, and confidence interval methods. It also covers the distinction between dependent and independent samples, crucial for selecting the right statistical tools, and outlines alternate methods for different scenarios, such as when population standard deviations are unknown or assumed equal.

Takeaways
  • 📚 The video provides an overview of Lesson 9.2 from the textbook 'Essentials of Statistics' by Mario Triola, focusing on making inferences about two means from independent samples.
  • 🔑 Lesson 9.1, which covers inferences about two proportions, was discussed previously, and the current lesson focuses on inferences about two means with independent samples.
  • 🚫 The class will not cover inferences about two means with dependent samples from the textbook, even though it is available for self-study.
  • 📈 The lesson is divided into five learning outcomes, starting with understanding the difference between dependent and independent samples and choosing the right statistical tools accordingly.
  • 📊 The video script discusses the rationale behind the formulas and procedures for the p-value method, critical value method, and confidence interval method, all related to the sampling distribution of a sample mean.
  • 📝 The script outlines equivalent methods for testing claims about two sample means when the population standard deviations are unknown and not assumed to be equal.
  • 🔍 The p-value method is demonstrated with an example problem to test a claim about two means using data from two independent samples.
  • 📉 The critical value method is introduced for testing claims about two means when population standard deviations are unknown and not assumed equal, using a similar scenario as the p-value method.
  • 📋 The confidence interval method is used to construct an estimate of the difference between two population means and to test claims under the same conditions as the previous methods.
  • 🛠 Alternate methods for testing claims or constructing confidence intervals are discussed for situations where the standard deviations are unknown but assumed equal, one is known and the other is not, or both are known.
  • 🔑 The video aims to make students aware of the various methods available for statistical analysis, even if not all will be covered in class, emphasizing the importance of understanding the context of the data to choose the appropriate method.
Q & A

  • What is the main topic of Lesson 9.2 in the textbook 'Essentials of Statistics' by Mario Triola?

    -The main topic of Lesson 9.2 is making inferences about two means, specifically when dealing with two independent samples.

  • What is the difference between the content covered in Lesson 9.1 and Lesson 9.2?

    -Lesson 9.1 is about inferences about two proportions, while Lesson 9.2 focuses on inferences about two means with two independent samples.

  • Why is it important to distinguish between dependent and independent samples in statistical analysis?

    -It is important to distinguish between dependent and independent samples because it determines which statistical analysis tools are appropriate for the data at hand.

  • What are the three methods mentioned for testing a claim about two population means?

    -The three methods mentioned are the p-value method, the critical value method, and the confidence interval method.

  • What is the purpose of constructing a confidence interval estimate of the difference between two population means?

    -The purpose is to provide an estimate of the range within which the true difference between the two population means is likely to fall, with a certain level of confidence.

  • How is the rationale behind the p-value method, critical value method, and confidence interval method related to the sampling distribution?

    -The rationale behind these methods is based on the understanding of the sampling distribution of a sample mean and how it translates to the distribution of the difference between two sample means.

  • What is the significance of knowing whether the population standard deviations are known or unknown in the context of the lesson?

    -Knowing whether the population standard deviations are known or unknown is significant because it affects the choice of method for testing a claim about two sample means or constructing a confidence interval estimate.

  • What are the learning outcomes of Lesson 9.2 as outlined in the video script?

    -The learning outcomes include understanding the difference between dependent and independent samples, the rationale behind the statistical methods, the equivalent methods for testing a claim when population standard deviations are not known, testing a claim about two means using different methods, and understanding alternate methods when certain requirements are not met.

  • Why might the methods for testing a claim about two means change if the population standard deviations are assumed to be equal or if one is known and the other is not?

    -The methods change because different assumptions about the population standard deviations lead to different statistical formulas and procedures for calculating confidence intervals or testing hypotheses.

  • What is the purpose of discussing alternate methods for testing a claim about two population means in the last video of the lesson?

    -The purpose is to inform students about the different methods that can be used under various circumstances, such as when the population standard deviations are unknown but assumed to be equal, or when one is known and the other is not.

  • How does the video script suggest students should approach learning about the different cases for making inferences about two means?

    -The script suggests that students should be aware of the different cases and methods available for making inferences about two means, even if they are not covered in class, and encourages them to explore these in the textbook on their own.

Outlines
00:00
📚 Introduction to Lesson 9.2 on Inferences about Two Means

This paragraph introduces the video's focus on Lesson 9.2 from 'Essentials of Statistics' by Mario Triola, which discusses making inferences about two means using independent samples. The video is based on Pearson's slides modified for the course. It clarifies that while Lesson 9.1 covered inferences about two proportions, this lesson will delve into inferences about two means for independent samples. The instructor also mentions a section in the textbook about dependent samples that won't be covered in the class but encourages students to explore it independently. The paragraph outlines the lesson's key concepts, including testing claims about population means using different statistical methods and constructing confidence intervals for the difference between population means. It also introduces the lesson's five learning outcomes, starting with understanding the difference between dependent and independent samples and the importance of this distinction in choosing the right statistical tools.

05:02
🔍 Exploring Statistical Methods for Independent Samples

The second paragraph delves into the specifics of the statistical methods to be discussed in the video for analyzing independent samples. It outlines the rationale behind the formulas and procedures for the p-value method, critical value method, and confidence interval method, emphasizing the importance of understanding the sampling distribution of a sample mean and its application to the distribution of the difference between two sample means. The paragraph also mentions equivalent methods for testing claims about two sample means when the population standard deviations are unknown and not assumed to be equal. The instructor plans to demonstrate these methods using an example problem in the video. Additionally, the paragraph briefly mentions alternate methods for testing claims or constructing confidence intervals when certain conditions are not met, such as when the population standard deviations are known or assumed to be equal, or when one is known and the other is not.

Mindmap
Keywords
💡Inferences about two means
This term refers to statistical methods used to make conclusions about the difference between the means of two groups. It is central to the video's theme as it introduces the subject of the lesson, which is to analyze whether there is a significant difference between the means of two independent samples. The script discusses this in the context of using different statistical methods such as the p-value method, critical value method, and confidence interval method.
💡Independent samples
Independent samples are groups of data collected from two separate populations where the selection of individuals in one sample does not affect the selection in the other. The video emphasizes the importance of distinguishing between independent and dependent samples because it dictates which statistical tests are appropriate. For instance, the script mentions that if the samples are independent, the methods described in lesson 9.2 can be applied.
💡Dependent samples
Dependent samples, also known as paired samples, are data collected in such a way that each observation in one group can be paired with a specific observation in the other group. The video script explains that if samples are dependent, different statistical methods, such as those in lesson 9.3, should be used. This concept is crucial for choosing the right statistical tools for analysis.
💡P-value method
The p-value method is a statistical technique used to test a hypothesis by determining the probability of obtaining results as extreme as the observed data, assuming the null hypothesis is true. The video script outlines this method as one of the ways to make inferences about two means, highlighting its role in hypothesis testing within the context of independent samples.
💡Critical value method
The critical value method involves comparing the test statistic of a sample to a critical value from a statistical distribution to determine if the results are statistically significant. The script refers to this method as another approach to test claims about two population means when dealing with independent samples.
💡Confidence interval method
A confidence interval method provides a range of values that are likely to contain an unknown population parameter with a certain level of confidence. The video script discusses this method for estimating the difference between two population means, emphasizing its use in constructing confidence intervals for the difference between means of independent samples.
💡Population mean
A population mean refers to the average value of a particular variable for an entire population. In the context of the video, the script talks about making inferences about two population means, which involves estimating and comparing the average values of two different groups to determine if there is a significant difference between them.
💡Sample mean
A sample mean is the average of the values in a sample, used as an estimate for the population mean. The video script discusses the sampling distribution of a sample mean, which is foundational for understanding how to make inferences about the difference between two sample means.
💡Sampling distribution
The sampling distribution is the probability distribution of a given statistic based on a random sample. The script explains that the rationale behind the formulas and procedures for the p-value, critical value, and confidence interval methods is based on understanding the sampling distribution, especially the distribution of the difference between two sample means.
💡Population standard deviations
Population standard deviations refer to the measure of dispersion or variability within an entire population for a particular variable. The video script mentions that the methods for making inferences about two means can change depending on whether the population standard deviations are known or unknown and whether they are assumed to be equal.
💡Hypothesis testing
Hypothesis testing is a statistical method used to make decisions about populations based on sample data. The script outlines the process of hypothesis testing in the context of inferences about two means, explaining how to use the p-value method, critical value method, and confidence interval method to test claims about population means.
Highlights

Overview of Lesson 9.2 on making inferences about two means from the textbook 'Essentials of Statistics' by Mario Triola.

Lesson 9.2 focuses on inferences about two means with two independent samples, contrasting with Lesson 9.1 on inferences about two proportions.

The class will not cover inferences about two means with dependent samples from the textbook this semester.

Key concepts include testing claims about population means and constructing confidence intervals for the difference between two population means.

Lesson divided into five learning outcomes, starting with understanding the difference between dependent and independent samples.

Importance of determining sample dependency for selecting appropriate statistical analysis tools.

Explanation of the rationale behind the p-value method, critical value method, and confidence interval method.

Discussion on the sampling distribution of a sample mean and its relation to the distribution of the difference between two sample means.

Equivalent methods for testing claims about two sample means when population standard deviations are unknown and not assumed equal.

Demonstration of the p-value method using an example problem with two independent samples.

Introduction to the critical value method for testing claims about two means with unknown and not equal population standard deviations.

Confidence interval method for estimating the difference between two population means under the same circumstances.

Description of alternate methods for testing claims or constructing confidence intervals when specific requirements aren't met.

Three cases discussed for handling situations with unknown but assumed equal population standard deviations, known but not equal, and both known.

Awareness of different methods available for data analysis even if not covered in class.

Final video of the lesson will discuss the first learning outcome about the distinction between dependent and independent samples.

Transcripts

Browse More Related Video

9.1.0 Two Proportions - Lesson Overview, Key Concepts, Learning Outcomes

9.2.5 Two Means, Indep. Samples - Three Additional Cases for Hypothesis Tests, Confidence Intervals

math 119 Chapter 9 part 2

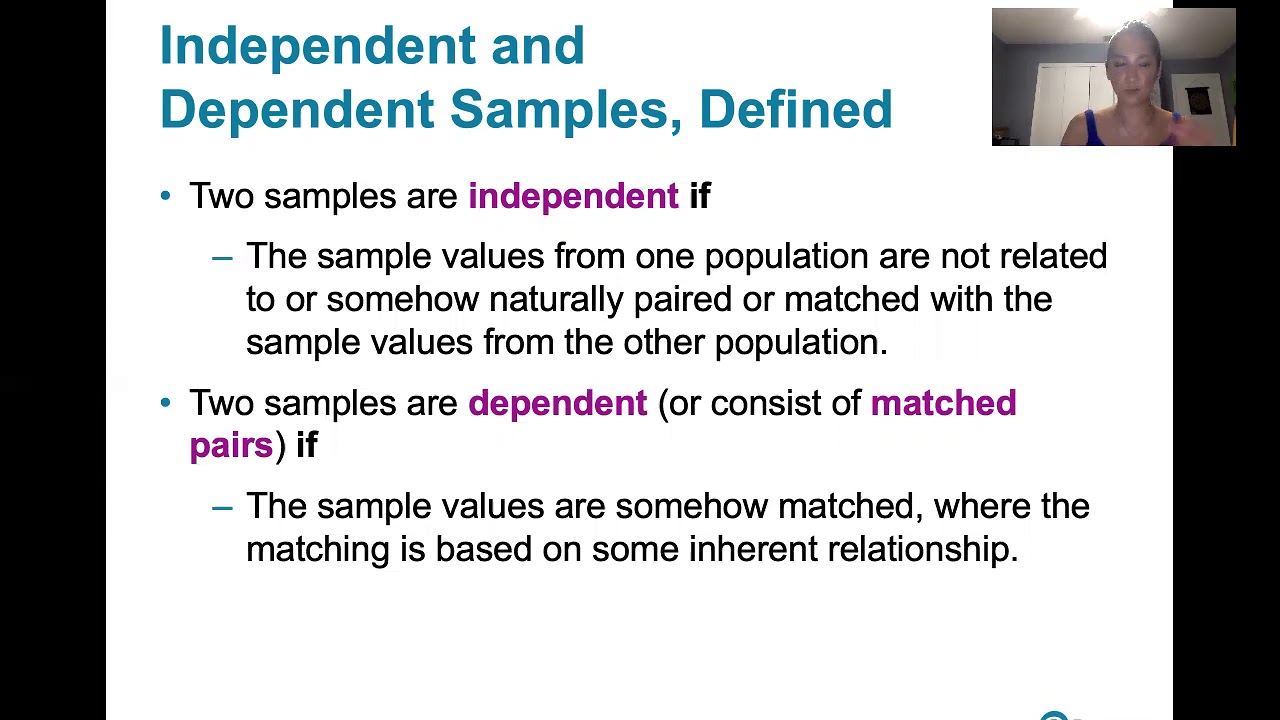
9.2.1 Two Means, Independent Samples - Independent and Dependent Samples

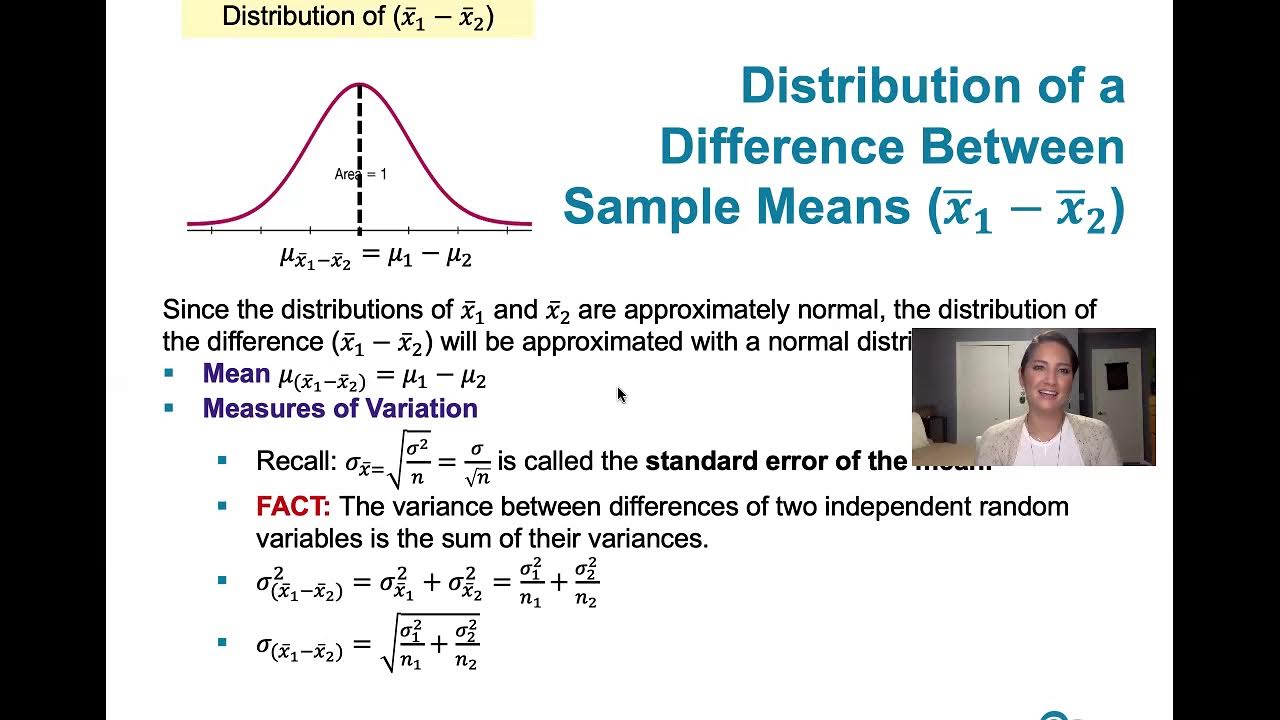
9.2.2 Two Means, Independent Samples - The Rationale Behind The Equivalent Methods, P-value Method

9.1.5 Two Proportions - Hypothesis Testing and Confidence Intervals When Requirements Are Not Met

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
StatisticsInferenceMeansIndependent SamplesEducationalLesson 9.2Mario TriolaEssentialsTextbookP-ValueConfidence Interval