7.2.3 Estimating a Population Mean - Finding X-Bar and Margin of Error, Given a Confidence Interval

Sasha Townsend - Tulsa
30 Oct 202005:16
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial explains how to determine the margin of error and the point estimate for a population mean, given a confidence interval. It clarifies that the point estimate is the sample mean, found by averaging the upper and lower limits of the interval. The margin of error is half the interval's length, calculated by subtracting the lower limit from the upper and dividing by two. An example illustrates the process using a 90% confidence interval for weight loss, where the sample mean and margin of error are calculated to be 2.1 pounds and 1.3 pounds, respectively.

Takeaways
  • πŸ“š The script discusses how to find the margin of error and point estimate for a population mean given a confidence interval.
  • πŸ” The point estimate of the population mean is the sample mean, calculated as the average of the upper and lower limits of the confidence interval.
  • πŸ“‰ To find the point estimate, add the upper and lower limits of the confidence interval and divide by two.
  • πŸ“ˆ The margin of error is the distance from the sample mean to the confidence interval limits, and it can be found by taking half the interval's length.
  • πŸ“ The script explains that the confidence interval is computed by adding and subtracting the margin of error from the sample mean.
  • 🧐 The confidence level, such as 90%, indicates the probability that the true population mean lies within the interval.
  • πŸ€” The script uses an example of a weight loss program to illustrate how to calculate the sample mean and margin of error from a given confidence interval.
  • πŸ“Š In the example, the 90% confidence interval for mean weight loss is between 0.8 and 3.4 pounds, suggesting the true mean lies within this range.
  • βš–οΈ The sample mean weight loss for the 40 adults in the study is calculated to be 2.1 pounds after one year.
  • πŸ“ The margin of error for the confidence interval is found to be 1.3 pounds, which is half the difference between the upper and lower limits.
  • πŸ”„ The script emphasizes that the margin of error can be verified by subtracting the lower limit from the upper limit and dividing by two.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is explaining how to find the margin of error and the point estimate, specifically the sample mean (x bar), for a population mean given a confidence interval.

  • What is the point estimate of the population mean?

    -The point estimate of the population mean is the sample mean, which is the average of the upper and lower limits of the confidence interval.

  • How is the sample mean calculated from the confidence interval?

    -The sample mean is calculated by taking the arithmetic mean of the upper and lower limits of the confidence interval, which is done by adding the two limits and dividing by two.

  • What is the margin of error in the context of a confidence interval?

    -The margin of error is the distance from the sample mean to either the upper or lower limit of the confidence interval. It represents the range within which the true population mean is likely to fall.

  • How can you find the margin of error given the confidence interval limits?

    -To find the margin of error, subtract the lower limit from the upper limit of the confidence interval, and then divide the result by two to get half the length of the interval.

  • What does the video mention about the confidence level of the weight loss program study?

    -The video mentions a 90% confidence level for the weight loss program study, meaning that if the study were repeated 100 times, the true population mean would be contained within the confidence interval in 90 of those instances.

  • What was the confidence interval estimate for the mean weight loss after 12 months in the Atkins weight loss program study?

    -The 90% confidence interval estimate for the mean weight loss after 12 months in the Atkins weight loss program study was between 0.8 pounds and 3.4 pounds.

  • How much was the mean weight loss for the 40 adults in the Atkins weight loss program study after one year?

    -The mean weight loss for the 40 adults in the study after one year was 2.1 pounds.

  • What is the margin of error for the weight loss confidence interval in the Atkins weight loss program study?

    -The margin of error for the weight loss confidence interval in the study is 1.3 pounds.

  • How can you verify the margin of error calculation?

    -You can verify the margin of error calculation by checking that the distance from the sample mean to the upper limit plus the distance from the sample mean to the lower limit equals twice the margin of error.

  • What does the 90% confidence interval suggest about the true population mean for the Atkins weight loss program?

    -The 90% confidence interval suggests that we can be 90% confident that the true population mean for the Atkins weight loss program lies between 0.8 pounds and 3.4 pounds of weight loss after 12 months.

Outlines
00:00
πŸ“Š Understanding Confidence Intervals and Margin of Error

This paragraph introduces the concept of finding the margin of error and point estimate, x-bar, for a population mean given a confidence interval. It explains that the point estimate is the sample mean, which is the average of the upper and lower limits of the confidence interval. The margin of error is calculated by taking half the length of the interval, which is the distance between the upper and lower limits. The paragraph uses an example of a weight loss program study to illustrate these calculations, showing how to find the sample mean and margin of error from a 90% confidence interval for mean weight loss.

05:02
βœ‚οΈ Calculating the Margin of Error with Confidence

The second paragraph builds on the previous discussion by providing alternative methods to calculate the margin of error. It emphasizes that the margin of error can be verified by subtracting the sample mean from the upper limit and then from the lower limit, both yielding the same result. This reinforces the concept that the margin of error is the distance from the sample mean to either the upper or lower limit of the confidence interval, and it confirms the accuracy of the calculations by showing that different approaches lead to the same outcome.

Mindmap
Keywords
πŸ’‘Margin of Error
The margin of error is a measure of the precision of an estimate. It represents the range within which the true population parameter is likely to fall, given a certain level of confidence. In the video, the margin of error is calculated by taking half the difference between the upper and lower limits of the confidence interval, which in the script's example is 1.3 pounds. This concept is central to understanding the reliability of the sample mean in estimating the population mean.
πŸ’‘Point Estimate
A point estimate is a single value that serves as the best guess for a population parameter based on a sample. In the context of the video, the point estimate for the population mean is represented by the sample mean (xΜ„), which is calculated as the arithmetic mean of the upper and lower limits of the confidence interval. The script illustrates this by averaging 0.8 and 3.4 pounds to find the sample mean of 2.1 pounds.
πŸ’‘Confidence Interval
A confidence interval provides a range of values within which the true population parameter is estimated to lie, with a certain level of confidence. The video discusses how to interpret a 90% confidence interval for the mean weight loss, indicating that if the interval were calculated many times, it would contain the true population mean 90% of the time.
πŸ’‘Sample Mean (xΜ„)
The sample mean (xΜ„) is the average of the values in a sample, used as an estimate for the population mean. In the script, the sample mean is found by averaging the upper and lower limits of the confidence interval, which is a method to estimate the population mean based on the sample data.
πŸ’‘Arithmetic Mean
The arithmetic mean is the sum of a set of numbers divided by the count of those numbers. It is a measure of central tendency. In the video, the arithmetic mean is used to calculate the point estimate of the population mean by averaging the confidence interval limits.
πŸ’‘Population Mean
The population mean is the average of all values in a population. The video's theme revolves around estimating this value using a sample mean and a confidence interval, with the script providing an example of estimating the mean weight loss for a population.
πŸ’‘Weight Loss Program
The weight loss program is the context within which the video's statistical concepts are applied. The script discusses an example where 40 adults used the Atkins weight loss program, and a 90% confidence interval for their mean weight loss was calculated.
πŸ’‘Confidence Level
The confidence level is the percentage that reflects the probability that the true population parameter lies within the calculated confidence interval. In the script, a 90% confidence level is used, which means there is a 90% probability that the interval contains the true population mean.
πŸ’‘Upper Limit
The upper limit of a confidence interval is the higher boundary of the range within which the population parameter is estimated to lie. In the script, the upper limit is 3.4 pounds, which is used along with the lower limit to calculate the margin of error and the sample mean.
πŸ’‘Lower Limit
The lower limit of a confidence interval is the lower boundary of the range. In the script, the lower limit is 0.8 pounds, and it is used in conjunction with the upper limit to determine the confidence interval's width and the margin of error.
πŸ’‘Interval Length
Interval length refers to the distance between the upper and lower limits of a confidence interval. The script explains that this length is twice the margin of error, and by dividing it by two, one can find the margin of error for the interval.
Highlights

The video discusses how to find the margin of error and point estimate for a population mean given a confidence interval.

The point estimate of the population mean is the sample mean, calculated as the average of the upper and lower limits of the confidence interval.

The margin of error is found by taking half the length of the confidence interval, which is the distance between the upper and lower limits.

The sample mean (x bar) is the midpoint between the confidence interval limits, found by averaging them.

The margin of error can also be calculated by subtracting the lower limit from the upper limit of the confidence interval and dividing by 2.

A 90% confidence interval for the mean weight loss after 12 months on the Atkins diet was found to be between 0.8 and 3.4 pounds.

The 90% confidence level means that if the intervals were created 100 times, 90 of them would contain the true population mean.

The sample mean weight loss for the 40 adults on the Atkins diet was calculated to be 2.1 pounds after 12 months.

The margin of error for the weight loss confidence interval was found to be 1.3 pounds.

The margin of error can be verified by subtracting the sample mean from the upper and lower limits of the interval.

The video provides a step-by-step explanation of how to calculate the sample mean and margin of error from a confidence interval.

The process involves understanding the relationship between the sample mean, margin of error, and the confidence interval limits.

The video emphasizes the importance of correctly interpreting confidence intervals and their implications for statistical analysis.

The example of the Atkins diet weight loss study demonstrates the application of these concepts in a real-world context.

The video clarifies common misconceptions about calculating the sample mean and margin of error from a confidence interval.

The method presented is applicable to any confidence interval, not just the specific example given in the video.

Transcripts

Browse More Related Video

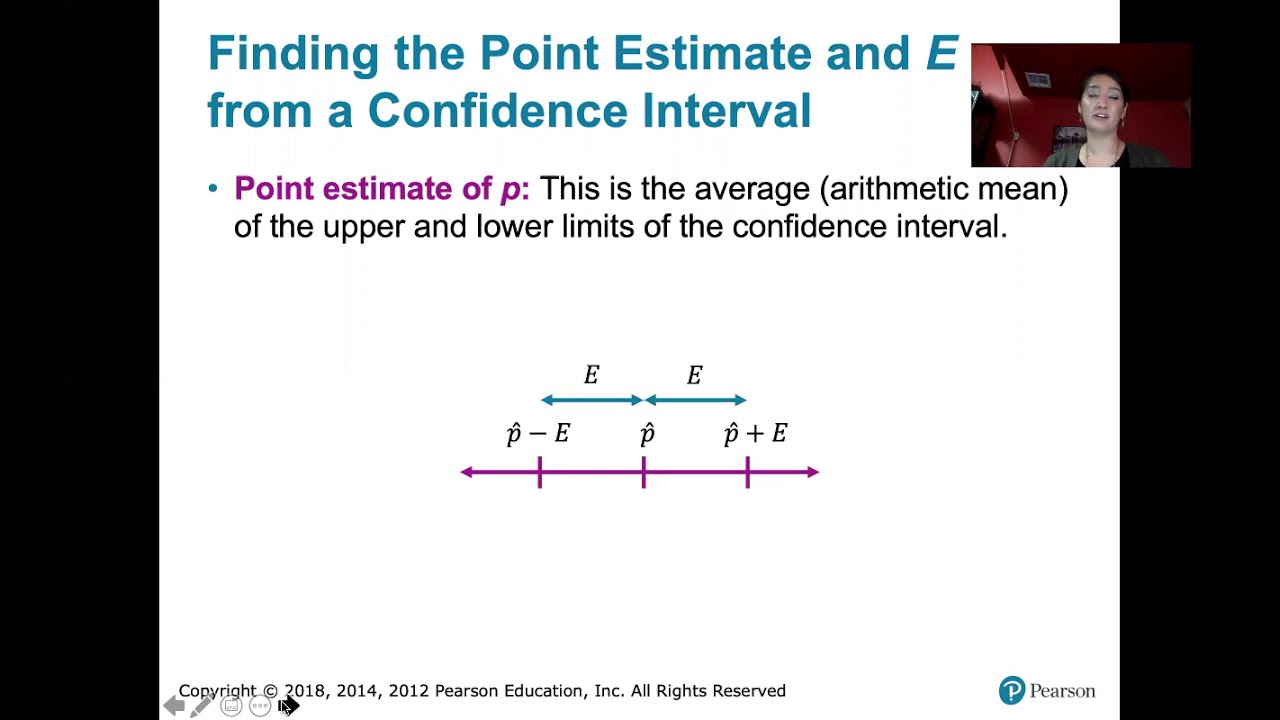
7.1.5 Estimating a Population Proportion - Given a Confidence Interval, Find p Hat and E.

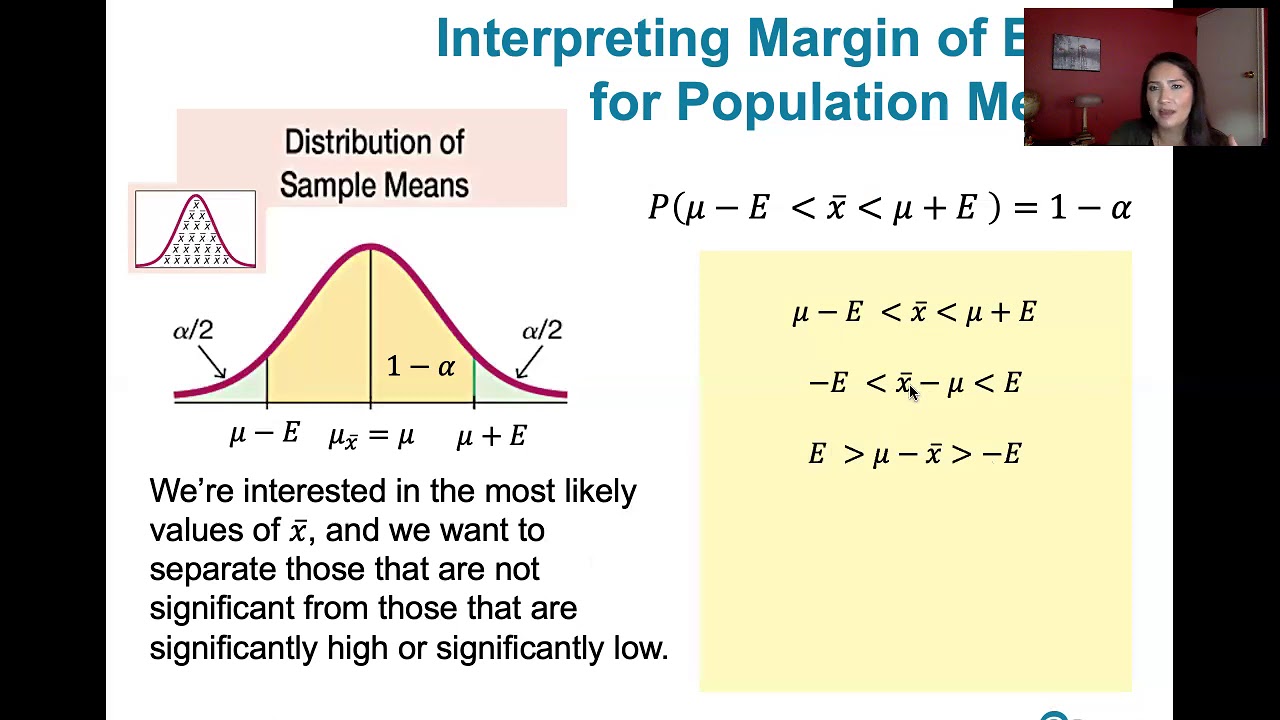
7.2.2 Estimating a Population Mean - Sample Mean, Margin of Error, and Finding Confidence Intervals

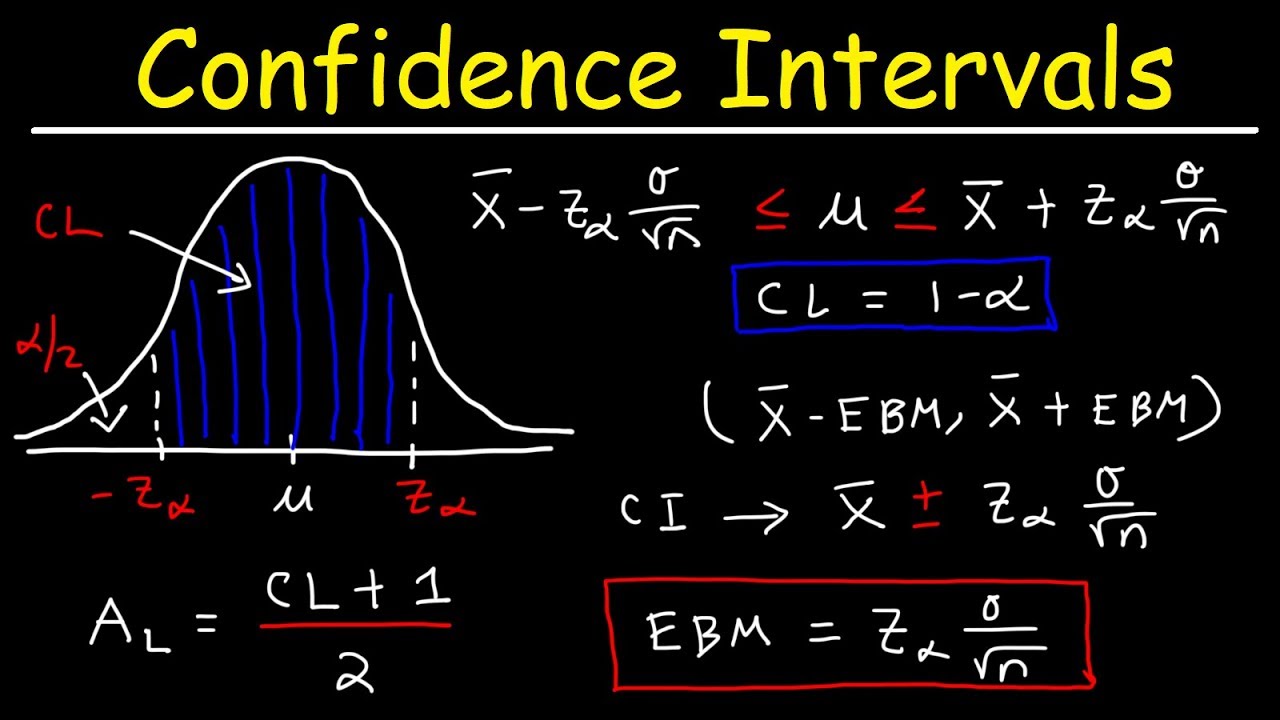
How To Find The Z Score, Confidence Interval, and Margin of Error for a Population Mean

Confidence interval example | Inferential statistics | Probability and Statistics | Khan Academy

Confidence Interval for a population proportion | Solved Problems

Confidence Interval for a population mean - t distribution

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Confidence IntervalSample MeanMargin of ErrorStatistical AnalysisWeight LossAtkins DietEducational ContentData InterpretationStatistical LearningPopulation Mean