4.1.3 Basics of Probability - Finding the probability of the complement of an event

Sasha Townsend - Tulsa
10 Oct 202005:29
EducationalLearning
32 Likes 10 Comments

TLDRThis video script explores the concept of the complement of an event in probability theory. It defines the complement as all outcomes where the event does not occur, using the example of rain to illustrate. The script explains how to calculate the probability of the complement by subtracting the event's probability from 1. It also applies this concept to a survey of adult smokers in the U.S., demonstrating how to estimate the probability of selecting a smoker and a non-smoker, reinforcing the idea that the sum of probabilities of an event and its complement equals one.

Takeaways
  • πŸ“š The video discusses the concept of the complement of an event in probability theory.
  • πŸ” The complement of an event A, denoted as \( \overline{A} \), includes all outcomes where event A does not occur.
  • 🌧️ An example given is that if event A is 'it will rain today,' then \( \overline{A} \) is 'it will not rain today'.
  • πŸ“‰ The complement of an event is used to find the probability of the event not occurring.
  • 🎯 If the probability of event A is known, the probability of \( \overline{A} \) is found by subtracting from 1 (e.g., \( 1 - P(A) \) ).
  • πŸ“ˆ The script explains how to interpret probabilities in terms of percentages and convert them back to decimal form.
  • 🌀️ The video uses the example of rain probability to explain how to calculate the complement probability.
  • 🚬 Another example involves estimating the probability of selecting a smoker or a non-smoker from a survey.
  • πŸ“Š The probability of a randomly selected adult being a smoker is approximated by the ratio of smokers in the survey to the total number of adults.
  • 🚭 Similarly, the probability of selecting a non-smoker is found by subtracting the smoker probability from 1.
  • πŸ” The concept of complements will be revisited in section 4.2, indicating its importance in the study of probability.
Q & A

  • What is the complement of an event in probability theory?

    -The complement of an event, typically denoted by a bar over the event symbol (e.g., AΜ… for the complement of event A), consists of all outcomes in which the event A does not occur.

  • How can you represent the event that it will not rain today if event A is that it will rain?

    -The event that it will not rain today can be represented as AΜ… or simply as 'not A'.

  • What is the relationship between the probabilities of an event and its complement?

    -The probabilities of an event A and its complement AΜ… must sum up to 1, as they are the only two possible outcomes of a binary situation.

  • If the probability of rain today is 0.7, what is the probability that it will not rain?

    -If the probability of rain (event A) is 0.7, then the probability that it will not rain (event AΜ…) is 1 - 0.7, which equals 0.3.

  • How do you interpret a probability of 0.7 in terms of percentage?

    -A probability of 0.7 can be interpreted as a 70% chance of the event occurring, by multiplying the decimal by 100.

  • What is the addition rule in probability theory, and how does it relate to complements?

    -The addition rule states that the probability of either event A or its complement AΜ… occurring is 1. This is derived from the fact that these are the only two outcomes and their probabilities must sum to 100% or 1.

  • In the given survey example, what is the estimated probability that a randomly selected adult is a smoker?

    -Based on the survey data, the estimated probability that a randomly selected adult is a smoker is 202 out of 1010, which is approximately 0.2 or 20%.

  • If there is a 20% chance of selecting a smoker from the survey, what is the chance of selecting a non-smoker?

    -If there is a 20% chance of selecting a smoker, then there is an 80% chance of selecting a non-smoker, calculated as 1 - 0.2.

  • What approach is used in the survey example to estimate the probability of an adult being a smoker?

    -The relative frequency approach is used, where the probability is approximated by the ratio of smokers in the sample to the total number of adults surveyed.

  • Why is it important to understand the concept of complements in probability?

    -Understanding complements is important as it helps in calculating the probability of the opposite outcome in a binary situation, which is crucial for making informed decisions based on probabilistic outcomes.

  • How will the concept of complements be revisited in the next section of the study?

    -The concept of complements will be revisited in section 4.2, potentially with further examples or a deeper exploration of its applications in probability theory.

Outlines
00:00
πŸ“š Understanding Event Complements and Probabilities

This paragraph introduces the concept of the complement of an event in probability theory. It explains that the complement, denoted by a bar over the event symbol, includes all outcomes where the event does not occur. The script uses the example of rain to illustrate this concept, explaining that if the probability of rain (event A) is 0.7, then the probability of no rain (event A') is calculated by subtracting 0.7 from 1, resulting in 0.3. The paragraph emphasizes the additive property of probabilities, where the sum of the probabilities of an event and its complement must equal 1, or 100%. It also provides a practical example involving a survey of 1010 adults, where 202 are smokers, to demonstrate the calculation of the probability of selecting a smoker and a non-smoker based on the sample data.

05:02
🚬 Estimating Probabilities with Survey Data

The second paragraph continues the discussion on probabilities, focusing on the application of survey data to estimate the likelihood of an event. It reiterates the calculation of the probability of selecting a smoker (0.2 or 20%) and a non-smoker (0.8 or 80%) from a sample of 1010 adults, where 202 are identified as smokers. The paragraph concludes with a reminder that the concept of complements will be revisited in section 4.2, indicating the importance of understanding these foundational concepts for further study in probability.

Mindmap
Keywords
πŸ’‘Complement of an event
The complement of an event, denoted by a bar over the event symbol (e.g., A), includes all outcomes where the event does not occur. It is a fundamental concept in probability theory, representing the opposite of a given event. In the video, the complement is used to illustrate the relationship between the probability of an event occurring and not occurring, such as the event of it raining and not raining.
πŸ’‘Probability
Probability is a measure of the likelihood that a particular event will occur, expressed as a number between 0 and 1. In the context of the video, it is used to quantify the chance of events like rain or selecting a smoker from a sample, emphasizing the importance of understanding both the event and its complement.
πŸ’‘Relative frequency
Relative frequency is an approach to estimating probabilities by using the ratio of the number of times an event occurs to the total number of trials. The video uses this method to estimate the probability of an adult being a smoker based on survey data, highlighting its application in real-world scenarios.
πŸ’‘Addition rule
The addition rule in probability is a principle stating that the probability of either one event or another occurring is the sum of their individual probabilities. The video script alludes to this rule when explaining that the probabilities of an event and its complement must add up to 1.
πŸ’‘Outcome
An outcome is a possible result of an experiment or event. The video discusses the complement of an event in terms of outcomes that do not satisfy the event condition, such as the outcome of it not raining on a given day.
πŸ’‘Interpretation
Interpretation in the context of the video refers to understanding the meaning of a given probability. For instance, a probability of 0.7 is interpreted as a 70% chance of rain, which helps in translating numerical probabilities into a more intuitive understanding.
πŸ’‘Sample
A sample is a subset of a population used to represent and make inferences about the entire population. The video uses survey data of 1010 adults as a sample to estimate the probability of an adult being a smoker in the United States.
πŸ’‘Approximation
Approximation is the process of estimating a value based on available data or information. The video uses the number of smokers in a sample to approximate the probability of selecting a smoker at random, demonstrating how sample data can inform about a larger population.
πŸ’‘Non-smoker
A non-smoker is an individual who does not smoke. The video contrasts the probability of selecting a smoker with that of a non-smoker, using the complement concept to determine the latter's probability as 1 minus the probability of the former.
πŸ’‘Survey
A survey is a method of data collection that involves asking a group of people questions to gather information. In the video, a survey of 1010 adults is mentioned to demonstrate how probabilities can be estimated from empirical data.
Highlights

The video discusses learning outcome number three from lesson 4.1, focusing on the concept of the complement of an event in probability.

The complement of an event, denoted by a bar, includes all outcomes where the event does not occur.

Examples are given to illustrate the concept, such as the event of raining and its complement of not raining.

The video explains that the sum of the probabilities of an event and its complement must equal one.

Probability of an event is interpreted as a percentage to understand the likelihood of occurrence.

The probability of the complement is calculated by subtracting the event's probability from 1.

An intuitive understanding is emphasized, where the complement's probability is the remaining chance after the event's probability is accounted for.

The addition rule in probability is mentioned as a formula to be derived later, which will support the understanding of complements.

A survey example is used to estimate the probability of a randomly selected adult being a smoker.

The relative frequency approach is applied to approximate the probability based on survey data.

The probability of a randomly selected adult being a smoker is calculated to be 0.2 or 20%.

The probability of selecting a non-smoker is deduced to be 0.8 or 80% by subtracting the smoker's probability from 1.

The video uses the survey data to approximate the total population's smoking habits.

The concept of complements will be revisited in section 4.2, indicating its importance in the study of probability.

The video concludes with a summary of the key points covered regarding the complement of an event and its probability.

Transcripts

Browse More Related Video

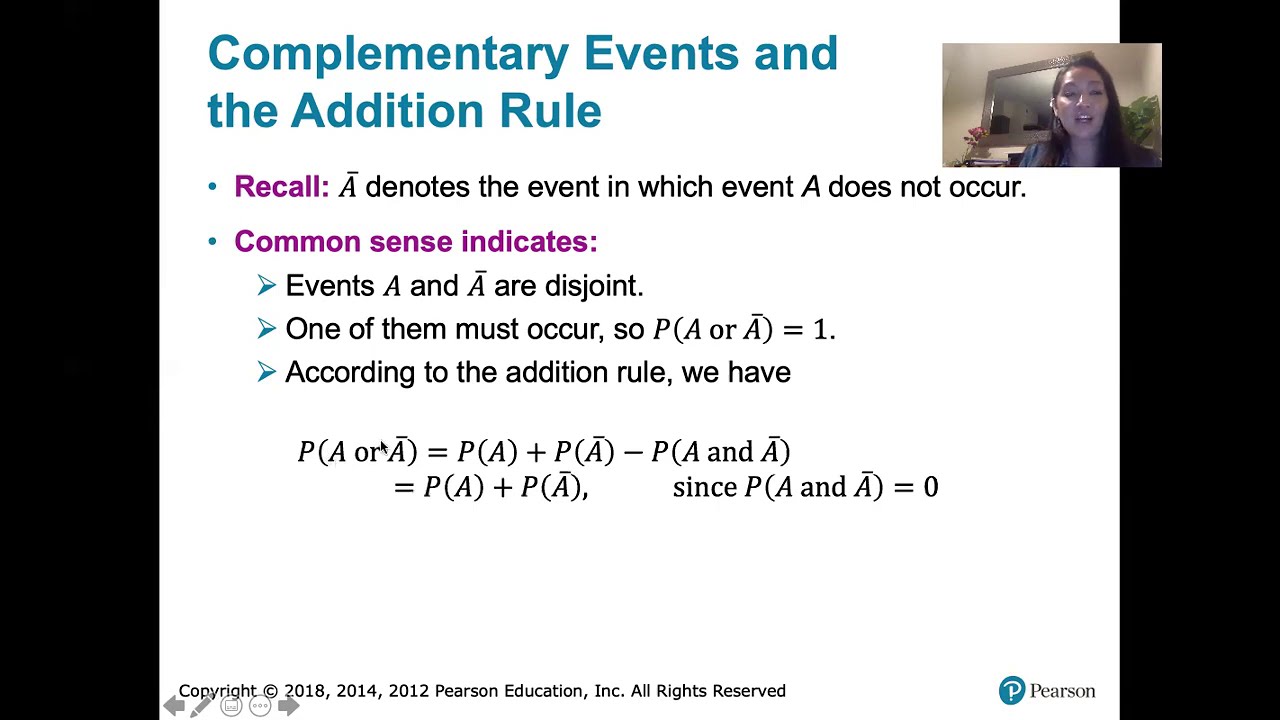
4.2.4 Addition and Multiplication Rules - The Rule of Complementary Events

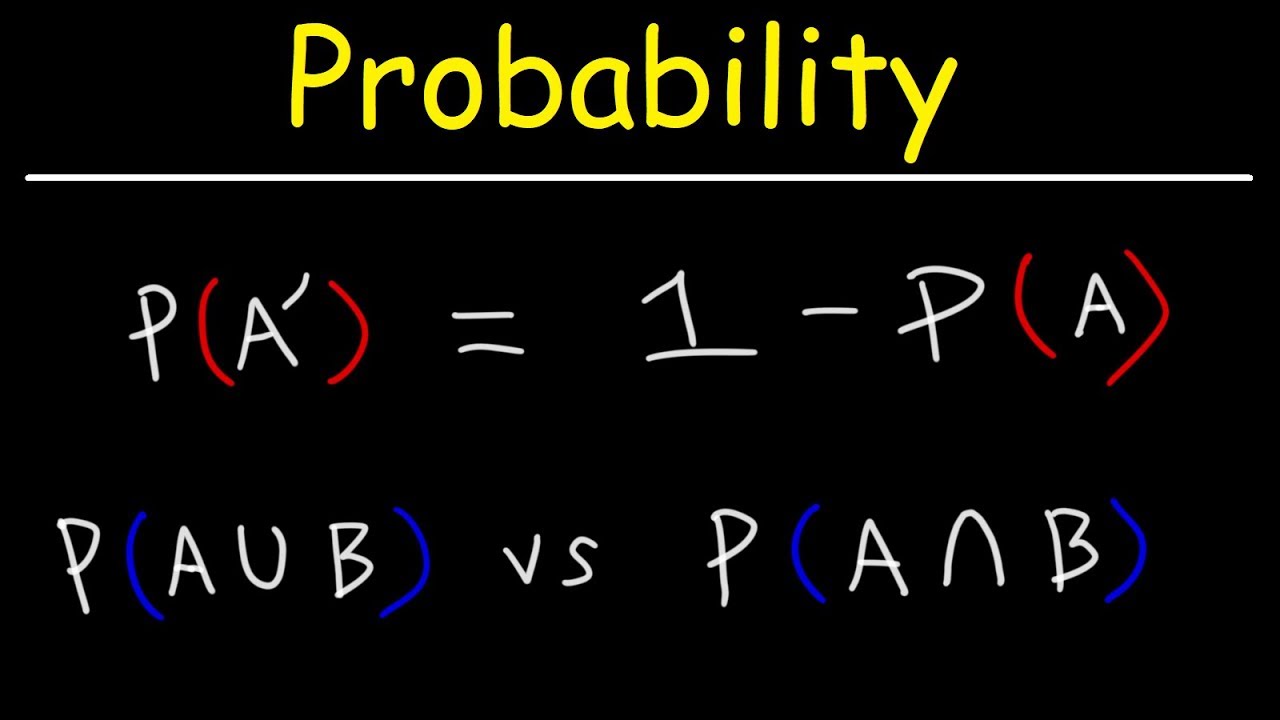
Probability of Complementary Events & Sample Space

Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams

Conditional Probability With Venn Diagrams & Contingency Tables

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

Lecture 3: Birthday Problem, Properties of Probability | Statistics 110

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityComplementEventsLearningEducationMathematicsStatisticsVideoLessonUnderstanding