Equations of Motion in Physics Made Easy - A Step-by-Step Explanation

Math and Science

13 Jun 202338:33

EducationalLearning

32 Likes 10 Comments

TLDRThis lesson aims to demystify the equations of motion for constant acceleration, emphasizing their practical applications in physics. The instructor breaks down the intimidating equations, highlighting two core equations derived from basic calculus. The lesson focuses on understanding these equations' structure and logic through unit analysis and graphical representation, ensuring comprehension before delving into their formal derivation in the next lesson. The goal is to equip students with a solid foundation to solve a variety of motion problems, emphasizing the importance of recognizing constant acceleration in real-world scenarios.

Takeaways

📚 The lesson aims to familiarize students with equations of motion, emphasizing they may seem intimidating but are easier to understand when broken down.
🔑 The core focus is on understanding the equations' purpose and logic before deriving them in a subsequent lesson, highlighting the importance of comprehension over mere memorization.
🚫 The equations of motion discussed are applicable only under conditions of constant acceleration, making it crucial to identify variable acceleration situations where these equations do not apply.
📉 The instructor clarifies that while there are five equations of motion presented, the first two are fundamental, derived from basic calculus, and the others are derived from these core equations.
🔄 The equations are initially introduced with a 'missing quantity' column to illustrate which variables are not included in each equation, aiding in problem-solving when certain information is unknown.
🔶 The variables X, X₀, V, V₀, a, and T are defined in the context of motion, with X₀ and V₀ representing initial position and velocity, and a representing constant acceleration.
📈 The lesson explains the physical meaning of the equations, using the concepts of velocity increasing linearly with time under constant acceleration, leading to a quadratic position function over time.
📊 A graphical representation is used to demonstrate the relationship between constant acceleration, linear velocity increase, and quadratic position change over time.
🧩 The instructor pieces together the equations by showing the logical and mathematical consistency between them, such as deriving velocity from position and acceleration from velocity.
🔍 The importance of understanding units in physics is emphasized, ensuring that each term in the equations contributes to a consistent unit of measurement, particularly meters per second for velocity.
📚 The lesson concludes with a preview of the next lesson, where the equations will be derived from first principles, reinforcing the idea that understanding the derivation deepens comprehension and application of the equations.

Q & A

What is the main goal of the lesson in the provided script?
-The main goal of the lesson is to introduce and explain the equations of motion, particularly focusing on their application with constant acceleration, and to demystify their intimidating appearance by breaking them down for better understanding.
Why are the equations of motion intimidating to some students?
-The equations of motion can be intimidating because they often appear complex and abstract. The instructor aims to break down this intimidation by showing how easy they are to understand and apply in practical situations.

Outlines

00:00

📚 Introduction to Equations of Motion

The instructor begins by setting the stage for a lesson on equations of motion, emphasizing that while these equations may seem daunting, they will be simplified for understanding. The lesson's goal is not to derive the equations but to familiarize students with them and ensure they make sense conceptually. It's mentioned that the equations are applicable in scenarios with constant acceleration, and their practical applications will be explored in future lessons, including horizontal motion, vertical motion under gravity, and curved path motion. The instructor also clarifies that while there are five equations of motion, the first two are fundamental and derived from basic calculus principles, with the rest being variations of these core equations.

05:01

🔍 Breakdown of Key Variables in Motion Equations

This paragraph delves into the specific variables involved in the equations of motion, such as position (x), initial position (x₀), acceleration (a), time (t), final velocity (v), and initial velocity (v₀). The lesson elaborates on how these variables interact within the equations.

Mindmap

Keywords

💡Equations of Motion

Equations of Motion are fundamental in physics to describe the relationship between an object's velocity, acceleration, time, and displacement. In the video, they are the central theme, with the instructor emphasizing their importance in understanding constant acceleration scenarios. The script breaks down these equations to demystify their intimidating appearance and to prepare students for their derivation in subsequent lessons.

💡Constant Acceleration

Constant Acceleration refers to an unchanging rate of change of velocity over time. It is a prerequisite for using the equations of motion discussed in the script. The instructor clarifies that these equations apply when acceleration remains constant, such as in the case of an object moving with uniform acceleration in a straight line, and not when the acceleration varies, such as in the case of a car accelerating erratically.

💡Derive

In the context of the video, 'derive' means to mathematically demonstrate the origin or validity of a formula or principle. The instructor mentions that in the next lesson, they will derive the equations of motion from first principles using calculus, which will provide a deeper understanding of why these equations are true and how they are interconnected.

💡Initial Conditions

Initial Conditions are the starting values for position and velocity at the beginning of a time interval. The script explains that these conditions are essential for solving motion problems and are represented by terms like 'X naught' (X₀) for initial position and 'V naught' (V₀) for initial velocity in the equations of motion.

💡Final Conditions

Final Conditions denote the position and velocity of an object at the end of a time interval. The script discusses how these conditions, represented by 'X' for final position and 'V' for final velocity, are calculated using the equations of motion, which incorporate initial conditions, acceleration, and time.

💡Displacement

Displacement, symbolized as 'Delta X' (ΔX) in the script, refers to the change in position of an object. It is the difference between the final position (X) and the initial position (X₀). The instructor uses this concept to explain one of the equations of motion, which relates displacement to initial velocity, acceleration, and time.

💡Velocity

Velocity is a vector quantity that represents the rate of change of an object's position with respect to time. The script discusses both initial and final velocities (V₀ and V) and how they relate to acceleration and displacement in the context of the equations of motion.

💡Acceleration

Acceleration is the rate at which an object's velocity changes over time. In the script, acceleration is symbolized by 'a' and is a key component of the equations of motion. The instructor emphasizes that for the equations to be applicable, acceleration must be constant, which is a critical condition for their validity.

💡Gravitational Acceleration

Gravitational Acceleration, denoted as 'g' in the script, is the acceleration due to gravity. It is approximately constant near the Earth's surface and is used in the equations of motion to describe the motion of objects in free fall. The instructor mentions that these equations can be slightly modified to account for the constant gravitational acceleration.

💡Prototype

In the script, 'prototype' refers to a conceptual or graphical model that represents the typical behavior of velocity, acceleration, and position when acceleration is constant. The instructor uses prototypes to help students visualize and understand the relationships between these quantities, such as the linear relationship between velocity and time, and the quadratic relationship between position and time.

Highlights

The lesson aims to demystify equations of motion, making them accessible and understandable.

Equations of motion are primarily used for scenarios with constant acceleration.

The instructor emphasizes that these equations are not derived in this lesson but will be in the next.

Core equations are derived from first principles and basic calculus, forming the foundation for other equations.

The importance of understanding the practical value of these equations is stressed, including their use in horizontal and vertical motion.

The concept of 'missing quantity' in equations is introduced to tackle problems with unknown variables.

Transcripts

Browse More Related Video

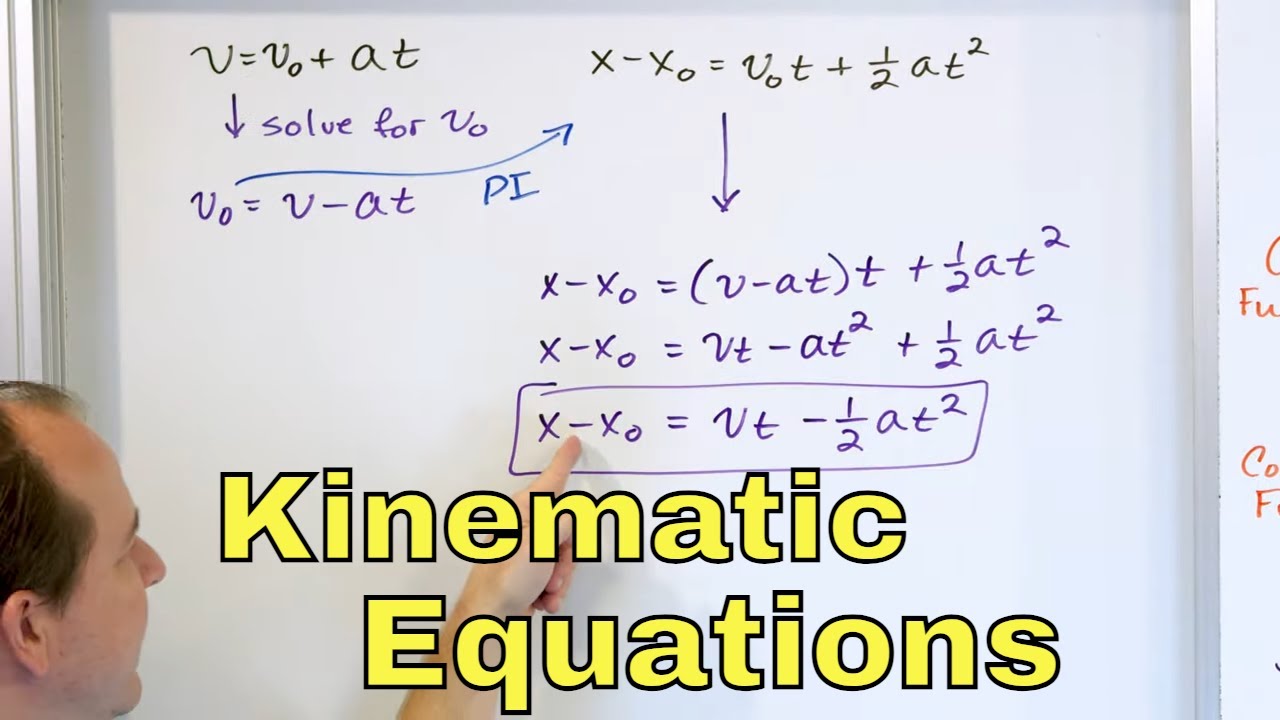
Deriving the Kinematic Equations of Motion w/ Constant Acceleration in Physics - [1-2-13]

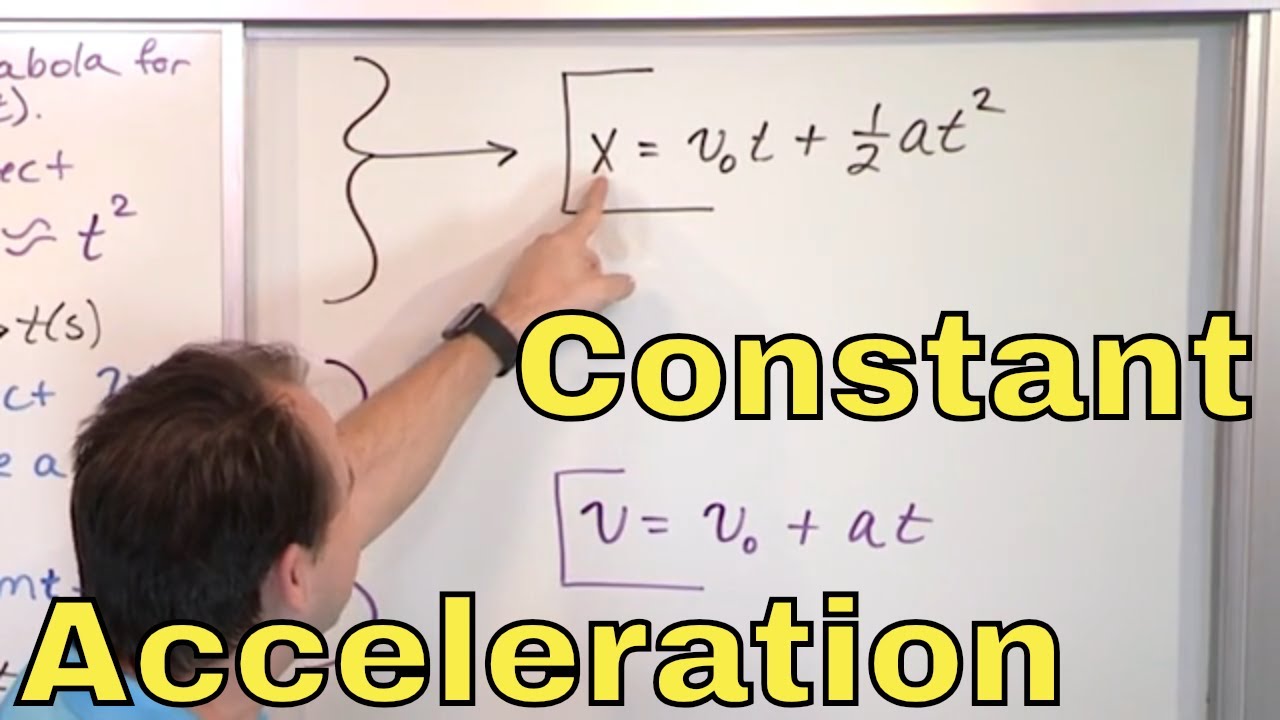
01 - Motion with Constant Acceleration in Physics (Constant Acceleration Equations)

Differential Equations with Velocity and Acceleration (Differential Equations 7)

AP Physics 1: Rotational Kinematics Review

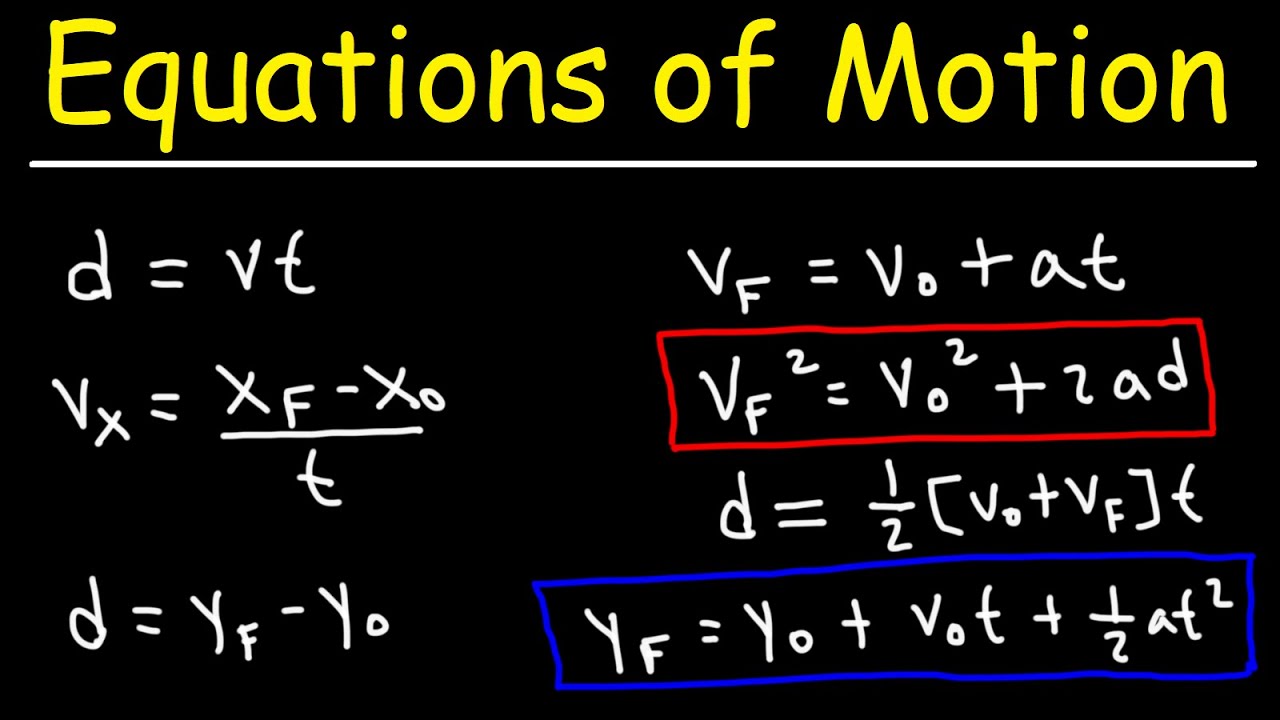
Equations of Motion

Kinematic Equations in One Dimension | Physics with Professor Matt Anderson | M2-04