AP Calculus AB: Lesson 2.2 The Derivative Function
TLDRThis lesson explores the concept of the derivative function, focusing on the limit definition to compute derivative values for various points. The instructor demonstrates the process for the function f(x) = 4x - x^2, leading to a conjecture about the general formula for f'(a). The video also covers how to graph derivatives and provides practice examples for different functions, including linear and quadratic functions, and even piecewise linear functions, highlighting the importance of understanding the slope of tangent lines and the instantaneous rate of change.
Takeaways
- 📚 The lesson focuses on understanding the derivative function and computing derivative values using the limit definition.
- 🔍 Activity one involves calculating the derivative values for a given function at specific points (f'(0), f'(1), f'(2), f'(3)) to observe patterns and make conjectures.
- 📉 The process involves simplifying the function f(x) = 4x - x^2 at different points and finding the limit as h approaches 0 to determine the derivative at those points.
- 📈 A conjecture is made based on the observed pattern that the derivative decreases by 2 as the input value increases by 1, leading to a general formula for f'(a).
- 📝 The script demonstrates the transition from finding the derivative at specific points to deriving a general formula for the derivative function, f'(x) = 4 - 2x.
- 📉 The importance of understanding the derivative as the slope of the tangent line at a point on the graph is emphasized, with various interpretations such as 'slope of the curve' or 'instantaneous rate of change'.
- 📈 The script includes examples of graphing the derivative function, showing how to plot points and sketch the graph of the derivative based on the original function.
- 🔢 The process of finding the derivative for different types of functions, including linear, quadratic, and square root functions, is explained with step-by-step calculations.
- 📚 The lesson concludes with practice problems to reinforce the understanding of finding and graphing derivative functions for various mathematical functions.
- 🔑 Key takeaways include the method of finding the derivative function, the significance of the derivative in real-world problems, and the ability to graph the derivative function even without its explicit equation.
- 🚀 The lesson sets the stage for further exploration of the derivative in the context of real problems in upcoming lessons.
Q & A
What is the main topic of the lesson presented by Michelle Crummel?
-The main topic of the lesson is the concept of the derivative function, specifically how to compute derivative values using the limit definition for the function f(x) = 4x - x^2.
What is the purpose of calculating f'(a) for different values of 'a' in the lesson?
-The purpose is to observe that the work to find f'(a) is the same regardless of the value of 'a', and to come up with a conjecture for the value of f'(4) and f'(5), and a general formula for f'(a).
What is the limit definition used to compute the derivative values in the lesson?
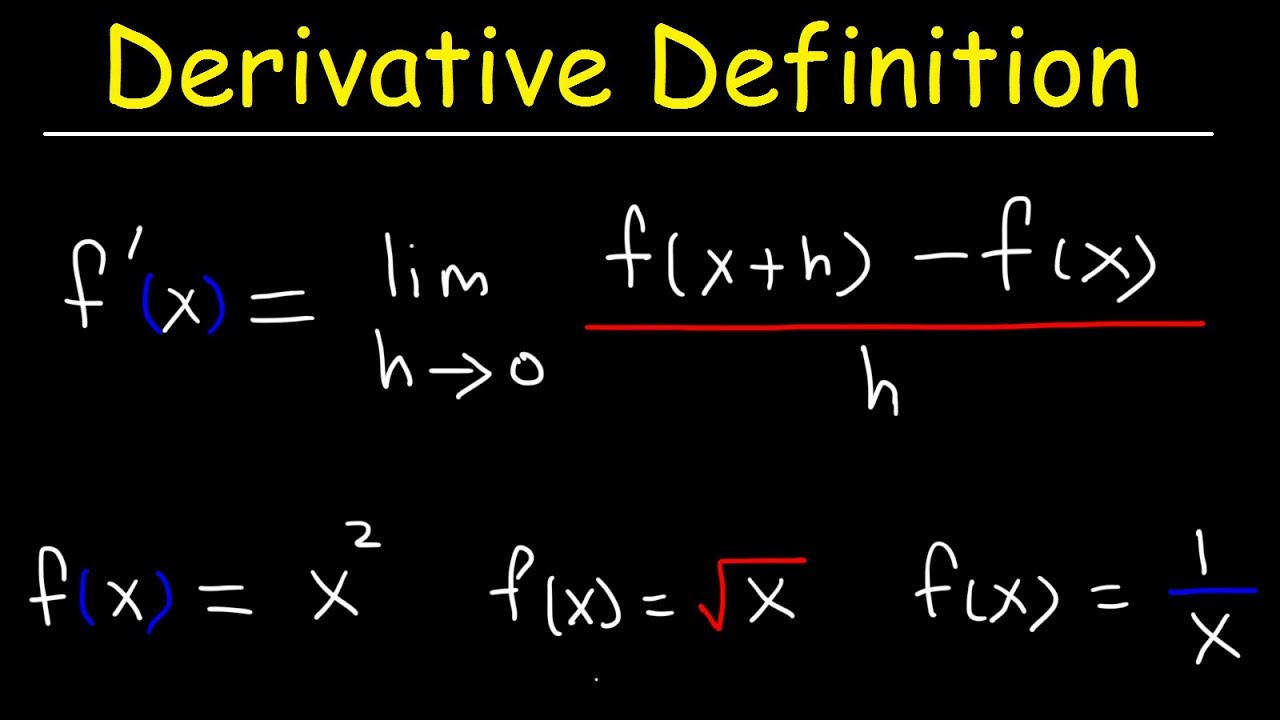
-The limit definition used is f'(a) = lim(h → 0) [(f(a + h) - f(a)) / h].
What is the conjecture for the value of f'(4) based on the pattern observed in the lesson?
-The conjecture for the value of f'(4) is that it would be -4, based on the observed pattern of the derivative decreasing by 2 as the value of 'a' increases by 1.
What is the general formula conjectured for f'(a) in the lesson?
-The general formula conjectured for f'(a) is -2a + 4, which is derived from observing the pattern in the values of the derivative for different 'a'.
How does the lesson explain the process of finding the derivative of a function?
-The lesson explains the process by first showing the steps to find the derivative at specific points using the limit definition, then generalizing the process to find the derivative function f'(x) for any x.
What is the advantage of finding the derivative equation f'(x) instead of just calculating f'(a) for specific values?
-The advantage is that once the derivative equation f'(x) is found, it can be used to quickly determine the derivative at any point without repeating the limit process for each specific value.
How does the lesson relate the derivative function to the slope of the tangent line at a point on a graph?
-The lesson explains that the derivative function f'(x) gives the slope of the tangent line to the graph of f at any point x, allowing for the calculation of the instantaneous rate of change at that point.
What is the significance of the derivative function in understanding the behavior of a function?
-The derivative function is significant as it provides information about the slope of the tangent lines to the graph of the function at different points, indicating where the function is increasing, decreasing, or has a horizontal tangent (maxima, minima, or points of inflection).
How does the lesson demonstrate the process of graphing the derivative function without its equation?
-The lesson demonstrates this by using the graph of the original function f(x) to estimate the slopes at various points and then plotting these points to sketch the graph of the derivative function.
Outlines
📚 Introduction to Derivative Function
Michelle Crummel introduces the concept of the derivative function, focusing on the function f(x) = 4x - x^2. She outlines an activity to compute the derivative values at specific points using the limit definition. The goal is to observe patterns in the derivative values and conjecture a general formula for f'(a) based on the observed values. The process begins with finding f'(0) using the limit as h approaches 0, simplifying the expression to isolate the derivative, and finding that f'(0) equals 4.
🔍 Derivative Values and Patterns
The script continues with the calculation of derivative values at points f'(1), f'(2), and f'(3). Through simplification and factoring, it is found that f'(1) = 2, f'(2) = 0, and f'(3) = -2. A pattern is observed where the derivative decreases by 2 as the input value increases by 1. Based on this, conjectures are made for f'(4) and f'(5), and a general formula f'(a) = -2a + 4 is proposed, illustrating the relationship between input and output values.
📉 Derivative Function and Its Definition
The explanation shifts to the definition of the derivative function, f'(x), as the limit of (f(x+h) - f(x))/h as h approaches 0. This definition provides the slope of the tangent line at any arbitrary point x on the graph of f. The script differentiates between finding the slope at a specific point (f'(a)) and the slope at any point (f'(x)). The process of finding the derivative for the given function f(x) = 4x - x^2 is demonstrated, resulting in the derivative function f'(x) = 4 - 2x.
📈 Graphing the Derivative Function
The script explains how to graph the derivative function by plotting points that correspond to the slopes of tangent lines at various x-coordinates of the original function f(x). It provides a method to sketch the graph of the derivative without the derivative equation, using the original function's graph to estimate slopes and plot points. The process involves identifying points where the slope is zero, and using symmetry or estimation to plot additional points, ultimately connecting them to form the derivative graph.
🤔 Estimating Derivative Graphs from Function Graphs
The video script discusses techniques for estimating the graph of the derivative function from the graph of the original function. It emphasizes identifying points where the derivative is zero, as these correspond to horizontal tangent lines on the original graph. The process involves visual estimation of slopes at various points on the graph and plotting these on the derivative graph, providing a rough sketch of the derivative's behavior.
📘 Derivative Function for Various Types of Functions
The script presents several examples of finding the derivative function for different types of functions, including constant functions, linear functions, quadratic functions, and others. Each example demonstrates the process of applying the limit definition to find the derivative, simplifying expressions, and factoring out the variable h to isolate the derivative. The results include derivatives such as 0 for a constant function, 1 for a linear function with a slope of 1, 2z for a quadratic function z^2, and -1/t^2 for the function 1/t.
🔧 Derivative of Square Root Function
The final part of the script focuses on finding the derivative of the square root function. The process involves multiplying by the conjugate to simplify the expression and eliminate the h in the denominator. The limit is then taken as h approaches 0, resulting in the derivative of the square root function being 1/(2√y), with the original variable x replaced by y to maintain consistency with the function notation.
Mindmap
Keywords
💡Derivative
💡Limit Definition
💡Slope of the Tangent Line
💡Instantaneous Rate of Change
💡Conjecture
💡Linear Function
💡Graphical Interpretation
💡Tangent Line
💡Slope-Intercept Form
💡Piecewise Linear Function
💡Discontinuity
💡Square Root Function
Highlights
Introduction to the concept of the derivative function and its calculation using the limit definition.
Activity one involves computing the derivative values for a given function f(x) = 4x - x^2 at specific points using the limit definition.
Observation that the process to find f'(a) is consistent regardless of the value of a, leading to conjectures about f'(4) and f'(5).
Derivation of a general formula for f'(a) based on the observed pattern in derivative values.
Explanation of the process to find f'(0) by simplifying the limit definition and factoring out h.
Calculation of f'(1), demonstrating the simplification of the function and taking the limit as h approaches zero.
Finding f'(2) and f'(3), illustrating the pattern of the derivative decreasing by 2 as the input value increases by 1.
Conjecture that f'(4) might be -4 and f'(5) might be -6 based on the observed pattern.
General formula conjecture for f'(a) as -2a + 4, derived from the table of values.
Application of the conjectured formula to find f'(100), demonstrating the practicality of the derived formula.
Differentiation between the derivative at a specific point (f'(a)) and the derivative function (f'(x)) and their respective uses.
Graphical representation of the tangent lines at various points on the graph of f(x).
Introduction to the process of graphing the derivative function without an explicit equation.
Use of the graph of f(x) to estimate the graph of its derivative, including identifying points where the derivative is zero.
Practice examples of finding the derivative function for various types of functions, including linear and constant functions.
Derivation of the derivative function for the square root function, showcasing the use of algebraic manipulation.
Final discussion on interpreting the derivative in the context of real problems, setting the stage for future lessons.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: