Using Slater's Rules: 3 Examples

chemistNATE
2 Dec 201211:12
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into Slater's rules for calculating the effective nuclear charge (Zeff) experienced by electrons in an atom. It explains the process step-by-step, starting with writing the electron configuration, rearranging it, and then applying specific rules for different types of electrons like 3D, 5S, and 1s. The script provides examples using iron and strontium to demonstrate how to determine Zeff, highlighting the importance of shielding and its impact on an electron's interaction with the nucleus.

Takeaways
  • 📘 Slater's rules are a method for calculating the effective nuclear charge (Zeff) experienced by an electron in an atom.
  • 🔍 To find Zeff, you first need to write the electron configuration of the atom itself, following the order of filling.
  • 🔄 Rearrange the electron configuration from highest to lowest principal quantum number (n), grouping S and P electrons together.
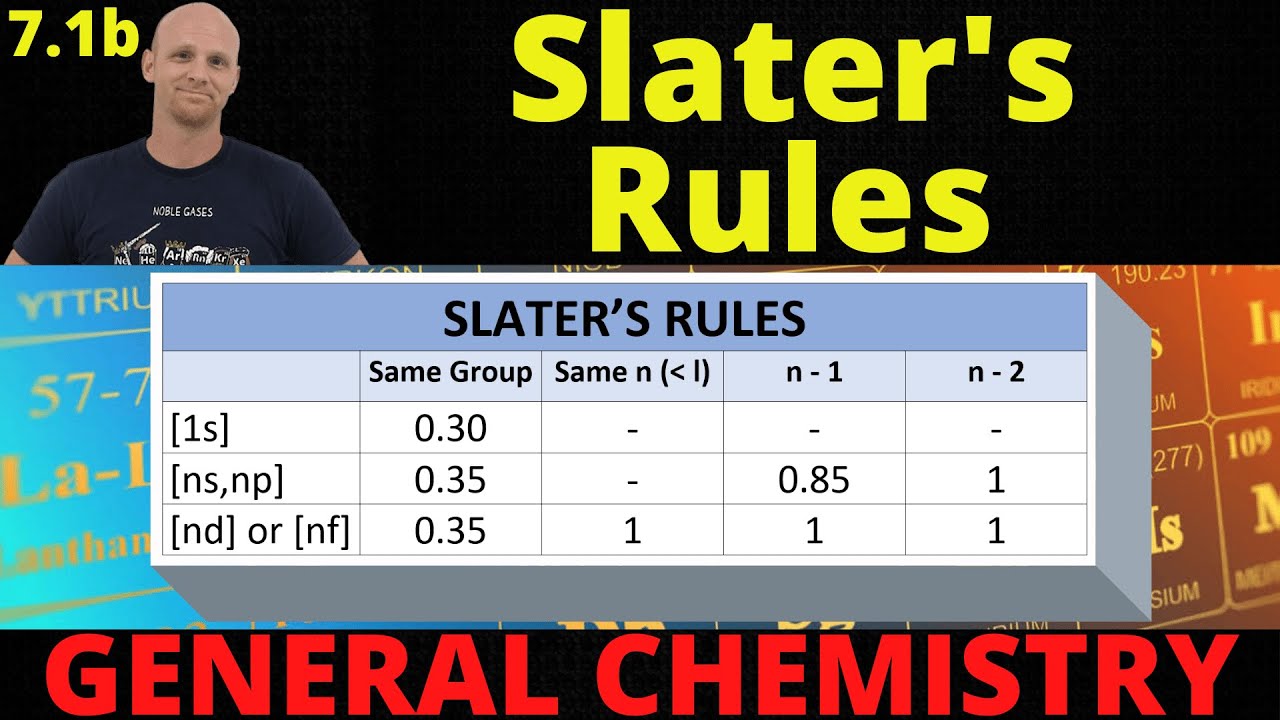
  • 🌟 For d or f electrons, the rule is to add 35 for each electron in the same group and one for each electron in a lower group.
  • 💡 The effective nuclear charge is calculated by subtracting the total shielding effect from the atomic number.
  • ⚙️ For a 3D electron in iron, the shielding calculation involves subtracting values based on electrons in the same and lower groups.
  • 🧲 The 3D electron in iron effectively 'sees' only 6.25 protons due to the shielding effect, which influences its removal ease.
  • 📊 For a 5S electron in strontium, the shielding calculation includes additional factors for electrons one and two or more shells lower.
  • 🔢 The 5S electron in strontium experiences a much lower Zeff of 2.85, indicating it is less influenced by the nucleus due to its distance and shielding.
  • 🚫 The only exception to the rules is for 1s electrons, where the total shielding is always 3, regardless of the atom.
  • 🎓 Understanding Slater's rules is crucial for students aiming to excel in chemistry, particularly in tests focused on atomic structure and electron configurations.
Q & A

  • What are Slater's rules used for?

    -Slater's rules are used to calculate the effective nuclear charge (Zeff) on an electron in an atom, which is the actual amount of positive charge that the electron experiences due to the presence of protons in the nucleus and the shielding effect of other electrons.

  • How does the number of protons in the nucleus affect the electron?

    -The number of protons in the nucleus determines the atomic number of an element. However, an electron far from the nucleus does not experience the full positive charge due to the shielding effect of other electrons, resulting in an effective nuclear charge that is less than the atomic number.

  • What is the significance of the electron configuration in Slater's rules?

    -The electron configuration is essential in Slater's rules because it provides the distribution of electrons in the atom, which is necessary to calculate the shielding effect on a specific electron.

  • Why is the order of filling important in the context of Slater's rules?

    -The order of filling is important because it dictates the sequence in which electrons are added to the atomic orbitals. This sequence affects the arrangement of electrons for the purpose of calculating the effective nuclear charge.

  • How are electrons in the same group treated in Slater's rules?

    -In Slater's rules, electrons in the same group (S and P orbitals with the same principal quantum number) are grouped together for the calculation of the effective nuclear charge.

  • What is the significance of the principal quantum number (n) in Slater's rules?

    -The principal quantum number (n) is significant because it determines the energy level of the electron and its distance from the nucleus. Slater's rules require the electron configuration to be rearranged from the lowest to the highest n value for the calculation.

  • How do you calculate the shielding effect for a 3D electron according to Slater's rules?

    -For a 3D electron, you add 35 for each electron in the same group (3D), and subtract 1 for each electron in a lower group. The total shielding is then used to calculate the effective nuclear charge.

  • What is the effective nuclear charge for a 3D electron in an iron (Fe) atom?

    -For a 3D electron in an iron atom, the effective nuclear charge is calculated by subtracting the total shielding (19.75) from the atomic number of iron (26), resulting in an effective nuclear charge of 6.25.

  • How does the effective nuclear charge differ for a 5S electron in strontium (Sr)?

    -For a 5S electron in strontium, the effective nuclear charge is calculated by considering the shielding from electrons in the same group (35), one shell lower (85), and two or more shells lower (1). The result is an effective nuclear charge of 2.85.

  • What is the special rule for calculating the effective nuclear charge of a 1s electron?

    -For a 1s electron, the total shielding is always 3, regardless of the element, because only the other 1s electron contributes to the shielding, and it does so by a constant amount.

  • Why is the effective nuclear charge for a 1s electron almost equal to the atomic number?

    -The effective nuclear charge for a 1s electron is almost equal to the atomic number because the 1s electron is in the closest shell to the nucleus and is not significantly shielded by electrons in higher energy levels.

Outlines
00:00
🔬 Understanding Slater's Rules for Effective Nuclear Charge

This paragraph introduces Slater's rules, a method for calculating the effective nuclear charge experienced by an electron in an atom. The narrator explains that electrons don't experience the full positive charge of the nucleus due to shielding by other electrons. The process begins with writing the electron configuration of the atom, followed by rearranging and grouping the electrons based on their energy levels. For a 3D electron in iron, the calculation involves adding 35 for each electron in the same group and one for each electron in a lower group, resulting in a total shielding effect. The effective nuclear charge is then found by subtracting this shielding from the atomic number, revealing how much positive charge the electron effectively 'sees'.

05:01
📚 Applying Slater's Rules to Strontium's 5S Electron

The second paragraph delves into applying Slater's rules to calculate the effective nuclear charge for a 5S electron in strontium, element number 38. The electron configuration is written out, and electrons are rearranged and grouped by their principal quantum number. For S electrons, the shielding calculation includes adding 35 for each electron in the same group, 85 for each electron one shell lower, and one for each electron two or more shells lower. After summing up the shielding values, the effective nuclear charge is calculated by subtracting the total shielding from the atomic number, showing that the 5S electron experiences significantly less positive charge due to the distance from the nucleus and shielding by other electrons.

10:02
🌟 Special Consideration for 1s Electrons' Effective Nuclear Charge

The final paragraph addresses a special case in Slater's rules for calculating the effective nuclear charge of a 1s electron. Unlike other electrons, a 1s electron's view of the nucleus is only blocked by the other 1s electron, contributing a constant shielding of 3. The example given is for neodymium, where the 1s electron sees almost all of the protons due to its proximity and minimal shielding. The narrator acknowledges the contrived nature of Slater's rules but emphasizes their effectiveness in educational settings, encouraging learners to master the method for success in related assessments.

Mindmap
Keywords
💡Slater Rules
Slater Rules are a set of guidelines used to calculate the effective nuclear charge (Zeff) experienced by an electron in an atom. In the video, Slater Rules are the central methodology for determining how much positive charge an electron 'sees' due to the presence of other electrons in the atom. For example, the script explains how to apply Slater Rules to calculate the Zeff for a 3D electron in iron, emphasizing the importance of understanding electron shielding.
💡Effective Nuclear Charge (Zeff)
Effective Nuclear Charge, or Zeff, is the net positive charge experienced by an electron due to the nucleus and other electrons in an atom. The script explains that Zeff is less than the actual number of protons in the nucleus because inner electrons shield the outer electrons from the full charge. The concept is crucial in understanding chemical properties and electron behavior, as illustrated with the 3D electron in iron and the 5S electron in strontium.
💡Electron Configuration
Electron Configuration refers to the distribution of electrons in an atom's orbitals. The script begins by emphasizing the need to write the electron configuration for an atom, such as iron, to apply Slater Rules. This configuration is essential for understanding the arrangement of electrons and how they contribute to the effective nuclear charge.
💡Order of Filling
Order of Filling is the sequence in which atomic orbitals are filled with electrons. The video script mentions the order of filling when explaining the electron configuration of iron, where 4S comes before 3D, which is a deviation from the typical n+1 rule due to the specific energy levels of these orbitals.
💡Shielding
Shielding in the context of atomic structure refers to the phenomenon where inner electrons reduce the effective nuclear charge experienced by outer electrons. The script explains how to calculate the shielding effect for different types of electrons, such as the 3D electron in iron, by considering electrons in the same group and lower groups.
💡Atomic Number
Atomic Number represents the number of protons in an atom's nucleus and is unique to each element. In the script, the atomic number is used to start the calculation of Zeff, as it sets the initial positive charge that will be adjusted by the shielding effect of other electrons.
💡Strontium
Strontium is a chemical element with the atomic number 38. The script uses strontium as an example to demonstrate the calculation of Zeff for a 5S electron, showing how the electron configuration and shielding affect the final Zeff value.
💡Neodymium
Neodymium is a rare earth element used in the script to illustrate the special case of calculating Zeff for a 1s electron. The script explains that the shielding for a 1s electron is always 3, regardless of the element, because only the 1s electrons themselves contribute to the shielding effect.
💡Electron Shells
Electron Shells are regions around the nucleus where electrons are most likely to be found. The script discusses electron shells when explaining the electron configuration and the shielding effect, particularly noting that electrons in the same shell or one shell lower have a significant impact on the Zeff.
💡Orbitals
Orbitals are regions within an electron shell where electrons reside. The script refers to different types of orbitals, such as s, p, and d orbitals, when explaining the electron configuration and the application of Slater Rules. The type of orbital is important for determining the shielding effect on electrons.
Highlights

Slater's rules are explained for calculating the effective nuclear charge on an electron in an atom.

Three types of questions involving effective nuclear charge calculations are introduced.

The concept of effective nuclear charge as the actual amount of positive charge an electron 'sees' is clarified.

Step-by-step guide begins with writing the electron configuration for the atom.

Electron configurations are rearranged from highest to lowest principal quantum number (n).

Grouping of s and p orbitals with the same n value is explained for Slater's rules.

Different rules for calculating effective nuclear charge are provided for d or f electrons.

A numerical example is given for calculating the effective nuclear charge of a 3d electron in iron.

The significance of shielding in determining the effective nuclear charge is discussed.

The final effective nuclear charge is calculated by subtracting shielding from the atomic number.

A second example is provided for calculating the effective nuclear charge of a 5s electron in strontium.

Rules for s electrons are detailed, including additional shielding factors.

The effective nuclear charge for a 1s electron is a special case with a fixed shielding value.

The practical implications of effective nuclear charge on the ease of electron removal are mentioned.

The transcript acknowledges the contrived nature of Slater's rules but emphasizes their utility.

Encouragement is given for students to learn Slater's rules for academic success.

Transcripts

Browse More Related Video

7.1b Slater's Rules | General Chemistry

How To Calculate The Effective Nuclear Charge of an Electron

Effective Nuclear Charge, Shielding effect, & Periodic Properties Tutorial; Crash Chemistry Academy

Effective Nuclear Charge - Chemistry Tutorial

Trick for Slater's Rule, calculation of screening constant and effective nuclear charge.

Electron Configuration - Basic introduction

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Slater RulesEffective Nuclear ChargeElectron ConfigurationAtomic StructureChemistry EducationPeriodic TableElectron ShieldingQuantum MechanicsPhysics ConceptsEducational Content