Descriptive Statistics: The Mode
TLDRIn this educational video, the concept of mode as a measure of central tendency is introduced, drawing from its Latin and French roots to convey its statistical significance. The video explains the mode's definition as the most frequent observation in a dataset and contrasts its behavior with the mean and median. It demonstrates the mode's instability in small samples and its reliability with larger ones, using a census example. The presenter also explores the relationships between mode, median, and mean in various data distributions, including symmetric and skewed scenarios. A challenging question is posed, asking viewers to create a dataset where the median is less than the mode, which is less than the mean, stimulating critical thinking and engagement.
Takeaways
- 📚 The mode is derived from Latin 'modus' and is related to the concept of 'fashionable' or 'most frequent' observation in statistics.
- 🔍 The mode is defined as the value with the highest frequency in a data set, making it a measure of central tendency.
- 🤔 The mode can be sensitive and less reliable in small samples, as changing a single observation can affect its determination.
- 📈 In larger samples, such as a census, the mode becomes more stable and representative of the central tendency.
- 📊 The mode can be visualized in a bar chart, where the highest bar represents the mode.
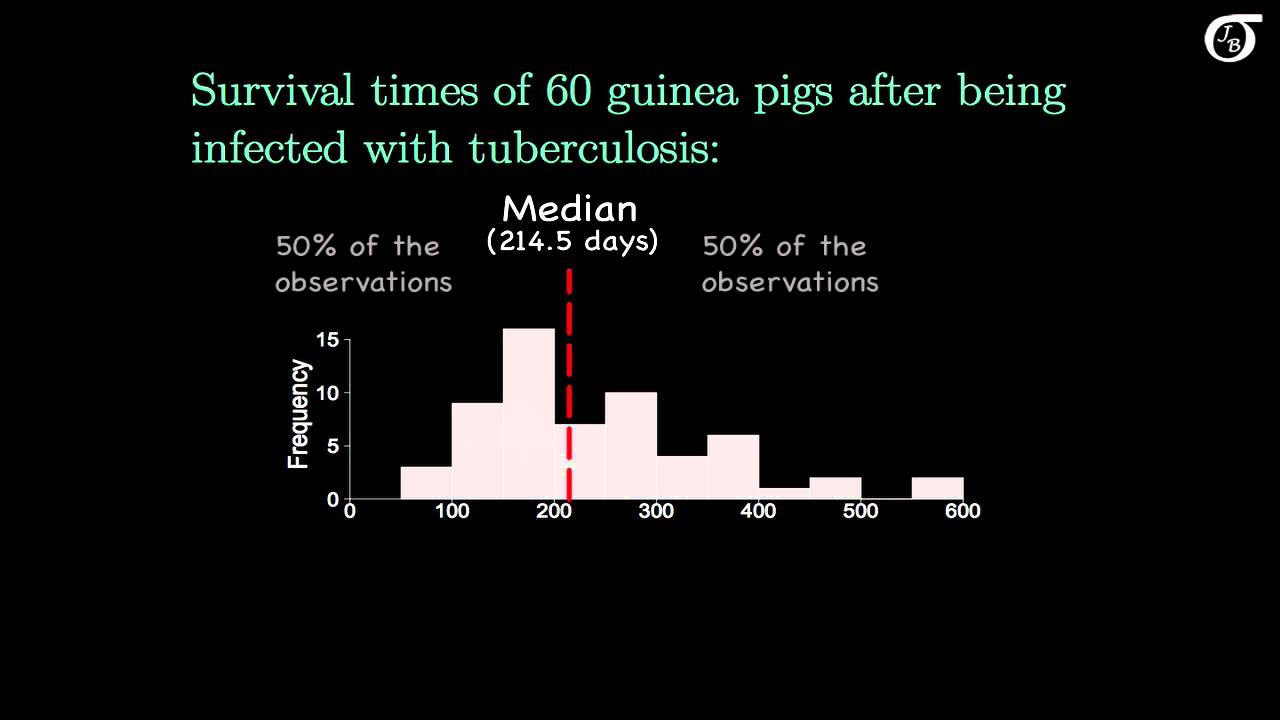
- 🔢 The median is the middle value in an ordered data set, and in the given example, it is also two children per family.
- 🧮 The mean is calculated by multiplying each value by its frequency, summing them, and dividing by the total number of observations.
- 📊 In symmetric distributions, the mean, median, and mode coincide and are all located at the center.
- 📈 In bimodal distributions, there are two modes of equal frequency, which can complicate the representation of central tendency.
- 📉 In positively skewed distributions, the mode is less than the median, which in turn is less than the mean, due to the influence of extreme values.
- 💡 The challenge is to create a data set with integer values from 0 to 10 where the median is less than the mode, which is less than the mean, reversing the typical relationship.
Q & A
What is the definition of the mode in statistics?
-The mode is the observation with the highest frequency in a dataset.
How does the mode differ from the mean and the median?
-The mode is the most frequently occurring value, while the mean is the average of all values, and the median is the middle value when the data is ordered.
Why is the mode considered unreliable for small samples?
-In small samples, minor changes in the data can drastically alter the mode, making it less stable and less representative of central tendency.
How does the mode behave in a large dataset, such as a census?
-In large datasets, the mode becomes more stable and representative. For example, in a census, the mode can indicate the most common number of children per family.
What is a bimodal distribution?
-A bimodal distribution has two modes, or two values that occur with the highest frequency.
How do the mean, median, and mode relate in a symmetric distribution?
-In a symmetric distribution, the mean, median, and mode are all equal and located at the center of the distribution.
What happens to the mean, median, and mode in a positively skewed distribution?
-In a positively skewed distribution, the mode is the lowest, followed by the median, and the mean is the highest.
Why might the median be preferred over the mean in some cases, such as house prices?
-The median is less affected by extreme values, making it a better measure of central tendency for skewed distributions like house prices, where a few high values can significantly raise the mean.
What challenge question does the video pose regarding skewed data distributions?
-The challenge is to create a sample of integer values from 0 to 10 where the median is less than the mode, and the mode is less than the mean.
What is the importance of understanding the relationship between mean, median, and mode in statistical analysis?
-Understanding these relationships helps in choosing the appropriate measure of central tendency for different datasets and provides insights into the distribution's characteristics.
Outlines
📊 Introduction to Mode and Its Properties
The video begins with an introduction to the concept of mode, which is derived from the Latin word 'modus' meaning mood or fashion. It explains that the mode in statistics is the value that appears most frequently in a data set, using the example of the number 28 being the mode in a given dataset. The presenter notes that the mode can be sensitive in small samples, as changing a single observation can alter the mode. However, with larger samples, such as a census, the mode becomes more stable and representative. The video also poses a challenge question at the end of this section, inviting viewers to consider the relationships between mode, mean, and median.
🔍 Relationships Among Mode, Median, and Mean
This paragraph explores the relationships between mode, median, and mean in different types of data distributions. It starts by explaining that in a symmetric distribution, such as a normal distribution, the mode, median, and mean all coincide at the center. However, in a bimodal distribution, there are two modes of equal frequency, and the mean and median may not coincide with the modes. The discussion then moves to asymmetric distributions, specifically positively skewed ones, where the mode is the most frequent value, the median is slightly higher, and the mean is significantly higher due to the influence of a long tail of high values. The video uses the example of house prices to illustrate how these measures can be used in different contexts. Finally, the presenter challenges viewers to create a dataset where the median is less than the mode, which in turn is less than the mean, reversing the typical order observed in positively skewed distributions.
Mindmap
Keywords
💡Mode
💡Mean
💡Median
💡Central Tendency
💡Skewed Distribution
💡Bimodal
💡Frequency
💡Census
💡Weighted Mean
💡Challenge Question
Highlights
The mode is defined as the observation with the highest frequency in a dataset.
The term 'mode' originates from Latin 'modus' and has connections to music and fashion.
The mode can be fickle in small samples, changing with the modification of a single observation.
In larger samples, the mode can be a more reliable measure of central tendency.
An example of mode in a large dataset is given by the Australian census question on family children.
The mode can be visualized in a bar chart, showing the most frequent observation.
The median is the middle observation in an ordered dataset.
The mean is calculated by summing all observations and dividing by the number of observations.
In symmetric distributions, the mean, median, and mode are all equal and represent the center.
Bimodal distributions have two modes of equal frequency.
In positively skewed distributions, the mode is less than the median, which is less than the mean.
The mean can be influenced by a long tail of high-value observations in a distribution.
Different measures of central tendency are appropriate depending on the context of the data.
The presenter challenges viewers to create a dataset where the median is less than the mode, which is less than the mean.
The challenge requires a dataset with integer values from 0 to 10 and a single mode.
The video encourages viewers to share their solutions in the comments section.
The presenter, Justin Seltzer, invites feedback and interaction from viewers.
Transcripts
Browse More Related Video
Measures of Central Tendency
Descriptive Statistics: The median
Mean, Median and Mode in Statistics | Statistics Tutorial | MarinStatsLectures

Mean, Median and Mode - Measures of Central Tendency

Mean, Median, and Mode: Measures of Central Tendency: Crash Course Statistics #3

Skewness - Right, Left & Symmetric Distribution - Mean, Median, & Mode With Boxplots - Statistics
5.0 / 5 (0 votes)
Thanks for rating: