AP Physics C: Rotational Dynamics Review - 1 of 2 (Mechanics)
TLDRThis educational video script from Flipping Physics dives into the principles of rotational dynamics for AP Physics C Mechanics. It begins by explaining the concept of kinetic energy in rotating objects and introduces the moment of inertia, highlighting its units and its role as 'rotational mass.' The script proceeds to derive moment of inertia for various objects like a uniform thin hoop and rod, emphasizing the differences in calculations based on the axis of rotation. It also covers the parallel axis theorem, torque, and its vector nature, and introduces the rotational form of Newton's second law. The video concludes with discussions on rolling without slipping and the importance of memorizing key equations, offering a comprehensive review of rotational dynamics.
Takeaways
- 📚 The video script is a review of rotational dynamics as part of the AP Physics C Mechanics curriculum.
- 🚀 Total kinetic energy of a rotating object is the sum of the kinetic energies of each piece of the object, treating it as a system of particles.
- 🔍 Kinetic energy for each particle is calculated using the formula \( \frac{1}{2} \) mass times velocity squared, with tangential velocity being radius times angular velocity.
- 🌌 Moment of inertia is defined as the sum of the mass of each particle times the square of the distance from the axis of rotation, and it's analogous to mass in linear motion.
- 📐 The units for moment of inertia are kilograms times meters squared, sometimes referred to as 'rotational mass'.
- 📉 The moment of inertia for a rigid object with shape is found using an integral, summing \( r^2 \) with respect to mass for each infinitesimally small piece of the object.
- 🛠 The moment of inertia of a uniform thin hoop about its cylindrical axis is the mass of the hoop times the radius squared.
- 🔨 The moment of inertia of a uniform rigid rod about its center of mass is \( \frac{1}{12} \) times the mass times the length squared.
- 🔄 The parallel axis theorem allows calculating the moment of inertia about an axis parallel to and a distance away from the center of mass.
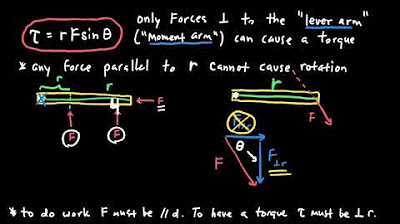
- ⚙️ Torque (τ) is a vector and is calculated as the product of the distance from the axis of rotation to the point of force application, the force magnitude, and the sine of the angle between them.
- 🤔 Torque is the rotational equivalent of force and causes angular acceleration, similar to how force causes linear acceleration.
Q & A
What is the total kinetic energy of a rotating object in terms of its individual pieces?
-The total kinetic energy of a rotating object equals the sum of the kinetic energies of each piece of the object, treating the object as a system of particles.
How is the kinetic energy of each particle in a rotating object calculated?
-The kinetic energy of each particle is calculated using the formula one half times mass times the tangential velocity squared, where the tangential velocity is the radius times the angular velocity of the particle.
What is the moment of inertia and how is it defined?
-Moment of inertia is a measure of an object's resistance to rotational motion. It is defined as the sum of the mass of each particle times the square of the distance from the axis of rotation (r squared).
What are the units for moment of inertia and why is it sometimes called rotational mass?
-The units for moment of inertia are kilograms times meters squared (kg·m²). It is sometimes called rotational mass because its units are similar to linear mass, reflecting its role in rotational dynamics analogous to mass in linear dynamics.
How can the moment of inertia of a rigid object with shape be defined using an integral?
-The moment of inertia of a rigid object with shape can be defined as the integral with respect to mass of r squared, where r is the distance each infinitesimally small piece of the object is from the object's axis of rotation.
What is the difference between the moment of inertia equations and the equation for the position of the center of mass?
-The moment of inertia equation involves the integral of r squared with respect to mass, while the center of mass equation involves the integral of r with respect to mass. They are often confused due to their similarity but represent different physical properties.
How is the moment of inertia of a uniform, thin hoop about its cylindrical axis derived?
-The moment of inertia of a uniform, thin hoop about its cylindrical axis is derived by integrating r squared with respect to mass, where r is a constant equal to the hoop's radius, leading to the formula mass times the radius squared.
What is the moment of inertia of a uniform, rigid rod about its center of mass?
-The moment of inertia of a uniform, rigid rod about its center of mass is derived to be 1/12 times the mass of the rod times the length of the rod squared.
How does the parallel axis theorem relate the moment of inertia about the center of mass to another axis parallel to it?
-The parallel axis theorem states that the moment of inertia about some other axis is equal to the moment of inertia about the center of mass plus the mass of the object times the square of the distance (D squared) between the new axis of rotation and the center of mass.
What is torque and how is it calculated?
-Torque is a measure of the force that can cause an object to rotate and is calculated as the cross product of the distance vector (r) and the force vector (F), or as r F sine theta, where r is the distance from the axis of rotation, F is the force magnitude, and theta is the angle between r and F.
What is the relationship between torque and angular acceleration according to the rotational form of Newton's second law?
-The rotational form of Newton's second law states that net torque equals moment of inertia times angular acceleration, similar to how net force equals mass times linear acceleration in the linear form of the law.
How is the direction of torque determined?
-The direction of torque is determined using the right-hand rule, where you point your fingers along the direction of r, curl them towards the direction of the force, and your thumb points in the direction of the torque.
What are the conditions for an object rolling without slipping?
-An object rolls without slipping when the velocity of the center of mass equals the radius times the angular velocity, and the acceleration of the center mass equals the radius times the angular acceleration.
Outlines
📚 Introduction to Rotational Dynamics
This paragraph introduces the concept of rotational dynamics within the AP Physics C Mechanics curriculum. It explains the kinetic energy of a rotating rigid object, which is treated as a system of particles. Each particle's kinetic energy is summed up to find the total kinetic energy of the object. The concept of tangential velocity is introduced, and the moment of inertia is defined as the sum of the mass of each particle times the square of its distance from the axis of rotation. The rotational kinetic energy formula is derived, and the units for moment of inertia are discussed, highlighting its similarity to mass in linear motion, hence the term 'rotational mass'. The paragraph also differentiates between the equations for moment of inertia and the center of mass, and it concludes with an introduction to deriving the moment of inertia for specific shapes like a uniform thin hoop.
🔍 Deriving Moment of Inertia for Different Shapes
The second paragraph delves into the derivation of moment of inertia for different shapes, starting with a uniform thin hoop rotating about its cylindrical axis. The integral expression for moment of inertia is simplified by recognizing that all mass elements are at a constant distance from the axis of rotation. The moment of inertia for a uniform thin rod about its center of mass is derived next, using linear mass density and integral calculus. The limits of integration are set based on the rod's length, and the resulting moment of inertia is expressed in terms of the rod's mass and length. The paragraph also covers the moment of inertia for a rod about one end, using similar integral calculus techniques but with different limits. The parallel axis theorem is introduced, which allows calculating the moment of inertia about an axis parallel to the center of mass by adding the mass times the square of the distance from the center of mass to the new axis of rotation.
🔧 Understanding Torque and Its Calculation
This paragraph explores the concept of torque, its definition, and its calculation. Torque is represented by the Greek letter tau and is calculated as the product of the distance from the axis of rotation to the point of force application (r), the force (F), and the sine of the angle (theta) between r and F. The paragraph explains that torque is a vector and discusses the physical interpretation of r, F, and theta. It also introduces the concept of the moment arm or lever arm, which is the perpendicular distance from the axis of rotation to the line of action of the force. The right-hand rule is highlighted as a tool to determine the direction of torque, and the paragraph concludes with a discussion on the relationship between torque and energy, noting that while torque is measured in newton meters, it is distinct from energy measured in joules.
🚀 Rotational Dynamics: Newton's Second Law and Rolling Without Slipping
The final paragraph discusses the rotational form of Newton's second law, which relates net torque to moment of inertia and angular acceleration. It emphasizes the importance of identifying the objects, axis of rotation, and positive direction when summing torques. The paragraph also touches on the concept of rolling without slipping, providing equations that describe the relationship between the velocity and acceleration of the center of mass and the angular velocity and acceleration of an object. The significance of these equations in the context of rotational dynamics is highlighted, and the paragraph concludes with a reminder that these equations must be memorized as they are not provided on the AP equation sheet. The review ends with an invitation to continue learning about rotational dynamics through further review sessions or the AP Physics C review web page.
Mindmap
Keywords
💡Rotational Dynamics
💡Moment of Inertia
💡Kinetic Energy
💡Angular Velocity
💡Rigid Object
💡System of Particles
💡Rotational Kinetic Energy
💡Parallel Axis Theorem
💡Torque
💡Right-Hand Rule
Highlights
Introduction to rotational dynamics as part of the AP Physics C Mechanics curriculum.
Explanation of kinetic energy in a rotating rigid object as the sum of kinetic energies of individual pieces.
Derivation of the equation for kinetic energy, including the substitution of tangential velocity.
Introduction and definition of moment of inertia as a sum of mass times the square of the radius.
The equation for rotational kinetic energy in terms of moment of inertia and angular velocity squared.
Discussion on the units of moment of inertia and its comparison to rotational mass.
Clarification of the difference between moment of inertia and center of mass equations.
Derivation of moment of inertia for a uniform, thin hoop about its cylindrical axis.
Explanation of the terms 'uniform', 'thin', and 'cylindrical axis' in the context of a hoop.
Derivation of moment of inertia for a uniform, thin rod about its center of mass.
Use of linear mass density to find moment of inertia of a rod about its center of mass.
Calculation of moment of inertia for a uniform, rigid rod about one end.
Introduction and explanation of the parallel axis theorem.
Application of the parallel axis theorem to find moment of inertia about an end of a uniform rod.
Example using the parallel axis theorem for a uniform, thin hoop about its rim.
Introduction to the concept of torque, its definition, and components.
Explanation of torque as a vector and its magnitude in terms of r, F, and theta.
Discussion on the units of torque and its equivalence to joules.
Clarification of torque as a cause of angular acceleration, akin to a rotational force.
Introduction to the rotational form of Newton's second law relating net torque, moment of inertia, and angular acceleration.
Emphasis on identifying objects, axis of rotation, and positive direction when summing torques.
Implication of mass on pulleys affecting the tension forces on either side.
Use of the right-hand rule to determine the direction of torque.
Conditions for rolling without slipping and their corresponding equations.
Differentiation between rolling without slipping and rolling with slipping.
Importance of memorizing equations for rolling without slipping not on the AP equation sheet.
Transcripts
Browse More Related Video

College Physics 1: Lecture 25 - Moment of Inertia and Rotational Dynamics
AP Physics 1 Torque and Rotational Motion Review
AP Physics 1: Rotational Dynamics Review

AP Physics 1 - Rotational Dynamics

Moment of Inertia | Physics with Professor Matt Anderson | M12-05

10. Rotations, Part II: Parallel Axis Theorem
5.0 / 5 (0 votes)
Thanks for rating: