10. Rotations, Part II: Parallel Axis Theorem
TLDRIn this engaging lecture, Professor Ramamurti Shankar delves into the dynamics of rotations, focusing on the concepts of moment of inertia, angular momentum, and torque. He introduces the Parallel Axis Theorem and its applications, emphasizing the importance of the center of mass in calculating moments of inertia and kinetic energy. Through illustrative examples, including the rolling tire and the pulley system, Shankar demonstrates how to analyze complex motion involving both translation and rotation, highlighting the conservation of energy and angular momentum principles. The lecture also touches on the practical applications of these concepts, such as in the case of an ice skater's spin, where reducing the moment of inertia results in an increase in rotational speed and kinetic energy.
Takeaways
- 📚 The fundamental concept of rotational motion involves a body rotating around a point, with key quantities including the point of rotation, mass (M), moment of inertia (I), angular velocity (ω), angular momentum (L), and torque (τ).
- 🌀 The moment of inertia depends on the specific point around which the body is rotating and can be calculated using the Parallel Axis Theorem, which states that I = I_CM + Md^(2), where I_CM is the moment of inertia about the center of mass and d is the distance from the center of mass to the chosen axis.
- 🔧 Torque is the rotational equivalent of force and is given by τ = r × F × sin(θ), where r is the distance from the point of rotation, F is the applied force, and θ is the angle between the force and the radius vector.
- 🚀 The conservation of angular momentum is a key principle in rotational dynamics, stating that the total angular momentum of an isolated system remains constant when no external torques are applied.
- 🎢 In the absence of external torques, the kinetic energy of a rotating body can be expressed as the sum of the kinetic energy of the center of mass translation (½ MV^(2)) and the kinetic energy of rotation about the center of mass (½ Iω^(2)).
- 🛠️ The kinetic energy of a rolling object (like a wheel) can be calculated using the combined translational and rotational energies, with the rotational energy being specific to the point of contact with the ground.
- 🧗 The dynamics of a system with pulleys and masses involves both translational and rotational motion, where the acceleration of the mass is related to the angular acceleration of the pulley by the radius of the pulley.
- 🏎️ For a rotating tire or disk, the kinetic energy is not just due to its translational motion but also includes the rotational energy, which can be calculated using the moment of inertia at the center of mass and the angular velocity.
- 🎪 The Icecapade example demonstrates how changing the moment of inertia (e.g., by pulling in the arms) can increase the angular velocity and thus the kinetic energy of a rotating body without changing its angular momentum.
- 💡 The increase in kinetic energy when a figure skater spins faster comes from the work done by the skater's muscles to bring the dumbbells closer to the body, which is analogous to lifting weights in a zero-gravity environment.
- 🔄 The conservation of energy principle is a powerful tool for verifying the correctness of physical calculations, as it ensures that the total mechanical energy of an isolated system remains constant throughout the motion.
Q & A
What is the main concept of the second part of the lecture?
-The main concept of the second part of the lecture is the rotation of rigid bodies, focusing on the essential ideas such as the point of rotation, mass, moment of inertia, angular momentum, torque, and the Parallel Axis Theorem.
How is the moment of inertia related to the point of rotation?
-The moment of inertia is related to the point of rotation in that it varies depending on the distance from the point of rotation. For a discrete set of points, it is the sum of the product of each point's mass and the square of its distance from the point of rotation. For a continuous distribution, this sum is replaced by an integral.
What is the Parallel Axis Theorem and how does it simplify calculations?
-The Parallel Axis Theorem states that if you know the moment of inertia with respect to the center of mass, you can find the moment of inertia with respect to any other parallel axis by adding the mass of the entire body times the square of the distance between the center of mass and the new axis. This simplifies calculations as it eliminates the need to perform the integral all over again for different axes.
How does the kinetic energy of a system relate to the center of mass?
-The kinetic energy of a system can be expressed as the kinetic energy associated with the center of mass motion plus the kinetic energy relative to the center of mass. This is true for any collection of masses, whether they form a rigid body or are loosely connected particles.
What is the significance of the center of mass in the study of rigid bodies?
-The center of mass is significant in the study of rigid bodies because it is the point around which the body can rotate without any translational motion relative to the body itself. It is also the point that simplifies calculations, as the momenta of all particles relative to the center of mass sum up to zero, and the kinetic energy can be separated into translational and rotational parts.
How does the lecture connect rotational motion to linear motion in the context of rolling without slipping?
-The lecture connects rotational motion to linear motion by showing that when a body, like a tire, rolls without slipping, the linear velocity of the center of mass is equal to the angular velocity times the radius of the tire. This relationship allows for the calculation of the kinetic energy of the tire, which includes both translational and rotational components.
What is the relationship between angular momentum and torque?
-Angular momentum (L) is related to torque (τ) through the equation τ = Iα, where I is the moment of inertia and α is the angular acceleration. This relationship is analogous to the linear momentum equation (P = mv), where P is the linear momentum, m is the mass, and v is the linear velocity.
How does the moment of inertia change when a mass is added to a rotating body?
-When a mass is added to a rotating body, the moment of inertia changes depending on the distance of the added mass from the axis of rotation. For a rigid body like a disk, the moment of inertia is given by I = MR^2/2, where M is the mass and R is the radius. Adding a mass m at a distance r from the center increases the moment of inertia by m*r^2.
What is the significance of the conservation of angular momentum in physics?
-The conservation of angular momentum is a fundamental principle in physics that states that in the absence of external torques, the angular momentum of a system remains constant. This principle is crucial for understanding and analyzing rotational motion, as it allows us to predict how a system will behave when subjected to internal forces or changes in configuration.
What is the role of the Parallel Axis Theorem in calculating the moment of inertia of complex objects?
-The Parallel Axis Theorem plays a crucial role in calculating the moment of inertia of complex objects by allowing us to find the moment of inertia about any axis parallel to a known axis (usually the center of mass). It simplifies the calculation by stating that the moment of inertia about the new axis is equal to the moment of inertia about the parallel axis through the center of mass plus the mass of the body times the square of the distance between the two axes.
How does the lecture illustrate the concept of angular acceleration?
-The lecture illustrates the concept of angular acceleration through the equation τ = Iα, where τ is the torque applied to the rigid body, I is the moment of inertia, and α is the angular acceleration. It explains that angular acceleration is the rotational counterpart to linear acceleration and is the rate of change of angular velocity, similar to how linear acceleration is the rate of change of linear velocity.
Outlines
📚 Introduction to Rotations and Key Concepts
Professor Ramamurti Shankar begins the lecture by summarizing the main points from the previous session on rotations of rigid bodies. He emphasizes the importance of understanding the fundamental concepts, such as the point of rotation, mass (M), moment of inertia, angular velocity (ω), angular momentum (L), and torque (τ). The professor also introduces the Parallel Axis Theorem and its significance in calculating the moment of inertia with respect to different points. The lecture aims to provide a solid foundation for the students to build upon as they delve deeper into the topic of rigid body rotations.
🔄 Calculation of Moment of Inertia and Parallel Axis Theorem
The professor continues the discussion on the moment of inertia, explaining how it can be calculated for different objects, such as a rod and a disk, and how it varies depending on the point of rotation. He provides detailed calculations for the moment of inertia for a rod around its ends and the center of mass, and contrasts these with the calculations for a disk. The Parallel Axis Theorem is further elaborated, with Shankar highlighting its usefulness in simplifying the calculation of moment of inertia for any point by using the known moment of inertia at the center of mass. The lecture reinforces the understanding of rotational analogs to linear motion concepts and the importance of the center of mass in these calculations.
🌀 Proof of the Parallel Axis Theorem and Vector Calculations
Professor Shankar delves into the proof of the Parallel Axis Theorem, using vector calculations to demonstrate how the theorem is derived. He introduces the concept of finding the length of a vector through the dot product and applies this to the calculation of moment of inertia around a point. The professor walks through the proof step by step, explaining how the theorem allows for the simplification of calculations by eliminating the need to redo the integral for the moment of inertia when switching between points. The lecture emphasizes the importance of understanding the underlying principles and mathematical techniques to apply the theorem effectively.
🚀 Application of the Parallel Axis Theorem to Practical Scenarios
The professor illustrates the practical application of the Parallel Axis Theorem using the example of a coin or a disk. He explains how the theorem can be used to simplify the calculation of the moment of inertia when an object is rotated around an axis through a point other than the center of mass. The lecture also touches on the concept of kinetic energy in relation to the motion of rigid bodies, discussing how it can be broken down into translational and rotational components. The professor emphasizes the usefulness of the Parallel Axis Theorem in solving real-world problems involving rotational motion.
🌟 Kinetic Energy of a Collection of Masses and Rigid Bodies
Professor Ramamurti Shankar discusses the concept of kinetic energy in a system of masses, differentiating between the kinetic energy associated with the center of mass motion and the kinetic energy relative to the center of mass. He explains how the total kinetic energy of a system can be expressed as the sum of these two components. The lecture then applies this concept to the case of a rigid body, highlighting that the only motion allowed for a co-moving observer with the center of mass is rotational. The professor further explains that in such a case, the kinetic energy can be expressed as the sum of the translational kinetic energy and the rotational kinetic energy, providing a deeper understanding of the dynamics of rigid bodies.
🚗 Rolling Without Slipping and Energy Calculations
The lecture explores the concept of rolling without slipping, a scenario where a rigid body, such as a tire, moves in such a way that there is no relative sliding at the point of contact. The professor explains how the linear velocity and angular velocity are related in this case, and how this relationship affects the kinetic energy of the system. He uses the example of a rolling tire to demonstrate how the total kinetic energy can be calculated by combining the translational and rotational energies. The lecture also touches on the conservation of energy, showing how the potential energy of the system is converted into kinetic energy as the object rolls down a ramp.
💫 Application of Conservation of Energy in Rotational Motion
Professor Shankar applies the Law of Conservation of Energy to problems involving rotational motion, specifically focusing on a cylinder rolling without slipping down a ramp. He shows how the potential energy at the top of the ramp is converted into kinetic energy at the bottom, and how the moment of inertia affects the final velocity of the cylinder. The lecture emphasizes the importance of considering both the translational and rotational components of energy in such calculations. The professor also provides a method for determining the velocity of the cylinder at the bottom of the ramp, taking into account the moment of inertia and the height of the ramp.
🚀 Torque and Angular Acceleration in Rigid Body Motion
The lecture discusses the application of torque and its relationship with angular acceleration in the context of rigid body motion. Professor Shankar uses the example of a disk, or merry-go-round, to demonstrate how the application of torque, either through manual effort or through the use of rockets, results in angular acceleration. He explains how the moment of inertia of the disk affects the angular acceleration and provides a formula for calculating the acceleration. The lecture also touches on the concept of rolling without slipping and how it relates to the conservation of angular momentum.
🔧 Pulley Systems and Conservation of Angular Momentum
The professor explores pulley systems as an example of combined translational and rotational motion, focusing on the conservation of angular momentum. He explains how the acceleration of a mass attached to a pulley is related to the angular acceleration of the pulley itself. The lecture provides a step-by-step approach to solving for the acceleration of the mass and the tangential acceleration at the point of contact with the pulley. The professor also emphasizes the importance of checking solutions using the Law of Conservation of Energy and the principles of torque and angular momentum.
🎇 Angular Momentum Conservation in Isolated Systems
The lecture discusses the conservation of angular momentum in systems where the net external torque is zero. Professor Shankar uses the example of a spinning disk and a second disk at rest to illustrate how the angular momentum is conserved when the two disks combine. He also explores the scenario where a mass with linear momentum is added to a spinning disk, emphasizing that the angular momentum of the system must remain constant. The lecture highlights the importance of understanding the relationship between linear and angular momentum in solving complex problems involving both types of motion.
🧘 Changes in Rotational Speed and Energy
The professor discusses the change in rotational speed and energy in the context of an ice skater spinning with dumbbells. He explains how the skater's action of bringing the weights closer to the body results in an increase in angular velocity and kinetic energy, despite the angular momentum remaining constant. The lecture explores the concept of work done in changing the rotational speed and how it is derived from the skater's own effort. The professor emphasizes the importance of understanding the distinction between linear and rotational motion and the energy transformations involved in such scenarios.
Mindmap
Keywords
💡Rotational Inertia
💡Angular Velocity
💡Torque
💡Angular Momentum
💡Parallel Axis Theorem
💡Kinetic Energy
💡Conservation of Energy
💡Angular Acceleration
💡Work
💡Center of Mass
Highlights
Essential concepts of rotational dynamics are summarized, including the moment of inertia, angular velocity, and torque.
The moment of inertia depends on the point of rotation and can be calculated using the Parallel Axis Theorem.
Angular momentum is analogous to linear momentum, with torque being the rotational equivalent of force.
The Parallel Axis Theorem is derived and explained, showing its utility in simplifying calculations for moments of inertia.
The kinetic energy of a system can be expressed in terms of both translational and rotational contributions.
The concept of rolling without slipping is introduced, with implications for the kinetic energy of rotating objects.
The law of conservation of energy is applied to test the validity of solutions in rotational dynamics.
A disk rolling down a ramp provides an example of how rotational inertia affects the motion of objects.
The connection between linear and angular velocities is discussed, particularly in the context of rolling without slipping.
The impact of external torques on the angular momentum of a system is explored, emphasizing the conservation of angular momentum in the absence of external torques.
The concept of angular momentum is applied to analyze the behavior of a system when a mass is added or removed from a rotating body.
The effect of changing the moment of inertia on the angular velocity and kinetic energy of a rotating system is discussed, with examples from ice skating.
The role of work done by the system itself, such as a skater pulling in their arms, in changing the rotational kinetic energy is highlighted.
The importance of working with symbols in physics problems is emphasized for testing solutions and understanding underlying principles.
The concept of angular momentum conservation is applied to a variety of scenarios, including collisions and the addition of mass to a rotating body.
The relationship between the distribution of mass and the moment of inertia is discussed, with implications for the rotational dynamics of objects.
The lecture concludes with a discussion on the practical applications of rotational dynamics, emphasizing the importance of understanding both translational and rotational aspects of motion.
Transcripts
Browse More Related Video

9. Rotations, Part I: Dynamics of Rigid Bodies
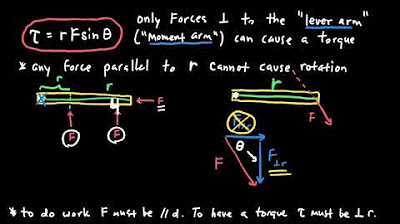
AP Physics 1 Torque and Rotational Motion Review

Rotational Energy | Physics with Professor Matt Anderson | M12-17
Rotation Free Response Paragraph Questions (AP Physics 1)
AP Physics 1: Rotational Dynamics Review

AP Physics Workbook 7.I Collisions "Old and Lack Full Explanation"
5.0 / 5 (0 votes)
Thanks for rating: