Calculus 1: Tangent and Normal Lines Example Problems
TLDRThis educational video script focuses on the application of derivatives to find equations of tangent and normal lines. It emphasizes using the slope-point form for these equations, which requires identifying a slope and a point. The script demonstrates how to find the slope by taking the derivative of a function at a specific point, exemplified with the function f(x) = x^3 - 1. It then explains the process for finding the normal line, which involves using the negative reciprocal of the tangent line's slope. The video also covers additional examples, including using the natural logarithm function and the cosine function, to illustrate the concepts further. The speaker encourages viewers to visit their website for over 400 solved calculus problems and step-by-step explanations.
Takeaways
- 📚 The video discusses the application of derivatives in finding equations of tangent and normal lines.
- 📈 The slope-point form is essential for finding the equation of any line, requiring both a slope and a point.
- 📍 The point (1,0) is identified as the key point for constructing the equations of the tangent and normal lines.
- 🔍 To find the y-value at the point x=1, the script involves plugging x=1 into the given function, resulting in y=0.
- 🧭 The slope of the tangent line is determined by the derivative of the function at the point of tangency, which is x=1 in this case.
- 📉 The derivative of the function \( f(x) = x^3 - 1 \) is \( f'(x) = 3x^2 - 1 \), and \( f'(1) = 2 \), giving the slope of the tangent line.
- 📐 The equation of the tangent line is derived using the slope \( m = 2 \) and the point (1,0).
- ⚖️ The normal line's slope is the negative reciprocal of the tangent line's slope, which is \( -\frac{1}{2} \) in this example.
- 🔄 For the normal line, the script uses the point (1,0) and the negative reciprocal slope to form the equation.
- 🌐 The video also covers an example involving the natural logarithm function and its derivative to find the tangent and normal lines.
- 📝 The video concludes with an invitation to visit the speaker's website for more calculus examples and questions.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the application of derivatives to find equations of tangent and normal lines.
What is the general form used to find the equation of a line?
-The general form used to find the equation of a line is the slope-point form, which requires a slope and a point.
How is the point used in the slope-point form determined in the context of the script?
-In the context of the script, the point is determined by plugging in the given x-value into the function to find the corresponding y-value.
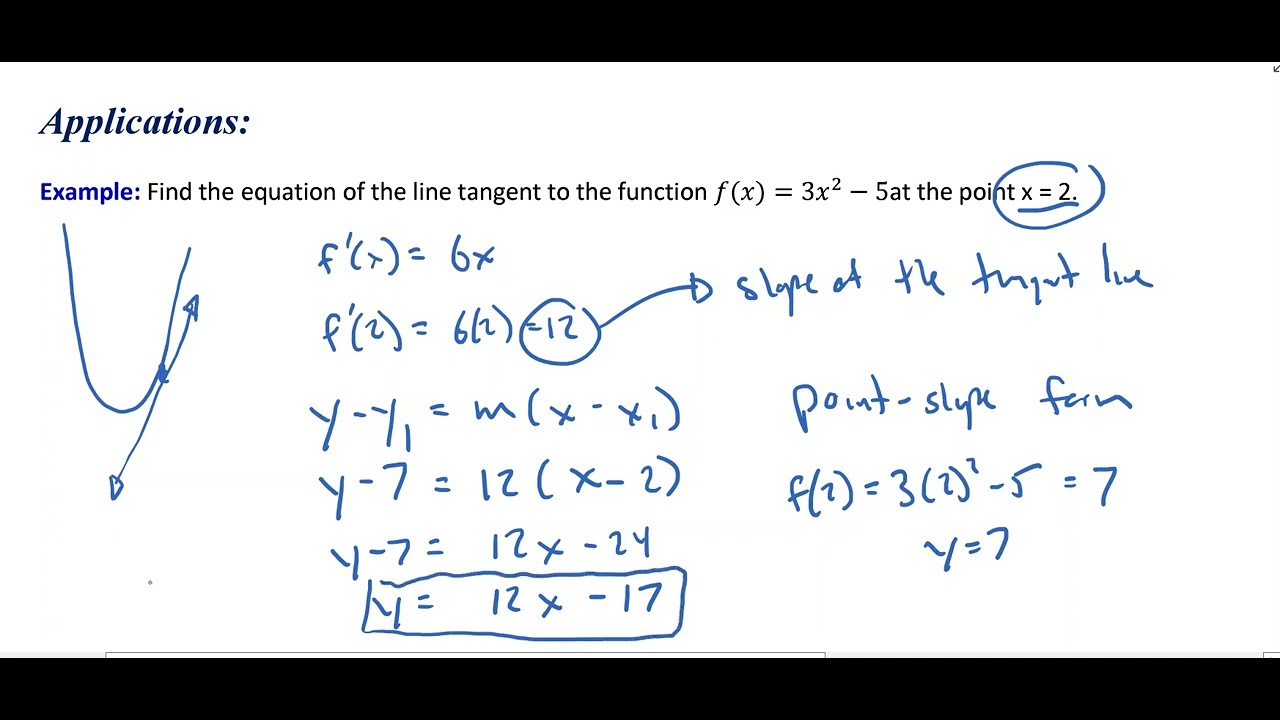
What is the role of calculus in finding the equation of a tangent line?
-In the script, calculus is used to find the slope of the tangent line by taking the derivative of the function at the given point.
What is the derivative of the function f(x) = x^3 - 1 at x = 1?
-The derivative of the function f(x) = x^3 - 1 is f'(x) = 3x^2. At x = 1, f'(1) = 3(1)^2 - 1 = 2.
How is the slope of the tangent line related to the derivative of the function?
-The slope of the tangent line is equal to the value of the derivative of the function at the given point.
What is the equation of the tangent line to the graph of f(x) = x^3 - 1 at x = 1?
-The equation of the tangent line at x = 1 is y - 0 = 2(x - 1), which simplifies to y = 2x - 2.
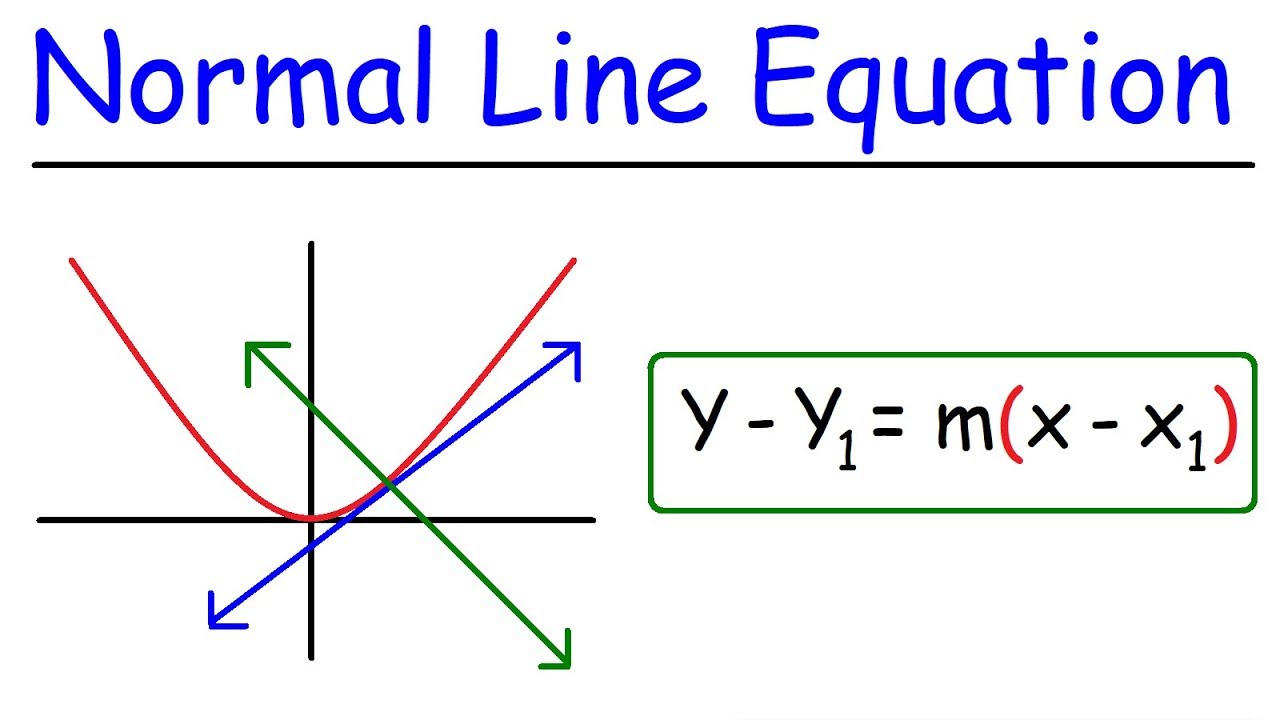
How is the slope of the normal line related to the slope of the tangent line?
-The slope of the normal line is the negative reciprocal of the slope of the tangent line.
What is the process to find the equation of the normal line?
-To find the equation of the normal line, one must first find the slope of the tangent line using the derivative, then use the negative reciprocal of that slope and the given point to write the equation in slope-point form.
What is the equation of the normal line to the graph of f(x) = x^3 - 1 at x = 1?
-The equation of the normal line at x = 1 is y - 0 = -1/2(x - 1), which simplifies to y = -1/2x + 1/2.
What additional resources does the script mention for further learning?
-The script mentions a website with free access to over 400 calculus questions solved step-by-step for further learning.
Outlines
📚 Introduction to Derivative Applications
This paragraph introduces the concept of using derivatives to find equations of tangent and normal lines. The speaker emphasizes the importance of the slope-point form for writing the equation of a line, which requires both a slope and a point. The example provided uses the point (1, 0) and demonstrates how to find the y-value by substituting x=1 into the given function, resulting in y=0. The speaker then explains that the slope of the tangent line is the derivative of the function at the given point, and for the normal line, the slope is the negative reciprocal of the tangent line's slope.
🔍 Deriving the Equation of a Tangent Line
The speaker elaborates on finding the equation of a tangent line using the derivative of a function. In the example, the derivative of the function f(x) = x^3 - 1 is calculated at x=1, resulting in a slope (m) of 2. The equation of the tangent line is then formulated using the slope-point form as y - 0 = 2(x - 1). The speaker clarifies that this form is preferred over the slope-intercept form in calculus problems involving derivatives.
📐 Understanding the Equation of a Normal Line
The speaker explains the process of finding the equation of a normal line, which is perpendicular to the tangent line. The normal line's slope is the negative reciprocal of the tangent line's slope. Using the same example, the speaker shows how to set up the equation for the normal line and solve for it, resulting in y = -1/2(x - 1).
📘 Applying Derivatives to Find Tangent and Normal Lines for a New Function
The speaker applies the concepts discussed to a new function, ln(x), and finds the equations of the tangent and normal lines at a specific point. The derivative of ln(x) is calculated, and the slope for the tangent line at x=1 is determined to be 1. The equations for both the tangent line (y = x - 1) and the normal line (y = -x - 1) are derived and presented.
📚 Deriving Tangent and Normal Lines for Trigonometric Functions
The final example involves a trigonometric function, cos(x), and the speaker demonstrates how to find the tangent and normal lines at x = π/4. The derivative of cos(x) is calculated, and the slope for the tangent line is found to be root 2/2. The equations for the tangent line (y + root 2 = root 2 * (x - π/4)) and the normal line (y - root 2 = -1/root 2 * (x - π/4)) are derived and simplified.
📝 Conclusion and Additional Resources
The speaker concludes the video by summarizing the process of using derivatives to find tangent and normal lines and encourages viewers to visit their website for more examples and practice problems. Over 400 calculus questions with step-by-step solutions are offered as a resource for further learning.
Mindmap
Keywords
💡Derivatives
💡Tangent Lines
💡Normal Lines
💡Slope-Point Form
💡Slope
💡Equation of a Line
💡Natural Logarithm
💡Derivative at a Point
💡Negative Reciprocal
💡Sine Function
Highlights
Introduction to the application of derivatives in finding equations of tangent and normal lines.
The importance of using the slope-point form for any line equation.
Finding the point on the line, which is the easiest part of the equation.
Calculating the y-value by plugging in the x-value into the function.
The general equation of a line passing through a given point.
Utilizing calculus to determine the slope of the tangent line.
The tangent line's slope is the derivative of the function at a specific point.
Deriving the function to find the slope of the tangent line.
Writing the equation of the tangent line without converting to slope-intercept form.
Understanding that the normal line is perpendicular to the tangent line.
Calculating the slope of the normal line as the negative reciprocal of the tangent line's slope.
Using the slope-point form to write the equation of the normal line.
Finding the derivative of a logarithmic function to determine the slope.
Writing the equation for a tangent line with a slope of 1.
Writing the equation for a normal line with a slope of negative 1.
Applying the process to trigonometric functions, specifically cosine.
Deriving the cosine function to find the slope for the tangent line.
Writing the final equations for the tangent and normal lines of a cosine function.
Providing additional resources for learning more about calculus problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: