Expected profit from lottery ticket | Probability and Statistics | Khan Academy
TLDRIn this lottery game, Ahmed picks two numbers from 0-9 and one letter from the alphabet, with the possibility of repetition. The ticket costs $5, and Ahmed's chosen ticket is 04R. The video explores the expected net profit (E[X]) of playing this game, considering the probabilities and outcomes of winning the grand prize ($10,405), a small prize ($100), or losing. The calculations reveal an unusual scenario where the expected net profit is positive at $2.81, suggesting it would be rational for a player to participate in this lottery, contrary to most games where the operator expects to profit.
Takeaways
- 🎰 Ahmed plays a lottery game involving choosing two numbers from 0 to 9 and one letter from the English alphabet.
- 🔢 He can pick the same number twice, and the game costs $5 to play.
- 🏆 Winning the grand prize requires matching both numbers and the letter, which pays out $10,405.
- 💰 Matching only the letter, but not the numbers, wins a small prize of $100.
- ❌ Any other outcome results in a loss with no prize.
- 📈 The expected value of the game is calculated by multiplying the probabilities of each outcome by the net profit from those outcomes.
- 🎯 The probability of winning the grand prize is 1 in 2600.
- 📚 To find the probability of the small prize, subtract the probability of winning the grand prize from the probability of getting the letter right (1 in 26).
- 🤔 The probability of losing (getting neither prize) is calculated by subtracting the probabilities of winning from 1.
- 🧮 The expected net profit from playing the game with ticket 04R is calculated to be approximately $2.81.
- 💡 This lottery game is unusual as it has a positive expected net profit for the player, which is not common in most lottery games.
Q & A
What is the lottery game that Ahmed is playing?
-Ahmed is playing a lottery game where he picks two numbers from zero to nine and one letter from the 26-letter English alphabet. He can choose the same number twice. If his ticket matches the two numbers and one letter in order, he wins the grand prize.
What are the prizes for matching different parts of the ticket in the lottery game?
-If Ahmed matches the two numbers and one letter, he wins the grand prize of $10,405. If only the letter matches, he wins a small prize of $100. Any other outcome results in a loss with no prize.
What is the cost for Ahmed to play the lottery game?
-The cost for Ahmed to play the lottery game is $5.
What is the ticket Ahmed has chosen in the lottery game?
-Ahmed has chosen the ticket 04R for the lottery game.
What is the random variable X defined as in the context of this lottery game?
-The random variable X is defined as the net profit from playing the lottery game with the ticket 04R.
What is the expected value of the net profit from playing the ticket 04R?
-The expected value of the net profit from playing the ticket 04R is calculated by multiplying the probabilities of each outcome by their respective net profits and summing them up.
What is the net profit for Ahmed if he wins the grand prize?
-The net profit for Ahmed if he wins the grand prize is $10,405 minus the $5 he paid to play, which equals $10,400.
How is the probability of winning the small prize calculated?
-The probability of winning the small prize is calculated by taking the probability of getting the letter right (1 in 26) and subtracting the probability of winning the grand prize (1 in 2600).
What is the net profit for Ahmed if he wins the small prize?
-The net profit for Ahmed if he wins the small prize is $100 minus the $5 he paid to play, which equals $95.
What is the probability of Ahmed losing the game and receiving nothing?
-The probability of Ahmed losing the game and receiving nothing is calculated by subtracting the probabilities of winning the small and grand prizes from 1, which simplifies to 25/26.
What is the expected net profit of playing the lottery game according to the calculations?
-The expected net profit of playing the lottery game, according to the calculations, is approximately $2.81 when rounded to the nearest penny.
Why is the expected net profit for Ahmed positive in this lottery game?
-The expected net profit for Ahmed is positive because the potential gains from winning the grand and small prizes, when weighted by their probabilities, exceed the cost of playing the game ($5).
Outlines
🎰 Lottery Game Mechanics and Expected Net Profit Calculation
The video script introduces a lottery game where a participant, Ahmed, picks two numbers from 0 to 9 and one letter from the English alphabet. The ticket costs $5, and Ahmed has chosen '04R'. The script defines a random variable X representing the net profit from playing. The expected value of X is calculated by multiplying the probabilities of winning the grand prize ($10,405) or the small prize ($100) by their respective net profits after the ticket cost. The grand prize probability is calculated as 1 in 2600, considering the independent chances of picking the correct number and letter. The small prize probability is the chance of getting the letter right but not both numbers, which is 1 in 26 minus the probability of winning the grand prize. The expected net profit is then calculated by adding the products of probabilities and net profits for both winning scenarios and the loss scenario, resulting in a positive expected net profit of $2.81, suggesting that it might be rational to play this particular lottery game.
📊 Probability Calculations and the Unusual Positive Expected Value
This paragraph delves into the detailed probability calculations for the lottery game described in the previous paragraph. It explains how to determine the probability of winning the small prize by subtracting the probability of winning the grand prize from the probability of getting the letter right. The script then calculates the probability of losing, which is everything else not covered by the winning scenarios, by using the formula 1 minus the sum of the probabilities of winning the small and grand prizes. The expected net profit is computed by adding up the products of the probabilities of each outcome and their respective net profits. Interestingly, the expected net profit for playing the game is positive at $2.81, which is unusual for lottery games, as typically the operators have a positive expected profit and players have a net loss. This positive expected value suggests that, from a mathematical perspective, it could be a good decision to play this game.
Mindmap
Keywords
💡Lottery Game
💡Random Variable
💡Expected Value
💡Net Profit
💡Grand Prize
💡Small Prize
💡Probability
💡Independent Events
💡Cost to Play
💡Outcomes
💡Positive Expected Net Profit
Highlights
Ahmed plays a lottery game where he picks two numbers from 0-9 and one letter from the English alphabet.
Ahmed can choose the same number twice and must match two numbers and one letter to win the grand prize.
The grand prize is $10,405, and the small prize for matching only the letter is $100.
The cost to play the game is $5, and any other outcome results in a loss.
Ahmed's chosen ticket is 04R.
The random variable X represents the net profit from playing the lottery game.
Expected value is calculated as the probability of each outcome times the net profit.
The probability of winning the grand prize is one in 2600.
The probability of winning the small prize is calculated by subtracting the grand prize probability from the chance of getting the letter right.
The probability of losing, or not winning anything, is one minus the sum of probabilities of the small and grand prizes.
The net profit for winning the grand prize is $10,400 ($10,405 - $5).
The net profit for winning the small prize is $95 ($100 - $5).
The net profit for losing is -$5, as the player pays $5 and receives nothing.
The expected net profit from playing the lottery game is calculated with the probabilities and net profits.
The expected net profit from playing ticket 04R is $2.81.
This lottery game is unusual as it offers a positive expected net profit for the player.
Typically, lotteries are designed so that the organizers have a positive expected profit and players have a loss.
Playing this game makes rational sense for Ahmed as he expects a net profit.
Transcripts
Browse More Related Video
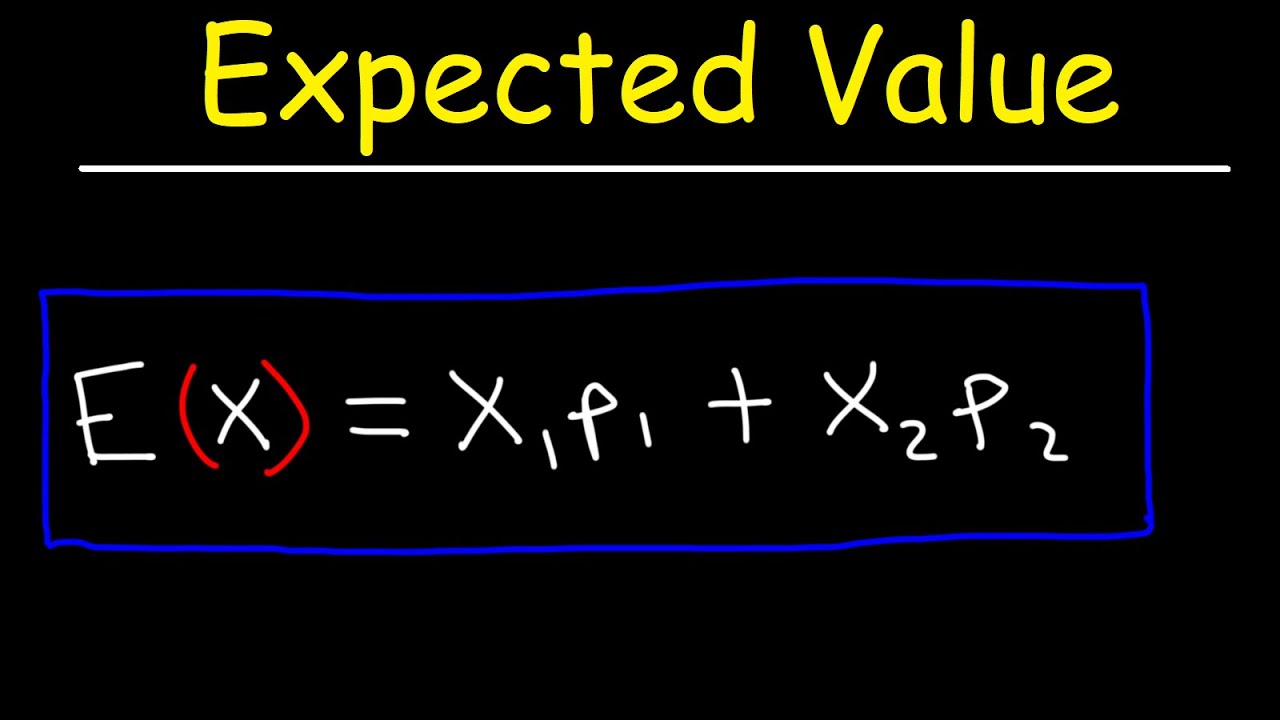
How To Calculate Expected Value

Expected value while fishing | Probability and Statistics | Khan Academy

4.4.5 Counting - Permutations and Combinations and the Combinations Rule
Math 11 - Section 3.5

Business Calculus - More Optimization
Section 2.7 - Applications of Derivatives to Business and Economics
5.0 / 5 (0 votes)
Thanks for rating: