Expected value while fishing | Probability and Statistics | Khan Academy

Khan Academy
10 Apr 201408:24
EducationalLearning
32 Likes 10 Comments

TLDRIn this video script, the host discusses two betting scenarios involving fishing for trout and sunfish in a pond. Bet one involves Jeremy catching three sunfish consecutively, with varying payouts based on the outcome. The expected value of this bet is calculated to be a $5 profit. Bet two offers a 50/50 chance of winning $50 or losing $25, based on catching at least two sunfish in three attempts. The expected value here is $12.50. The host concludes that while both bets are favorable, bet two has a higher expected payoff and should be chosen consistently.

Takeaways
  • 🎣 The scenario involves fishing in a pond with 10 trout and 10 sunfish, where fish are released after being caught.
  • 🤔 Jeremy offers two betting options based on the type of fish caught and the number of sunfish caught in a sequence.
  • 💰 Bet one involves a potential loss of $100 if Jeremy catches three sunfish in a row, or a gain of $20 otherwise.
  • 📊 The expected value is calculated by considering the probabilities of the outcomes and their respective payoffs.
  • 📚 The probability of catching three sunfish in a row is calculated as (1/2) * (1/2) * (1/2) = 1/8.
  • 🧮 The expected value for bet one is calculated to be a positive $5, indicating a favorable outcome for the player.
  • 🐟 Bet two offers a payoff of $50 for catching at least two sunfish in three tries, or a loss of $25 otherwise.
  • 🎲 The probability of catching at least two sunfish in three tries is determined to be 4 out of 8 possible outcomes, or 1/2.
  • 🔢 The expected value for bet two is calculated as $12.50, which is higher than that of bet one.
  • 🤝 Jeremy is willing to take both bets multiple times, and the player is advised to take bet two for a higher expected payoff.
  • 📈 The script concludes that bet two is the better option, suggesting that the player should choose it over bet one for maximizing expected value.
Q & A

  • What are the two types of fish in the pond?

    -The two types of fish in the pond are trout and sunfish.

  • How many trout and sunfish are there in the pond?

    -There are ten trout and ten sunfish in the pond, making a total of twenty fish.

  • What are the terms of Bet number one?

    -In Bet number one, if Jeremy catches three sunfish in a row, you will pay him 100 dollars. Otherwise, he will pay you 20 dollars.

  • What is the expected value of Bet number one?

    -The expected value of Bet number one is a positive five dollars.

  • How is the probability of catching three sunfish in a row calculated?

    -The probability of catching three sunfish in a row is calculated by multiplying the probability of catching a sunfish each time (1/2 * 1/2 * 1/2), which equals 1/8 or one-eighth.

  • What are the terms of Bet number two?

    -In Bet number two, if you catch at least two sunfish out of the next three fish you catch, Jeremy will pay you 50 dollars. Otherwise, you will pay him 25 dollars.

  • What is the expected value of Bet number two?

    -The expected value of Bet number two is 12.50 dollars.

  • How many ways can you catch at least two sunfish in three attempts?

    -There are four ways to catch at least two sunfish out of three attempts: sunfish, sunfish, sunfish; sunfish, sunfish, trout; sunfish, trout, sunfish; and trout, sunfish, sunfish.

  • What is the probability of catching at least two sunfish in three attempts?

    -The probability of catching at least two sunfish in three attempts is one half, or four out of eight possible outcomes.

  • Which bet has a higher expected payoff according to the script?

    -Bet number two has a higher expected payoff compared to Bet number one.

  • What is the recommendation if you want to maximize your expected value?

    -The recommendation is to take Bet number two all of the time, as it has a higher expected value.

Outlines
00:00
🎣 Calculating the Expected Value of a Fishing Bet

In this paragraph, the script discusses a scenario where Jeremy offers two betting options on a fishing trip. The first bet involves Jeremy catching three sunfish in a row, with a payoff of $100 if he loses and $20 if he wins. The expected value is calculated by considering the probability of catching three sunfish consecutively, which is 1/8, given the pond has ten trout and ten sunfish and the fish are released after each catch. The expected profit from this bet is found to be $5. The second bet is not fully explained in this paragraph, but it involves catching at least two sunfish in the next three catches.

05:01
📊 Analyzing the Probability and Expected Value of a Second Fishing Bet

This paragraph continues the discussion on the fishing bets, focusing on the second bet. It outlines the possible outcomes of catching sunfish and trout in any combination over three attempts, resulting in eight equally likely scenarios. The probability of catching at least two sunfish in three tries is calculated as 4 out of 8, or 1/2. The expected value of this bet is then determined by weighing the potential winnings of $50 against the loss of $25, resulting in an expected profit of $12.50. The script concludes by suggesting that both bets are favorable, but the second bet has a higher expected payoff, and thus should be chosen.

Mindmap
Keywords
💡Expected Value
Expected value is a fundamental concept in probability theory and statistics, referring to the average amount one can expect to win or lose per bet when the same uncertain situation is repeated indefinitely. In the video's theme, it is used to calculate the potential profit or loss from the two betting scenarios presented. The script calculates the expected value for both bet one and bet two, showing that bet two has a higher expected payoff.
💡Betting
Betting is the act of wagering money or something of value on an event with an uncertain outcome, with the primary intent of winning additional money or goods. In the context of the video, two friends are engaged in a fishing activity and are considering two different betting scenarios based on the types of fish they catch, illustrating the concept of expected value in a real-world scenario.
💡Probability
Probability is a measure of the likelihood that a particular event will occur, expressed as a number between 0 and 1, where 0 indicates impossibility and 1 indicates certainty. The video uses probability to determine the odds of catching sunfish or trout in the pond, which is essential for calculating the expected value of the bets.
💡Sunfish
Sunfish is a common name for a group of freshwater fish species that are often found in ponds and lakes. In the video, sunfish are one of the two types of fish in the pond, and catching them is part of the conditions for winning the bets. The probability of catching sunfish is crucial for determining the expected value of the bets.
💡Trout
Trout is a type of fish that is also found in freshwater environments and is the second type of fish in the pond mentioned in the video. The script discusses the probability of catching trout as part of the betting conditions, which is essential for calculating the expected value of the bets.
💡Random Variable
A random variable is a variable whose possible values are numerical outcomes of a random phenomenon. In the video, the random variables are defined as the potential profits or losses (x and y) from the two bets. These variables are used to calculate the expected values for each bet.
💡Profit
Profit in the context of the video refers to the monetary gain or loss resulting from the bets. The script discusses the expected profit from each bet, which is calculated by considering the probabilities of different outcomes and the amounts won or lost in each scenario.
💡Payoff
Payoff in this context refers to the amount of money won or lost in a bet. The video script calculates the expected payoff for each bet, which is the potential profit or loss one can expect to receive based on the probabilities of the outcomes.
💡Risk
Risk is the possibility of losing money or something of value. In the video, risk is inherent in the betting scenarios, as there is a chance of losing money if the conditions of the bet are not met. The expected value calculations help to assess the level of risk associated with each bet.
💡Calculation
Calculation in the video refers to the mathematical process of determining the expected value and payoffs for the bets. The script provides a step-by-step calculation for both bets, showing how to use probability and the conditions of the bets to arrive at the expected values.
💡Decision Making
Decision making is the process of selecting a course of action from among various alternatives. In the video, the concept of decision making is applied to choosing which bet to take based on the calculated expected values. The higher expected value of bet two suggests that it is the better decision.
Highlights

Jeremy offers two different betting scenarios involving catching fish.

Bet one involves a potential payout of $100 if Jeremy catches three sunfish consecutively.

In bet one, the alternative scenario is paying Jeremy $20 if the condition is not met.

Bet two proposes a $50 reward for catching at least two sunfish in three attempts.

The downside of bet two is paying $25 if the condition of catching at least two sunfish is not fulfilled.

The expected value of bet one is calculated using the probability of catching three sunfish consecutively.

The probability of catching a sunfish is determined to be 1/2, considering the pond's fish composition.

The consecutive probability of catching three sunfish is calculated as (1/2)^3.

The expected value calculation for bet one results in a potential profit of $5.

Bet two's expected value involves considering all possible outcomes of catching three fish.

There are eight equally likely outcomes when catching three fish.

Four out of the eight outcomes result in catching at least two sunfish.

The probability of winning bet two is one half, leading to an expected value calculation.

The expected value of bet two is determined to be $12.50.

A comparison between the two bets shows bet two has a higher expected payoff.

The recommendation is to take bet two for maximizing expected value.

Jeremy is willing to take both bets 50 times, suggesting a strategy for maximizing profit.

Transcripts

Browse More Related Video

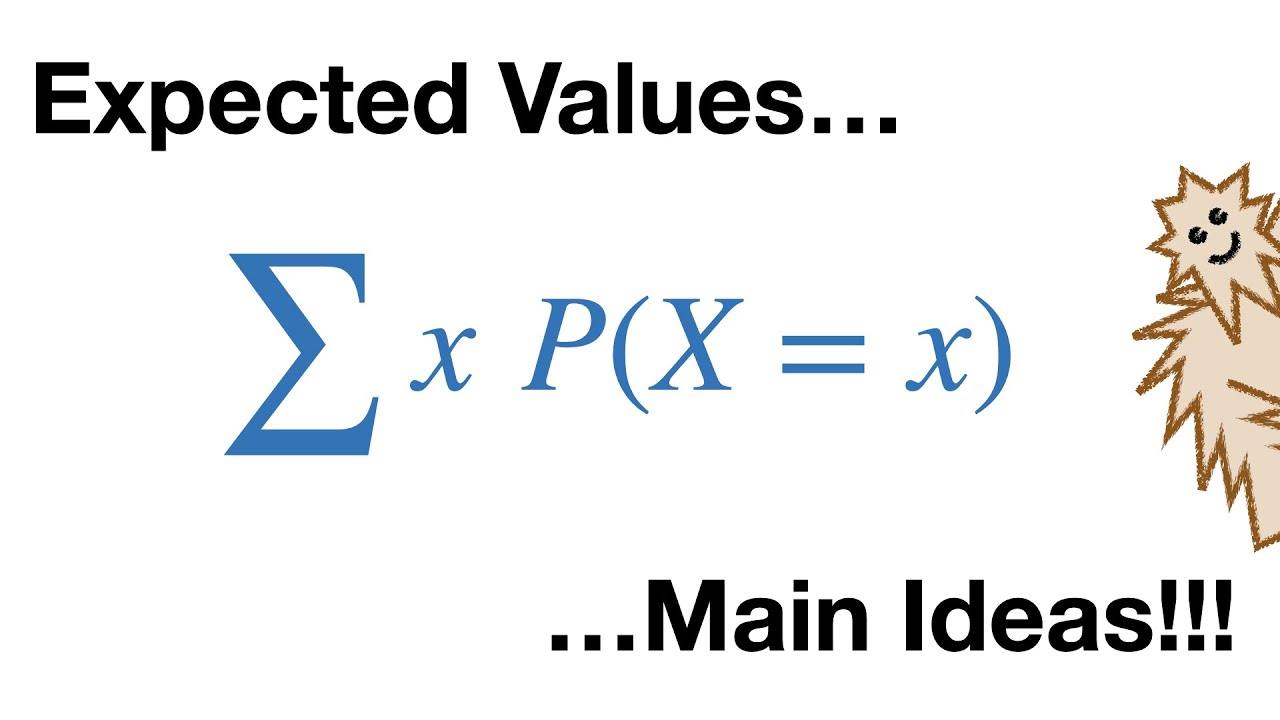
Expected Values, Main Ideas!!!

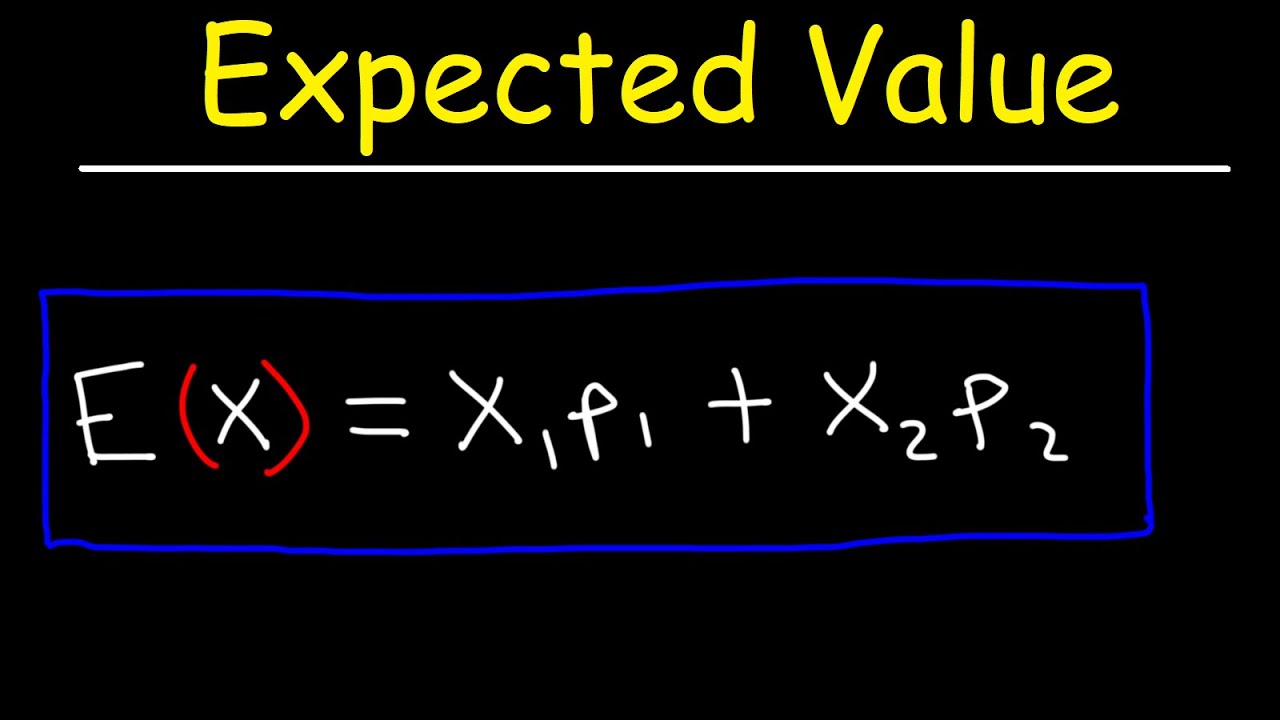
How To Calculate Expected Value

Expected profit from lottery ticket | Probability and Statistics | Khan Academy

Physics 15 Torque (6 of 27) What is a Couple?

Bernoulli Distribution

Win More Bets: Top Sports Betting Strategies: Losing Streaks, Line Shopping & NBA Post-All-Star Tips

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
ProbabilityExpected ValueBettingFishingSunfishTroutGamblingMathematicsDecision MakingRisk Analysis