Lesson 20 - Alternating Series Test (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial segment introduces the concept of alternating series in advanced calculus, a type of series where terms alternate between positive and negative values. The presenter explains the general form of an alternating series and emphasizes the importance of the terms approaching zero for the series to potentially converge. The core of the lesson is the Alternating Series Test, which is a method to determine the convergence of such series. The explanation is aimed at making the topic accessible and straightforward, highlighting the significance of the sign alternation in the series' behavior.

Takeaways
  • 📚 The video discusses advanced calculus, specifically focusing on a type of series known as alternating series.
  • 🔍 The goal is to learn how to test whether an alternating series converges or not, which is considered one of the simpler tests in series convergence.
  • 🔄 An alternating series is characterized by terms that alternate in sign, switching between positive and negative with each term.
  • 📝 The general form of an alternating series is represented as \( \sum_{n=1}^{\infty} (-1)^{n-1} \frac{1}{n} \), where the \( (-1)^{n-1} \) ensures the sign alternation.
  • 👉 The script provides an example of an alternating series: \( 1 - \frac{1}{2} + \frac{1}{3} - \frac{1}{4} + \frac{1}{5} - \ldots \).
  • 🌟 The importance of the terms approaching zero for a series to potentially converge is highlighted, which is a fundamental concept in series analysis.
  • 📉 The script introduces the 'alternating series test' as a key method to apply in this section for determining convergence.
  • 📌 The alternating series test is presented as the core content of the section, emphasizing its significance in the study of alternating series.
  • 📚 The video is educational, aiming to teach viewers about the properties and tests for alternating series within the broader context of calculus.
  • 🎯 The alternating series test is positioned as a practical tool for students to apply when analyzing the convergence of alternating series.
Q & A

  • What is an alternating series?

    -An alternating series is a series where the terms alternate in sign, meaning they switch between positive and negative values with each term. It can be represented as a sum where each term is of the form (-1)^(n-1)/n, where n is the term number.

  • What is the general form of an alternating series?

    -The general form of an alternating series is given by the sum from n=1 to infinity of (-1)^(n-1) * a_n, where a_n represents the nth term of the series.

  • What does the alternating series test involve?

    -The alternating series test involves checking if the limit of the absolute value of the terms of the series as n approaches infinity is zero. If this condition is met, the series may converge.

  • What is the significance of the term (-1)^(n-1) in the alternating series?

    -The term (-1)^(n-1) is used to alternate the sign of each term in the series. When n is odd, the term is positive, and when n is even, the term is negative, thus ensuring the alternating pattern.

  • What does it mean for a series to converge?

    -A series converges if the sum of its terms approaches a finite value as more terms are added. In other words, the series does not diverge to infinity.

  • What is a necessary condition for a series to have the potential to converge?

    -A necessary condition for a series to have the potential to converge is that the terms of the series approach zero as n approaches infinity.

  • Is the limit of the terms going to zero a sufficient condition for convergence?

    -No, the limit of the terms going to zero is a necessary but not sufficient condition for convergence. The series may still diverge even if the terms approach zero.

  • How does the alternating series test differ from other convergence tests?

    -The alternating series test specifically applies to series with alternating signs and involves checking the limit of the absolute value of the terms. It is a special case of the more general convergence tests.

  • What is the role of the 'a_n' in the alternating series test?

    -In the alternating series test, 'a_n' represents the nth term of the series without the alternating sign. The test checks if the limit of 'a_n' as n approaches infinity is zero.

  • Can you provide an example of an alternating series?

    -An example of an alternating series is the sum from n=1 to infinity of (-1)^(n-1) * (1/n), which alternates between positive and negative terms and includes terms like 1 - 1/2 + 1/3 - 1/4 + ...

Outlines
00:00
📚 Introduction to Alternating Series

This paragraph introduces the concept of alternating series within the context of advanced calculus. It explains that an alternating series is characterized by terms that switch between positive and negative signs, providing the example of a series with terms like 1/2, -3/4, 5/8, etc. The general form of an alternating series is given as the sum from n=1 to infinity of (-1)^(n-1)/n, which ensures the sign alternation. The importance of the terms approaching zero for the series to potentially converge is also highlighted, setting the stage for the Alternating Series Test to be discussed in subsequent paragraphs.

Mindmap
Keywords
💡Alternating Series
An alternating series is a sequence of terms where the signs of the terms alternate between positive and negative. This concept is central to the video's theme as it is the type of series being discussed. In the script, the series is exemplified by terms like 'positive one half negative three-fourths positive five eighths negative,' which clearly shows the pattern of sign alternation.
💡Convergence
Convergence in the context of series refers to the property where the sum of an infinite sequence of terms approaches a finite value. The video aims to explore tests to determine if an alternating series converges. The script mentions that 'if we find that the limit of the elements of the sequence... goes to zero, then the series may or may not converge,' indicating the importance of convergence in analyzing series.
💡Limit
The limit is a fundamental concept in calculus that describes the value that a function or sequence approaches as the input approaches some value. In the video, the limit is used to test for the convergence of an alternating series, as stated: 'if I found then the series may or may not converge okay all it's saying is that in order for the series to have a hope and a prayer of converging the elements the things that you're adding together must approach the zero.'
💡Test
In the context of the video, a test refers to a method or set of criteria used to determine the convergence of an alternating series. The script introduces the 'alternating series test,' which is a specific method to apply in this section for analyzing series, emphasizing its importance as 'the bread and butter of the section.'
💡Signs Alternate
The term 'signs alternate' is used to describe the pattern in an alternating series where the terms switch between positive and negative values. The script illustrates this with the phrase 'positive, negative, positive, negative,' which is a direct reference to how the signs change in an alternating series.
💡General Form
The general form of an alternating series is a mathematical representation that captures the alternating pattern of signs. The script provides an example of this form: 'and is equal to 1 to infinity of negative 1 to the N, minus 1 over N,' which shows how the signs alternate and the terms are structured in a series.
💡Terms
In the context of series, 'terms' refer to the individual elements being summed. The video discusses the importance of these terms approaching zero for the series to potentially converge, as mentioned in the script: 'if we find that this goes to zero then what if I found then the series may or may not converge.'
💡Infinite Series
An infinite series is a series that has an infinite number of terms. The video is focused on a specific type of infinite series, namely the alternating series. The script discusses the concept of adding up an infinite number of terms, as in 'if we find that the limit of the elements of the sequence that we're adding up and goes to infinity of a sub n.'
💡Sequence
A sequence in mathematics is an ordered list of numbers or terms. In the video, the sequence refers to the ordered list of terms in an alternating series. The script mentions the sequence in the context of taking the limit of its elements: 'if we find that the limit of the elements of the sequence that we're adding up.'
💡Universal Truth
The term 'universal truth' in the script refers to a fundamental principle or rule that applies across the board. In this case, it is used to emphasize the necessity for the terms of a series to approach zero for the series to possibly converge, as stated: 'that's just a universal truth they don't approach zero, you're just you're not going to make any headway.'
Highlights

Introduction to the study of alternating series in advanced calculus.

Exploring methods to test the convergence of alternating series.

Alternating series defined as series with terms that flip from positive to negative.

Explanation of the pattern in alternating series: positive, negative, positive, negative, etc.

General form of an alternating series presented with a mathematical formula.

The significance of the term (-1)^{n-1} in creating the alternating sign pattern.

The necessity for terms of a series to approach zero for potential convergence.

Introduction of the Alternating Series Test as a key concept in the section.

The Alternating Series Test is described as relatively straightforward.

The test involves checking if the sequence's terms approach zero as n goes to infinity.

Alternating series are a special case in the broader context of series convergence.

The mathematical representation of the Alternating Series Test is provided.

Emphasis on the importance of the sign alternation in the terms of the series.

The alternating series is characterized by its unique sign-flipping pattern.

The test is applied to determine the convergence of alternating series in this section.

The transcript concludes with a focus on the practical application of the Alternating Series Test.

Transcripts

Browse More Related Video

Calculus BC – 10.7 Alternating Series Test for Convergence

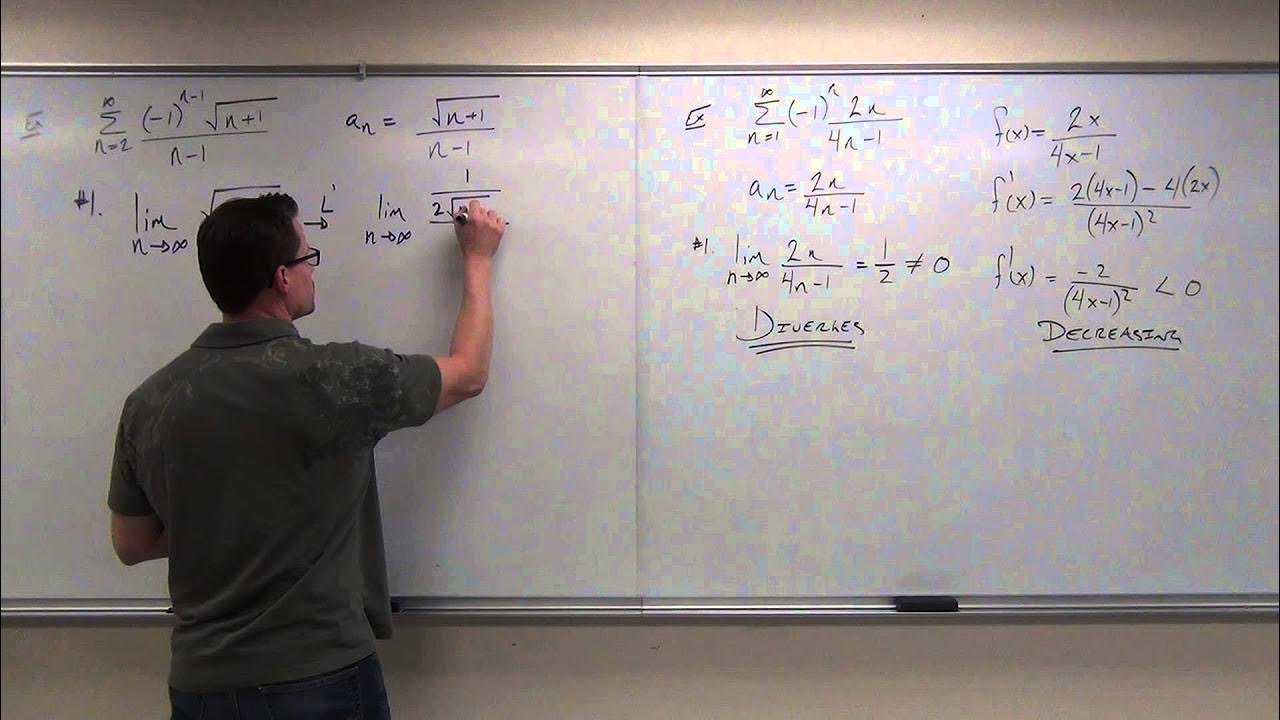
Calculus 2 Lecture 9.5: Showing Convergence With the Alternating Series Test, Finding Error of Sums

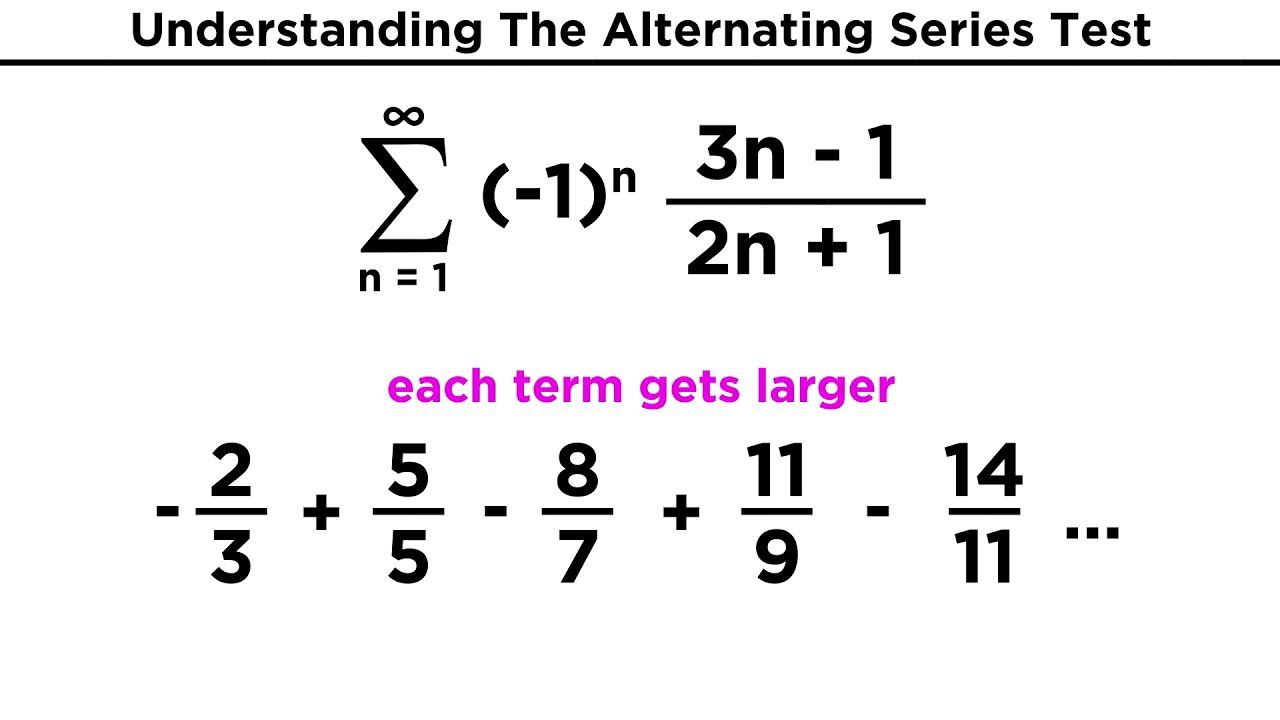
Alternating Series, Types of Convergence, and the Ratio Test

Alternating Series

Infinite Series Multiple Choice Practice for Calc BC (Part 4)

AP Calculus BC Lesson 10.7

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Alternating SeriesCalculus TutorialSeries ConvergenceMathematics EducationAdvanced MathConvergence TestSeries AnalysisEducational ContentMath ConceptsInfinite Series