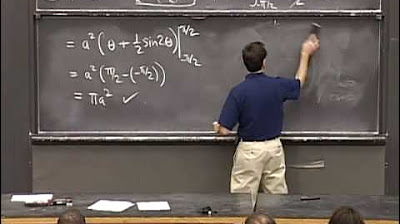Lesson 15 - Area And Length In Polar Coordinates (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial segment delves into the application of polar coordinates in advanced calculus, specifically focusing on the concepts of area and length calculations. The instructor clarifies that integration in polar coordinates is not as daunting as it sounds, being fundamentally similar to regular integration but with a shift in variables. The lesson begins with the area of a circle's sector, introducing the formula \( \frac{1}{2}r^2\theta \) without proof, emphasizing its importance. The script then transitions to setting up integration problems by defining limits in theta for a polar function, illustrating the process with a diagram of a polar graph in the coordinate system.

Takeaways
  • πŸ“š The section is about integrating in polar coordinates, which is a culmination of the topics discussed in previous sections on polar equations and coordinates.
  • πŸ“ Polar coordinates are useful for solving problems with circular symmetry due to their simplicity, especially when dealing with equations of circles.
  • πŸ” Integration in polar coordinates is not fundamentally different from regular integration; it involves integrating with respect to different variables, such as theta, instead of x.
  • πŸ“ˆ The script introduces the concept of calculating the area of a section of a circle, specifically a pie wedge, using polar coordinates.
  • 🌐 The area of a pie wedge in a circle is given by the formula \( \frac{1}{2} r^2 \theta \), where \( r \) is the radius and \( \theta \) is the angle.
  • πŸ“ The importance of understanding the setup of the problem is emphasized, as it is crucial for correctly applying integration in polar coordinates.
  • πŸ“‰ The script mentions that the area calculation for a pie wedge is provided without proof, indicating that the focus is on application rather than theoretical derivation.
  • πŸ“Œ The concept of 'theta' is introduced as the variable that describes the openness of the pie wedge and is crucial in determining the area.
  • πŸ“Š The script discusses the representation of a polar function in a coordinate system, showing how different values of theta result in different radii.
  • πŸ“ˆ The process of defining the limits of integration in polar coordinates is illustrated by selecting specific angles alpha and beta to define the region of interest.
  • πŸ“š The script is part of a tutorial series on advanced calculus, indicating that the content is aimed at individuals with a solid foundation in calculus.
Q & A

  • What is the main topic of this section of the advanced calculus tutorial?

    -The main topic of this section is area and length in polar coordinates, which is essentially integration in polar coordinates.

  • Why are polar coordinates useful for certain problems?

    -Polar coordinates are useful for problems involving circular symmetry because they simplify the equations and make it easier to solve such problems.

  • What is the significance of the equation of a circle in polar coordinates?

    -The equation of a circle in polar coordinates is simple, which makes it easier to work with and lends itself to having simple functions in polar coordinates.

  • What is the fundamental process of integration as described in the script?

    -The fundamental process of integration involves taking little slices of the function and adding them up, which is the same in polar coordinates as it is in rectangular coordinates.

  • What is the formula for the area of a pie wedge of a circle in polar coordinates?

    -The area of a pie wedge of a circle is given by the formula one-half r squared times theta, where r is the radius and theta is the angle of the wedge.

  • Why is it important to understand the area of a pie wedge in polar coordinates?

    -Understanding the area of a pie wedge is important because it forms the basis for integration in polar coordinates, allowing for the calculation of areas and volumes with circular symmetry.

  • What is the relationship between the angle theta and the area of the pie wedge in polar coordinates?

    -The area of the pie wedge is directly proportional to the angle theta, meaning that larger angles result in larger areas of the wedge.

  • How does the script suggest setting up a problem for integration in polar coordinates?

    -The script suggests that setting up a problem for integration in polar coordinates involves understanding the variables involved and the geometry of the problem, similar to regular integration but with different variables.

  • What is the role of the radius R in the area calculation of a pie wedge in polar coordinates?

    -The radius R is squared in the area calculation formula, indicating that the area of the pie wedge increases with the square of the radius.

  • How does the script introduce the concept of integration in polar coordinates without overwhelming the learner?

    -The script introduces the concept by relating it to the familiar process of integration in rectangular coordinates and emphasizing that the fundamental process remains the same, just with different variables.

Outlines
00:00
πŸ“š Introduction to Polar Coordinates and Integration

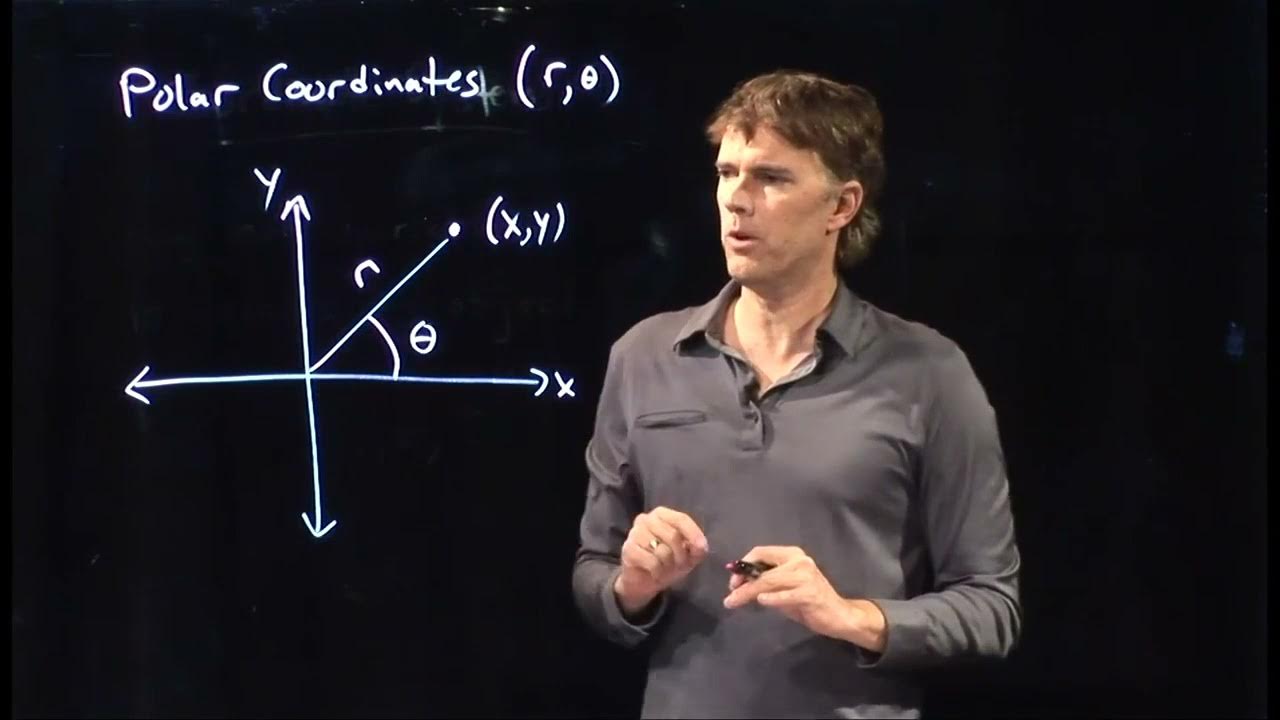
In this introductory paragraph, the speaker welcomes viewers to an advanced calculus tutorial focused on polar coordinates. The main theme revolves around the culmination of topics discussed in previous sections, such as understanding polar coordinates, converting between polar and rectangular forms, and the simplicity of polar functions, especially for problems with circular symmetry. The speaker emphasizes that while the topic of integration in polar coordinates might sound complex, it is fundamentally no different from regular integration, only with different variables. The goal is to integrate along different dimensions, such as theta, instead of the x-axis. The paragraph sets the stage for learning about the area of a section of a circle in polar coordinates, introducing the formula for the area of a pie wedge as a precursor to more complex integration problems.

Mindmap
Keywords
πŸ’‘Advanced Calculus
Advanced Calculus refers to the study of mathematical methods that go beyond the basic principles of differentiation and integration. In the context of this video, it is the overarching subject matter that the tutor is teaching. The script discusses the application of calculus to polar coordinates, which is a more advanced topic in the field of mathematics.
πŸ’‘Polar Equations
Polar equations are mathematical equations that describe the position of points in the plane using polar coordinates, which consist of a distance from a reference point (the pole) and an angle from a reference direction (the polar axis). The video script mentions polar equations as a culmination of previous discussions on polar coordinates, indicating their importance in solving problems with circular symmetry.
πŸ’‘Polar Coordinates
Polar Coordinates is a two-dimensional coordinate system where each point on a plane is determined by a radius and an angle from the origin. The script introduces the concept of polar coordinates early on, emphasizing their utility in simplifying problems that exhibit circular symmetry, such as the equation of a circle.
πŸ’‘Rectangular Coordinates
Rectangular Coordinates, also known as Cartesian Coordinates, is a coordinate system that specifies each point uniquely in a plane by a pair of numerical values, in the form (x, y). The script discusses the conversion between polar and rectangular coordinates, which is a fundamental concept when working with polar equations.
πŸ’‘Polar Functions
Polar Functions are mathematical functions that are defined in terms of polar coordinates. The video script mentions these functions in the context of their appearance and the process of converting between rectangular and polar forms, highlighting their relevance in calculus problems involving polar coordinates.
πŸ’‘Circular Symmetry
Circular Symmetry refers to a property of shapes or mathematical functions where they exhibit symmetry about a central point or axis, forming circles or circular patterns. The script points out that problems involving circular symmetry are often easier to solve using polar coordinates due to their inherent simplicity in such contexts.
πŸ’‘Integration
Integration is a key concept in calculus that involves finding the accumulated value of a function over an interval, often represented as the area under a curve. The script introduces integration in polar coordinates as the main topic of the section, emphasizing that it is not fundamentally different from regular integration but involves different variables.
πŸ’‘Theta (ΞΈ)
Theta, often denoted by the symbol ΞΈ, is the angle in polar coordinates that represents the rotation from the reference direction (polar axis) to the line segment connecting the origin to a point on the plane. In the script, theta is used to describe the angle that defines the size of a pie wedge in a circle and is a variable that might be integrated over in polar coordinate integration.
πŸ’‘Area
Area, in the context of this video, refers to the measure of the two-dimensional space enclosed within a shape. The script discusses the area of a section of a circle, specifically a pie wedge, and provides a formula for calculating it using polar coordinates, which is essential for understanding integration in polar coordinates.
πŸ’‘Radius (R)
The Radius, denoted as R in the script, is the distance from the center (pole) of a circle to any point on the circumference. It is a fundamental component of polar coordinates and is used in the formula for the area of a pie wedge, as mentioned in the script, to calculate the area based on the angle ΞΈ.
Highlights

Introduction to the advanced calculus section focusing on polar equations and polar coordinates.

Culmination of topics in polar coordinates with a focus on area and length calculations.

Integration in polar coordinates is equivalent to tackling area and length problems.

Polar coordinates are beneficial for solving problems with circular symmetry due to their simplicity.

Conversion between rectangular and polar coordinates is a fundamental concept.

Polar functions are easier to work with when dealing with circular symmetry.

Integration in polar coordinates involves integrating along theta instead of x.

Fundamentally, polar coordinate integration is similar to regular integration.

Understanding the setup of the problem is crucial for successful integration.

Learning about the area of a section of a circle is a precursor to integration.

Area of a pie wedge in a circle is given by the formula (1/2) * r^2 * theta.

The area formula involves R squared and theta, indicating the size of the wedge.

The importance of the area formula for pie wedges in understanding polar coordinate integration.

Introduction to a coordinate system and a function represented in polar equation.

Demonstration of how to represent a polar function in a specific arc.

Defining the limits of integration in polar coordinates with angles alpha and beta.

Setting up the problem for integration by defining the start and end points in theta.

Transcripts

Browse More Related Video

Lec 33 | MIT 18.01 Single Variable Calculus, Fall 2007

Double Integrals in Polar Coordinates

Polar Coordinate System | Physics with Professor Matt Anderson | M3-02

AP Calculus BC Lesson 9.8

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

Calc 2, Exam 1 walkthrough (Fall 2023)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Advanced CalculusPolar CoordinatesIntegrationArea CalculationLength CalculationCircular SymmetryPolar FunctionsTheta IntegrationMathematics TutorialEducational Content