Lesson 9 - Area Of A Surface Of Revolution (Calculus 2 Tutor)

Math and Science
18 Aug 201604:00
EducationalLearning
32 Likes 10 Comments

TLDRThis tutorial segment dives into the concept of calculating the surface area of a shape formed by revolving a function around an axis, a process known as 'surface of revolution'. The instructor begins by explaining the idea using a simple analogy of a paper towel tube, a basic example of a surface of revolution. They then illustrate how to find the surface area of more complex shapes by applying the same principle: examining the circumference at each point along the length and multiplying by the length of the shape. The focus is on developing a methodical approach to tackle the problem, ensuring students can calculate the surface area of any function revolved around the x-axis.

Takeaways
  • πŸ“š The section focuses on the concept of finding the surface area of a shape formed by revolving a function around an axis.
  • 🎨 The idea is to visualize a 3-dimensional shape created by rotating a 2-dimensional curve around the x-axis, like a vase or a container.
  • πŸ“ To find the surface area, the tutor suggests using a method similar to calculating the surface area of a cylinder, which is a basic example of a surface of revolution.
  • πŸ”„ The process involves considering the circumference of the shape at any given point and multiplying it by the length of the shape.
  • πŸ“ The circumference is a one-dimensional measure around the end of the shape, which is then used to calculate the total surface area.
  • πŸ“ˆ The method is applied to any arbitrary shape that has been rotated around the x-axis, not just simple shapes like cylinders.
  • πŸ“‰ The tutor emphasizes the need for a bullet-proof method to calculate the surface area of these shapes, suggesting a systematic approach.
  • πŸ“ The script mentions the importance of understanding arc length, which is the starting point for calculating the surface area of the revolved shape.
  • πŸ“Š The tutor provides a visual example of a shape and its rotation to form a 3-dimensional object, highlighting the areas of interest for surface area calculation.
  • 🧩 The function that defines the shape is a key component in the process, as the surface area calculation is directly related to the function's properties.
  • πŸ” The script ends with a focus on the circumference as a critical part of the surface area calculation, leaving the audience with a clear next step in the process.
Q & A

  • What is the main topic of this section of the advanced calculus tutorial?

    -The main topic of this section is the area of a surface of revolution, which involves finding the surface area of shapes formed by revolving a function around an axis.

  • What is a surface of revolution and why is it important to calculate its area?

    -A surface of revolution is a three-dimensional shape formed by revolving a two-dimensional curve or function around an axis. Calculating its area is important for various applications in physics, engineering, and geometry where the surface area plays a critical role in understanding the shape's properties.

  • Can you provide a simple example of a surface of revolution?

    -A simple example of a surface of revolution is a cylinder, which is formed by revolving a straight line around the x-axis.

  • How is the surface area of a cylinder calculated?

    -The surface area of a cylinder is calculated by multiplying the circumference of the circular end by the height of the cylinder. The circumference is given by 2Ο€r, where r is the radius of the cylinder.

  • What is the general approach to finding the surface area of any arbitrary shape that has been rotated around an axis?

    -The general approach involves looking at the circumference of the shape at each point along the axis of rotation and then multiplying this by the length of the shape to find the total surface area.

  • Why is it necessary to have a 'bullet-proof' way to calculate the surface area of a surface of revolution?

    -A 'bullet-proof' method ensures accuracy and reliability in calculations, which is crucial for mathematical consistency and for applications where precise measurements are required.

  • What is the relationship between arc length and the surface area of a surface of revolution?

    -Arc length is the length of a curve along the x-axis, and it plays a role in determining the circumference at each point of the shape when it is revolved around the axis. This circumference is then used to calculate the surface area.

  • How does the script suggest we visualize the process of finding the surface area of a shape that has been rotated?

    -The script suggests visualizing the process by comparing it to the circumference of a paper towel tube, where the circumference is multiplied by the length of the tube to find the surface area.

  • What is the significance of the function in the context of a surface of revolution?

    -The function is significant because it defines the shape of the curve that is being revolved around the axis to form the three-dimensional surface. The surface area calculation is based on the properties of this function.

  • What are the components of the surface area calculation for a surface of revolution that the script mentions?

    -The components mentioned in the script include the circumference of the shape at each point along the axis of rotation and the length of the shape, which are used to calculate the total surface area.

Outlines
00:00
πŸ“š Introduction to Surface Area of Revolution

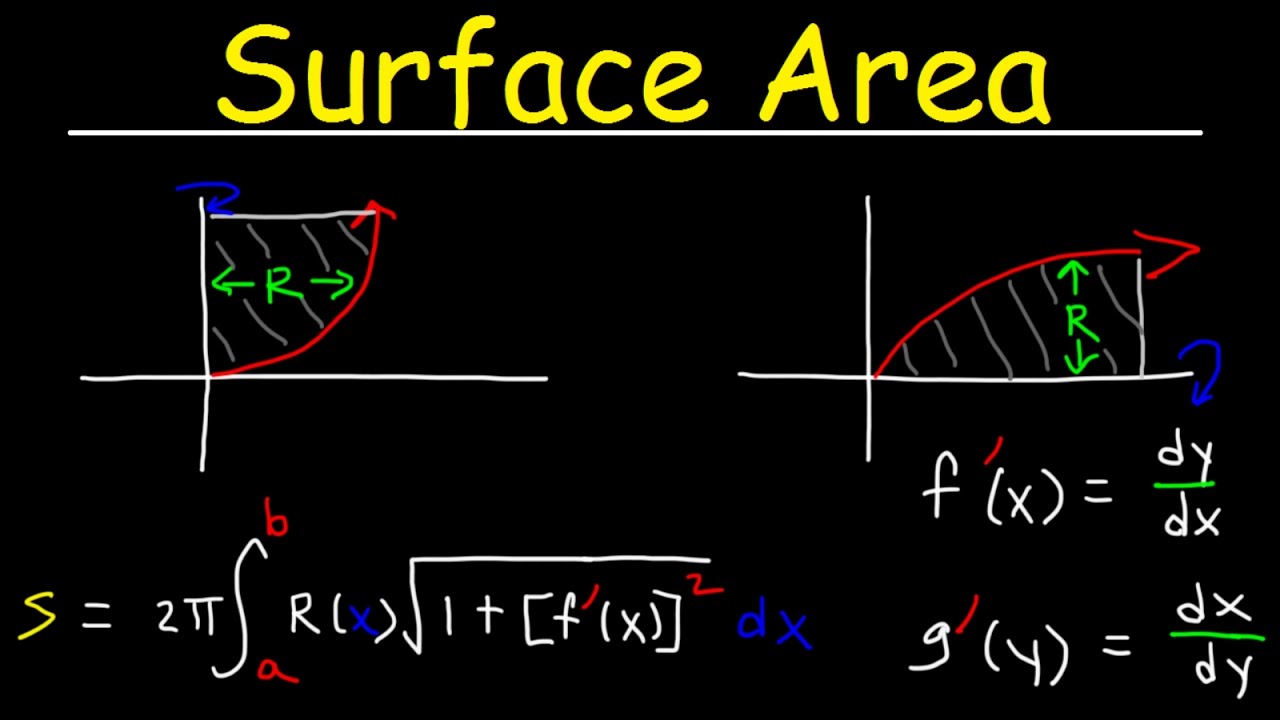
This paragraph introduces the concept of the surface area of revolution, which is the area of a three-dimensional shape formed by revolving a two-dimensional curve around an axis. The instructor uses the analogy of a function as a boundary to create a shape like a vase when rotated around the x-axis. The goal is to find the surface area of such shapes, which are symmetrical due to the revolving process. The paragraph sets the stage for the mathematical exploration that will follow, emphasizing the importance of having a systematic method to calculate these areas.

Mindmap
Keywords
πŸ’‘Advanced Calculus
Advanced Calculus refers to a higher level of calculus studies that delve into more complex concepts beyond the introductory level. In the context of the video, it indicates that the content is intended for individuals who have a solid understanding of calculus and are ready to tackle more sophisticated topics such as surface area of revolution.
πŸ’‘Arc Length
Arc length is a measure of the distance along the curved path between two points on a circle or any curve. In the video, arc length is foundational knowledge that is built upon to understand how to calculate the surface area of a shape that has been revolved around an axis.
πŸ’‘Surface Area of Revolution
This concept refers to the calculation of the total area of the surface formed when a two-dimensional shape or curve is rotated around an axis, creating a three-dimensional object. The video's main theme revolves around explaining how to determine this area for various shapes.
πŸ’‘Three-Dimensional Shape
A three-dimensional shape, or 3D shape, is an object with length, width, and height. In the script, the tutor discusses creating 3D shapes like vases or containers by revolving a 2D curve around the x-axis, which is a key concept in finding the surface area of revolution.
πŸ’‘Revolved Function
A revolved function is a mathematical function that, when rotated around a certain axis, forms a 3D shape. The video explains how to calculate the surface area of these shapes, which are created by revolving a function around an axis.
πŸ’‘Symmetric
Symmetric, in the context of the video, refers to shapes that are mirror images of each other across an axis. This property is inherent in shapes formed by revolving a function around an axis and is important for understanding the uniformity in the surface area calculations.
πŸ’‘Cylinder
A cylinder is a basic geometric shape that consists of two parallel circular bases connected by a curved surface. The script uses a cylinder as an example of a surface of revolution, explaining how its surface area can be calculated by multiplying the circumference of the base by the height of the cylinder.
πŸ’‘Circumference
Circumference is the total length around a circle or circular path. In the video, the tutor explains that the surface area of a cylinder, and by extension more complex shapes of revolution, can be found by considering the circumference of the circular cross-section and its relation to the length of the shape.
πŸ’‘Coordinate System
A coordinate system is a standardized way of assigning pairs of numerical values, known as coordinates, to each point on a plane. In the script, the coordinate system is used to visualize and discuss the shapes and functions that are being revolved around the x-axis to form 3D shapes.
πŸ’‘Function
In mathematics, a function is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. The video script mentions functions as the basis for the shapes that are revolved to create 3D objects, with the function governing the arc length of the shape.
Highlights

Introduction to the topic of area of a surface of revolution in advanced calculus.

Building upon the concept of arc length from the previous section.

Explanation of forming a 3-dimensional shape by revolving a function around an axis.

Desire to find the surface area of shapes that have been revolved.

The importance of having a bullet-proof method for calculating surface areas.

Using a roll of paper towels as an example of the simplest surface of revolution.

Conceptual understanding of surface area calculation through the example of a cylinder.

Formula for the surface area of a cylinder: circumference multiplied by the length.

Application of the technique of circumference and length multiplication to any shape.

Introduction of an arbitrary shape and its rotation around the x-axis to form a 3D shape.

Visual representation of the resulting shape after rotation, resembling a vase.

Identification of the area of interest for surface area calculation along the shape.

Acknowledgment that the shape is governed by a function, f(x).

The analogy of finding circumference as a step towards calculating surface area.

Transcripts

Browse More Related Video

Calculus Chapter 4 Lecture 36 Surface Area

Calculating volume by known cross sections

Surface Area of Revolution By Integration Explained, Calculus Problems, Integral Formula, Examples

Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method

Lesson 12 - Surface Area Of Revolution In Parametric Equations

The Math of Bubbles // Minimal Surfaces & the Calculus of Variations #SoME3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Surface AreaCalculus 2Revolved ShapesArc LengthCylinder Geometry3D ShapesMath TutorialArea CalculationAdvanced MathEducational Content