5 Examples of Solving Centripetal Force Problems - IB Physics
TLDRThis script offers an in-depth exploration of centripetal force in physics, presenting five distinct scenarios to illustrate its application. It begins with horizontal circular motion, discussing objects on a spinning surface, the effect of holding a bar, and the role of friction in a car's turn. It then transitions to banked roads, explaining how the angle assists in turning. The script also covers tension at an angle with a spinning ball on a wire and delves into vertical circular motion, including examples of a car going over a hill and trough. Finally, it tackles the complexities of a loop-de-loop, detailing the forces at play at various points. Throughout, the script emphasizes the importance of understanding the net force in circular motion and provides mathematical insights to solve related problems.
Takeaways
- 🔄 Centripetal force problems can be categorized into common situations, and understanding these can help solve a variety of physics problems.
- 📚 For horizontal circular motion, the net centripetal force is horizontal, and vertical forces cancel out, simplifying the force equations.
- 🧲 In horizontal uniform circular motion, friction can act as the centripetal force, pulling an object toward the center of the circle.
- 🤔 When an object is held in place on a spinning surface, both friction and an applied force (like holding a bar) contribute to the centripetal force.
- 🚗 In a car turning scenario, the centripetal force is provided by the friction between the tires and the road.
- 🛣️ Banked roads or tracks can reduce the reliance on friction for centripetal force by using the normal force component to assist in turning.
- 🎢 The physics of a car going over a hill or through a trough involves understanding how gravity and normal forces combine to create centripetal force.
- 🤹♂️ Tension in a horizontal circular motion, such as a ball on a wire, can be broken down into components to find the centripetal force.
- 🔄 At the top of a loop-de-loop, the minimum velocity required to maintain circular motion is when the centripetal force equals the force of gravity.
- 🔄 At the bottom of a loop-de-loop, the centripetal force is the difference between the normal force and gravity, highlighting the role of the normal force in circular motion.
- 📉 As velocity decreases, the centripetal force also decreases, which can lead to objects losing the necessary force to maintain their circular path, especially at the top of a loop.
Q & A
What is the formula for centripetal force in the context of circular motion?
-The formula for centripetal force in circular motion is \( F_c = \frac{mv^2}{r} \), where \( F_c \) is the centripetal force, \( m \) is the mass of the object, \( v \) is the velocity, and \( r \) is the radius of the circular path.
Why do forces in the y-direction cancel out in horizontal circular motion?
-In horizontal circular motion, the net centripetal force is perfectly horizontal, which means that all y-components of the forces cancel out. This is because the motion is confined to the horizontal plane, and no vertical acceleration occurs.
What is the role of friction in an object resting on a horizontally spinning surface?
-Friction plays a crucial role in an object resting on a horizontally spinning surface by providing the necessary horizontal force that pulls the object toward the center of the circle, allowing it to maintain circular motion.
How does holding onto a bar while spinning help in horizontal circular motion?
-Holding onto a bar while spinning provides an applied force that assists the force of friction. This reduces the amount of friction needed from the surface to keep the person moving in a circle, making it easier to maintain the motion.
What is the centripetal force acting on a car turning on a flat road?
-The centripetal force acting on a car turning on a flat road is the force of friction between the tires and the road surface, which is directed horizontally towards the center of the circular path.
Why are banked roads beneficial for vehicles making turns?
-Banked roads are beneficial because they angle the surface to help provide a component of the normal force that points towards the center of the circular path, reducing the reliance on friction and making it easier for vehicles to turn.
How does the normal force on a banked road contribute to the centripetal force?
-On a banked road, the normal force has a component (normal force times sine of the angle) that points towards the center of the circle, contributing to the centripetal force and allowing the vehicle to maintain its circular path.
What is the relationship between the tension in a wire and the centripetal force when a ball is attached and spinning in a horizontal circle?
-The tension in the wire provides the centripetal force necessary for the ball to move in a horizontal circle. The tension is broken down into components, with the horizontal component (tension times sine of the angle) equal to the centripetal force \( \frac{mv^2}{r} \).
How does the radius of curvature relate to circular motion?
-The radius of curvature is the radius of the imaginary full circle that would be formed if the circular path, such as a hill, were extended. It is used to describe the curvature of the path and is crucial in calculating the centripetal force on an object moving with constant velocity along that path.
What is the minimum speed required for a car to maintain circular motion at the top of a loop-de-loop?
-The minimum speed required for a car to maintain circular motion at the top of a loop-de-loop is when the centripetal force equals the force of gravity acting on the car, ensuring that there is no net force causing the car to fall off the loop.
How does the normal force compare to the force of gravity at the bottom and top of a loop-de-loop?
-At the bottom of a loop-de-loop, the normal force must be greater than the force of gravity to provide the centripetal force needed for circular motion. At the top, the normal force must be equal to the force of gravity when the car is at its minimum speed to maintain the motion.
What force prevents a car from slipping down due to gravity while moving sideways on a loop-de-loop?
-While moving sideways on a loop-de-loop, the force of friction prevents the car from slipping down due to gravity, balancing out the downward force and allowing the car to continue its circular motion.
Outlines
🔄 Understanding Centripetal Force in Horizontal and Vertical Circles
This paragraph introduces the concept of centripetal force and its application in physics problems. It outlines five different scenarios to illustrate how centripetal force operates in various situations. The explanation begins with horizontal circular motion, emphasizing that in a perfect horizontal circle, all vertical forces cancel out, simplifying the problem to forces in the x-direction. The paragraph also touches on vertical circular motion, noting that the net force must lie in the plane of the circle. Three initial situations are introduced, starting with an object on a horizontally spinning surface, where friction provides the centripetal force. The explanation includes a free body diagram and a force table to demonstrate the relationship between gravitational force, normal force, and friction.
🚗 Centripetal Force in Horizontal Uniform Circular Motion
The second paragraph delves into horizontal uniform circular motion with three distinct examples. The first scenario considers an object on a spinning horizontal surface, where the centripetal force is provided by friction. The paragraph explains how the force of friction is calculated and how it equates to mv^2/r. The second situation introduces an individual holding a bar while spinning, adding an applied force to the equation, which reduces the reliance on friction. The third example discusses a car turning, where the road's friction serves as the centripetal force. A banked road is then introduced as a design feature to reduce the dependency on friction, with a detailed explanation of the forces involved and how the normal force's components contribute to the centripetal force.
🤹♂️ Tension at an Angle and Circular Motion in Horizontal Planes
This paragraph presents a scenario where a ball attached to a wire is spinning in a horizontal circle, introducing the concept of tension at an angle. The explanation involves calculating the radius of the ball's path based on the wire's length and the angle of the wire. A free body diagram illustrates the forces acting on the ball: tension and gravity. The force of tension is then broken down into its components, and a force table is used to show how these components relate to the centripetal force mv^2/r. The paragraph concludes by demonstrating how to use the equations derived from the force table to find missing variables in the problem.
🎢 Vertical Circular Motion: Hills, Troughs, and Loop-de-loops
The fourth paragraph explores vertical circular motion, starting with a car going over a hill, which is conceptualized as part of a larger circular path with a 'radius of curvature.' The forces acting on the car are identified as gravity and the normal force, with the net centripetal force calculated as the difference between these two forces. The discussion then moves to a trough, where the normal force exceeds gravity, and the centripetal force is the sum of the normal force and gravity. The paragraph culminates with a complex example of a car navigating a loop-de-loop, requiring an understanding of the forces at play at various points along the loop to maintain circular motion.
🔄 Minimum Velocity for Circular Motion in a Loop-de-loop
The final paragraph focuses on determining the minimum velocity required for a car to successfully navigate a loop-de-loop. It explains that the centripetal force must equal the force of gravity at the top of the loop to maintain motion. As the car's velocity decreases, the centripetal force also decreases, necessitating adjustments in the other forces to maintain equilibrium. The paragraph describes the forces at the bottom, side, and top of the loop and how they contribute to the centripetal force. It concludes by emphasizing that the minimum velocity is when the centripetal force equals the force of gravity, and provides insights into the relationship between normal force, centripetal force, and gravity at different points of the loop.
Mindmap
Keywords
💡Centripetal Force
💡Uniform Circular Motion
💡Free Body Diagram
💡Friction
💡Normal Force
💡Gravity
💡Tension
💡Banked Road
💡Loop-de-loop
💡Radius of Curvature
Highlights
Understanding centripetal force in horizontal and vertical circular motion with five example scenarios.
Horizontal circular motion implies net centripetal force is horizontal with all Y components canceling out.
Vertical circular motion requires centripetal force to exist on the plane of the circle, never pointing outside.
An object on a horizontally spinning surface experiences centripetal force provided by friction.
Holding onto a bar while spinning reduces the frictional force needed to maintain circular motion.
In a car turning, friction acts as the centripetal force, allowing the turn without skidding.
Banked roads facilitate turns by altering the relationship between normal force, gravity, and centripetal force.
The angle of a banked road can be determined by the components of the normal force.
Tension at an angle in horizontal circular motion can be decomposed into X and Y components.
The radius of motion in a horizontal circular path can be calculated using the length of the rope and the angle.
Circular motion over a hill involves gravity and normal force to provide the centripetal force.
The maximum speed of a car over a hill occurs when the centripetal force equals gravity.
In a trough, the centripetal force is the normal force minus gravity, determining the car's motion.
Loop-de-loop motion requires analyzing forces at different points to maintain circular motion.
The minimum velocity for a loop-de-loop is when centripetal force equals gravity at the top.
At the minimum velocity, the normal force at the bottom of a loop-de-loop is twice the force of gravity.
Mastering these examples provides a foundation for solving various circular motion problems.
Transcripts
Browse More Related Video
Dynamics of Uniform Circular Motion

Centripetal force problem solving | Centripetal force and gravitation | Physics | Khan Academy
Understanding Circular Motion
Centripetal Acceleration & Force - Circular Motion, Banked Curves, Static Friction, Physics Problems

Uniform Circular Motion Free Body Diagrams
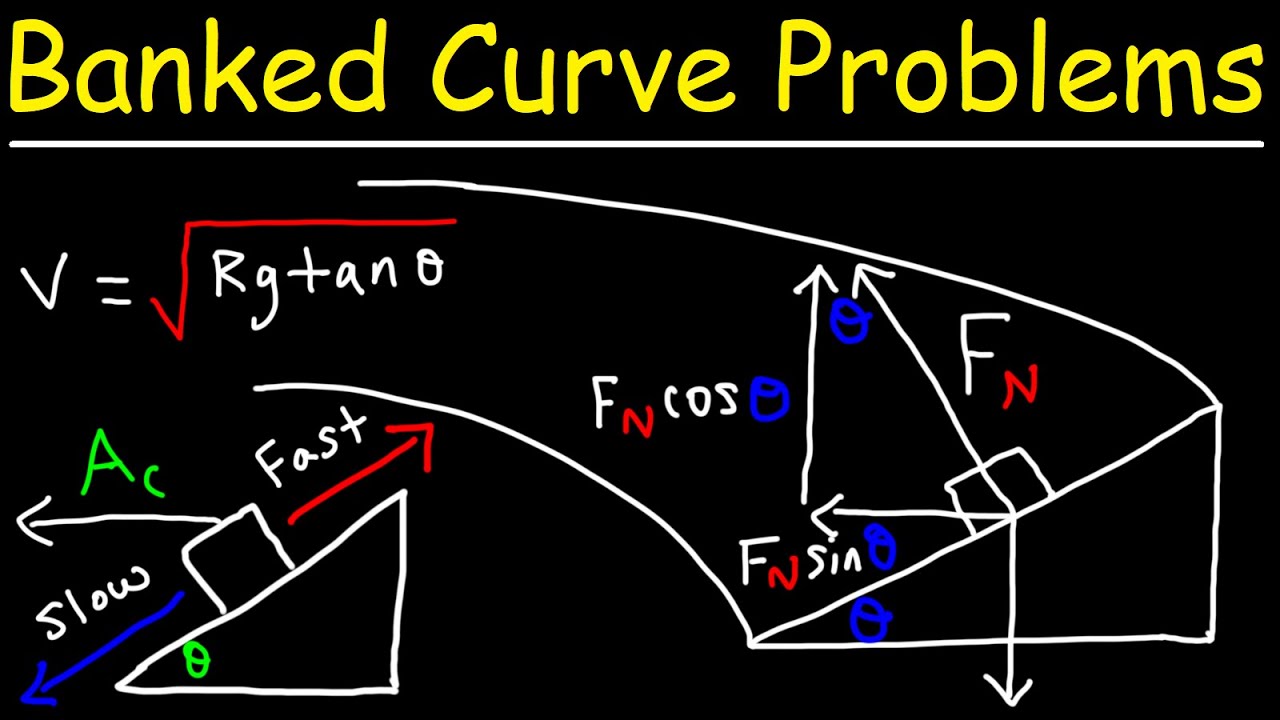
Banked turn Physics Problems
5.0 / 5 (0 votes)
Thanks for rating: