How to solve Complex Numbers using Casio Calculator #jonahemmanuel #complexnumbers

Excellence Academy
9 Jun 202310:26
EducationalLearning
32 Likes 10 Comments

TLDRThis instructional video teaches viewers how to use a Casio FX 991es Plus calculator to perform arithmetic operations on complex numbers. The presenter guides through setting the calculator to complex number mode, demonstrating addition, subtraction, multiplication, and division of complex numbers. Additionally, the video covers how to calculate the argument and modulus of a complex number, as well as finding its conjugate, providing a comprehensive guide for students to enhance their understanding and calculations involving complex numbers.

Takeaways
  • 📚 The video is a tutorial on using a calculator to perform operations with complex numbers.
  • 🔢 The specific operations covered include addition, subtraction, multiplication, and division of complex numbers.
  • 💡 The calculator used in the tutorial is a Casio FX 991es Plus.
  • 🔑 The first step is to set the calculator to complex number mode, which is essential for all subsequent operations.
  • 👉 The 'Eng' button is used to access the imaginary unit 'i', which is crucial for working with complex numbers.
  • 📈 To add complex numbers, you add the real parts together and the imaginary parts together.
  • ➖ For subtraction, you subtract the real parts and the imaginary parts of the complex numbers.
  • 🔄 Multiplication of complex numbers involves using brackets and following specific mathematical rules.
  • 📉 Division of complex numbers is also demonstrated, with a separate tutorial video provided for detailed steps.
  • 📐 The video shows how to find the argument (angle) of a complex number using the calculator's argument function.
  • 🎯 The conjugate of a complex number can be found easily by changing the sign of the imaginary part.
  • 📏 The modulus of a complex number, along with its argument, can be obtained simultaneously using specific calculator functions.
Q & A

  • What is the first step to solve complex numbers problems using a calculator?

    -The first step is to put the calculator in complex number mode.

  • How do you switch the calculator to complex number mode on a Casio FX 991es Plus?

    -You go to the 'mode' option on the calculator, navigate to 'comp' for complex, and select '2' to enable complex number mode.

  • What does 'I' represent in the context of complex numbers on the calculator?

    -'I' represents the imaginary unit, which is equal to the square root of -1.

  • How do you perform addition of complex numbers on the calculator?

    -You add the real parts together and the imaginary parts together, then combine the results.

  • Can you give an example of adding two complex numbers using the calculator?

    -Sure, if you add 2 - 3i and 4 + 5i, you would add the real parts (2 + 4 = 6) and the imaginary parts (-3 + 5 = 2), resulting in 6 + 2i.

  • What is the process for subtracting complex numbers on the calculator?

    -You subtract the real parts from each other and the imaginary parts from each other, then combine the results.

  • How do you multiply complex numbers using the calculator?

    -You put each complex number in brackets and multiply them as per the rules of complex multiplication.

  • What does the 'argument' of a complex number represent?

    -The 'argument' of a complex number is the angle it makes with the positive real axis in the complex plane, and it can be found using the calculator's argument function.

  • How can you find the argument of a complex number using the calculator?

    -You use the argument function by pressing shift, then the complex number mode (2), and then the argument option (1), followed by entering the complex number.

  • What is the conjugate of a complex number and how do you find it on the calculator?

    -The conjugate of a complex number is obtained by changing the sign of the imaginary part. On the calculator, you use the conjugate function by pressing shift, then the complex number mode (2), and then the conjugate option (2).

  • How can you find both the modulus and argument of a complex number simultaneously using the calculator?

    -After entering the complex number, press shift and then control number 2, followed by 3 to get both the modulus (Arrow) and the argument (Theta).

  • What is the modulus of a complex number and how can you calculate it with the calculator?

    -The modulus of a complex number is its distance from the origin in the complex plane. You can calculate it using the calculator by entering the complex number and then using the modulus function (option 3 after pressing shift and control number 2).

Outlines
00:00
📚 Introduction to Calculator Operations with Complex Numbers

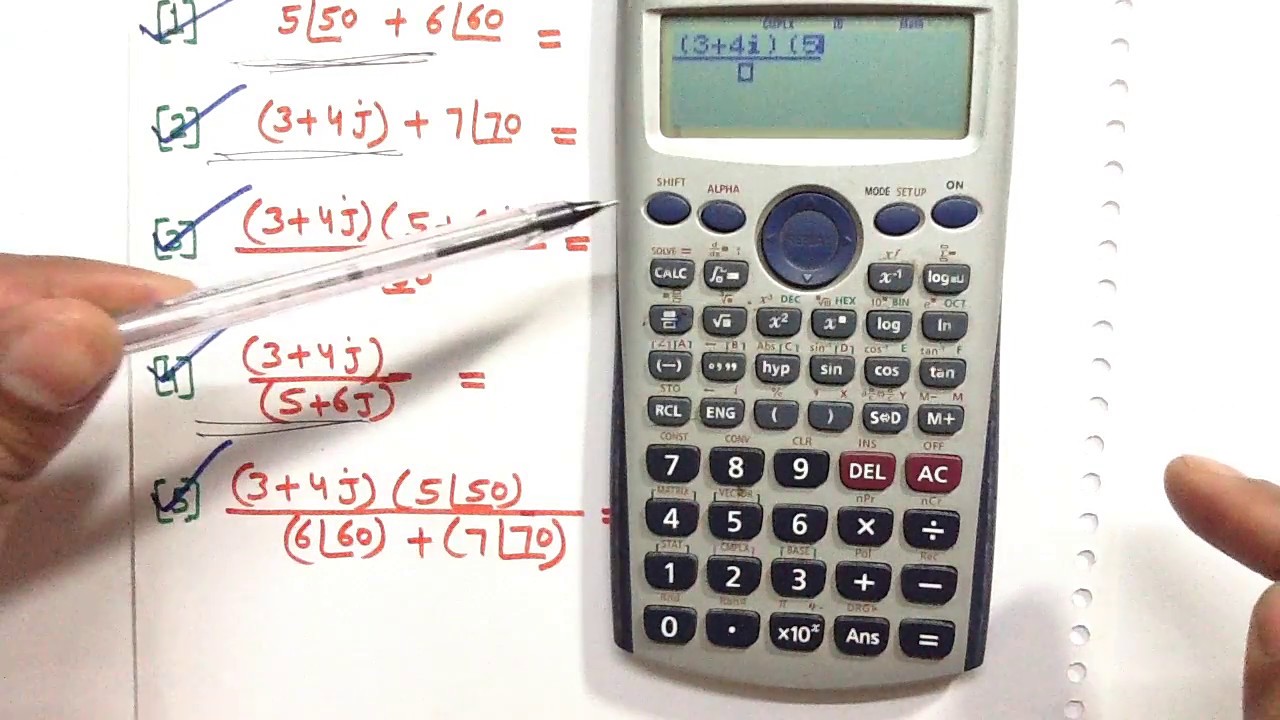
This paragraph introduces the video's focus on using a calculator to perform operations with complex numbers. The instructor begins by welcoming viewers to the class and stating the goal of the tutorial. The Casio FX 991es Plus calculator is presented, and it's mentioned that there's a prior tutorial on complex numbers, which will be linked in the video description. The primary task is to set the calculator to complex number mode, which is achieved by navigating through the 'mode' menu and selecting 'complex'. The importance of this step is emphasized as it enables the calculator to handle complex numbers correctly. The instructor then demonstrates how to add complex numbers using the calculator, providing an example and showing the result.

05:01
🔍 Advanced Calculator Techniques for Complex Numbers

This paragraph delves into more advanced calculator techniques for working with complex numbers. It starts by referencing a previous video that explains the step-by-step process for solving complex number problems. The instructor then moves on to show how to find the argument (angle) of a complex number using the calculator's built-in functions. The process involves using the 'complex' and 'argument' functions to quickly determine the angle. The paragraph also covers how to find the conjugate of a complex number, which involves changing the sign of the imaginary part. The instructor provides examples for both the argument and conjugate, demonstrating the calculator's capabilities. Additionally, a method to find both the modulus and argument of a complex number simultaneously is introduced, showcasing the calculator's efficiency in handling complex number operations.

10:03
👋 Conclusion and Encouragement for Further Exploration

In the concluding paragraph, the instructor summarizes the video's content, which includes teaching how to add, subtract, multiply, and divide complex numbers using a calculator. They also covered how to find the argument, modulus, and conjugate of a complex number. The instructor expresses hope that the tutorial will help viewers improve their understanding and calculations with complex numbers. They sign off by saying goodbye and indicating that they will see the viewers in the next calculator class, encouraging further learning and exploration of complex numbers.

Mindmap
Keywords
💡Calculator
A calculator is an electronic device used to perform arithmetic operations and more complex calculations. In the context of this video, the Casio FX 991es Plus calculator is specifically used to solve problems involving complex numbers. The script demonstrates how to input complex numbers and perform operations like addition, subtraction, multiplication, and division using the calculator.
💡Complex Numbers
Complex numbers are numbers that consist of a real part and an imaginary part, often written in the form a + bi, where 'a' and 'b' are real numbers, and 'i' is the imaginary unit with the property that i^2 = -1. The video's theme revolves around complex numbers, showing viewers how to use a calculator to perform various operations with them.
💡Complex Mode
Complex mode is a setting on a scientific calculator that allows it to perform operations with complex numbers. The script emphasizes the importance of switching the calculator to complex mode before attempting to solve complex number problems, as this enables the calculator to handle 'i' as the imaginary unit.
💡Addition
Addition is the mathematical operation of combining two or more numbers to find their total or sum. In the script, the process of adding complex numbers is explained, where the real parts are added together and the imaginary parts are added together separately, resulting in a new complex number.
💡Subtraction
Subtraction is the mathematical operation of taking one number away from another. The video script illustrates how to subtract one complex number from another by subtracting their real parts and their imaginary parts separately, yielding a result in complex number form.
💡Multiplication
Multiplication is a fundamental arithmetic operation where numbers are combined by repeated addition. The script demonstrates the multiplication of complex numbers, which involves a specific set of rules to ensure the correct result is obtained, as shown in the video.
💡Division
Division is the process of splitting a number into a number of equal parts or groups. The video explains how to divide one complex number by another, which typically involves multiplying by the conjugate of the divisor and simplifying the result.
💡Argument
In the context of complex numbers, the argument (or angle) of a complex number is the angle it makes with the positive real axis in the complex plane. The script shows how to use the calculator to find the argument of a complex number, which is an essential part of understanding its geometric representation.
💡Conjugate
The conjugate of a complex number is obtained by changing the sign of the imaginary part while keeping the real part the same. For example, the conjugate of a + bi is a - bi. The script explains how to find the conjugate of a complex number using the calculator, which is useful for various mathematical operations, including division of complex numbers.
💡Modulus
The modulus (or magnitude) of a complex number is a measure of its distance from the origin in the complex plane. It is calculated as the square root of the sum of the squares of the real and imaginary parts. The video script describes how to calculate the modulus of a complex number using the calculator, which is important for understanding the size or length of the complex number.
Highlights

Introduction to using a calculator for complex number operations

Setting calculator to complex number mode using 'mode' and selecting 'complex'

Engaging with the calculator's 'Eng' button to access complex number 'i'

Demonstration of adding complex numbers using the calculator

Explanation of adding real and imaginary parts separately for complex number addition

Subtraction of complex numbers by subtracting real and imaginary parts

Multiplication of complex numbers using brackets and the equal button

Division of complex numbers by changing operation and using equal

Finding the argument (angle) of a complex number using calculator functions

Using the 'argument' function to quickly find the angle of complex numbers

Conjugate of a complex number obtained by changing the sign of the imaginary part

Method to find both modulus and argument of a complex number simultaneously

Calculating the modulus using the 'modulus and argument' function on the calculator

Practical applications of calculator functions for complex number problems

Encouragement to explore calculator functions and try with other examples

Summary of operations demonstrated: addition, subtraction, multiplication, division, modulus, and argument of complex numbers

Closing remarks and sign-off for the calculator heart class

Transcripts

Browse More Related Video

how to use CASIO FX-991ES in (ELECTRICAL ENGINEERING ) (Polar and Rectangular )------1

Casio FX-991ES Plus and FX-115ES Plus 2nd Edition, Learn All Features

Complex Numbers - Basic Operations

Ch. 1.6 Complex Numbers

Imaginary Numbers - Basic Introduction

Basic complex analysis | Imaginary and complex numbers | Precalculus | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Complex NumbersCalculator TutorialMathematics ClassAdditionSubtractionMultiplicationDivisionImaginary UnitArgumentModulusConjugate