Complex Numbers - Basic Operations
TLDRThis educational video script delves into the realm of complex numbers, offering a comprehensive guide on their various operations and applications. It begins with the basics of graphing complex numbers and calculating their absolute values, using the standard form a + bi. The script progresses to cover arithmetic operations such as addition, subtraction, multiplication, and division of complex numbers. It also explores solving equations involving complex numbers, simplifying square roots of negative numbers, and understanding the properties of i raised to different powers. The video aims to equip viewers with the ability to manipulate and solve complex number problems effectively, providing clear explanations and step-by-step examples.
Takeaways
- 🔍 The script explains the concept of complex numbers, including their standard form (a + bi), where 'a' is the real part and 'b' is the imaginary part.
- 📈 It covers how to graph complex numbers on the complex plane with the real part on the x-axis and the imaginary part on the y-axis.
- 🧮 The video demonstrates calculating the absolute value (or modulus) of a complex number using the formula √(a^2 + b^2), which represents the hypotenuse of a right triangle formed by the real and imaginary parts.
- 📚 Examples are provided to illustrate the process of graphing and calculating the absolute value for specific complex numbers, such as 3 + 4i, -5 + 12i, and 8 - 15i.
- 🤔 The script discusses simplifying square roots of negative numbers, which results in imaginary numbers, and introduces the concept of special right triangles to help with these calculations.
- 📉 It explains the process of simplifying powers of 'i' (the imaginary unit), using the cyclical nature of i, i^2, i^3, and i^4 to find the simplified form of higher powers of 'i'.
- ➕ The transcript covers the arithmetic operations of adding and subtracting complex numbers by combining like terms (real with real and imaginary with imaginary).
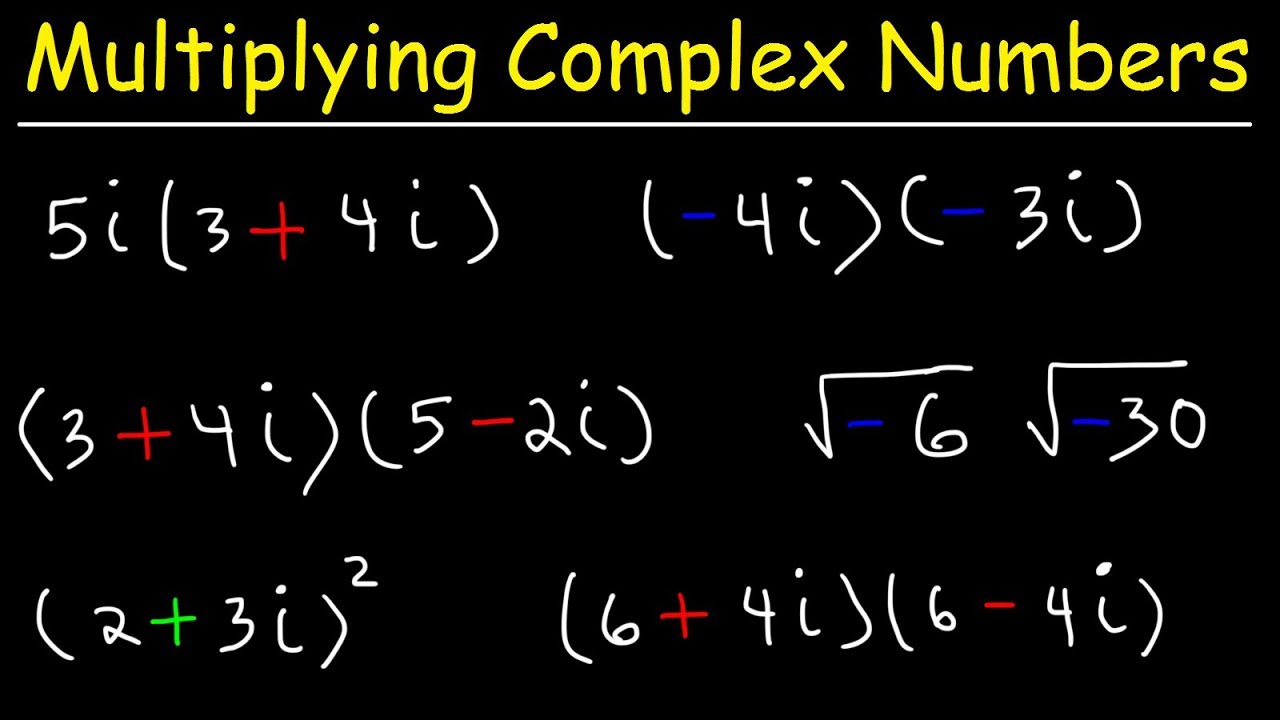
- ➗ It also explains how to multiply and divide complex numbers, including multiplying by the conjugate to eliminate the imaginary part and obtaining a real number.
- 🔢 The process of solving quadratic equations with complex solutions is detailed, including using the quadratic formula and factoring techniques.
- 🔑 The script highlights the relationship between the sum and product of the roots of a quadratic equation and the coefficients of the equation (sum = -b/a, product = c/a).
- 📝 Finally, the importance of understanding the difference between multiplying and dividing complex numbers and the need to use the conjugate in division to simplify expressions is emphasized.
Q & A
What is the standard form of a complex number?
-The standard form of a complex number is written as a plus bi, where 'a' represents the real portion and 'b' represents the imaginary portion, with 'i' being the imaginary unit.
How do you graph a complex number like 3 + 4i?
-To graph the complex number 3 + 4i, you move three units to the right on the real axis (x-axis) and four units up on the imaginary axis (y-axis), and mark the point at that location.
What is the absolute value or modulus of a complex number 3 + 4i?
-The absolute value of a complex number 3 + 4i is calculated as the square root of the sum of the squares of its real and imaginary parts, which is √(3^2 + 4^2) = √(9 + 16) = √25 = 5.
How do you simplify the square root of a negative number, such as the square root of -4?
-The square root of a negative number is an imaginary number. For example, the square root of -4 is 2i, because (2i)^2 = 4i^2 = 4*(-1) = -4.
What are the most common right triangles used in complex number calculations?
-The most common right triangles used in complex number calculations are the 3-4-5, 5-12-13, and 8-15-17 triangles, which are based on Pythagorean triples.
How do you add complex numbers, for example, 5 + 2i and 3 + 7i?
-To add complex numbers, you combine like terms. For 5 + 2i and 3 + 7i, you add the real parts (5 + 3) and the imaginary parts (2i + 7i) to get 8 + 9i.
What is the process for multiplying complex numbers?
-To multiply complex numbers, you use the distributive property (FOIL). For example, for (a + bi) and (c + di), you would multiply (a*c) + (a*di) + (b*c) + (b*di), and then combine like terms.
Can you provide an example of dividing one complex number by another?
-To divide one complex number by another, you multiply the numerator and denominator by the conjugate of the denominator. For example, to divide (4 + 3i) by (5 - 2i), you would multiply both by the conjugate of (5 - 2i), which is (5 + 2i), and simplify the resulting expression.
How do you solve a quadratic equation with complex solutions?
-To solve a quadratic equation with complex solutions, you can use the quadratic formula: x = (-b ± √(b^2 - 4ac)) / 2a. If the discriminant (b^2 - 4ac) is negative, the solutions will be complex.
What is the relationship between the solutions of a quadratic equation and its coefficients?
-The sum of the solutions of a quadratic equation ax^2 + bx + c = 0 is given by -b/a, and the product of the solutions is given by c/a.
Outlines
📚 Introduction to Complex Numbers
This paragraph introduces the concept of complex numbers, which consist of a real part and an imaginary part. It explains the standard form of complex numbers, denoted as 'a + bi', where 'a' is the real number, and 'b' is the coefficient of the imaginary unit 'i'. The imaginary unit 'i' is defined as the square root of -1. The paragraph also outlines the topics that will be covered in the video, such as graphing complex numbers, calculating their absolute values, and performing arithmetic operations like addition, subtraction, multiplication, and division.
📈 Graphing and Absolute Value of Complex Numbers
The speaker demonstrates how to graph complex numbers on the complex plane, which consists of a horizontal real axis and a vertical imaginary axis. By using the example of the complex number 3 + 4i, the process involves moving three units along the real axis and four units along the imaginary axis to plot the point. The absolute value, or modulus, of a complex number 'a + bi' is calculated as the square root of 'a^2 + b^2', which geometrically represents the distance from the origin to the point on the complex plane. The paragraph also provides additional examples, including negative 5 + 12i and 8 - 15i, to illustrate the graphing and absolute value calculation processes.
🔍 Simplifying Square Roots of Negative Numbers
This section discusses the simplification of the square roots of negative numbers, which result in imaginary numbers. The imaginary unit 'i' is equal to the square root of -1. The speaker simplifies several expressions involving the square root of negative integers, such as √(-4) which simplifies to '2i', and √(-9) which simplifies to '3i'. The paragraph also covers the simplification of non-perfect square negatives like √(-18), which becomes '3√2i' after factoring out the largest perfect square, 9, and taking the square root of the remaining 2.
🌐 Powers of i and Simplifying Complex Expressions
The speaker explains the cyclical nature of powers of the imaginary unit 'i'. It is established that i^2 = -1, i^3 = -i, and i^4 = 1. This pattern repeats every four powers. The paragraph then applies this knowledge to simplify expressions like i^201 by breaking down the exponent and using the established pattern. The process involves dividing the exponent by 4 and using the quotient and remainder to determine the equivalent power of 'i' that can be simplified. Several examples are provided to illustrate this process.
🧩 Addition and Subtraction of Complex Numbers
This paragraph focuses on the addition and subtraction of complex numbers. The process involves combining like terms, meaning the real parts are added or subtracted together, and the imaginary parts are combined separately. Examples given include adding (5 + 2i) + (3 + 7i) to get (8 + 9i), and subtracting (4 + 8i) - (3 - 5i) to obtain (1 + 13i). The absolute value of the resulting complex number is also calculated as the square root of the sum of the squares of the real and imaginary parts.
🤔 Multiplication of Complex Numbers and i^2
The multiplication of complex numbers is explained, with an emphasis on the property that i^2 equals -1. The process involves the distributive property (FOIL method) and combining like terms. An example given is (5 - 3i)(4 + 7i), which results in (41 + 23i) after multiplying and simplifying. The paragraph also touches on multiplying complex numbers by their conjugates, which eliminates the imaginary parts and results in real numbers.
🔢 Division of Complex Numbers and Conjugates
The paragraph explains how to divide complex numbers by multiplying the numerator and denominator by the conjugate of the denominator. This process is demonstrated with the example of (4 + 3i) / (5 - 2i), which simplifies to (14 + 23i) / 29 after multiplying by the conjugate (5 + 2i). The result is then expressed in standard form, a + bi. The concept of multiplying by i to eliminate the imaginary unit in division is also introduced.
📉 Solving Quadratic Equations with Complex Solutions
The speaker discusses solving quadratic equations that result in complex solutions. The quadratic formula is used to find the roots of equations that are not factorable. The process involves identifying the coefficients a, b, and c, and applying the formula x = (-b ± √(b^2 - 4ac)) / (2a). The solutions are presented in the form of a ± bi. An example given is 3x^2 + 4x + 7 = 0, which yields complex solutions after applying the quadratic formula.
🔍 Factoring Sum and Difference of Perfect Squares
This paragraph covers the factoring of quadratic expressions that represent the sum and difference of perfect squares. The difference of perfect squares, such as x^2 - 36, can be factored into (x + 6)(x - 6), resulting in real solutions. In contrast, the sum of perfect squares, like x^2 + 36, factors into (x + 6i)(x - 6i), yielding complex solutions. The paragraph also includes examples of solving for x in various quadratic equations using factoring.
📝 Writing Quadratic Equations from Given Solutions
The process of constructing a quadratic equation from given solutions, both real and imaginary, is explained. If given imaginary solutions like 3i, the equation is formed by setting the product of factors equal to zero, such as (x - 3i)(x + 3i) = 0, which simplifies to x^2 + 9 = 0. If given one of a pair of complex solutions, such as 4 + 3i, the conjugate 4 - 3i is also considered, and the equation is formed by multiplying the conjugate pairs, resulting in x^2 - 8x + 25 = 0.
📉 Sum and Product of Roots in Quadratic Equations
The final paragraph discusses finding the sum and product of the roots of a quadratic equation in the form ax^2 + bx + c. The sum of the roots is given by -b/a, and the product by c/a. Examples are provided to illustrate the calculation of these values, and the results are confirmed by solving the equations and checking that the sum and product of the solutions match the calculated values.
Mindmap
Keywords
💡Complex numbers
💡Standard form
💡Graphing complex numbers
💡Absolute value
💡Special triangles
💡Simplifying square roots
💡Imaginary unit 'i'
💡Arithmetic operations
💡Conjugate
💡Quadratic equations
Highlights
Introduction to complex numbers and their standard form representation as a + bi.
Explanation of graphing complex numbers on the real and imaginary axes.
Calculating the absolute value of a complex number using the formula √(a² + b²).
Demonstration of graphing and finding the absolute value of 3 + 4i.
Use of special right triangles to simplify calculations of absolute values.
Simplification of square roots of negative numbers resulting in imaginary numbers.
Process for adding and subtracting complex numbers by combining like terms.
Multiplication of complex numbers using the FOIL method and combining like terms.
Division of complex numbers by multiplying the numerator and denominator by the conjugate of the denominator.
Solving quadratic equations with complex solutions using factoring and the quadratic formula.
Explanation of how to find the sum and product of the roots of a quadratic equation.
Solving for x in equations involving complex numbers by equating real and imaginary components.
Method to construct a quadratic equation from given complex solutions.
Understanding the properties of i raised to various powers and their results.
Techniques for simplifying complex fractions by separating them into real and imaginary parts.
Approach to divide a complex number by an imaginary number by multiplying by i.
Strategy to handle complex equations with multiple variables by isolating real and imaginary parts.
Final thoughts on the importance of mastering complex number operations for various mathematical applications.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: