Refraction of Light
TLDRIn this AP Physics essentials video, Mr. Andersen explores the concept of light refraction, illustrating how light bends when transitioning between different media due to changes in speed. He introduces Snell's Law to quantify refraction, using variables like the angle of incidence, angle of refraction, and indices of refraction. The video also covers total internal reflection and the critical angle, providing an analogy of a marching band to help visualize the bending of light. Finally, Mr. Andersen demonstrates the application of Snell's Law through a PHET simulation, guiding viewers to predict refraction angles and understand the principles behind light's behavior as it enters various mediums.
Takeaways
- 🌟 Refraction is the bending of light as it moves between different media due to changes in speed.
- 🔍 Light does not bend when it travels through a uniform medium like air, but it does when it hits a surface like glass.
- 📐 When light enters a medium at a straight angle (normal incidence), there is no refraction.
- 💡 Snell’s Law quantifies refraction with the formula n1 * sin(angle of incidence) = n2 * sin(angle of refraction), where n1 and n2 are the indices of refraction of the two media.
- 📉 The index of refraction is related to the speed of light in a medium; a higher index means slower speed.
- 🔄 As light enters a medium where it travels slower, it bends towards the normal (perpendicular line).
- 🔄 Conversely, when light enters a faster medium, it bends away from the normal.
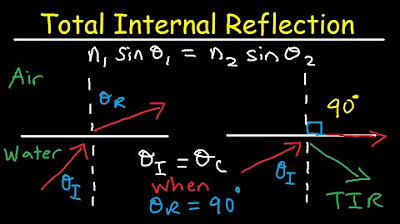
- 🔄 The critical angle is the angle of incidence beyond which light is no longer refracted but totally internally reflected.
- 👣 The marching band analogy helps visualize refraction by imagining people maintaining their spacing as they speed up or slow down.
- 🔮 Total internal reflection occurs when light is reflected entirely back into the original medium at an angle greater than the critical angle.
- 🔧 Snell’s Law can be used to predict the angle of refraction given the indices of refraction and the angle of incidence, and vice versa.
Q & A
What is refraction of light?
-Refraction of light is the bending of light as it passes from one medium to another, changing speed and direction due to the different optical densities of the media.
Why does light bend when it hits a glass surface?
-Light bends when it hits a glass surface because the speed of light changes as it moves from air (a less dense medium) to glass (a denser medium), causing the light to change direction.
What is the normal line in the context of refraction?
-The normal line is an imaginary line perpendicular to the surface of the medium at the point where the light ray enters, and it is used as a reference for measuring the angles of incidence and refraction.
How does Snell’s Law help in quantifying refraction?
-Snell’s Law allows us to calculate the angle of refraction when light passes from one medium to another by relating the indices of refraction of the two media and the angles of incidence and refraction.
What are the variables used in Snell’s Law formula?
-In Snell’s Law formula, the variables are the angle of incidence (measured from the normal), the angle of refraction (also measured from the normal), and the indices of refraction (n1 and n2) of the two media involved.
What is meant by the critical angle in refraction?
-The critical angle is the angle of incidence at which the angle of refraction becomes 90 degrees, causing the light to travel along the boundary between the two media, leading to total internal reflection.
What is total internal reflection?
-Total internal reflection occurs when light, moving from a denser to a less dense medium, hits the boundary at an angle greater than the critical angle, causing all the light to be reflected back into the denser medium with no transmission.
What is the marching band analogy used to explain refraction?
-The marching band analogy compares the behavior of light to a group of marching band members trying to maintain equal spacing as they move from a fast medium (like a parking lot) to a slow medium (like sand). As they enter the slower medium, they bend towards the normal, illustrating how light bends towards the normal when it slows down.
How does the speed of light change as it moves through different media?
-The speed of light decreases as it moves from a less dense medium (like air) to a denser medium (like glass or water), causing the light to bend towards the normal line.
How can Snell’s Law be used to predict the behavior of light when it hits a sphere?
-Snell’s Law can be used to predict whether the light will bend towards or away from the normal when it hits a sphere, depending on the indices of refraction of the media and the angle of incidence. This can help explain phenomena like the inversion of light seen through a glass sphere.
What is the relationship between the index of refraction and the speed of light in a medium?
-The index of refraction is inversely related to the speed of light in a medium. A higher index of refraction indicates a slower speed of light in that medium.
Outlines
🌟 Introduction to Light Refraction
Mr. Andersen introduces the concept of light refraction, explaining how light bends when it moves from one medium to another with a different speed. He illustrates this with a laser light passing from air into glass, where bending occurs due to the change in speed. The video uses an analogy of a marching band to help understand the concept, where the band members represent light waves that slow down when entering a denser medium, causing the light to bend towards the normal line. The importance of the angle of incidence and the angle of refraction is highlighted, with the normal line being perpendicular to the boundary between the two media.
📚 Snell's Law and Refraction Analysis
The script delves into Snell's Law, a formula used to calculate the refraction of light. It defines variables such as the angle of incidence, angle of refraction, and the indices of refraction (n1 and n2) for the two media involved. The relationship between the indices of refraction and the speed of light in the media is explained, with the index of refraction being inversely proportional to the speed of light in the medium. The concept of the critical angle is introduced, where beyond this angle, light is no longer refracted but undergoes total internal reflection. The marching band analogy is revisited to demonstrate how the direction of light bending can be predicted when light enters a medium at an angle.
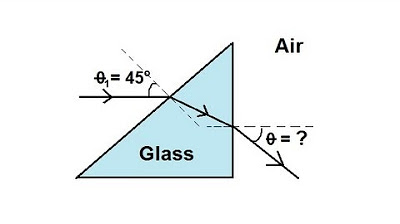
🔍 Practical Applications and PHET Simulations
The video script explores practical applications of refraction, such as the behavior of light passing through a prism and the formation of images through a sphere. It uses a PHET simulation to visually demonstrate refraction and to practice using Snell's Law to calculate the angle of refraction given the angle of incidence and the indices of refraction of the media. The script guides viewers through solving for the unknowns in Snell's Law, such as determining the angle of refraction when the angle of incidence and the indices of refraction are known. It also discusses the phenomenon of total internal reflection, which occurs when light is incident at an angle greater than the critical angle, causing it to be reflected entirely back into the original medium.
Mindmap
Keywords
💡Refraction
💡Reflection
💡Medium
💡Normal
💡Angle of Incidence
💡Angle of Refraction
💡Snell’s Law
💡Index of Refraction
💡Critical Angle
💡Total Internal Reflection
💡Marching Band Analogy
Highlights
Introduction to the concept of refraction, which is the bending of light.
Demonstration of light bending when it hits a glass surface compared to moving through air.
Explanation of refraction as a change in the speed of light moving through different media.
Illustration of light traveling straight through a flat glass surface without refraction.
Discussion on light being reflected, absorbed, or transmitted when moving between media.
Clarification that the video focuses on transmitted light and the absence of refraction at a straight angle.
Description of light bending towards the normal when it slows down entering a new medium.
Introduction of Snell’s Law as a method to quantify the bending of light.
Definition of angle of incidence and angle of refraction in the context of Snell’s Law.
Explanation of the index of refraction (n1 and n2) and its role in Snell’s Law formula.
Introduction of the concept of critical angle and total internal reflection.
Use of a marching band analogy to help understand the direction of light bending.
Application of the marching band analogy to explain light entering a faster medium.
Demonstration of refraction using a prism and the marching band analogy.
Use of a PHET simulation to visualize refraction and the effect of changing angles.
Explanation of how refraction inverts the image seen through a sphere.
Application of Snell’s Law in a PHET simulation to calculate the angle of refraction.
Demonstration of total internal reflection and its occurrence at the critical angle.
Real-world example of total internal reflection seen in the reflection of a sea turtle.
Summary of the ability to describe models of light traveling through boundaries using Snell’s Law.
Emphasis on the marching band analogy as a tool for predicting refraction.
Transcripts
Browse More Related Video
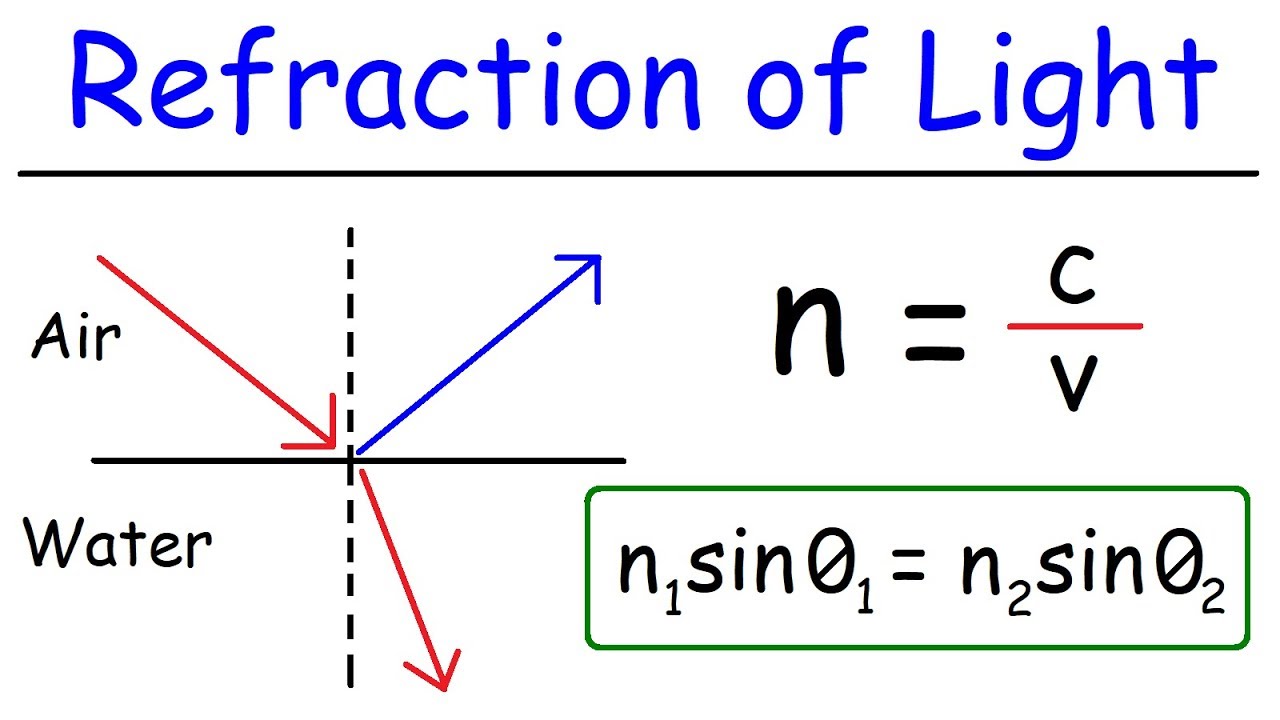
Refraction of Light

Snell's Law & Index of Refraction Practice Problems - Physics
Total Internal Reflection of Light and Critical Angle of Refraction Physics

Refraction and Snell's law | Geometric optics | Physics | Khan Academy

Snell's Law & Index of Refraction - Wavelength, Frequency and Speed of Light
Physics 52 Refraction and Snell's Law (3 of 11) Light Ray Through A Prism
5.0 / 5 (0 votes)
Thanks for rating: