Calculus - Slope, Concavity, Max, Min, and Inflection Point (1 of 4) Trig Function
TLDRThis lecture online delves into the concepts of slope, concavity, and points of maximum, minimum, and inflection of a function using derivatives. The presenter explains the slope as the steepness of a function and uses a graphic to illustrate positive and negative slopes. Concavity is described as the change in slope, with examples of concave up and down regions. Maximum and minimum points are identified where the slope is zero, and inflection points are distinguished as places where the slope changes from increasing to decreasing or vice versa. The lecture aims to guide viewers on how to evaluate a curve for these critical points, highlighting the importance of understanding the differences between horizontal and near-vertical inflection points.
Takeaways
- 📈 The slope of a function represents its steepness at particular points, with positive slopes indicating an increase to the right and negative slopes indicating a decrease.
- 📊 Concavity describes how the slope of a function changes, with concave down sections indicating a decrease in slope and concave up sections indicating an increase in slope.
- 🔍 To find local maximum or minimum points on a graph, look for points where the slope (first derivative) is zero.
- 🔄 Inflection points are where the concavity of a curve changes, either from concave up to concave down or vice versa.
- 📍 There are two types of inflection points: horizontal, where the slope is zero, and non-horizontal, where the slope does not go to zero but the concavity changes.
- 🤔 To determine whether a zero slope indicates a maximum, minimum, or inflection point, additional testing is required.
- 📉 A curve is considered concave down where the slope decreases from large to small or negative, and concave up where the slope increases from negative to positive.
- 📈 The first derivative of a function gives the slope at any point, which is crucial for identifying maximum, minimum, and inflection points.
- 📚 The second derivative can be used to determine concavity, with positive values indicating concave up and negative values indicating concave down.
- 🔑 Understanding the concepts of slope, concavity, and inflection points is essential for analyzing the behavior of functions and their graphs.
- 📚 The lecture aims to teach how to use derivatives to find slopes, maximum, minimum, and inflection points, which are fundamental in evaluating the properties of a curve.
Q & A
What is the slope of a function and how is it represented on a graph?
-The slope of a function represents the steepness or incline of the function at particular points. On a graph, a positive slope indicates that the function rises as you move to the right, while a negative slope indicates that it falls.
How can you determine if a function is concave up or concave down?
-Concavity indicates how the slope of a function is changing. If the slope decreases from positive to negative, the function is said to be concave down. Conversely, if the slope increases from negative to positive, the function is concave up.
What is the significance of a point on a graph where the slope is zero?
-A point on a graph where the slope is zero is significant because it can indicate either a maximum or a minimum point on the curve. However, it could also be an inflection point if the concavity changes without the slope reaching zero.
What is the difference between a local maximum and a local minimum point on a graph?
-A local maximum point is where the function reaches a peak within a certain area, and the slope is zero at that point. A local minimum point is where the function reaches a trough, and similarly, the slope is zero. These are local because they may not be the highest or lowest points on the entire graph.
Can an inflection point occur at a point where the slope is not zero?
-Yes, an inflection point can occur where the slope is not zero. This type of inflection point is characterized by a change in concavity without the slope reaching zero, and it is often referred to as a 'near vertical' inflection point.
How can you identify an inflection point on a graph?
-An inflection point can be identified by observing a change in the concavity of the curve, either from concave up to concave down or vice versa. It is not necessarily indicated by a zero slope, and further analysis is required to distinguish it from a maximum or minimum point.
What is the relationship between the second derivative and concavity?
-The second derivative of a function is directly related to its concavity. A positive second derivative indicates that the function is concave up, while a negative second derivative indicates that it is concave down.
How do you find the points where the slope of a function is positive or negative?
-To find where the slope is positive or negative, you would analyze the first derivative of the function. If the first derivative is positive, the slope is increasing, and if it is negative, the slope is decreasing.
What is the purpose of using derivatives to analyze a function's graph?
-Derivatives are used to analyze a function's graph to determine key features such as the slope at various points, the locations of maximum and minimum points, and the inflection points where the concavity changes.
Can you find all maximum, minimum, and inflection points by just looking at the graph?
-While you can visually estimate the locations of maximum, minimum, and inflection points on a graph, using derivatives provides a precise mathematical method to find and confirm these points.
Why is it important to distinguish between different types of inflection points?
-Differentiating between types of inflection points is important because it provides a deeper understanding of the function's behavior. Horizontal inflection points occur where the slope is zero, while near-vertical inflection points occur where the concavity changes without the slope being zero.
Outlines
📈 Introduction to Slope, Concavity, and Inflection Points
The script introduces the concepts of slope, concavity, and inflection points of a function using derivatives. It explains that the slope represents the steepness of a function at specific points, which can be positive or negative, and is determined by the first derivative of the function. Concavity describes how the slope changes, with concave down indicating a decreasing slope and concave up indicating an increasing slope. Inflection points are where the concavity changes, either from concave down to up or vice versa. The script also distinguishes between horizontal and non-horizontal inflection points, noting that not all inflection points are where the slope is zero, and additional tests are needed to determine the nature of these points. The importance of identifying maximum and minimum points, as well as understanding where the slope is positive or negative and the curve is concave up or down, is highlighted.
🔍 Locating Inflection Points and Understanding Their Types
This paragraph delves deeper into the identification of inflection points, which are found where the curve transitions from concave down to concave up or from concave up to concave down. It clarifies that not all inflection points are vertical or horizontal, and further examination is required to determine their specific type. The paragraph also discusses the presence of additional inflection points, suggesting that for every change in concavity, there is an inflection point. The script emphasizes the need to understand the different types of inflection points and their implications on the curve's behavior. It sets the stage for applying these concepts through examples to find various points on a graph, including maximum, minimum, and inflection points.
Mindmap
Keywords
💡Slope
💡Concavity
💡Derivative
💡Maximum Point
💡Minimum Point
💡Inflection Point
💡Positive Slope
💡Negative Slope
💡Concave Up
💡Concave Down
Highlights
Introduction to slope, concavity, maximum/minimum points, and inflection points using derivatives.
Understanding slope as the steepness of a function at particular places.
Positive slope indicates a rising function from left to right.
Negative slope indicates a falling function from left to right.
Concavity explains how the slope of a curve changes.
Concave down indicates a slope that goes from large to small to negative.
Concave up indicates a slope that increases from negative to positive.
Maximum points are where the slope is zero and positive before and after.
Minimum points are where the slope is zero and negative before and after.
Inflection points are where the concavity changes without the slope being zero.
Horizontal inflection points occur where the slope is zero but not a max or min.
Non-horizontal inflection points are where concavity changes without a zero slope.
Differentiating between types of inflection points requires further testing.
Finding where the slope is positive or negative is crucial for curve analysis.
Identifying regions of concave up and concave down is essential for curve evaluation.
Inflection points are found where the curve transitions from concave down to up or vice versa.
The importance of distinguishing between horizontal and near-vertical inflection points.
The lecture aims to provide techniques for finding maximum, minimum, and inflection points on a graph.
Transcripts
Browse More Related Video

MATH1325 Lecture 10 2 - Concavity & Points of Inflection

Inflection points (graphical) | AP Calculus AB | Khan Academy

What the Second Derivative Tells You about a Graph

Calculus 1 Lecture 3.4: The Second Derivative Test for Concavity of Functions
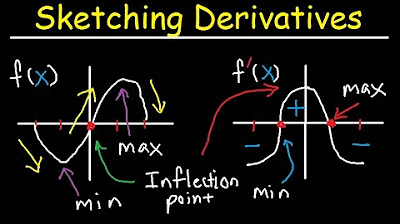
Sketching Derivatives From Parent Functions - f f' f'' Graphs - f(x), Calculus

Business Calculus - Math 1329 - Section 3.2 - Concavity and Points of Inflection
5.0 / 5 (0 votes)
Thanks for rating: