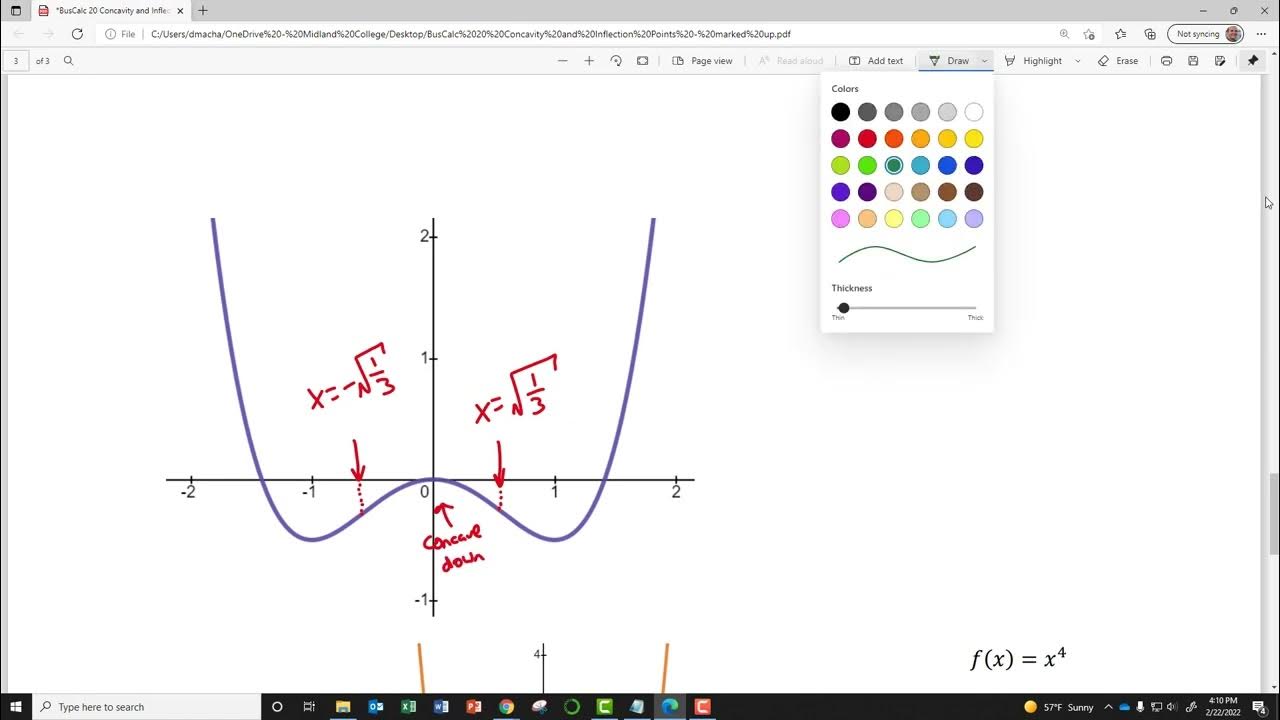
Learn how to determine concavity and point of inflection AP style
TLDRThe video script is an educational walkthrough on how to determine the concavity of a function using calculus. The presenter instructs viewers to first find the first and second derivatives of the function. By setting the second derivative to zero, potential points of inflection are identified as x = 0 and x = 2. To assess the concavity, the presenter uses test points within the intervals of negative infinity to zero, zero to two, and two to positive infinity. The sign of the second derivative at these test points indicates whether the function is concave up or down in each interval. The presenter emphasizes the importance of understanding the change in concavity from positive to negative, which signifies a point of inflection. The script concludes with a concise explanation of how to label points of inflection by describing the change in concavity. The presenter encourages viewers to apply these methods efficiently, highlighting the mathematical reasoning behind each step.
Takeaways
- 📚 The script is about understanding concavity by analyzing a function's second derivative.
- 🔍 The process involves taking the first and second derivatives of the function to find points of inflection.
- 🔢 Setting the second derivative equal to zero helps identify possible points of inflection.
- 📝 Factoring techniques are used to solve for x, which yields potential inflection points.
- 📉 The script discusses intervals from negative infinity to zero, zero to two, and two to positive infinity to analyze concavity.
- 📍 Test points such as x equals negative 1, 1, and 3 are chosen to determine the sign of the second derivative.
- 🔎 Squaring a negative number results in a positive value, which is used to quickly assess the concavity without full calculations.
- ✍️ The script emphasizes the importance of writing the conclusions clearly, stating whether the function is concave up or down on each interval.
- 📌 Points of inflection are labeled by describing the change in concavity from positive to negative or vice versa.
- 📝 The script provides a method to write two statements for each point of inflection to indicate the change from concave up to down or down to up.
- 🚀 The overall message is that understanding and identifying concavity can be done quickly and efficiently with the right approach.
Q & A
What is the main topic discussed in the script?
-The main topic discussed in the script is the concept of concavity and how to determine it using the first and second derivatives of a function.
What is the first step in determining concavity according to the script?
-The first step in determining concavity is to take the first derivative of the function.
Why is the second derivative important in the context of concavity?
-The second derivative is important because it helps identify points of inflection and determine whether the function is concave up or concave down.
How does one identify possible points of inflection?
-Possible points of inflection are identified by setting the second derivative equal to zero and solving for x.
What factoring technique is mentioned in the script to find possible points of inflection?
-The script mentions factoring out a 12x to find possible points of inflection, which results in the factors x - 2.
What are the possible inflection points mentioned in the script?
-The possible inflection points mentioned in the script are x equals 0 and x equals 2.
How does one determine the intervals to test for concavity?
-The intervals to test for concavity are determined by considering the possible points of inflection and dividing the number line into segments between these points.
What test points are chosen in the script to determine the concavity of the function?
-The test points chosen in the script are x equals negative 1, x equals 1, and x equals 3.
How does the script suggest evaluating the concavity without doing the full calculations?
-The script suggests using mental math and understanding the properties of squaring negative numbers to quickly determine if the second derivative will be positive or negative.
What is the conclusion drawn from the concavity analysis in the script?
-The conclusion is that the function is concave up on the interval from negative infinity to zero, concave down on the interval from zero to two, and concave up again on the interval from two to infinity.
How should one describe a point of inflection according to the script?
-According to the script, a point of inflection should be described by stating that the second derivative is changing from positive to negative or vice versa, indicating a change in concavity.
Outlines
📚 Understanding Concavity and Points of Inflection
This paragraph discusses the process of determining concavity and points of inflection in a mathematical function. The speaker explains the importance of examining the second derivative and setting it equal to zero to find possible points of inflection. Factoring techniques are used to solve for x, resulting in x = 0 and x = 2 as potential inflection points. The speaker then describes how to analyze intervals and select test points to determine the sign of the second derivative, which indicates whether the function is concave up or down. The paragraph concludes with a brief mention of the writing portion, where the speaker would state the intervals of concavity without fully evaluating the test points.
📝 Correctly Labeling Points of Inflection
The second paragraph focuses on the correct way to label points of inflection. The speaker emphasizes that it's not enough to simply state that the second derivative changes signs; one must also describe the change in concavity. The explanation involves identifying when the second derivative changes from positive to negative or vice versa, which indicates a change in concavity. The speaker provides examples of how to articulate this change at x = 0 and x = 2, suggesting that these points are indeed points of inflection. The paragraph ends with a reminder that while these calculations can be done quickly, it's crucial to go the extra step in explanation to ensure clarity and accuracy.
Mindmap
Keywords
💡Concavity
💡Second Derivative
💡Points of Inflection
💡Factoring Techniques
💡Intervals
💡Test Points
💡Positive/Negative
💡Squaring
💡Writing Portion
💡Points of Inflection Labeling
Highlights
Introduction to the concept of concavity and the importance of the second derivative.
Explanation of the process to find points of inflection by setting the second derivative equal to zero.
Technique of factoring to solve for x to identify possible inflection points.
Identification of x = 0 and x = 2 as potential inflection points.
Discussion on analyzing intervals from negative infinity to zero, zero to two, and two to positive infinity.
Selection of test points - x = -1, x = 1, and x = 3 for the second derivative.
Mental calculation approach for determining the sign of the second derivative without full evaluation.
Use of squaring negatives to always result in a positive value for the second derivative.
Explanation of how the sign of the second derivative changes with different test points.
Conclusion on concavity based on the sign of the second derivative at test points.
Writing portion of the process to articulate the concavity intervals.
Concave up interval from negative infinity to zero based on f''(-1) > 0.
Concave down interval from zero to two based on f''(1) < 0.
Concave up interval from two to positive infinity.
Identification of changes in concavity from up to down and vice versa.
Emphasis on the importance of describing the change in concavity at points of inflection.
Labeling x = 0 and x = 2 as points of inflection with a change from concave up to down and down to up.
Encouragement to perform calculations quickly and efficiently.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: