Geometry of the Second Derivative (1 of 4: Reviewing the Derivative)

Eddie Woo
11 Dec 201508:49
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video script, the presenter explores the concept of a cubic function without relying on calculus. They begin by factorizing a simple cubic equation, revealing it to be a perfect square, which helps visualize the function's graph with three roots. The presenter then introduces the derivative to find stationary points, differentiating the function to locate these points more precisely. They emphasize the importance of understanding the geometry of the function, especially the location and nature of stationary points, which are crucial for sketching the function's graph accurately. The script concludes with a practical demonstration of plotting the function, including its intercepts and stationary points, providing a hands-on approach to understanding cubic functions.

Takeaways
  • 📚 The lesson focuses on understanding a cubic function without calculus by factorizing it.
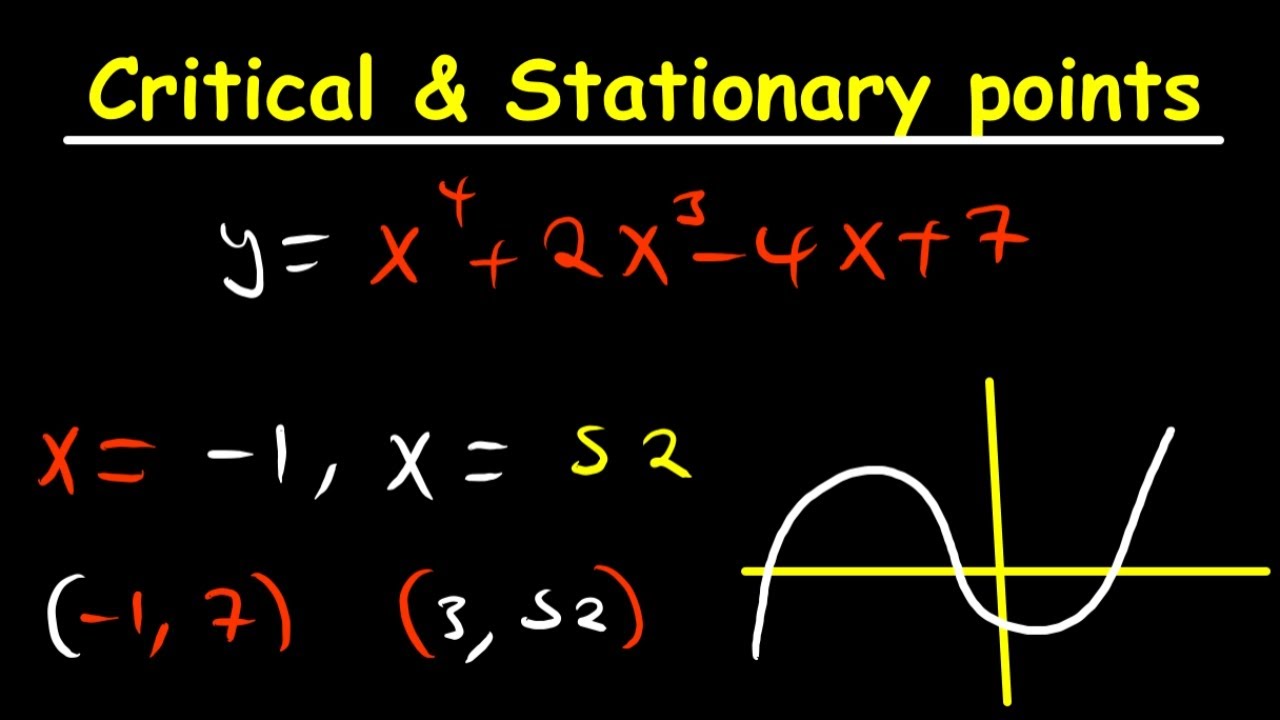
  • 🔍 The cubic function is factorized to x(x - 3)^2, which helps visualize the function's graph.
  • 📈 The function has three roots, one at x = 0 and two at x = 3, indicating a repeated root.
  • 📉 The leading coefficient of the cubic function is positive, suggesting the function will rise to positive infinity as x increases.
  • 🔑 The derivative of the function is computed to find stationary points, which are critical for understanding the function's geometry.
  • 📌 The first derivative is factorized to 3(x - 1)(x - 3), indicating stationary points at x = 1 and x = 3.
  • 📐 The stationary point at x = 3 is also a root, so its y-coordinate is known to be 0.
  • 📊 The y-coordinate for the stationary point at x = 1 is calculated to be 4, giving us the point (1,4).
  • 📝 The teacher emphasizes the importance of differentiating between zeros (where the function equals zero) and roots (where the graph intersects the x-axis).
  • 🖌️ The final graph of the function is drawn with the roots, stationary points, and the behavior at infinity in mind.
  • 📚 The lesson concludes with a practical approach to sketching the cubic function, highlighting the importance of the derivative in understanding its shape.
Q & A

  • What is the main topic of the video script?

    -The main topic of the video script is the analysis of a cubic function using factorization and differentiation to understand its geometry and behavior.

  • What type of function is being discussed in the script?

    -The script discusses a cubic function, which is a polynomial of degree three.

  • How does the instructor begin the analysis of the cubic function?

    -The instructor begins by factorizing the cubic function to understand its roots and general shape without using calculus.

  • What is the significance of factorizing the cubic function?

    -Factorizing the cubic function helps in identifying the roots and the general shape of the graph, which is essential for understanding the function's behavior.

  • How many roots does the instructor expect for the cubic function?

    -The instructor expects three roots for the cubic function, as cubic functions typically have three roots.

  • What is the role of the derivative in the analysis of the cubic function?

    -The derivative is used to find the stationary points of the function, which are the points where the function's rate of change is zero.

  • What is a stationary point in the context of this script?

    -A stationary point is a point on the graph of the function where the first derivative is zero, indicating a local maximum, minimum, or point of inflection.

  • How does the instructor find the x-values for the stationary points?

    -The instructor finds the x-values for the stationary points by setting the first derivative of the function equal to zero and solving for x.

  • What is the importance of the leading coefficient in determining the end behavior of a cubic function?

    -The leading coefficient determines whether the cubic function will rise or fall as x approaches positive or negative infinity. A positive leading coefficient means the function will rise to positive infinity as x increases.

  • How does the instructor use the second derivative to further analyze the cubic function?

    -The script does not explicitly mention the use of the second derivative. However, typically, the second derivative can be used to determine the concavity of the function and further analyze the nature of the stationary points.

  • What is the final step the instructor asks the viewers to do?

    -The final step the instructor asks the viewers to do is to draw the function, its first derivative, and possibly the second derivative on a set of axes to compare their geometric features.

Outlines
00:00
📚 Introduction to Factorizing a Cubic Function

The first paragraph introduces the concept of factorizing a cubic function without the need for calculus. The speaker begins by factorizing a simple cubic function, taking a factor of x out and leaving a quadratic that is a perfect square. This allows the speaker to predict the shape of the function and its roots. The speaker also discusses the importance of the leading coefficient, which is positive in this case, indicating the function will grow large for large positive x values. Additionally, the speaker touches on the concept of stationary points and how they can be found using derivatives.

05:00
📈 Sketching the Function and Finding Stationary Points

The second paragraph focuses on sketching the cubic function and determining its stationary points geometrically. The speaker emphasizes the importance of drawing the function on a set of axes, with the first derivative and second derivative to be considered later. The speaker identifies the x-intercepts and stationary points, noting that one of the stationary points coincides with a root of the function. By substituting x-values into the function, the speaker finds the y-coordinates for the stationary points, which allows for a more accurate sketch of the function. The speaker also uses a horizontal line as a guide to ensure the function behaves as expected when plotted.

Mindmap
Keywords
💡Cubic Function
A cubic function is a polynomial equation of degree three. It represents a curve that typically has a 'U' shape and can have up to three real roots or x-intercepts. In the video, the cubic function is the main subject of the investigation, and the speaker begins by factorizing it to understand its behavior without calculus. The function given is factorized to 'x(x - 3)^2', which helps in visualizing the graph and identifying the roots.
💡Factorization
Factorization is the process of breaking down a polynomial into a product of its factors. It is a fundamental concept in algebra used to simplify expressions and solve equations. In the context of the video, the cubic function is factorized to 'x(x - 3)^2', which simplifies the process of graphing the function and identifying its roots and behavior.
💡Roots
Roots of a function are the values of x for which the function equals zero. They represent the points where the graph of the function intersects the x-axis. The video discusses finding the roots of the cubic function, which are x = 0 and x = 3, by factorizing the function and setting it equal to zero.
💡Stationary Points
Stationary points, also known as critical points, are points on the graph of a function where its derivative is zero or undefined. They often indicate local maxima or minima. In the video, the speaker calculates the first derivative of the cubic function to find the x-values of the stationary points, which are essential for understanding the function's geometry.
💡Derivative
The derivative of a function measures the rate at which the function's output (or value) changes with respect to changes in its input. It is a fundamental concept in calculus and is used to analyze the behavior of functions. In the video, the first derivative of the cubic function is computed to find the stationary points, which helps in understanding the function's shape and turning points.
💡Perfect Square
A perfect square is a quadratic expression that can be written as the square of a binomial. It represents a parabola that opens either upwards or downwards. In the video, after factorizing the cubic function, the remaining quadratic expression '(x - 3)^2' is identified as a perfect square, which simplifies the graphing process and understanding of the function's shape.
💡Graphing
Graphing is the process of plotting the graph of a mathematical function or relation on a set of axes. It helps visualize the behavior of the function. The video focuses on graphing the cubic function by using its factorized form and the information about its roots and stationary points to sketch an accurate representation.
💡Leading Coefficient
The leading coefficient of a polynomial is the coefficient of its highest degree term. It determines the end behavior of the polynomial function. In the video, the leading coefficient of the cubic function is identified as positive (1), which indicates that the function will rise to positive infinity as x goes to positive or negative infinity.
💡Y-Intercept
The y-intercept is the point where a graph intersects the y-axis. It is found by setting x = 0 in the function's equation. In the video, the y-intercept of the cubic function is determined to be at the origin (0,0), which is a key point in graphing the function.
💡X-Intercept
The x-intercepts, also known as roots, are the points where a graph intersects the x-axis. They are found by setting the function equal to zero and solving for x. In the video, the x-intercepts of the cubic function are identified as x = 0 and x = 3, which are crucial for graphing the function and understanding its behavior.
💡Second Derivative
The second derivative of a function is the derivative of its first derivative. It provides information about the concavity of the function and can be used to determine the nature of stationary points, such as whether they are local maxima, minima, or points of inflection. In the video, the speaker mentions getting the second derivative, which would further analyze the function's behavior but does not compute it within the provided transcript.
Highlights

Introduction to a simple cubic function for investigation without calculus.

Factorization of the cubic function to understand its roots and behavior.

Identification of a perfect square within the factorized quadratic.

Graphical representation of the cubic function with three roots.

Understanding the leading coefficient's impact on the function's end behavior.

Differentiation of the cubic function to find stationary points.

Derivative's role in revealing more about the function's geometry.

Finding the x-values for stationary points using the first derivative.

Clarification on the difference between zeros and intercepts of a function.

Graphical placement of stationary points based on the first derivative.

Misunderstanding about the symmetry of cubic functions compared to parabolas.

Calculation of the y-coordinate for the stationary point at x=1.

Plotting the function with known intercepts and stationary points.

Importance of accurate plotting for understanding function behavior.

Use of horizontal lines as guides for stationary points in graphing.

Emphasis on the final graphical result rather than the working process.

Instruction to draw three sets of axes for comparing function, first, and second derivatives.

Transcripts

Browse More Related Video

Finding Stationary Points and Determining their Nature

Draw Cubic Graph Grade 12

Critical points & Stationary points

Sketching a Derivative from the Graph of a Function

AP Calc - Graphing f from f'

BusCalc 12.6 Sketching Functions/Graphs

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Cubic FunctionsFactorizationDerivativesGeometryRootsStationary PointsGraph SketchingMathematics EducationConceptual UnderstandingEducational ContentMath Tutorial