ILLUSTRATING CIRCULAR FUNCTIONS || PRE-CALCULUS

WOW MATH

11 Dec 202121:12

EducationalLearning

32 Likes 10 Comments

TLDRThis video lesson delves into the world of circular functions, illustrating how to calculate them using the Pythagorean theorem. The instructor explains the concepts of sine, cosine, tangent, cosecant, secant, and cotangent in the context of a right triangle formed by a point on the circle and the axes. The video provides formulas for each trigonometric function and demonstrates how to derive them when the radius is equal to one, known as the unit circle. It also covers the behavior of these functions in different quadrants and how to find the remaining functions when one is given. The lesson is enriched with examples, such as calculating the trigonometric functions for a point given in a specific quadrant. The video concludes with a reminder of the positive values of circular functions in each quadrant, making it an informative and practical guide for those studying trigonometry.

Takeaways

📚 The video lesson is about illustrating circular functions using the unit circle and the Pythagorean theorem.
📐 It explains that r represents the distance of point P from the origin, and x and y are the horizontal and vertical distances from the y-axis and x-axis, respectively.
📈 The sine, cosine, and tangent functions are introduced as ratios of sides in a right triangle where the hypotenuse is the radius r.
🔄 The reciprocal functions cosecant (csc), secant (sec), and cotangent (cot) are also explained, relating them to the primary trigonometric functions.
🌐 The unit circle is highlighted where the radius is equal to one, simplifying the formulas for the circular functions.
📈 Special angles in the first quadrant (30, 45, 60, and 90 degrees) are used to derive the values of circular functions.
📊 The script provides examples of how to find the values of all circular functions given the sine or cosine of an angle in a specific quadrant.
📝 It discusses the signs of the circular functions in different quadrants, noting that all functions are positive in the first quadrant and explains which are positive in others.
🔢 The video includes step-by-step calculations for finding the remaining circular functions when given one function value, using algebraic manipulation.
📚 The importance of understanding the quadrant in which the angle lies is emphasized for determining the signs of the trigonometric function values.
👨‍🏫 The instructor provides a comprehensive guide for learning about circular functions, encouraging viewers to like, subscribe, and follow for more tutorials.

Q & A

What is the main topic of the video lesson?
-The main topic of the video lesson is illustrating circular functions, which are trigonometric functions defined in terms of a circle's geometry.
What is the role of the Pythagorean theorem in the context of circular functions?
-The Pythagorean theorem is used to relate the radius 'r', and the horizontal and vertical distances 'x' and 'y' from the origin to a point 'P', forming a right triangle with the hypotenuse as the radius of the circle.
What are the basic trigonometric functions discussed in the video?
-The basic trigonometric functions discussed are sine (sin), cosine (cos), and tangent (tan).
What are the reciprocal trigonometric functions and how are they related to the basic functions?
-The reciprocal trigonometric functions are cosecant (csc), secant (sec), and cotangent (cot). They are the reciprocals of sine, cosine, and tangent, respectively.
How is the sine of an angle defined in terms of the circle's geometry?
-The sine of an angle (sin θ) is defined as the ratio of the length of the side opposite the angle (y) to the length of the hypotenuse (r), which is the radius of the circle.
What is the definition of the cosine of an angle in the context of circular functions?
-The cosine of an angle (cos θ) is defined as the ratio of the length of the side adjacent to the angle (x) to the length of the hypotenuse (r), the radius of the circle.
How is the tangent of an angle related to the sides of the right triangle formed by the circle's radius and coordinates?
-The tangent of an angle (tan θ) is the ratio of the length of the side opposite the angle (y) to the length of the side adjacent to the angle (x).
What happens to the values of the circular functions when the radius of the circle is equal to one?
-When the radius of the circle is equal to one, the circular functions can be simplified to the coordinates of the point on the circle, with sine and cosine being equal to the y and x coordinates, respectively.
In which quadrants are all the circular functions positive?
-All the circular functions are positive in the first quadrant.
How can you find the remaining circular functions if you are given the sine of an angle and it is in the first quadrant?
-If you know the sine of an angle and it is in the first quadrant, you can find the radius 'r' using the Pythagorean theorem, and then calculate the remaining functions using the definitions of sine, cosine, tangent, cosecant, secant, and cotangent.
What are the signs of the circular functions in the second quadrant?
-In the second quadrant, sine and cosecant are positive, while cosine, secant, tangent, and cotangent are negative.
How can you determine the trigonometric functions of an angle if you are given a point on the terminal side of the angle?
-Given a point on the terminal side of an angle, you can determine the trigonometric functions by calculating the radius 'r' using the Pythagorean theorem and then applying the definitions of the trigonometric functions using the coordinates of the point and the radius.
What is the significance of the special angles in trigonometry?
-Special angles, such as 30, 45, and 60 degrees, are significant because they divide the first quadrant into equal parts and have known values for the trigonometric functions, making calculations easier.
What is the process for finding the trigonometric functions if you are given the cosine and cotangent of an angle?
-If you are given the cosine and cotangent of an angle, you can use the definitions of these functions to find the x and y coordinates, calculate the radius 'r', and then determine the remaining trigonometric functions.

Outlines

00:00

📚 Introduction to Circular Functions

This paragraph introduces the concept of circular functions, specifically focusing on the unit circle and the trigonometric relationships between the radius, x-coordinate, and y-coordinate. The Pythagorean theorem is used to express the radius in terms of x and y, forming the basis for understanding sine, cosine, and tangent. The paragraph explains these primary trigonometric functions in the context of a right triangle with the hypotenuse as the radius of the circle.

05:00

🔍 Exploring Reciprocal Trigonometric Functions

The second paragraph delves into the reciprocal functions of sine, cosine, and tangent, which are cosecant (csc), secant (sec), and cotangent (cot) respectively. It explains how these functions are derived from the primary trigonometric functions and provides the formulas for each. The paragraph also discusses the behavior of these functions in different quadrants, noting which are positive and which are negative based on the angle's location.

10:02

📐 Calculating Trigonometric Functions Given an Angle

In this paragraph, the script provides a step-by-step example of how to calculate the values of trigonometric functions when given an angle and its sine value in the first quadrant. It demonstrates the process of finding the radius, x-coordinate, and subsequently the remaining trigonometric functions using the Pythagorean theorem and the definitions of sine, cosine, and tangent.

15:03

📉 Determining Trigonometric Functions for a Point in a Specific Quadrant

The fourth paragraph presents an example of finding the trigonometric functions for a point located in the third quadrant. It explains how to calculate the radius and then use it to find the values of sine, cosine, tangent, and their reciprocals, taking into account the signs of the coordinates in the third quadrant.

20:13

🎓 Applying Trigonometric Functions in Various Quadrants

The final paragraph offers a comprehensive example that includes determining the trigonometric functions for an angle where the cosine and cotangent are given specific values, indicating the angle's quadrant. It walks through the process of finding the sine, tangent, and their reciprocals, as well as the signs of these functions in different quadrants, reinforcing the understanding of trigonometric functions across the coordinate plane.

👋 Conclusion and Call to Action

The concluding paragraph thanks viewers for watching the video, encouraging them to like, subscribe, and hit the bell for more tutorial videos. It emphasizes the educational value of the content and the channel's role in guiding viewers through their learning journey.

Mindmap

Keywords

💡Circular Functions

Circular functions are trigonometric functions that relate to the coordinates of a point on the unit circle. In the video, they include sine, cosine, tangent, cosecant, secant, and cotangent. These functions are essential for understanding relationships between angles and distances in trigonometry.

💡Pythagorean Theorem

The Pythagorean Theorem is a fundamental principle in geometry stating that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. In the video, this theorem is used to derive the relationship between x, y, and r (the hypotenuse or radius).

💡Sine Theta

Sine theta (sin θ) is a circular function defined as the ratio of the opposite side to the hypotenuse in a right triangle. The video explains that for a given angle theta, sin θ = y/r, where y is the vertical distance from the x-axis and r is the radius.

💡Cosine Theta

Cosine theta (cos θ) is a circular function defined as the ratio of the adjacent side to the hypotenuse in a right triangle. In the video, it is explained as cos θ = x/r, where x is the horizontal distance from the y-axis and r is the radius.

💡Tangent Theta

Tangent theta (tan θ) is a circular function defined as the ratio of the opposite side to the adjacent side in a right triangle. The video describes it as tan θ = y/x, providing an example where this relationship is used to solve for unknown sides.

💡Unit Circle

The unit circle is a circle with a radius of one, centered at the origin of the coordinate system. In the video, the unit circle is used to simplify the definitions of trigonometric functions, showing that sin θ = y and cos θ = x when the radius is one.

💡Reciprocal Functions

Reciprocal functions in trigonometry include cosecant (csc θ), secant (sec θ), and cotangent (cot θ). These are the reciprocals of sine, cosine, and tangent respectively. The video details how these functions are derived and used, such as csc θ = 1/sin θ.

💡Quadrants

Quadrants refer to the four sections of the coordinate plane divided by the x and y axes. The video discusses the properties of trigonometric functions in each quadrant, noting which functions are positive in each (e.g., sine and cosecant are positive in Quadrant II).

💡Hypotenuse

The hypotenuse is the longest side of a right triangle, opposite the right angle. In the video, it is denoted as r and is essential in defining the trigonometric functions sine, cosine, and secant, among others.

💡Special Angles

Special angles are specific angles like 30°, 45°, 60°, and 90° that have well-known sine, cosine, and tangent values. The video references these angles to show how trigonometric functions can be applied and calculated easily for these common angles.

Highlights

Introduction to illustrating circular functions using the Pythagorean theorem.

Explanation of the trigonometric circle setup with point P, origin O, and axes distances x and y.

Definition of sine theta as the ratio of the opposite side to the hypotenuse in a right triangle.

Cosine theta defined as the ratio of the adjacent side to the hypotenuse.

Tangent theta introduced as the ratio of the opposite side to the adjacent side.

Cosecant theta explained as the reciprocal of sine theta.

Secant theta as the reciprocal of cosine theta.

Cotangent theta defined as the reciprocal of tangent theta.

Chord tangent, denoted as cot theta, explained as adjacent over hypotenuse.

Unit circle concept where the radius is equal to one.

Derivation of circular functions for the unit circle, simplifying the formulas.

Sign conventions of circular functions in different quadrants.

Example problem solving using given sine theta value in quadrant one.

Calculation of remaining circular functions given sine theta in an example.

Finding trigonometric functions for a point in quadrant three.

Solving for sine, cosine, and tangent given a point's coordinates.

Using given values to find cosecant, secant, and cotangent.

Example of determining trigonometric functions when cosine and cotangent are given as positive.

Final problem involving finding all trigonometric functions from given cosine and cotangent values.

Conclusion and invitation to like, subscribe, and follow for more educational content.

Transcripts

Browse More Related Video

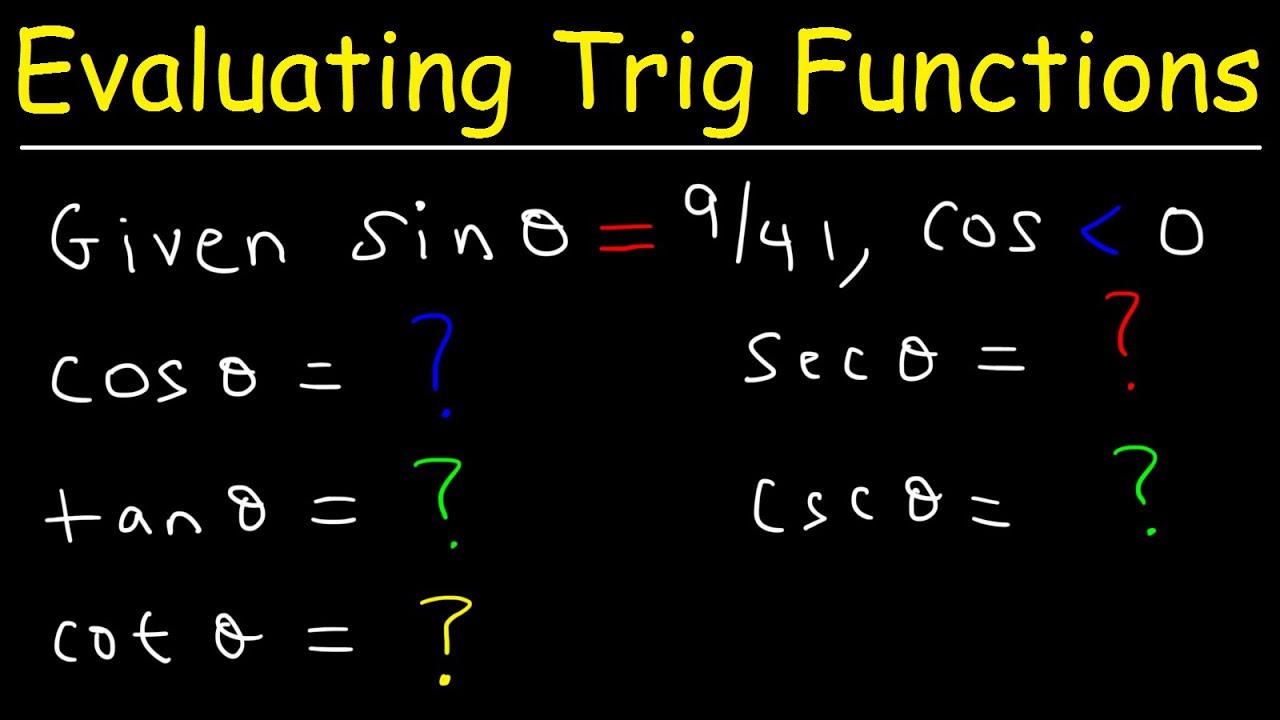
How To Find The Exact Value of the Five Remaining Trigonometric Functions

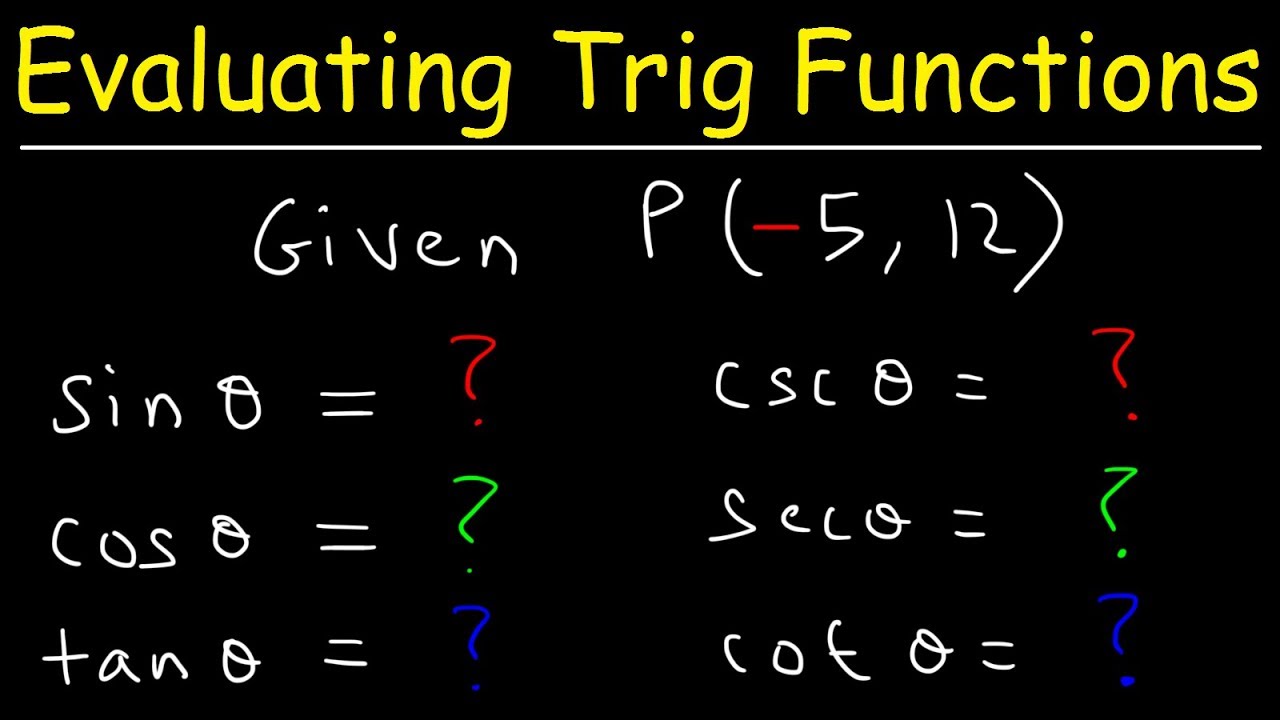
Evaluating Trigonometric Functions Given a Point on the Terminal Side - Trigonometry

Trigonometric Functions and the Unit Circle (Precalculus - Trigonometry 6)

An Indepth Look at Using Inverse Trig Functions (Precalculus - Trigonometry 21)

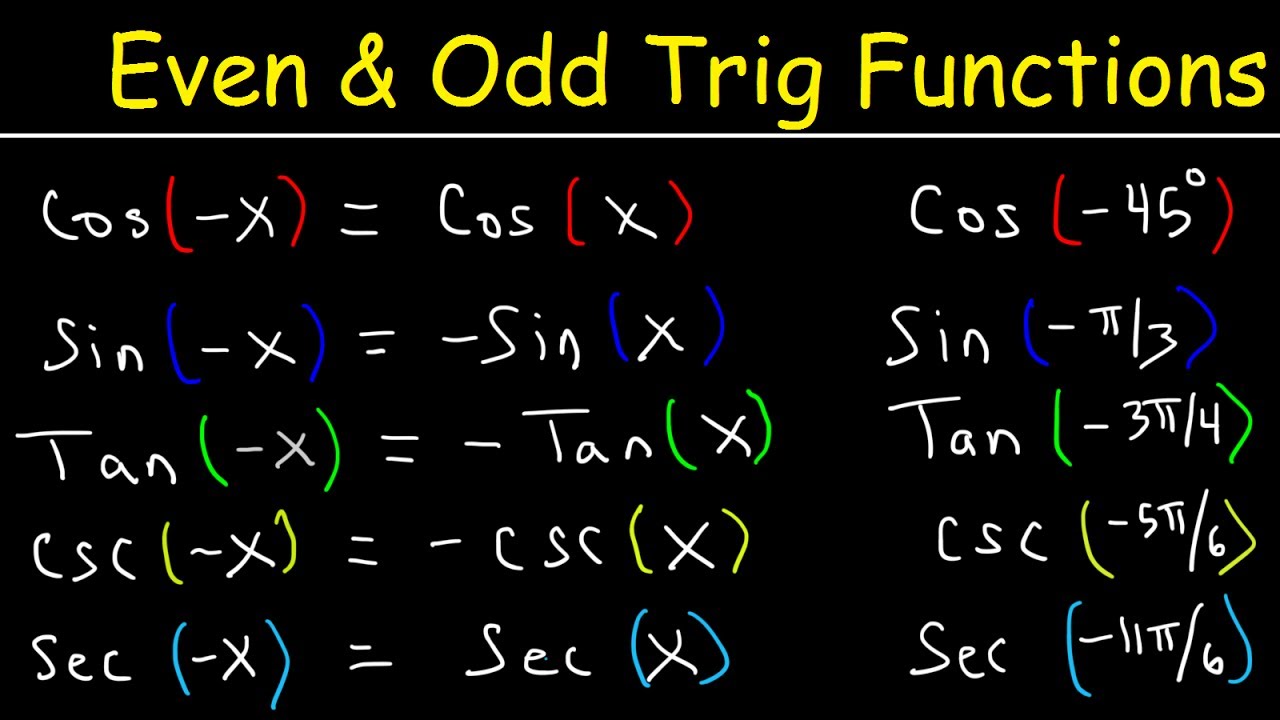
Even and Odd Trigonometric Functions & Identities - Evaluating Sine, Cosine, & Tangent

Trig - 0.7 Trig Functions of Any Angle

Related Tags

Trigonometry Circular Functions Sine Theta Cosine Theta Tangent Theta Cosecant Theta Secant Theta Cotangent Theta Pythagorean Theorem Trigonometric Ratios Math Tutorial Educational Content