How To Find The Exact Value of the Five Remaining Trigonometric Functions

The Organic Chemistry Tutor
14 Oct 201707:11
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript details a mathematical exploration of trigonometric functions given specific conditions. It begins by determining the quadrant of a triangle where sine is positive and cosine is negative, leading to quadrant two. The sides of the triangle are used to find cosine, tangent, secant, cosecant, and cotangent. The process is repeated for different scenarios: when cosine is positive and tangent is negative (quadrant four), and when secant is negative and sine is positive (quadrant two). Each case involves using the Pythagorean theorem to find missing sides and then calculating the trigonometric functions based on those sides. The summary highlights the step-by-step approach to solving trigonometric problems by identifying the correct quadrant and applying mathematical principles to find the values of the trigonometric functions.

Takeaways
  • 📚 The script discusses finding the values of trigonometric functions given certain conditions.
  • 📐 The first scenario involves sine(theta) = 9/41 and cosine(theta) < 0, indicating the triangle is in quadrant two.
  • 📏 The hypotenuse is determined to be 41, and the opposite side is 9, forming a 9-40-41 right triangle.
  • 🔍 Cosine(theta) is calculated as -40/41, tangent(theta) as 9/-41, and their reciprocals are found for secant, cosecant, and cotangent.
  • 🔢 In the second scenario, cosine(theta) = 1/3 and tangent(theta) < 0, placing the triangle in quadrant four.
  • 🧩 The Pythagorean theorem is used to find the missing side of the triangle, which is 2√2.
  • 📉 Sine(theta) is found to be -2√2/3, and other trigonometric functions are derived accordingly.
  • 📚 The third scenario starts with secant being equal to negative four and sine(theta) > 0, placing the triangle in quadrant two.
  • 🔢 Cosine(theta) is deduced to be -1/4, and the missing side of the triangle is calculated to be √15.
  • 📈 Sine(theta) is then calculated as √15/4, and the reciprocals are found for cosecant, tangent, and cotangent.
  • 📝 Rationalization is used to simplify the expressions for cosecant and cotangent in the third scenario.
Q & A

  • What is the value of sine theta given in the script?

    -The value of sine theta is given as positive 9 over 41.

  • Which quadrant does the angle theta lie in based on the given sine and cosine values?

    -Theta lies in quadrant two because sine is positive and cosine is negative.

  • How is the triangle drawn in the script with sine and cosine values?

    -The triangle is drawn with the opposite side corresponding to 9, the hypotenuse to 41, and the adjacent side to -40 in quadrant two.

  • What is the value of cosine theta when tangent theta is less than zero and cosine theta is equal to one over three?

    -The value of cosine theta is one over three, and the triangle is drawn in quadrant four.

  • How is the missing side of the triangle calculated using the Pythagorean theorem in the second scenario?

    -The missing side (b) is calculated as the square root of (3^2 - 1^2), which is the square root of 8, and simplifies to 2 times the square root of 2.

  • What is the value of sine theta when cosine theta is one over three?

    -Sine theta is negative two square root two over three in quadrant four.

  • What is the value of secant when it is given as negative four?

    -If secant is negative four, then cosine is negative one over four.

  • In which quadrant is the triangle drawn when secant is negative four and sine is greater than zero?

    -The triangle is drawn in quadrant two because cosine is negative and sine is positive.

  • How is the missing side of the triangle found when secant is negative four?

    -The missing side (b) is found using the Pythagorean theorem as the square root of (4^2 - (-1)^2), which is the square root of 15.

  • What is the value of tangent theta when secant is negative four?

    -Tangent theta is the square root of 15 divided by -1 in quadrant two.

  • How are the reciprocal trigonometric functions (secant, cosecant, cotangent) calculated in the script?

    -The reciprocal functions are calculated by taking the reciprocal of the original trigonometric function's value, and rationalizing where necessary.

Outlines
00:00
📚 Finding Trigonometric Functions Given Sine and Cosine

This paragraph explains how to determine the values of trigonometric functions when sine of theta equals 9/41 and cosine theta is less than zero. The process involves identifying the quadrant where the angle lies based on the signs of sine and cosine, which leads to quadrant two. The triangle is then drawn with sine corresponding to the opposite side and cosine to the adjacent side over the hypotenuse. Using the Pythagorean theorem, the missing side is calculated. The remaining trigonometric functions are found by applying trigonometric identities and reciprocal relationships. The tangent, secant, and cosecant are calculated directly, while the cotangent is found by taking the reciprocal of the tangent and rationalizing the result.

05:01
🔍 Determining Trigonometric Functions with Cosine and Tangent

The second paragraph focuses on finding the trigonometric functions when cosine theta equals 1/3 and tangent theta is less than zero, indicating the triangle is in quadrant four. The hypotenuse is determined from the cosine value, and the missing side is found using the Pythagorean theorem. The sine is then calculated as the opposite side over the hypotenuse. The tangent is the opposite side over the adjacent side, and the secant is the reciprocal of the cosine. The cosecant is the reciprocal of the sine, which is rationalized to obtain the final value. The cotangent is the reciprocal of the tangent, rationalized to give the final value, completing the set of trigonometric functions for the given conditions.

Mindmap
Keywords
💡sine
The sine function is a fundamental trigonometric function that relates the ratio of the length of the opposite side to the hypotenuse in a right-angled triangle. In the video, sine is used to establish the relationship between the angle theta and the sides of the triangle. The script mentions 'sine of theta is equal to 9 over 41', which sets the foundation for finding the other trigonometric functions.
💡cosine
Cosine is another basic trigonometric function that represents the ratio of the adjacent side to the hypotenuse in a right-angled triangle. The script discusses the sign of cosine to determine the quadrant of the angle theta, stating 'cosine theta is less than zero', which indicates that the triangle is in the second quadrant where cosine values are negative.
💡quadrant
A quadrant refers to one of the four equal areas created by the intersection of the x-axis and y-axis in the Cartesian coordinate system. The video uses the concept of quadrants to determine the location of the triangle and the signs of the trigonometric functions. For example, the script states that since sine is positive and cosine is negative, the triangle must be in quadrant two.
💡tangent
Tangent is a trigonometric function defined as the ratio of the opposite side to the adjacent side in a right-angled triangle. The video calculates the tangent of theta as 'nine over negative forty one' in the first scenario and later as 'negative two square root two over one' in the second scenario, illustrating its use in different contexts.
💡secant
Secant is the reciprocal of the cosine function. It is used to find the relationship between the hypotenuse and the adjacent side. In the video, the script uses the secant value 'negative four' to deduce that cosine is 'negative one over four', which helps in determining the angle's quadrant and the triangle's dimensions.
💡Pythagorean theorem
The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. The video applies this theorem to find the missing side of the triangle, as shown when calculating 'b is equal to the square root of eight'.
💡reciprocal
A reciprocal is a number which, when multiplied by the original number, results in one. In trigonometry, the reciprocal functions are secant, cosecant, and cotangent. The video explains how to find these functions by taking the reciprocal of cosine, sine, and tangent, respectively, as seen in 'secant is the reciprocal of cosine so it's negative forty one over forty'.
💡rationalize
Rationalizing a denominator is the process of eliminating square roots from the denominator of a fraction. The video demonstrates this process when finding the cosecant value, where 'negative three square root two over two' is rationalized to 'negative three square root two over four'.
💡hypotenuse
The hypotenuse is the longest side of a right-angled triangle, opposite the right angle. The video consistently uses the hypotenuse as the reference for calculating trigonometric ratios, such as '41 is the hypotenuse of the triangle' and 'three is the hypotenuse' in different scenarios.
💡opposite side
In a right-angled triangle, the opposite side is the side that is opposite the angle being considered. The video uses the opposite side to define the sine function, as in 'sine is opposite over hypotenuse', and to calculate the tangent and cotangent functions.
💡adjacent side
The adjacent side is the side of a right-angled triangle that is next to the angle being considered, but not the hypotenuse. The video uses the adjacent side to define the cosine function, as in 'cosine theta is adjacent over hypotenuse', and to calculate the tangent and cotangent functions.
Highlights

Sine of theta is given as 9/41 and cosine theta is less than zero, indicating the triangle is in quadrant two.

Sine is positive in quadrants one and two, while cosine is negative in quadrants two and three.

A 9-40-41 right triangle is used to determine the trigonometric functions.

Cosine theta is calculated as -40/41 using the adjacent side over the hypotenuse.

Tangent theta is found to be 9/-41, which is opposite over adjacent.

Secant is the reciprocal of cosine, resulting in -41/40.

Cosecant is the reciprocal of sine, calculated as 41/9.

Cotangent is the reciprocal of tangent, yielding -41/9.

Cosine theta is given as 1/3 and tangent theta is less than zero, placing the triangle in quadrant four.

Cosine is positive in quadrants one and four, and tangent is negative in quadrants two and four.

Using the Pythagorean theorem, the missing side of the triangle is calculated as 2√2.

Sine theta is determined as -2√2/3, based on the opposite side over the hypotenuse.

Tangent theta is -2√2/1, found by opposite over adjacent.

Secant is the reciprocal of cosine, which is 3 in this case.

Cosecant is rationalized to -3√2/4, the reciprocal of sine.

Cotangent is rationalized to -√2/4, the reciprocal of tangent.

Secant is given as -4, and sine is greater than zero, indicating the triangle is in quadrant two.

Cosine is deduced as -1/4 from the negative secant value.

The missing side of the triangle is found to be √15 using the Pythagorean theorem.

Sine theta is calculated as √15/4, opposite over hypotenuse.

Cosecant is 4/√15, the reciprocal of sine, and then rationalized.

Tangent theta is √15/-1, opposite over adjacent.

Cotangent is -√15/15, the reciprocal of tangent, after rationalization.

Transcripts

Browse More Related Video

ILLUSTRATING CIRCULAR FUNCTIONS || PRE-CALCULUS

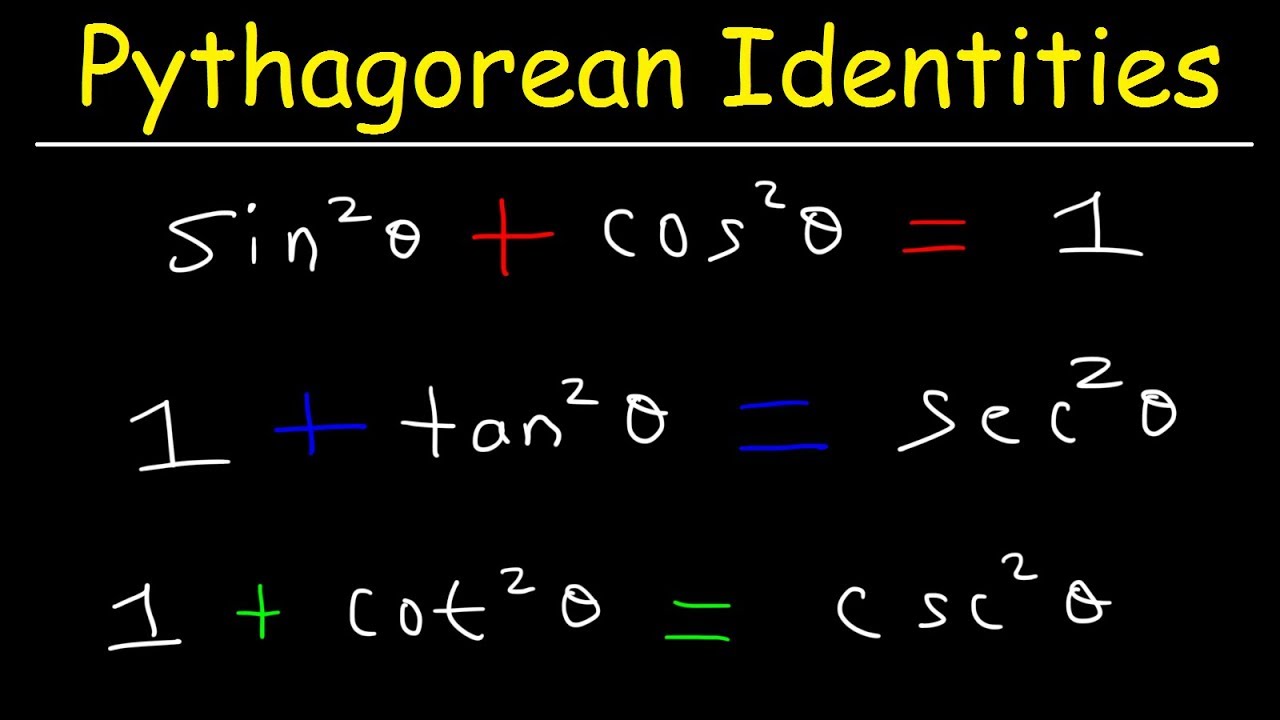
Pythagorean Identities - Examples & Practice Problems, Trigonometry

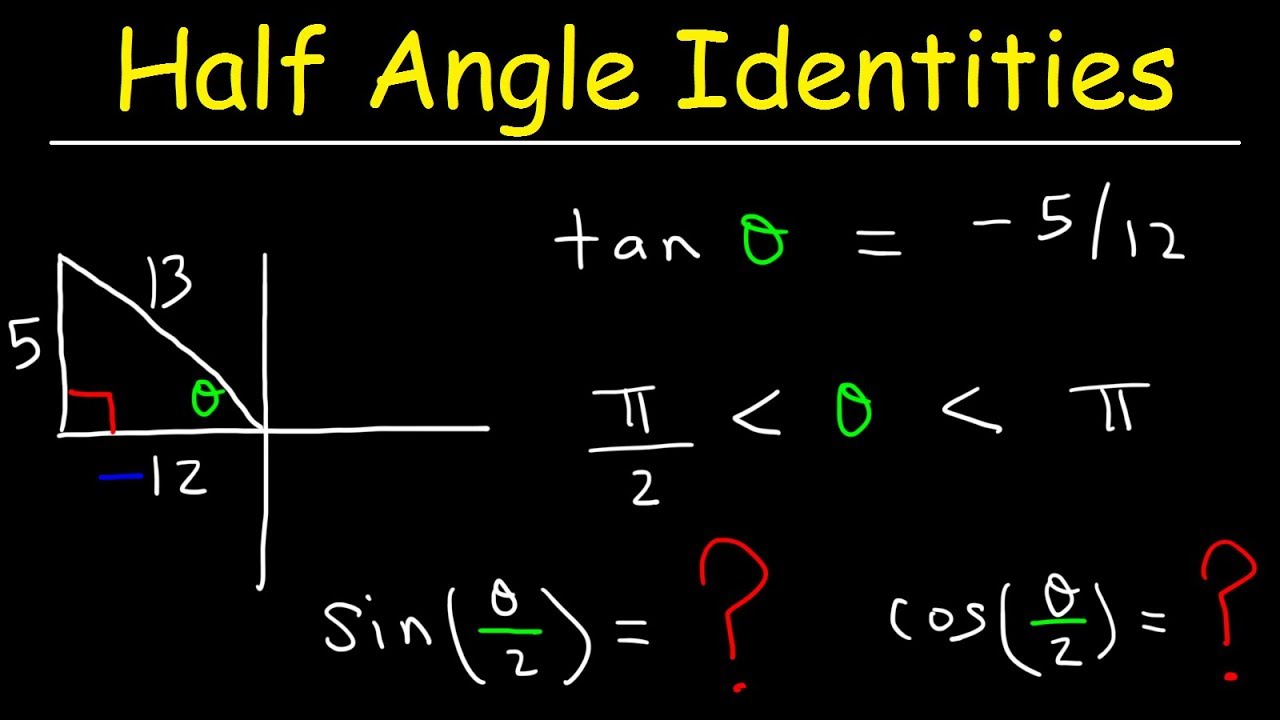
Right Triangle Trigonometry and Half Angle Identities & Formulas

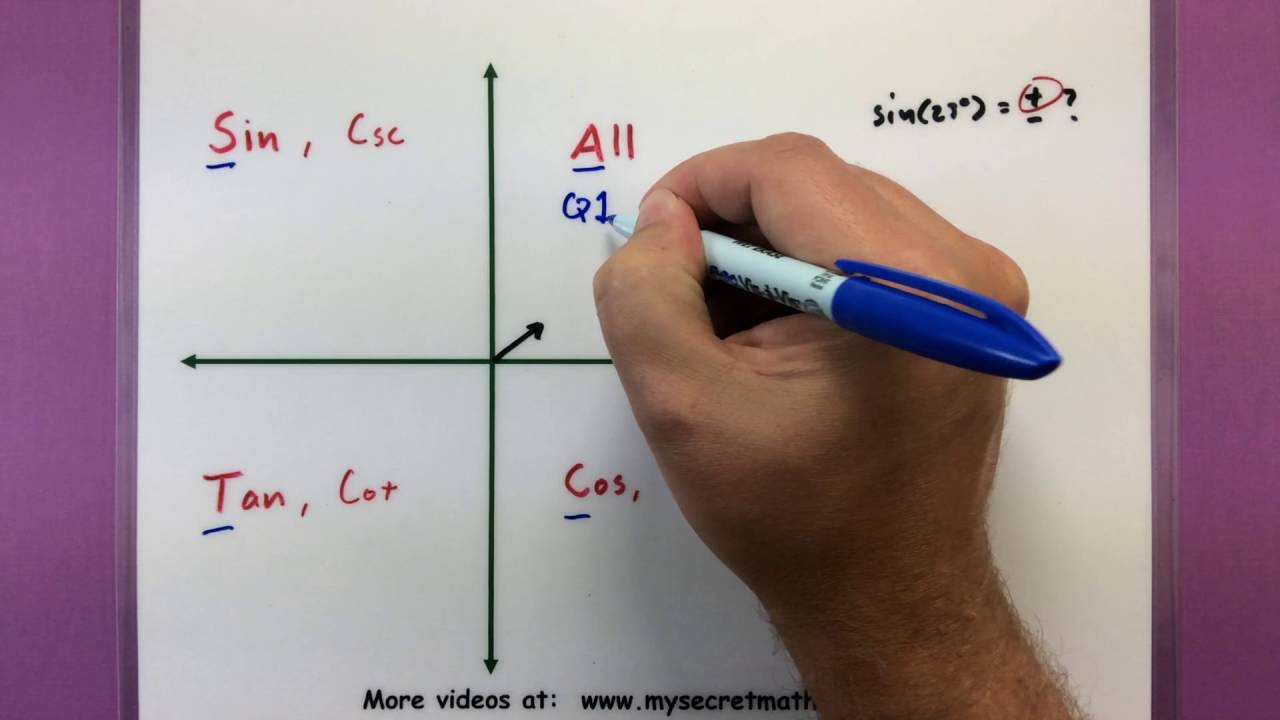
Trigonometry - The signs of trigonometric functions

Introduction to Right Triangle Trigonometry (Precalculus - Trigonometry 30)

Trigonometric Functions of Any Angle - Unit Circle, Radians, Degrees, Coterminal & Reference Angles

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryProblem SolvingMath ScriptQuadrant AnalysisPythagorean TheoremReciprocal FunctionsTrigonometric IdentitiesCosineSineTangent