Long Division With Polynomials - The Easy Way!
TLDRThis instructional video offers a step-by-step guide on dividing polynomials using long division. It begins with a simple example, dividing \(x^2 + 5x + 6\) by \(x + 2\), and progresses to more complex cases, such as dividing \(2x^3 + 8x^2 - 6x + 10\) by \(x - 2\) and \(6x^4 - 9x^2 + 18\) by \(x - 3\). The video demonstrates the process of dividing, multiplying, and subtracting to find the quotient and remainder, ultimately solving each polynomial division problem. The clear explanation and methodical approach make it an engaging and educational resource for understanding polynomial long division.
Takeaways
- 📚 The video focuses on dividing polynomials using long division, starting with the example of dividing \(x^2 + 5x + 6\) by \(x + 2\).
- 📝 The process begins by placing the divisor \(x + 2\) on the outside and the dividend \(x^2 + 5x + 6\) on the inside.
- 🔢 The first step in long division is to divide the leading terms, \(x^2\) by \(x\), which equals \(x\).
- ➗ After dividing, the next step is to multiply the divisor \(x + 2\) by the result \(x\) and then subtract from the dividend.
- ✂ The subtraction step eliminates the \(x^2\) term, leaving \(3x + 6\).
- 🔄 The process repeats with dividing \(3x\) by \(x\), resulting in \(3\), and then multiplying and subtracting to simplify the expression further.
- 🎯 The remainder after the final subtraction is zero, indicating that \(x + 3\) is the quotient for the division of \(x^2 + 5x + 6\) by \(x + 2\).
- 📈 The video continues with another example, dividing \(2x^3 + 8x^2 - 6x + 10\) by \(x - 2\), encouraging viewers to pause and try the problem themselves.
- 📉 The method involves dividing each term by \(x\), multiplying the divisor by the result, and subtracting to simplify, repeating these steps until the remainder is found.
- 🔍 When a remainder is present, it is included in the final answer, written as the remainder divided by the divisor.
- 📚 The final example involves dividing \(6x^4 - 9x^2 + 18\) by \(x - 3\), with a reminder to be careful and to include all terms, including those with zero coefficients.
- 🏁 The video concludes by summarizing the steps and providing the final answers for each polynomial division problem.
Q & A
What is the main topic of the video?
-The main topic of the video is dividing polynomials using long division.
What is the first polynomial division example given in the video?
-The first example is dividing \(x^2 + 5x + 6\) by \(x + 2\).
What is the result of the first polynomial division example?
-The result of the first example is \(x + 3\).
What is the second polynomial division example in the video?
-The second example is dividing \(2x^3 + 8x^2 - 6x + 10\) by \(x - 2\).
What is the quotient of the second polynomial division example?
-The quotient of the second example is \(2x^2 + 12x + 18\).
What is the remainder of the second polynomial division example?
-The remainder of the second example is \(46\).
What is the third polynomial division example discussed in the video?
-The third example is dividing \(6x^4 - 9x^2 + 18\) by \(x - 3\).
What is the final answer for the third polynomial division example?
-The final answer for the third example is \(6x^3 + 18x^2 + 45x + 145 + \frac{453}{x - 3}\).
What are the three main steps in polynomial long division?
-The three main steps in polynomial long division are divide, multiply, and subtract.
What is the purpose of writing the remainder in the final answer of a polynomial division?
-The purpose of writing the remainder is to show what is left over after the division process, which cannot be divided further by the divisor.
How does the video script guide the viewer to perform polynomial long division?
-The video script guides the viewer through each step of the long division process with examples, explaining how to divide, multiply, and subtract terms at each stage.
Outlines
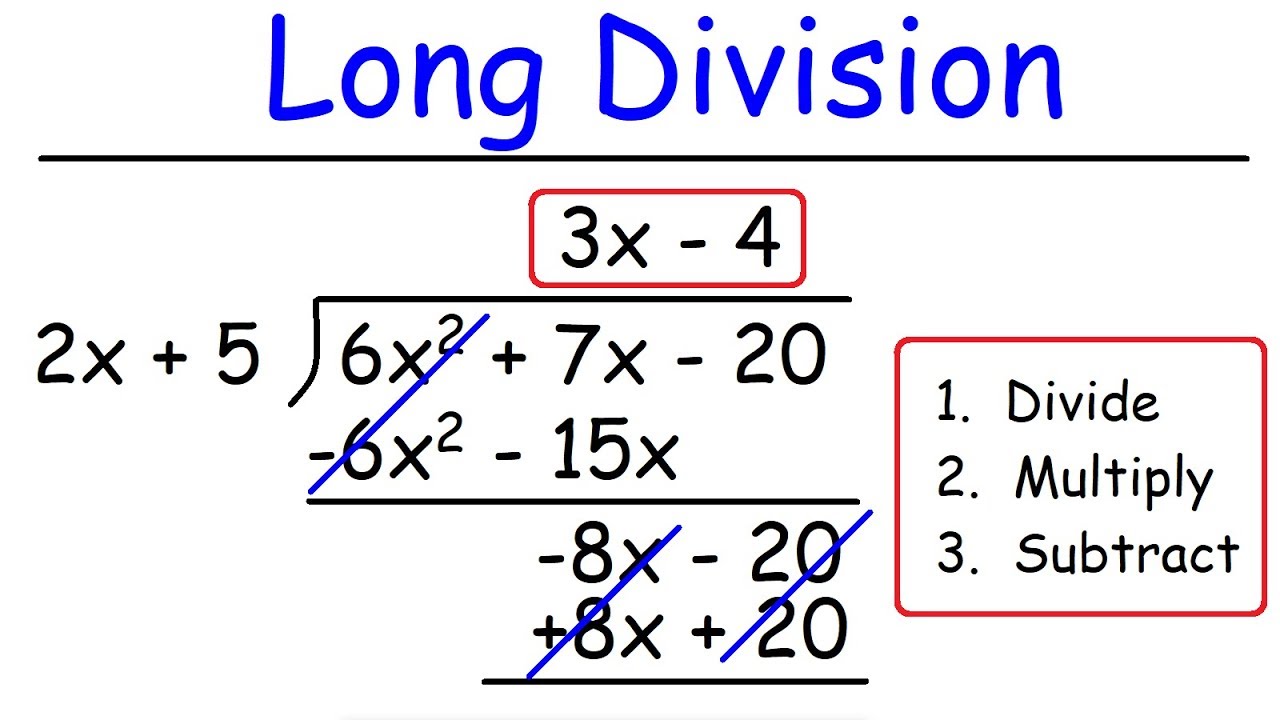
📚 Dividing Polynomials Using Long Division
This paragraph introduces the concept of dividing polynomials using long division. The first example involves dividing \( x^2 + 5x + 6 \) by \( x + 2 \). The process begins by setting up the division with the divisor \( x + 2 \) on the outside and the dividend \( x^2 + 5x + 6 \) on the inside. The division starts by dividing the first term \( x^2 \) by \( x \), resulting in \( x \). This is followed by multiplying \( x \) by the divisor \( x + 2 \) and subtracting the result from the dividend. The process is repeated for the remaining terms, leading to a quotient of \( x + 3 \) and a remainder of zero. The method is then applied to a second example, dividing \( 2x^3 + 8x^2 - 6x + 10 \) by \( x - 2 \), where the viewer is encouraged to pause the video and attempt the division themselves.
🔍 Continuing Polynomial Long Division with a Complex Example
The second paragraph continues the tutorial on polynomial long division with a more complex example. The task is to divide \( 2x^3 + 8x^2 - 6x + 10 \) by \( x - 2 \). The division starts by dividing \( 2x^3 \) by \( x \) to get \( 2x^2 \), and then multiplying \( 2x^2 \) by \( x - 2 \) and subtracting from the original polynomial. This process is repeated, with each term being divided by \( x \), multiplied by the divisor, and then subtracted from the current terms. The result is a quotient of \( 2x^2 + 12x + 18 \) and a remainder of 46, which is then expressed as \( 46 / (x - 2) \). The final answer is given as \( 2x^2 + 12x + 18 + 46 / (x - 2) \), which simplifies to \( 2x^3 + 8x^2 - 6x + 10 / (x - 2) \).
📘 Advanced Polynomial Long Division with Remainders
The third paragraph presents an advanced example of polynomial long division with a remainder. The polynomial \( 6x^4 - 9x^2 + 18 \) is to be divided by \( x - 3 \). The division process involves setting up the division with the correct powers of \( x \), including zero powers where necessary. The division of \( 6x^4 \) by \( x \) yields \( 6x^3 \), and the subsequent multiplication and subtraction steps are carried out. The process is repeated for the \( x^3 \) term, resulting in \( 18x^3 \), and then for the \( x^2 \) term, leading to \( 45x^2 \). The remainder is calculated, and the final expression is simplified to \( 6x^3 + 18x^2 + 45x + 145 + 453 / (x - 3) \). The remainder \( 453 \) is included in the final answer, demonstrating how to handle polynomial division with non-zero remainders.
Mindmap
Keywords
💡Polynomials
💡Long Division
💡Dividend
💡Divisor
💡Quotient
💡Remainder
💡Exponents
💡Distribute
💡Subtract
💡Multiply
💡Standard Order
Highlights
Introduction to dividing polynomials using long division.
Dividing x squared plus 5x plus 6 by x plus 2 as the first example.
Placing the divisor (x plus 2) on the outside and the dividend (x squared plus 5x plus 6) on the inside.
Step-by-step guide starting with dividing x squared by x.
Subtracting exponents to simplify the division process.
Multiplying the result by the divisor and distributing the negative sign.
Subtracting terms to simplify and bring down the next term.
Continuing the process with dividing 3x by x and multiplying by the divisor.
Resulting in a remainder of zero and the quotient x plus 3.
Moving on to the second example: dividing two x cubed plus eight x squared minus six x plus ten by x minus two.
Dividing 2x cubed by x to get 2x squared and multiplying accordingly.
Subtracting terms to simplify and proceed to the next step.
Repeating the divide, multiply, and subtract steps for the polynomial.
Finalizing the division with a remainder of 46 and expressing the result.
Starting the third problem: dividing six x to the fourth minus nine x squared plus eighteen by x minus three.
Following the same steps for dividing higher degree polynomials.
Calculating the remainder and expressing the final answer.
Emphasizing the importance of careful calculation and checking with a calculator.
Conclusion on how to divide polynomials using long division.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: