Dear Calculus 2 Students, This is why you're learning Taylor Series
TLDRThis video, sponsored by Brilliant, explores the necessity of approximations in solving complex real-world problems that are often mathematically intractable. It highlights the use of numerical methods, particularly Taylor and Maclaurin series, to approximate solutions to differential equations found in various fields such as engineering and physics. The script demonstrates how these series can simplify equations for tasks like analyzing forces in a car crash or the stress on a bridge, and even extends to more advanced topics like the speed of sound and energy equations. The video emphasizes the practicality of 'good enough' solutions and the educational value of Brilliant's courses in advanced mathematics and related fields.
Takeaways
- 🧑💼 The video discusses the complexity of real-world problems in engineering and physics that often involve solving difficult or impossible differential equations.
- 📚 Engineers and scientists use numerical methods and approximations, such as Taylor series, to find solutions to these equations when analytical methods fail.
- 📉 The Maclaurin series is introduced as a polynomial that approximates functions like e^x, improving with more terms and perfectly matching the original function if an infinite number of terms are included.
- 📈 The importance of initial conditions in differential equations is highlighted, as they provide the starting point for constructing a Taylor series approximation.
- 🔍 The script explains how to derive coefficients for a Taylor series by plugging in derivatives and initial conditions into the original equation.
- 🔧 Taylor series are used to approximate complex functions in various fields, such as physics, where they simplify equations for practical applications.
- 🚗 Examples include approximating the deformation of a car in a crash or the stress throughout a bridge due to traffic, which are crucial for safety and design.
- 📚 The script provides a step-by-step example of how to use a Taylor series to approximate a solution to a nonlinear differential equation.
- 📉 The video also touches on the use of approximations in physics, such as the speed of sound in air and the total energy of an object, which are derived from more complex equations using Taylor series.
- 🔬 The concept of asymptotic behavior is introduced, showing how Taylor series can be used to analyze the behavior of physical systems, like the electric field around charges.
- 🎓 The video concludes by emphasizing the importance of approximations in real-world applications and mentions the educational resource Brilliant.org for further learning.
Q & A
Why are differential equations often impossible to solve analytically?
-Differential equations are often impossible to solve analytically because they can become extremely complex, especially when dealing with real-world phenomena such as fluid flow, heat transfer, and structural stresses. These equations can involve multiple variables and non-linear relationships that make finding an exact solution impractical or impossible.
What is the purpose of using numerical methods to approximate solutions to difficult equations?
-Numerical methods are used to approximate solutions to difficult equations because they allow engineers and scientists to find solutions that are close enough to the exact ones for practical purposes. These approximations are essential for designing structures, analyzing physical phenomena, and making predictions where exact solutions are unattainable.
What is a Maclaurin series and how does it relate to the approximation of functions?
-A Maclaurin series is a type of Taylor series that is centered around the point x = 0. It is a polynomial that approximates a function by matching the function's derivatives at x = 0 with those of the polynomial. The more terms included in the series, the better the approximation, with an infinite number of terms providing a perfect match.
How do Taylor series help in solving real-world problems?
-Taylor series help in solving real-world problems by providing a way to approximate complex functions with polynomials. This allows for easier computation and analysis, especially in fields like engineering and physics where exact solutions to differential equations may not be feasible.
What is the significance of the first derivative and y-value matching at x equals zero in a Maclaurin series?
-The significance of the first derivative and y-value matching at x equals zero in a Maclaurin series is that it ensures the polynomial approximation starts off correctly. This means the polynomial and the function it approximates have the same value and slope at the point of expansion, which is crucial for the accuracy of the approximation near that point.
Can you provide an example of how a Taylor series is used to approximate a function?
-An example from the script is the approximation of e^x using its Maclaurin series. By including more terms of the series, the polynomial approximation becomes increasingly accurate. For instance, the first two terms give a tangent line approximation, which is accurate for x values close to zero, and adding more terms improves the approximation further.
How does the concept of Taylor series relate to the approximation of physical phenomena like car crashes?
-Taylor series relate to the approximation of physical phenomena like car crashes by allowing computers to simulate these events. The series provides a way to approximate the solutions to the often complex differential equations that describe the physics of such events, enabling engineers to predict outcomes and improve safety measures.
What is the role of initial conditions in the construction of a Taylor series?
-Initial conditions play a crucial role in the construction of a Taylor series because they provide the necessary values for the first few coefficients of the series. These conditions, such as the value of the function and its derivatives at a specific point, allow the series to be constructed and to accurately represent the function around that point.
How does the approximation of sine of theta as just theta simplify the equation for a simple pendulum?
-The approximation of sine of theta as just theta simplifies the equation for a simple pendulum by making it linear. This small angle approximation allows the motion of the pendulum to be modeled as simple harmonic motion, which is much easier to analyze and solve compared to the non-linear equation that would result without this approximation.
Can you explain how Taylor series are used to approximate the speed of sound in air?
-The speed of sound in air is approximated using the Maclaurin series of a more complex equation that describes this relationship. By taking the first two terms of the series, a linear approximation is obtained which works well around the reference temperature of 0 degrees Celsius. This approximation allows for quick calculations of the speed of sound at typical everyday temperatures.
Outlines
🔍 Complex Real-World Equations and Their Approximations
The first paragraph discusses the complexity of real-world problems such as analyzing forces on an aircraft, stresses on a building during an earthquake, and deformations in a car crash. It emphasizes the difficulty or impossibility of solving these equations analytically, which are often differential or partial differential equations. The solution to this problem is to use numerical methods and approximations, such as Taylor series, to find solutions that are close enough for practical purposes. The paragraph also explains the concept of the Maclaurin series as a polynomial approximation of e^x, which improves with more terms and eventually converges to the exact function if an infinite number of terms are included.
📐 Taylor Series and Local Approximations
The second paragraph delves into the concept of the Taylor series, which is a generalization of the Maclaurin series to functions not necessarily centered around zero. It explains that while Taylor series may not provide an infinite radius of convergence, they can offer local solutions for values of x close to the center point of the series. The paragraph gives examples of how approximations can be used in various contexts, such as approximating sine of X for small angles, simplifying the equation for a simple pendulum's motion, and approximating the speed of sound in air. It also touches on the topic of kinetic energy and its relation to the total energy of an object, including the famous equation E=mc^2, and how Taylor series can be used to derive approximations at low speeds.
🧮 Applications of Taylor Series in Physics and Engineering
The third paragraph explores further applications of Taylor series in physics and engineering. It discusses how Taylor series can simplify complex functions into more manageable forms, which is particularly useful in fields where precise solutions are not always necessary, but 'good enough' approximations are. The paragraph provides an example involving electric fields and charges, showing how a Taylor series can be used to approximate the behavior of the electric field at large distances from two charges of opposite signs. It concludes by highlighting the importance of approximation methods in real-world scenarios and mentions that there are entire courses dedicated to these techniques. The paragraph ends with a call to action for viewers interested in learning more about these topics to visit the sponsor's website, Brilliant.org.
Mindmap
Keywords
💡Sponsored
💡Differential Equations
💡Numerical Methods
💡Taylor Series
💡Maclaurin Series
💡Approximation
💡Initial Conditions
💡Radius of Convergence
💡Asymptotic Behavior
💡Brilliant.org
Highlights
The world's mathematical complications are exemplified by the difficulty in solving equations related to forces on an aircraft, stresses on buildings during earthquakes, and deformation in car crashes.
Often, there is no analytical method to find solutions for these complex real-world problems, necessitating the use of approximations.
Engineers and scientists rely on numerical methods to approximate solutions to differential equations that are frequently impossible to solve analytically.
Taylor series are introduced as a method to approximate solutions to complex equations, such as those simulating car crashes and airflow.
The Maclaurin series for e^x is explained as a polynomial that provides increasingly better approximations with more terms.
The importance of matching derivatives at a specific point, such as x=0, for creating an accurate Taylor series approximation is highlighted.
An example of using a Taylor series to approximate a solution to a nonlinear differential equation is provided.
The process of deriving coefficients for a Taylor series by using initial conditions and derivatives of the original equation is detailed.
Taylor series can provide local solutions for specific values of x, even if they do not converge to a perfect approximation for all x.
The Maclaurin series for sine is used to approximate sine of X for small values, simplifying complex problems like the simple pendulum equation.
The approximation of sine theta as theta for small angles is shown to simplify equations and provide reasonably accurate results.
The speed of sound in air is discussed as an example where a Taylor series approximation is used to simplify a complex equation.
Kinetic energy and its relation to the intrinsic energy of objects at rest are explained, with Taylor series showing the approximations involved.
The electric field behavior of two opposite charges is analyzed using a Taylor series to show the asymptotic behavior at large distances.
The concept of 'good enough' approximations is emphasized, as being professionally acceptable in many real-world applications.
Brilliant.org is introduced as a resource for learning more about power series, calculus, and other advanced math topics.
The video concludes with an invitation to support the channel and explore more on Patreon and social media.
Transcripts
Browse More Related Video

What Math Classes Do Engineers (and Physics Majors) Take?

Physics Students Need to Know These 5 Methods for Differential Equations
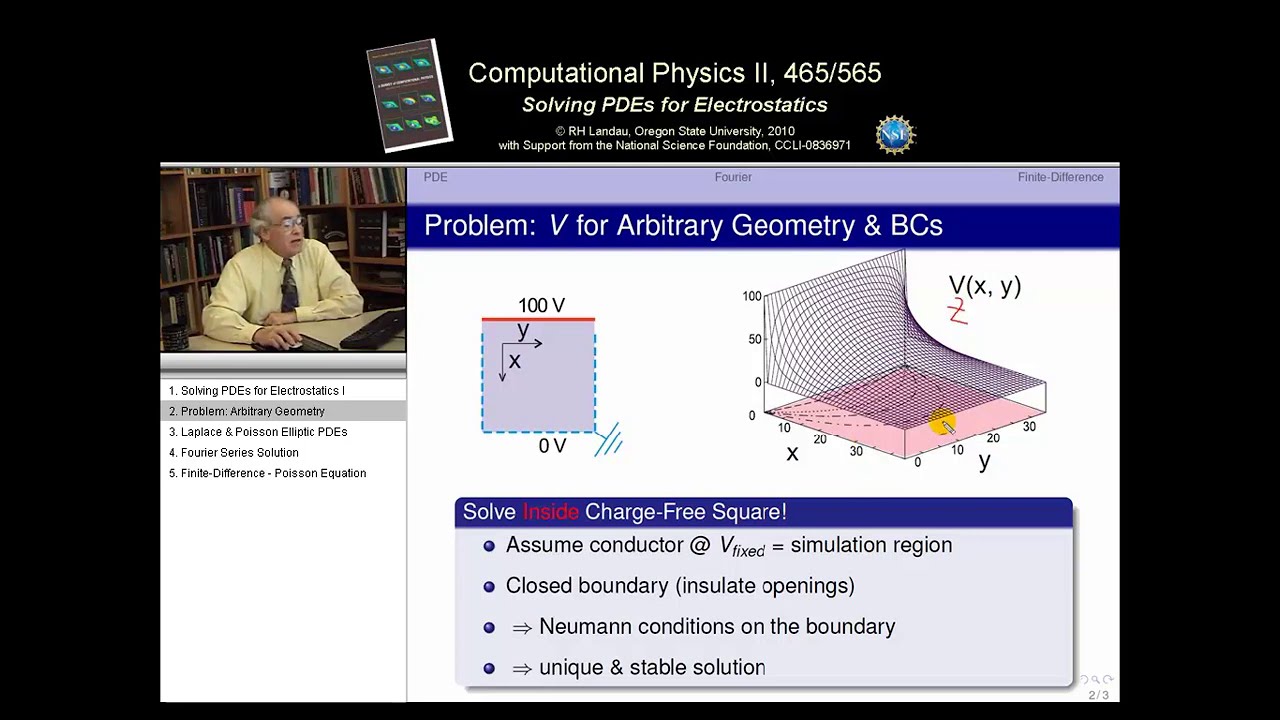
19.2 Electrostatic PDEs I

Lecture 22: Fields of Arrows Differential Equations
Business Calculus - Math 1329 - Section 5.1 - Indefinite Integration & Differential Equations

AP Calculus AB: Lesson 7.2 Slope Fields
5.0 / 5 (0 votes)
Thanks for rating: