Easily Explain or Interpret Meaning of a Derivative in the Context of the Problem
TLDRThe video script is a comprehensive guide on interpreting the meaning of derivatives in various contexts, a common topic in AP calculus exams. The speaker emphasizes the importance of understanding the context and units associated with the derivative, and provides a systematic approach to solving such problems. The method involves identifying what the input represents, determining if the original function is increasing or decreasing based on the derivative's sign, and including the correct units in the final answer. The script walks through several examples, including calculating the rate at which customers remove bananas from a display, the rate of grass clippings in a bin, and the rate of change in wind chill. The speaker also discusses the importance of not getting overwhelmed by the complexity of the problem and encourages viewers to practice and master the technique for successful problem-solving in exams.
Takeaways
- 📚 The video discusses the importance of using correct units when explaining the meaning of derivatives, which is a common topic in AP calculus exams.
- 🔍 Students are often asked to interpret a derivative they find or are given, which may involve using a calculator or approximation methods like the average rate of change.
- 📉 Identifying the input variable's context is crucial, as it helps in understanding what the function represents and how it behaves in terms of increasing or decreasing.
- 📈 The process of interpreting a derivative involves understanding whether the original function is increasing or decreasing based on the derivative's positive or negative value.
- 📌 Including units in the answer is essential as it provides a complete understanding of the derivative's meaning in the context of the problem.
- ⏱️ An example given in the video is interpreting F'(7), where '7' represents hours after a store opens, and F(t) represents the rate at which customers remove bananas from a display.
- 🌿 For another example, '15' in the context of a different problem represents days, with the function a(t) representing the amount of grass clippings remaining in a bin over time.
- ⛏️ In a problem involving gravel processing, G'(5) is used to interpret the rate at which unprocessed gravel arrives at a plant, with '5' representing hours after the plant opened.
- 🌡️ Estimating W'(12) from a table involves understanding that '12' is a time value in minutes, with W(t) representing the temperature of water in a tub.
- 🛤️ F'(4) in a given context could represent the rate of change of the distance between a road and the edge of water during a storm, with '4' representing hours after the storm began.
- ⚙️ The video emphasizes the importance of a systematic approach to solving and interpreting derivative problems, which can be applied across various contexts and problems.
- 📉 The interpretation of derivatives involves a step-by-step process: identifying the input's context, determining if the function is increasing or decreasing, calculating the value, and including the appropriate units.
Q & A
What is the commonality between the problems discussed in the video?
-The commonality is that each problem involves finding and interpreting the derivative of a function in a specific context, using correct units and explaining the meaning of the derivative within that context.
What is the first step in interpreting the meaning of a derivative in a given context?
-The first step is to identify what the input represents in the problem, such as what 'T' or 'R' stands for in the context of the function.
How does one determine if the original function is increasing or decreasing?
-One determines if the original function is increasing or decreasing based on whether the derivative's value is positive or negative.
What is the importance of including units in the answer when interpreting a derivative?
-Including units in the answer provides a complete understanding of the rate of change, specifying how the quantity represented by the function is changing per unit of the input variable.
In the context of the video, what does F'(7) represent?
-F'(7) represents the rate at which customers remove bananas from the display table, seven hours after the store opens.
What is the numerical value of F'(7) in the video?
-The numerical value of F'(7) is approximately negative 8.120.
How is the rate of change of grass clippings in a bin modeled in the second example?
-The rate of change of grass clippings in a bin is modeled by the function a(t), where 't' is measured in days.
What does the value of a'(15) signify in the second example?
-The value of a'(15) signifies that at 15 days, the amount of grass clippings remaining in the bin is decreasing by approximately 0.164 pounds per day.
In the context of the third example, what does G'(5) represent?
-G'(5) represents the rate at which unprocessed gravel arrives at a gravel processing plant, five hours after the plant opened for the day.
What is the numerical value of G'(5) in the third example?
-The numerical value of G'(5) is negative 24.588, indicating a decreasing rate.
How is the rate of change of water temperature in a tub represented in the fourth example?
-The rate of change of water temperature in a tub is represented by the function w(t), where 't' is measured in minutes.
What does the value of w'(12) signify in the fourth example?
-The value of w'(12) signifies that 12 minutes after the water starts heating, the temperature of the water in the tub is increasing by approximately 1.017 degrees Fahrenheit per minute.
Outlines
📚 Understanding Derivatives in AP Calculus
The video begins with an introduction to the commonality and doability of problems involving the interpretation of derivatives in AP Calculus. The presenter emphasizes the importance of using the correct units and not to be intimidated by such problems. The approach to solving these problems involves identifying the input variable's meaning, determining if the original function is increasing or decreasing based on the derivative's sign, and including units in the final answer. An example problem is used to illustrate the method, where F'(7) represents the rate at which customers remove bananas from a display table, measured in pounds per hour.
🌿 Interpreting the Rate of Change in Grass Clippings
The second paragraph focuses on another example problem, where the amount of grass clippings remaining in a bin is modeled by a function a(t). The presenter explains how to interpret the derivative a'(15), which represents the rate of change of grass clippings in the bin at 15 days. The units are derived from the function's units (pounds) divided by the input's units (days), resulting in pounds per day. The presenter advises using the problem's wording to describe the function and its rate of change for clarity and simplicity.
🏭 Analyzing the Rate of Unprocessed Gravel Arrival
The third paragraph discusses the interpretation of G'(5) in the context of a gravel processing plant. The presenter outlines the steps to understand the meaning of the derivative: identifying the input variable T, which represents hours after the plant opened; determining the rate of change of G(t), which is the rate at which unprocessed gravel arrives; and deciding if the rate is increasing or decreasing based on the derivative's value. The units for the derivative are derived from the function's units (tons per hour) divided by the input's units (hours), leading to tons per hour squared.
🌡️ Estimating the Rate of Water Temperature Change
In the fourth paragraph, the presenter tackles the problem of estimating W'(12) using data from a table. The process involves calculating the average rate of change to approximate the derivative. The presenter explains that W(t) represents the temperature of water in a tub at time T, and W'(12) indicates the rate of change of water temperature 12 minutes after heating begins. The units for this derivative are degrees Fahrenheit per minute, and the rate is increasing by approximately 1.017 degrees Fahrenheit per minute.
Mindmap
Keywords
💡Derivative
💡Units
💡Rate of Change
💡Interpretation
💡AP FRQs
💡Context
💡Increasing/Decreasing
💡Calculator
💡Problem-Solving
💡Average Rate of Change
💡Function
Highlights
The video discusses the importance of using the correct unit when interpreting the meaning of derivatives.
Derivatives are a common topic in AP calculus exams, making them an essential skill for students to master.
The process of interpreting a derivative involves identifying the input variable and what the function represents.
Understanding whether the derivative is positive or negative is crucial to determine if the original function is increasing or decreasing.
Units of the derivative are derived from the units of the function and the input variable.
An example problem is solved, demonstrating how to find the rate at which customers remove bananas from a display over time.
The video emphasizes the importance of reading the problem to understand the context and what the variables represent.
Another example involves interpreting the derivative in terms of grass clippings remaining in a bin over days.
The concept of rate of change is applied to the context of unprocessed gravel arriving at a plant to find the derivative's meaning.
The video shows how to estimate a derivative using data from a table and interpret it in the context of water temperature change.
The process of interpreting derivatives is demonstrated with a confusing problem, emphasizing the systematic approach to understanding.
The video explains how to interpret the derivative of wind chill in terms of wind velocity and its impact on temperature.
An example of estimating the derivative from a table is provided, focusing on the density of a bacteria population in a petri dish.
The systematic approach to interpreting derivatives is emphasized as a key strategy for solving a variety of problems.
The video concludes with a summary of the method for finding and interpreting the meaning of a derivative in context.
The importance of practice and familiarity with the process is highlighted to ensure success in interpreting derivatives.
The video provides a comprehensive guide to understanding derivatives, applicable to a wide range of calculus problems.
Transcripts
Browse More Related Video

All about Rate In-Rate Out AP Calculus FRQs

Calculus AB/BC – 4.3 Rates of Change in Applied Contexts Other Than Motion
2022 AP Calculus AB BC Free Response #1
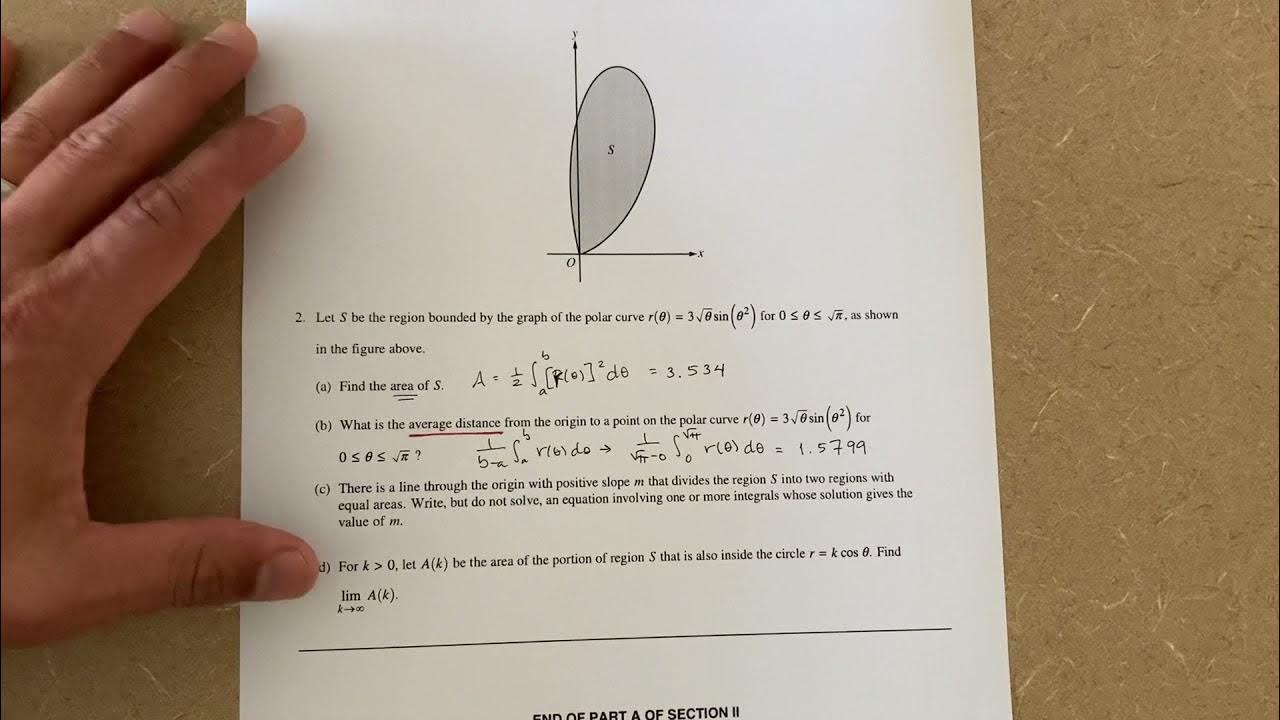
AP Calculus BC: Exam Prep 2021 Polar Curves FRQ

Using the Mean Value Theorem with a Table of Values

6 | FRQ (Calculator Active) | Practice Sessions | AP Calculus BC
5.0 / 5 (0 votes)
Thanks for rating: