6 | FRQ (Calculator Active) | Practice Sessions | AP Calculus BC

Advanced Placement
24 Apr 202313:55
EducationalLearning
32 Likes 10 Comments

TLDRIn this AP Calculus BC practice session, Tony Record and Bryan Passwater delve into a popular 'rate in and rate out' problem, a common feature on the AP Calc exam. They guide students through understanding the context of a factory conveyor belt scenario, where cans are placed and removed at different rates. The session emphasizes the importance of correctly storing functions in a calculator, interpreting derivatives, and applying the concepts of net rate of change and accumulation functions to determine the number of cans on the conveyor belt at a given time. The problem-solving process includes calculating the average rate of can removal, and finding the exact number of cans at a specific time, rounding to the nearest whole number for practical application.

Takeaways
  • 📚 The video is an AP Calculus BC practice session focusing on rate in and rate out problems.
  • 👨‍🏫 The instructors are Tony Record and Bryan Passwater, who guide students through a popular type of problem on the AP Calc exam.
  • 🏭 The problem scenario involves a factory machine placing labels on vegetable cans and workers removing them from a conveyor belt.
  • 📈 The rate of can placement is modeled by the function P(t) and the rate of can removal by R(t), both in cans per second.
  • 🕒 The initial condition states that there are 53 cans on the conveyor belt at time t equals zero.
  • 🧮 Part A of the problem asks to find P'(7) and explain its meaning, which is the rate of increase of cans on the conveyor belt at 7 seconds.
  • 📊 Part B requires determining the intervals of time when the number of cans on the conveyor belt is increasing, based on the net rate P(t) - R(t).
  • 🌟 The video emphasizes the importance of understanding and interpreting calculations, not just performing them.
  • 🔢 Part C involves calculating the average value of the rate of can removal from 10 to 40 seconds using a definite integral.
  • 📌 Part D asks for the number of cans on the conveyor belt at time 20, using the accumulation function and integration to find the amount from the given rates.
  • 🎯 The final answer for the number of cans on the conveyor belt at time 20, rounded to the nearest whole number, is 241.
Q & A

  • What is the main topic of the AP practice session discussed in the transcript?

    -The main topic of the AP practice session is the 'old rate in and rate out' problem, a popular problem type in the AP Calculus BC exam.

  • What is the context of the problem discussed in the video?

    -The context is a factory where a machine places labels on cans of vegetables and then puts them on a conveyor belt. Workers remove the cans from the conveyor belt to box them for shipping.

  • How is the rate at which cans are placed on the conveyor belt modeled?

    -The rate at which cans are placed on the conveyor belt is modeled by a function P(t), measured in cans per second.

  • What is the role of the function R(t) in the problem?

    -The function R(t) models the rate at which workers remove cans from the conveyor belt, also measured in cans per second.

  • What is the initial condition given in the problem?

    -The initial condition given is that at time t equals zero, there are 53 cans on the conveyor belt.

  • What does P'(7) represent in the context of the problem?

    -P'(7) represents the rate of increase (or the derivative) of the rate at which cans are placed on the conveyor belt at time t equals 7 seconds.

  • What is the value of P'(7) and what units are associated with it?

    -The value of P'(7) is 1.395 cans per second per second.

  • How does the net rate of change of cans on the conveyor belt relate to the functions P(t) and R(t)?

    -The net rate of change of cans on the conveyor belt is given by P(t) - R(t). If this value is greater than 0, the number of cans is increasing.

  • What is the interval of time during which the number of cans on the conveyor belt is increasing?

    -The number of cans on the conveyor belt is increasing on the interval 2.827 to 53.951 seconds.

  • What does the definite integral of R(t) with respect to t from 10 to 40 represent?

    -The definite integral of R(t) with respect to t from 10 to 40 represents the total number of cans removed from the conveyor belt during that time interval.

  • What is the average value of the rate R(t) from 10 to 40 seconds, and how many cans are on the conveyor belt at time 20?

    -The average value of the rate R(t) from 10 to 40 seconds is 4.170 cans per second. At time 20, there are approximately 241 cans on the conveyor belt.

  • What strategy is suggested for solving problems that involve finding an amount from a given rate?

    -The strategy suggested is to use the accumulation function and the modified fundamental theorem of calculus to find the amount at any given time by integrating the rate functions and adjusting for the initial amount.

Outlines
00:00
📚 Introduction to AP Calculus BC Practice - Rate In and Rate Out Problem

The video begins with Tony Record welcoming viewers back to AP calculus BC practice sessions. He is joined by Bryan Passwater, and they introduce a popular problem type for the AP calculus exam: the rate in and rate out problem. The problem involves a factory machine placing labels on vegetable cans and placing them on a conveyor belt, with workers removing the cans at a different rate. The problem requires understanding and applying concepts of derivatives and integrals to real-world scenarios. Tony emphasizes the importance of storing functions in the calculator and correctly using its features to find the derivative of the rate function at a specific time, which in this case is 1.395 cans per second per second at time t equals 7 seconds.

05:01
📈 Interpreting Rates and Understanding Increasing Cans on the Conveyor Belt

In this section, the focus is on understanding when the number of cans on the conveyor belt is increasing. It is explained that the given functions P(t) and R(t) represent rates of cans being placed or removed. The net rate of change of cans on the belt is determined by the difference between the rate in (P(t)) and the rate out (R(t)). The discussion involves using graphing calculators to visualize the functions and identify intervals where the net rate is positive, indicating an increase in the number of cans. The key points include understanding the concept of net rate, the use of graphing technology, and identifying the interval from 2.827 to 53.951 seconds where the number of cans is increasing.

10:04
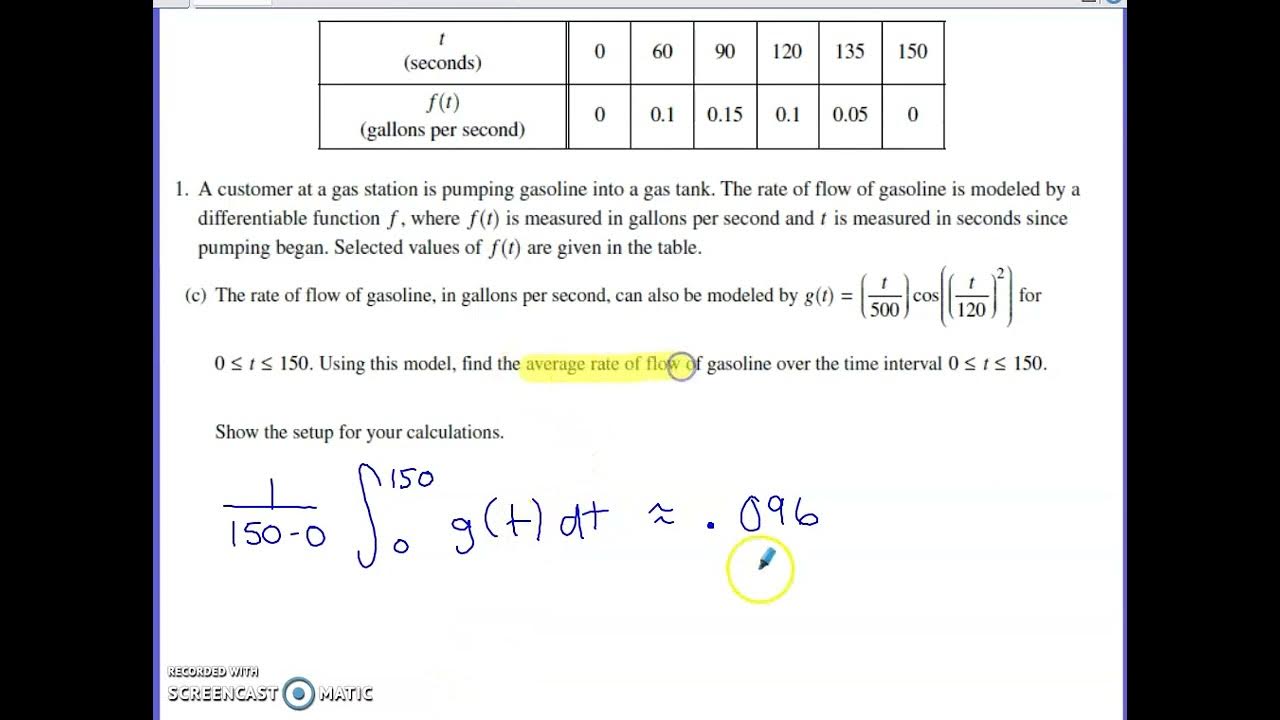
🧮 Calculating the Average Rate of Cans Removed from the Conveyor Belt

This paragraph focuses on calculating the average rate at which cans are removed from the conveyor belt between times 10 and 40 seconds. The calculation involves the definite integral of the rate function R(t) and the concept of the average value of a function. The process is explained step by step, including the use of calculator functions and the interpretation of the result. The average rate is found to be 4.170 cans per second, and the explanation emphasizes the importance of including units and the time interval in the justification.

📊 Determining the Number of Cans on the Conveyor Belt at Time 20

The final part of the video addresses how to find the number of cans on the conveyor belt at time 20 seconds. Bryan introduces the concept of the accumulation function and the modified fundamental theorem of calculus to find the amount from the given rates. The strategy involves starting with an initial amount of cans, adding the integral of the rate in, and subtracting the integral of the rate out. The initial amount is given as 53 cans, and the integrals are calculated for the time interval from 0 to 20 seconds. The result is 240.842 cans, which is rounded to 241 cans, highlighting the importance of attention to detail in exam scenarios.

Mindmap
Keywords
💡AP Calc Exam
The AP Calc Exam, or Advanced Placement Calculus Exam, is a standardized test in the United States that high school students take to demonstrate their proficiency in calculus. In the context of the video, the AP Calc Exam is the focus of the practice session, where the hosts discuss strategies and problems that are commonly found on the exam, such as rate in and rate out problems.
💡Rate In and Rate Out
Rate In and Rate Out refer to the rates at which items are added to and removed from a system, respectively. In the video, these rates are modeled by functions P(t) and R(t), which represent the number of cans placed on a conveyor belt and removed from it per second. Understanding these rates is crucial for determining the net change in the number of cans on the conveyor belt over time.
💡Derivative
In calculus, a derivative represents the rate of change of a function with respect to its independent variable. It is used to analyze how a quantity changes and to find the rate at which a function is increasing or decreasing at a particular point. In the video, the derivative of the rate functions (P'(t) and R'(t)) is used to determine the increasing or decreasing nature of the rate at which cans are placed on or removed from the conveyor belt.
💡Conveyor Belt
A conveyor belt is a mechanical device used to transport items, typically in a factory setting, from one location to another. In the video, the conveyor belt is used as a real-world example to explain the abstract concepts of calculus, specifically the rate at which items (cans of vegetables) are added to and removed from the belt over time.
💡Definite Integral
A definite integral represents the accumulated quantity or area under a curve over a specified interval. It is used to calculate the total change or accumulation of a quantity over time. In the video, the definite integral of the rate out function R(t) is used to find the total number of cans removed from the conveyor belt between two time intervals.
💡Graphing Calculator
A graphing calculator is an electronic device that can perform various mathematical calculations, including graphing functions, solving equations, and computing integrals. In the context of the video, the hosts emphasize the importance of using a graphing calculator to solve problems on the AP Calc Exam, particularly for visualizing functions and their derivatives.
💡Free-Response Question
A free-response question is a type of question on the AP Calc Exam that requires students to provide a complete answer, including calculations, explanations, and interpretations. These questions test not only the students' ability to perform calculations but also their understanding of the concepts and their ability to communicate their reasoning.
💡Net Rate of Change
The net rate of change is the overall rate at which a quantity changes when considering all contributing factors. It is calculated by summing the individual rates of change that are in the same direction and subtracting those that are in the opposite direction. In the video, the net rate of change is used to determine when the number of cans on the conveyor belt is increasing, by comparing the rate of cans being added (P(t)) to the rate of cans being removed (R(t)).
💡Accumulation Function
The accumulation function is a concept in calculus that relates the rate of change of a quantity to its actual amount over time. It is used to find the total amount of a quantity at a given time by integrating the rate function. In the video, the accumulation function is used to calculate the number of cans on the conveyor belt at a specific time by integrating the rate in and rate out functions and adjusting for the initial amount.
💡Average Value
The average value of a function over an interval is the mean of the function's values on that interval. It can be found by dividing the definite integral of the function over the interval by the length of the interval. In the context of the video, the average value is used to determine the average rate at which cans are removed from the conveyor belt between two time points.
Highlights

Tony Record and Bryan Passwater discuss popular problem types for the AP Calculus BC exam, focusing on rate in and rate out problems.

The problem involves a factory machine placing labels on vegetable cans and the rate at which workers remove these cans.

The rate functions P(t) and R(t) represent the number of cans placed and removed per second, respectively.

At time t equals zero, there are 53 cans on the conveyor belt.

The importance of storing rate functions in the calculator for solving active questions is emphasized.

Part A of the problem asks to find P'(7) and explain its meaning in context, using the acronym NUT (Nouns Units Time).

The net rate of change of cans on the conveyor belt is determined by P(t) - R(t), where a positive value indicates an increasing number of cans.

The intervals of time when the number of cans are increasing are found by comparing the graphs of P(t) and R(t).

Part B asks to determine when the number of cans on the conveyor belt is increasing and to provide a reason for the answer.

Part C involves calculating the average value of the rate at which cans are removed from the conveyor belt over a specific time interval.

The definite integral of R(t) from 10 to 40 is found to have an average value of 4.170 cans per second.

Part D requires finding the number of cans on the conveyor belt at time 20, using the accumulation function concept.

The strategy for solving Part D involves using the initial amount of cans, integrating the rate in, and subtracting the integral of the rate out.

The final answer for the number of cans on the conveyor belt at time 20 is approximately 241, after rounding to the nearest whole number.

The video series provides additional resources for understanding calculator-active questions and other AP Calculus topics.

The importance of attention to detail, such as rounding to the nearest whole number, is stressed for maximizing points on the AP exam.

The session concludes with encouragement to continue studying and utilizing the available resources for AP Calculus preparation.

Transcripts

Browse More Related Video

4 | FRQ (No Calculator) | Practice Sessions | AP Calculus BC

2 | FRQ (Calculator Active) | Practice Sessions | AP Calculus AB

1 | MCQ | Practice Sessions | AP Calculus BC

3 | FRQ (No Calculator) | Practice Sessions | AP Calculus BC

2023 AP Calculus AB & BC Free Response Question #1

2022 Live Review 6 | AP Calculus BC | Working with Parametric Equations and Vectors

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusRate ProblemsExam PrepConveyor BeltIntegrationDerivativesMath EducationCollege BoardStudy SkillsWebinar