Using the Mean Value Theorem with a Table of Values
TLDRThe video script discusses the Mean Value Theorem (MVT) and its application in solving problems involving tables of values, a common task in AP Calculus. The MVT states that if a function is continuous on a closed interval and differentiable on the open interval, there exists a point where the derivative equals the average rate of change over that interval. The video emphasizes that differentiability implies continuity, which is crucial for using the Intermediate Value Theorem (IVT). Two problem-solving approaches are presented: the first involves showing a sign change in the derivative over two intervals, using MVT twice and then IVT to conclude that the derivative equals zero for some value within the intervals. The second approach simplifies the problem by identifying the same y-values in the table, which allows for a quicker application of MVT. The video concludes with a tip to always look for identical y-values first, as it can significantly streamline the problem-solving process.
Takeaways
- 📚 The Mean Value Theorem (MVT) is a fundamental concept in calculus, used to solve problems involving tables of values and to understand the relationship between the derivative and the original function.
- 📈 To apply MVT, a function must be continuous on a closed interval and differentiable on the corresponding open interval, meaning the function includes its endpoints for continuity but not for differentiability.
- 🔄 MVT states that for a function that meets the criteria, there exists at least one point 'C' in the open interval where the derivative of the function (f'(C)) equals the average rate of change over the interval ((f(B) - f(A)) / (B - A)).
- 📌 Differentiability implies continuity, which is often a given or implied condition in calculus problems involving derivatives.
- 📉 If a function is twice differentiable, it means the first and second derivatives both exist and are continuous, allowing for the application of additional theorems like the Intermediate Value Theorem (IVT).
- 🔢 The IVT is crucial for problems involving the MVT as it guarantees a value exists within a continuous interval where a certain condition is met, such as a sign change in the derivative.
- ➗ When solving table problems using MVT, it's common to apply the theorem more than once to different intervals to find the necessary points where the derivative equals zero or has a specific value.
- 🔑 A useful strategy for solving table problems is to first look for the same y-values in the table before applying MVT, as this can simplify the problem significantly.
- 📉 In the given example, by showing that the derivative changes sign between two intervals, the IVT is used to conclude that there must be a point where the derivative is zero.
- 📋 The script demonstrates two types of problems: one where the function is twice differentiable and another where it is only once differentiable, illustrating the flexibility of the MVT and IVT in solving calculus problems.
- ✅ Always remember to verify that the function meets the conditions of the MVT before applying it to ensure the solution is valid.
Q & A
What is the Mean Value Theorem?
-The Mean Value Theorem states that if a function f(x) is continuous on the closed interval from A to B and differentiable on the open interval from A to B, then there exists a value C in the open interval from A to B such that the derivative of the function at C (f'(C)) is equal to the average rate of change of the function over the interval, which is (f(B) - f(A)) / (B - A).
What are the two conditions required for the Mean Value Theorem to apply?
-The two conditions are: 1) the function must be continuous on the closed interval from A to B, and 2) the function must be differentiable on the open interval from A to B.
What does it mean for a function to be twice differentiable?
-A function is twice differentiable if it is differentiable (and therefore continuous) and its derivative is also differentiable (and therefore continuous).
Why is continuity important when applying the Mean Value Theorem?
-Continuity is important because it allows the use of the Intermediate Value Theorem, which is often utilized in conjunction with the Mean Value Theorem to solve problems.
How does the Intermediate Value Theorem help in solving problems involving the Mean Value Theorem?
-The Intermediate Value Theorem guarantees that if a function is continuous on a closed interval and takes on certain values at the endpoints, then it takes on all values between those endpoints. This helps in identifying the existence of a value C where the derivative equals zero or has a sign change.
What is the significance of differentiability implying continuity?
-Differentiability implying continuity is significant because it means that if a function is differentiable on an interval, it is also continuous on that interval, which is a requirement for the Mean Value Theorem to apply.
In the first problem, why is the goal to show that f'(x) equals 0 for some x?
-The goal is to demonstrate the existence of a point where the derivative of the function is zero, which indicates a point of inflection or a change in the direction of the function's rate of change.
How does the speaker use the Mean Value Theorem to show that f'(x) must equal 0 for some x between 2 and 9?
-The speaker applies the Mean Value Theorem twice on different intervals (2 to 5 and 5 to 9) to show that the derivative of the function changes sign, indicating a point where the derivative is zero by invoking the Intermediate Value Theorem.
What is the speaker's hint or tip for solving table problems involving the Mean Value Theorem?
-The speaker suggests always looking for the same y-values in the table before using the Mean Value Theorem, as this can simplify the problem and reduce the length of the solution significantly.
In the second problem, why does the speaker choose to use the Mean Value Theorem on the interval from -2 to 6?
-The speaker chooses this interval because the function values at the endpoints of this interval are the same (f(-2) = f(6)), which directly leads to a conclusion that the average rate of change, and thus the derivative at some point C in this interval, must be zero.
What is the key takeaway from the video regarding the application of the Mean Value Theorem in solving problems?
-The key takeaway is the strategic use of the Mean Value Theorem in conjunction with the Intermediate Value Theorem to identify points of interest, such as where the derivative is zero or changes sign, by analyzing the function's behavior over specified intervals.
Outlines
📚 Introduction to Mean Value Theorem and Table Problems
The first paragraph introduces the Mean Value Theorem (MVT) and its application in solving problems that involve tables of values, which is a common task in AP Calculus. The MVT states that if a function f(x) is continuous on a closed interval [A, B] and differentiable on the open interval (A, B), then there exists some value C in the interval (A, B) such that the derivative of the function at C is equal to the average rate of change of the function over the interval [A, B]. The paragraph also emphasizes the importance of understanding that differentiability implies continuity and the role of the intermediate value theorem in solving these problems. The speaker then presents two types of problems: one where the function is twice differentiable and another where it is just differentiable, using the MVT to solve for a point where the derivative equals zero.
🔍 Applying Mean Value Theorem with Given Values
The second paragraph demonstrates a different approach to solving problems using the MVT when given specific values of a function at certain points. The speaker explains that if a function is differentiable, it is also continuous, satisfying the conditions for the MVT. The paragraph shows how to use the MVT to find an interval where the derivative of the function is zero, by looking for a sign change in the derivative's values across different intervals. The speaker also provides a tip for solving these problems more efficiently by first checking for the same y-values in the table before applying the MVT, which can simplify the process significantly.
Mindmap
Keywords
💡Mean Value Theorem
💡Continuous
💡Differentiable
💡Interval
💡Derivative
💡Table of Values
💡Intermediate Value Theorem
💡Slope of the Tangent Line
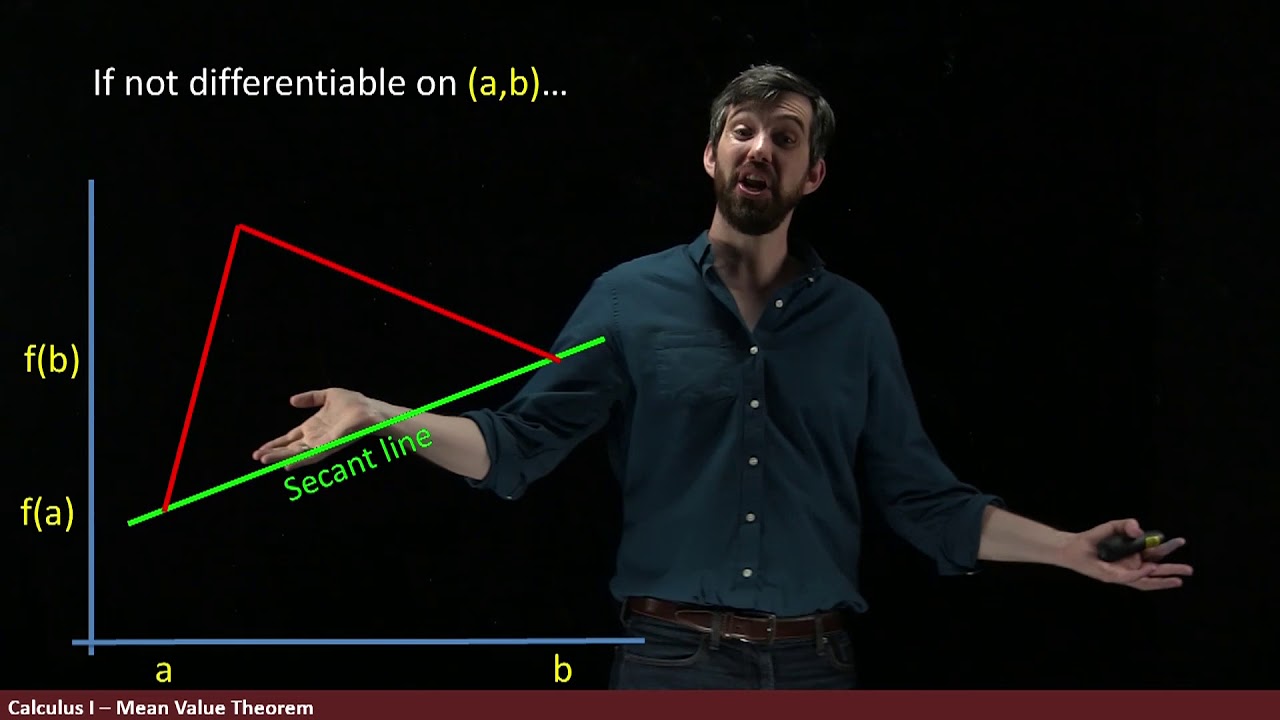
💡Secant Line
💡Twice Differentiable
💡Sign Change
Highlights
Mean Value Theorem is introduced as a tool for solving problems involving tables of values in AP calculus.
Two requirements for the Mean Value Theorem: continuity on a closed interval and differentiability on an open interval.
Differentiability implies continuity, which is often a given condition in problems.
Twice differentiable functions are both differentiable and continuous, with their derivatives also being continuous.
Continuity allows for the use of the Intermediate Value Theorem, which is frequently utilized in solving problems.
The Mean Value Theorem is applied twice in the first problem to show a sign change in the derivative.
The Intermediate Value Theorem is invoked to conclude that there exists some X where the derivative equals zero.
In the second problem, the Mean Value Theorem is used to find an interval where the slope of the secant line is zero.
The approach of looking for the same Y values before applying the Mean Value Theorem can simplify the problem-solving process.
The Mean Value Theorem is applicable on every interval where the function is differentiable.
The concept of the slope of the tangent line equaling the slope of the secant line through endpoints is central to the Mean Value Theorem.
The importance of understanding the implications of a function being twice differentiable for problem-solving.
Demonstration of using the Mean Value Theorem to find an unknown value of C within a given interval.
The video provides a step-by-step application of the Mean Value Theorem in the context of a table of values.
Highlighting the practical application of the Intermediate Value Theorem in conjunction with the Mean Value Theorem.
The strategy of using the Mean Value Theorem to identify a value where the derivative equals zero is demonstrated.
Illustration of an alternative approach to solving problems using the Mean Value Theorem when the same Y values are present.
The video concludes with a tip to always look for the same Y values first, which can significantly reduce the complexity of the problem.
Transcripts
Browse More Related Video

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

Mean Value Theorem and Rolle's Theorem
The MEAN Value Theorem is Actually Very Nice

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy
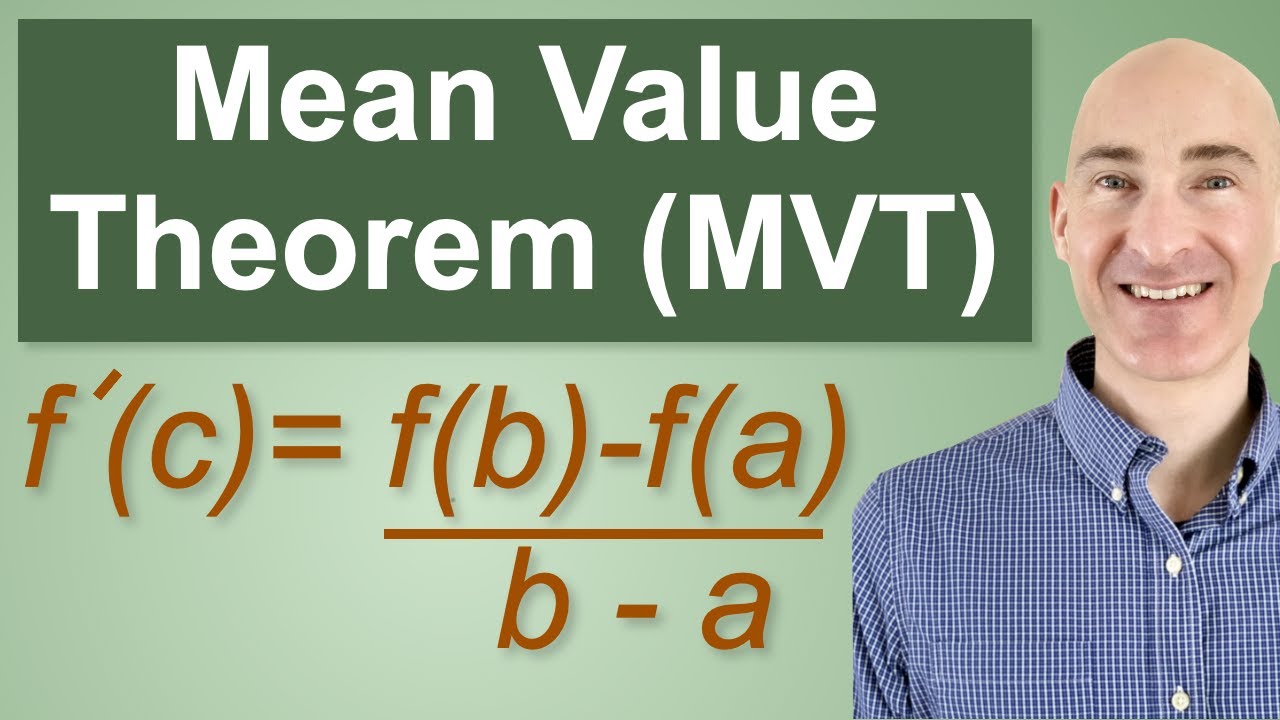
Mean Value Theorem with Example
5.0 / 5 (0 votes)
Thanks for rating: