Rotational Power, Work, Energy, Torque & Moment of Inertia - Physics Problems
TLDRThe video script delves into the concepts of rotational power, work, energy, and torque, explaining how work done on an object is calculated as the force times the displacement. It introduces the arc length formula and its relation to linear displacement and radius, and how these translate into the physics of torque. The script then draws parallels between linear and rotational work, highlighting the role of torque and angular displacement. It further explores the relationship between work, kinetic energy, and power, and how power is the rate of energy transfer, measured in watts. The video also explains the calculation of energy in terms of power and time, and the conversion between different units of power. Finally, it applies these principles to a problem involving a 15 kg disc accelerating from rest to 40 radians per second in 5 seconds, calculating the work and average power required for this acceleration using both rotational kinetic energy and torque methods.
Takeaways
- 📐 The work done on an object is calculated as the force acting on the object times the object's displacement.
- 🔄 Arc length (s) in a circle can be represented as the product of the angle (theta) and the radius (r), which is also applicable to linear displacement (d) in physics.
- ⚙️ Torque is the force times the moment arm or lever arm, which in the case of a circle, becomes the radius.
- 🔩 Rotational work is the product of torque and angular displacement, which is analogous to linear work being the product of force and displacement.
- ⚡ Work can also be calculated as the change in kinetic energy, both translational and rotational.
- 🌀 Rotational kinetic energy is given by one-half times the moment of inertia times the square of the angular speed (omega squared).
- ⏱️ Power is the rate at which work is done, calculated as work divided by time, and in the rotational context, it is torque times angular velocity.
- ⚖️ One watt represents the transfer of one joule of energy per second, and power indicates how fast energy can be transferred.
- 🔢 Energy can be calculated by multiplying power by time, with additional units like horsepower (746 watts) and kilowatt (1000 watts).
- 🤔 Torque is also equal to the product of the moment of inertia and angular acceleration, as per Newton's second law of rotation.
- 📉 To calculate the work required to accelerate a rotating object, you can use the change in rotational kinetic energy or the product of torque and angular displacement.
Q & A
What is the definition of work done on an object in physics?
-In physics, the work done on an object is equal to the force acting on the object times the object's displacement.
How is arc length related to angular displacement and radius in the context of circular motion?
-The arc length (s) is equal to the angle (theta) times the radius (r) of the circle. In physics, arc length (s) can be replaced with the linear displacement (d), where theta is the angular displacement and r is the radius of the circle.
What is torque and how is it related to force and radius?
-Torque is the force times the moment arm, or in the case of a circle or wheel, the force times the radius of the circle. It is the rotational equivalent of force.
How is rotational work calculated?
-Rotational work is calculated as the torque times the angular displacement.
What is the relationship between work, torque, and angular displacement?
-Just as work is equal to force times linear displacement, rotational work is equal to torque times angular displacement.
How is work related to kinetic energy?
-Work is equal to the change in kinetic energy, which is the final kinetic energy minus the initial kinetic energy.
What is the formula for translational kinetic energy?
-Translational kinetic energy is given by the formula one half (1/2) times mass (m) times velocity squared (v^2).
How is rotational kinetic energy calculated?
-Rotational kinetic energy is calculated as one-half (1/2) times the moment of inertia times the square of the angular speed (omega squared, ω^2).
What is power and how is it related to work and time?
-Power is the rate at which work is done, measured in watts, and is calculated as work divided by time.
What is the rotational equivalent of power?
-The rotational equivalent of power is torque times angular velocity.
How is energy calculated in terms of power and time?
-Energy is calculated by multiplying power (in watts) by time (in seconds).
What is the relationship between torque, inertia, and angular acceleration?
-Torque is equal to the product of inertia and angular acceleration, which is analogous to Newton's second law of rotation where force is mass times acceleration.
How can you calculate the work required to accelerate a disc from rest to a certain angular speed?
-The work required to accelerate a disc from rest to a certain angular speed can be calculated using the change in rotational kinetic energy, which is the final rotational kinetic energy minus the initial rotational kinetic energy (which is zero if the disc starts from rest).
How do you calculate the average power exerted on a rotating disc?
-The average power exerted on a rotating disc can be calculated by dividing the total work done on the disc by the time period over which the work was done, or by multiplying the torque by the average angular velocity.
Outlines
🔧 Rotational Power and Energy Concepts
This paragraph introduces the fundamental concepts of rotational power, work, energy, and torque. It explains how work done on an object is calculated using force and displacement, and how this translates into rotational work using torque and angular displacement. The paragraph also covers the relationship between force, linear displacement, and torque, as well as the calculation of work in terms of changes in kinetic energy, both translational and rotational. It concludes with the definition of power as work divided by time, and its rotational equivalent, torque times angular velocity, emphasizing the importance of power in measuring the rate of energy transfer.
📐 Calculating Work and Power for a Rotating Disk
The second paragraph presents a problem involving a 15-kilogram disc with a 2-meter radius that accelerates from rest to 40 radians per second in five seconds. It outlines two methods for calculating the work required to achieve this acceleration. The first method involves calculating the change in rotational kinetic energy, using the disc's mass and radius to find its moment of inertia, and then using the final angular speed to calculate the work done. The second method involves using torque and angular displacement, where torque is calculated using the disc's moment of inertia and angular acceleration, and angular displacement is found using the average angular speed over the time period. Both methods yield the same result of 24,000 joules of work done. The paragraph also touches on the calculation of average power exerted on the disk by dividing the work by the time period.
💡 Power Calculation and Angular Acceleration
The third paragraph focuses on calculating the average power exerted on the rotating disk. It begins by using the work done and the time period to find the power in watts. Then, it explores an alternative method using the torque and angular speed, where the average angular speed is determined by averaging the initial and final angular speeds. The torque, previously calculated, is then multiplied by this average speed to find the average power. The paragraph confirms that both methods yield the same result of 4,800 watts, or 4.8 kilowatts, highlighting the consistency in the calculation of power in rotational dynamics.
Mindmap
Keywords
💡Rotational Power
💡Work-Energy Principle
💡Torque
💡Angular Displacement
💡Inertia
💡Angular Acceleration
💡Power
💡Kinetic Energy
💡Linear Velocity
💡Angular Velocity
💡Newton's Second Law of Rotation
Highlights
The video focuses on rotational power, work, energy, and torque.
Work done on an object is equal to force times displacement.
Arc length (s) is equal to the angle (theta) times the radius in physics.
Torque is the force times the moment arm, which is the radius of the circle when force is applied.
Rotational work is calculated as torque times angular displacement.
Work is also the change in kinetic energy, both translational and rotational.
Rotational kinetic energy is given by one-half times inertia times the square of angular speed (omega squared).
Power is work divided by time and is the rate of energy transfer.
Power in rotation is torque times angular velocity.
A 500 watt motor converts 500 joules of electrical energy to mechanical energy every second.
Energy is calculated as power multiplied by time.
One horsepower is equivalent to 746 watts, and one kilowatt is a thousand watts.
Torque is equal to inertia times alpha, following Newton's second law of rotation.
Inertia and mass resist changes in motion, and torque is the rotational equivalent of force.
A problem is presented involving a 15 kg disc with a 2 m radius accelerating to 40 radians per second in 5 seconds.
The work required to accelerate the disc is calculated using the change in rotational kinetic energy.
The inertia of the disc is calculated as one-half times the mass times the radius squared.
The work done to accelerate the disc is found to be 24,000 joules.
The average power exerted on the disc is calculated by dividing the work by the time period.
Power can also be calculated using torque times the average angular speed.
Transcripts
Browse More Related Video
Work and Kinetic Energy - Physics
AP Physics 1 review of Energy and Work | Physics | Khan Academy
Work, Energy, & Power - Formulas and Equations - College Physics

AP Physics 1 review of Torque and Angular momentum | Physics | Khan Academy
Work, Energy, and Power - Basic Introduction
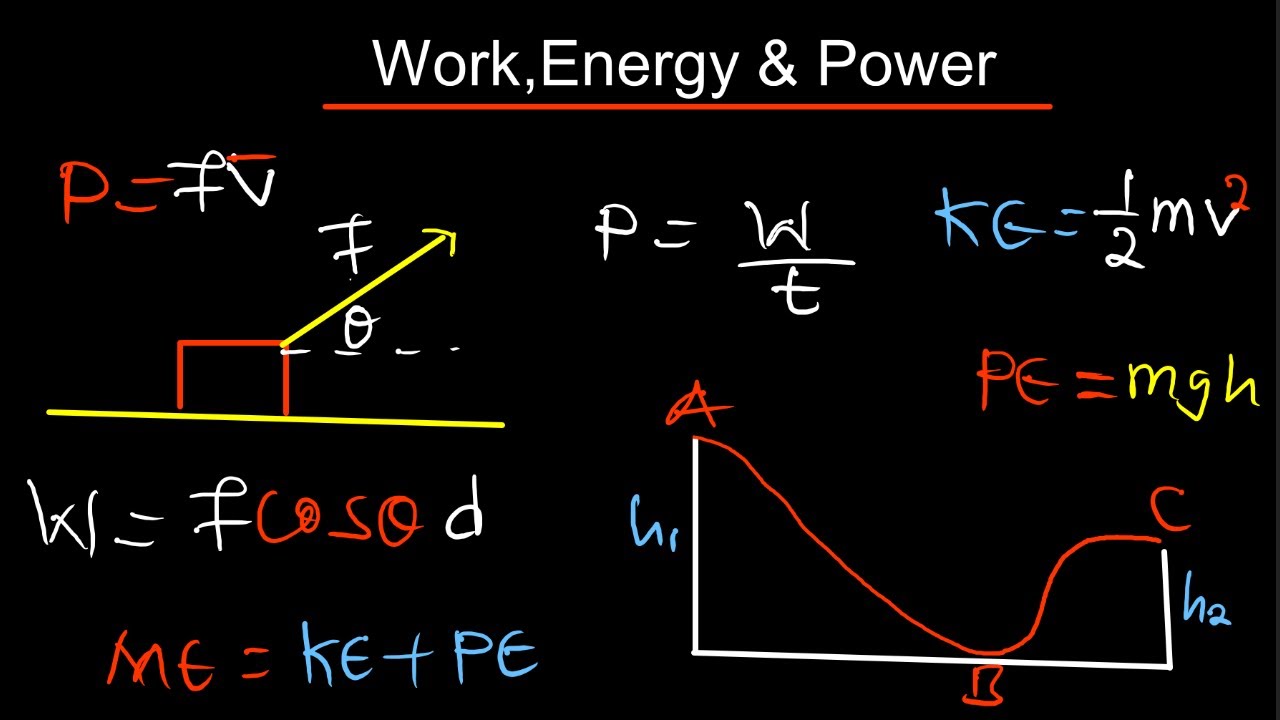
Work Energy and Power Full Topic
5.0 / 5 (0 votes)
Thanks for rating: