Thales's Theorem
TLDRIn this engaging video, Michael Stephens of 'Michael Stevens Living' explores the fascinating world of circles and their centers. He starts by using a compass to create a circle and identifies its center by the tiny divot it leaves. Then, he demonstrates an alternative method using a circular object from the 'Curiosity Box Best of Edition' to trace a circle and employs Thales' Theorem to locate its center. With the help of the 'Elements Book' from the same box, he uses geometric principles to draw diameters and find the circle's true center. The video dives into the axioms and properties of geometry, explaining congruence, isometries, and the sum of angles in a triangle. It concludes with a rigorous proof of Thales' Theorem, which states that the angle inscribed in a semicircle is always a right angle, and how it applies to finding the diameter of a circle. The episode is both educational and entertaining, offering viewers a deep dive into geometric theorems and their practical applications.
Takeaways
- 📏 The definition of a circle is the set of all points that are the same distance from a given point, known as the center.
- 🔍 Finding the center of a circle made with a compass is straightforward because it leaves a divot at the center point.
- ✏️ If you don't use a compass, finding the circle's center can be more challenging and may require estimation or mathematical methods.
- 🔵 Utilizing a circular object, like the Cipher Wheel from the Curiosity Box, can create a circle without a clear center point.
- ️⃣ Thales' Theorem can help find the center of a circle by using a right angle inscribed within the circle.
- 📐 Thales' Theorem states that the points where two rays extended from the right angle vertex touch the circle are diametrically opposed and the line passing through them passes through the center.
- 📏 To confirm the center, draw two diameters; their intersection point is the circle's center.
- 🤔 Understanding the axioms of geometry, such as isometries (distance-preserving transformations) and congruence, is crucial for proving theorems like Thales' Theorem.
- 🧩 Congruent figures have identical corresponding measurements, but they may not be in the same location.
- 🔺 In a triangle, if two sides and the included angle are known, the triangle is uniquely determined due to the properties of space.
- 🔶 The sum of the internal angles in any triangle equals 180 degrees, which is a fundamental property used in proving geometric theorems.
- 💠 In an isosceles triangle, the angles opposite the equal sides are equal, which is key to understanding Thales' Theorem in the context of a circle.
Q & A
What is the definition of a circle according to the script?
-A circle is defined as the set of all points that are the same distance from a given point, which is its center.
How does Michael Stephens identify the center of the first circle he creates?
-Michael Stephens identifies the center of the first circle by observing a tiny divot left by the sharp point of the compass on the paper.
What tool does Michael use to find the center of a circle when he doesn't have a compass?
-Michael uses Thales' Theorem to find the center of a circle. He uses a right angle from a book to create two diameters that intersect at the circle's center.
What is Thales' Theorem and how does it help in finding the center of a circle?
-Thales' Theorem states that if a right angle is inscribed in a circle, the two points where the sides of the right angle intersect the circumference of the circle are diametrically opposed. A line through these points passes through the center of the circle.
How does Michael use the Cipher Wheel from the Curiosity Box to make a circle?
-Michael uses the Cipher Wheel by tracing around its outside edge to create a circle without a divot in the center.
What is the significance of congruent triangles in the proof of Thales' Theorem?
-Congruence of triangles is used to establish that angles opposite equal sides in a triangle are equal, which is a crucial step in the geometric proof of Thales' Theorem.
What does Michael mean by 'isosceles triangles' and how are they used in the script?
-Isosceles triangles are triangles with at least two sides of equal length. Michael uses properties of isosceles triangles to show that angles opposite equal sides are equal, which helps in proving Thales' Theorem.
How does Michael demonstrate that the sum of internal angles in any triangle sums to 180 degrees?
-Michael demonstrates this by creating a triangle with two intersecting lines and showing that the angles at the intersection point, which are equal due to corresponding angles and vertical angles being equal, sum with the third angle to make 180 degrees.
What axioms or ground rules does Michael postulate to begin the proof of Thales' Theorem?
-Michael postulates that isometries, which are transformations that preserve distances such as translation and rotation, do not change the distances and angles of a figure. He also defines congruence and uses these concepts to establish the properties of parallel lines and triangles.
Why does Michael emphasize the importance of understanding the properties of parallel lines before proving Thales' Theorem?
-Understanding the properties of parallel lines is essential because it helps establish the relationship between angles created by a transversal intersecting two parallel lines, which is a key component in the geometric proof of Thales' Theorem.
What is the final step in Michael's proof of Thales' Theorem using the circle and the diameter?
-The final step involves drawing a radius from a chosen point on the circumference to the center of the circle and then connecting this point to two other points on the circumference to form two isosceles triangles. By showing that the angles opposite the equal sides of these triangles are equal and that their sum is a right angle, Michael proves Thales' Theorem.
How does the script demonstrate the educational value of hands-on geometric constructions?
-The script demonstrates the educational value by guiding the viewer through the process of creating circles and using geometric tools to discover and prove Thales' Theorem. It emphasizes the importance of understanding geometric properties and theorems through direct engagement and manipulation of shapes.
Outlines
🎨 Circle Crafting and Center Finding Techniques
The video begins with the host, Michael Stephens, expressing his enthusiasm for creating circles and introduces a unique method for determining their centers. He uses a 'comp butt', a tool that maintains a constant distance to draw a circle and leaves a divot that indicates the circle's center. Stephens then demonstrates how to find the diameter of the circle with a straight edge. He also explores an alternative circle-making technique using a circular object from the 'Curiosity Box Best of Edition' and the Ink's Cypher Wheel. After tracing a circle without a divot, he uses Thales' theorem to locate the circle's center by creating two diameters that intersect at the center point.
📐 Thales' Theorem and Axiomatic Geometry
The host delves into the geometric principles behind Thales' theorem, starting with basic axioms about isometries and congruence. He explains that isometries are transformations that preserve distances, and congruence means that two shapes can coincide perfectly if superimposed. Stephens uses these concepts to discuss the properties of triangles and how knowing two sides and the included angle is sufficient to establish triangle congruence. He then connects these ideas to the properties of quadrilaterals and the behavior of angles created by intersecting lines, setting the stage for a deeper exploration of Thales' theorem.
🧩 Exploring Parallel Lines and Angle Congruence
Stephens continues the geometric discussion by focusing on the properties of parallel lines and a transversal intersecting them. He demonstrates that corresponding angles are equal when a transversal cuts two parallel lines. Using this fact, he shows that angles opposite each other through an intersection point are also equal. He then draws two parallel lines and a line intersecting them to form a triangle, discussing how the angles opposite the sides created by the intersection are equal. This leads to the fundamental principle that the sum of internal angles in any triangle equals 180 degrees.
🔍 Isosceles Triangles and Angle Relationships
The host constructs an isosceles triangle to explore the relationship between its angles. By bisecting the angle at the base of the triangle, he creates two congruent triangles, which allows him to conclude that the angles opposite the equal sides are also equal. This insight is crucial for understanding Thales' theorem, as it shows that angles opposite equal sides in a triangle are equal, which is a key component in proving that the points where two diameters intersect on a circle's circumference are equidistant from the center.
🛰️ Thales' Theorem: The Final Proof
Stephens concludes the video by linking the properties of isosceles triangles to Thales' theorem. He draws a circle and selects a diameter, then chooses a third point on the circumference. By connecting this point to the center of the circle, he creates a radius and two isosceles triangles. Using the principle that angles opposite equal sides in a triangle are equal, he shows that the sum of these angles must be 90 degrees, thus proving that an inscribed angle formed by a diameter and a chord on a circle is a right angle. This final demonstration reinforces Thales' theorem and the geometric beauty of circles.
Mindmap
Keywords
💡Circle
💡Center of a Circle
💡Compass
💡Thales' Theorem
💡Diameter
💡Isometry
💡Congruence
💡Parallel Lines
💡Inscribed Angle
💡Isosceles Triangle
💡Axiom
Highlights
The video demonstrates how to make decorative circles and find their centers using a compass and other methods.
A circle is defined as the set of all points that are the same distance from a given point, known as the center.
The presenter uses a compass to draw a circle and identify its center by the tiny divot it leaves behind.
Thales' Theorem is introduced as a method to find the center of a circle without a divot, using a right angle.
The Elements Book from the Curiosity Box is used to apply Thales' Theorem, showcasing its sharp right angle.
The presenter discusses the concept of isometries and congruence in geometry, essential for understanding Thales' Theorem.
The video explains the properties of parallel lines and how they relate to the angles formed by a transversal intersecting them.
The internal angles of a triangle always sum up to 180 degrees, a key principle used in proving Thales' Theorem.
An isosceles triangle, having two sides of equal length, is used to demonstrate that angles opposite equal sides are also equal.
The presenter draws a circle with a known diameter to set up the conditions for applying Thales' Theorem.
By connecting points on the circumference with the center, two isosceles triangles are formed, crucial for the theorem's proof.
The angles of the inscribed triangle are shown to be complementary, summing up to a right angle, which validates Thales' Theorem.
The video concludes by reiterating the importance of Thales' Theorem in geometry and its practical application in finding circle centers.
The use of the Cipher Wheel from the Curiosity Box to draw a circle without a divot and estimate its center is demonstrated.
The presenter uses a purple marker to guess the circle's center, highlighting the imprecision of estimating without tools.
A detailed explanation of how to use Thales' Theorem to find the true center of a circle is provided.
The construction of an isosceles triangle and the properties of its angles are discussed in the context of the theorem.
The video includes a comprehensive explanation of the axioms and postulates that lead to the proof of Thales' Theorem.
Transcripts
Browse More Related Video
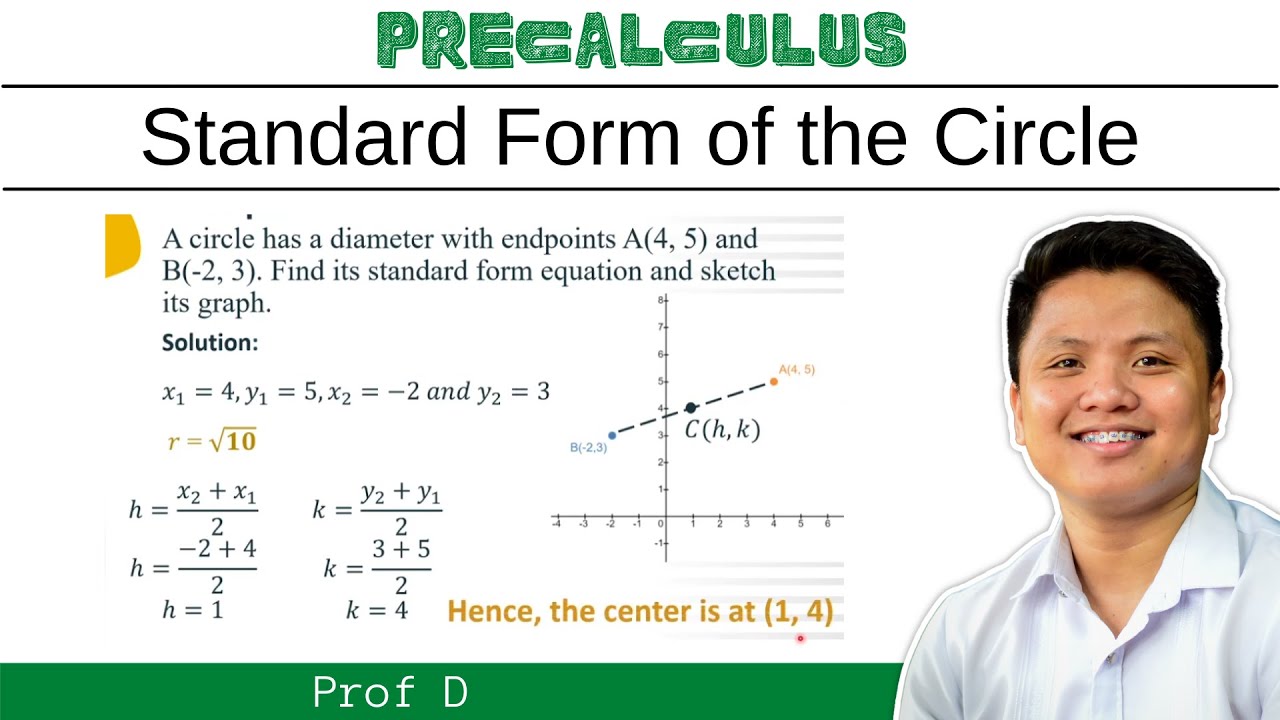
How to find the standard form of the circle | Circle | Conic Section | Pre-Calculus

PROBLEM SOLVING INVOLVING CIRCLES
Math Antics - Circles, What Is PI?
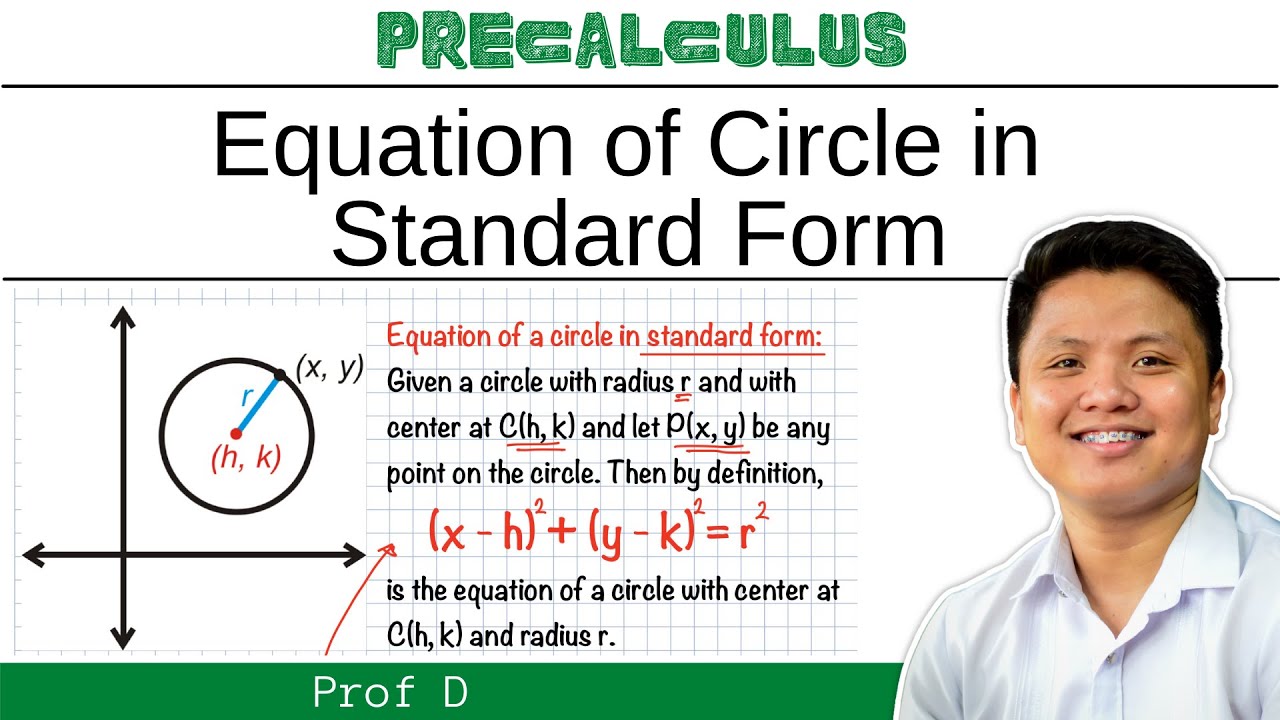
EQUATION OF CIRCLE IN STANDARD FORM | PROF D
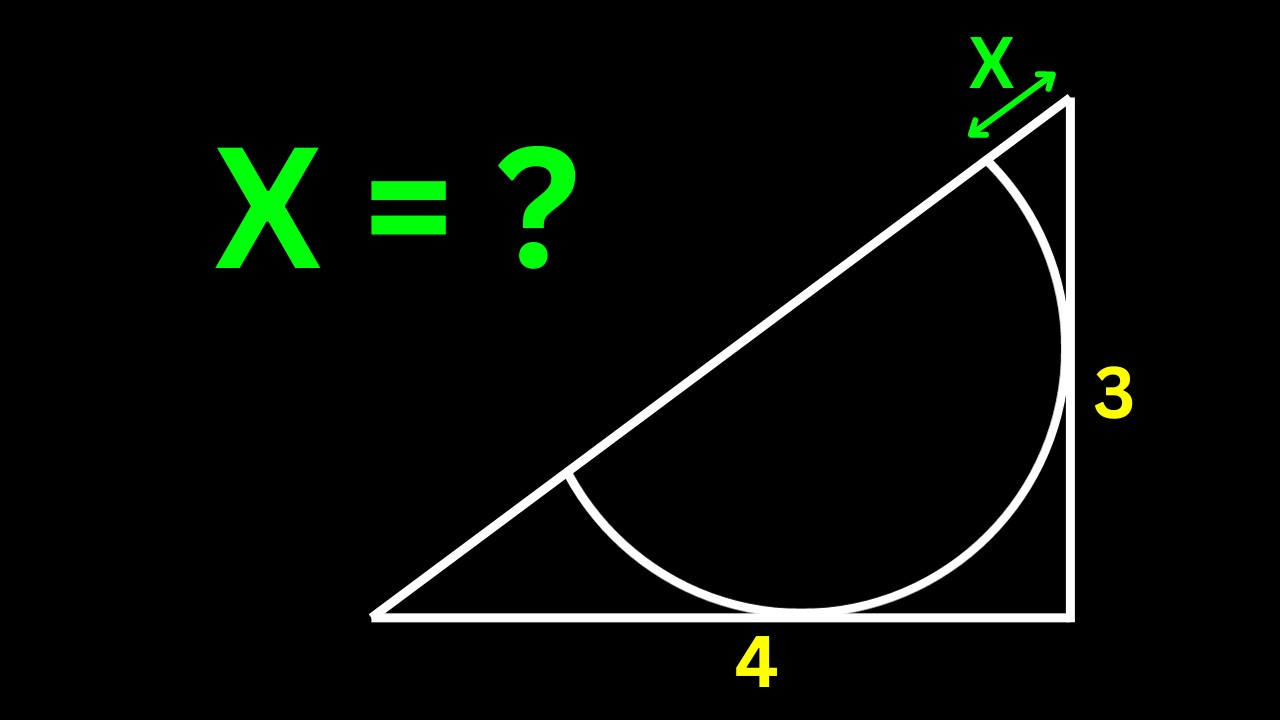
Canada Math Olympiad Problem | Best Math Olympiad Problems | Geometry
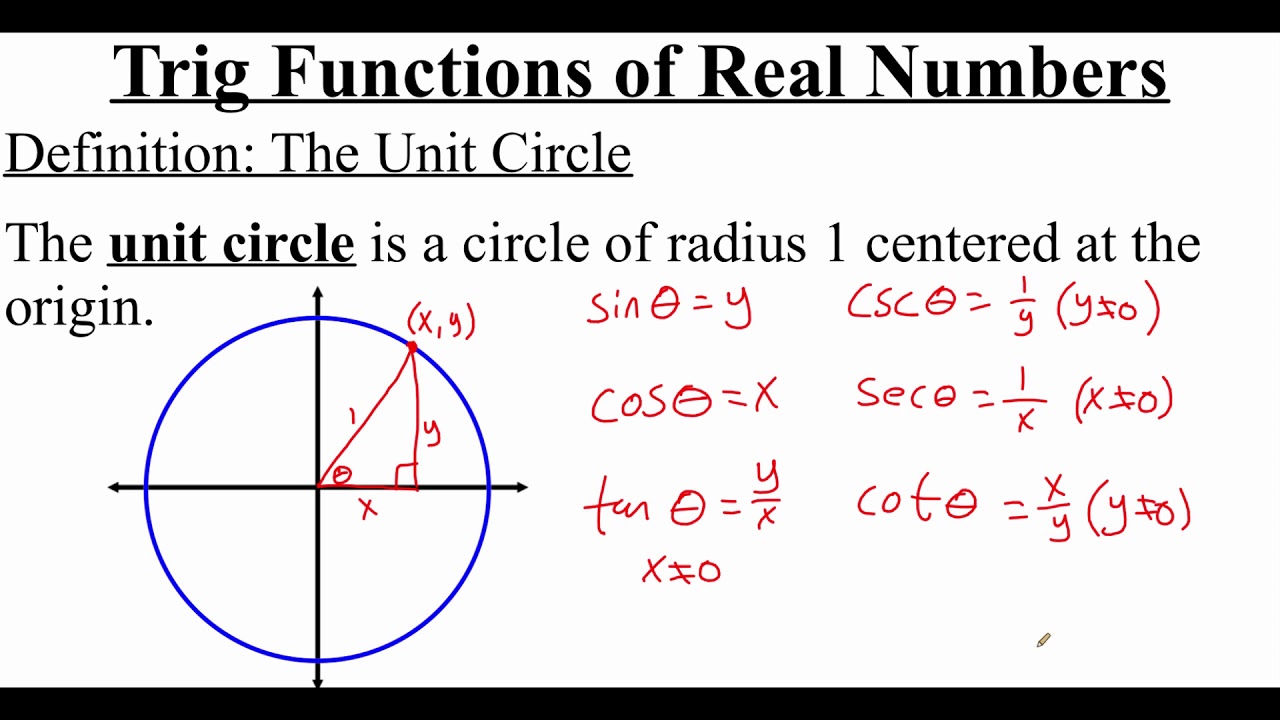
4.3.4 Trigonometric Functions of Real Numbers
5.0 / 5 (0 votes)
Thanks for rating: