Business and Social Science Calculus Final Exam Review
TLDRThe video script is a comprehensive review session for a Business Calculus course for Social Sciences, focusing on key concepts such as limits, derivatives, and integration. The instructor covers various methods for evaluating limits algebraically and graphically, including indeterminate forms and direct substitution. The session also explores derivatives, emphasizing the use of the difference quotient, product rule, quotient rule, and chain rule. The instructor demonstrates how to find the equation of a tangent line to a curve and how to apply derivatives to determine increasing/decreasing functions, local maxima/minima, and inflection points. Integration techniques are also discussed, with examples of indefinite and definite integrals, and the use of u-substitution. The summary concludes with a reminder about the final exam and an encouragement for a successful semester.
Takeaways
- 📚 The first topic covered in the course was limits, which should be understood both algebraically and graphically.
- 🔢 When dealing with limits, it's important to first try direct substitution and then manipulate the function if an indeterminate form like 0/0 is encountered.
- 🏞️ Graphs can be used to determine limits by observing the behavior of the function as it approaches certain values, including one-sided limits.
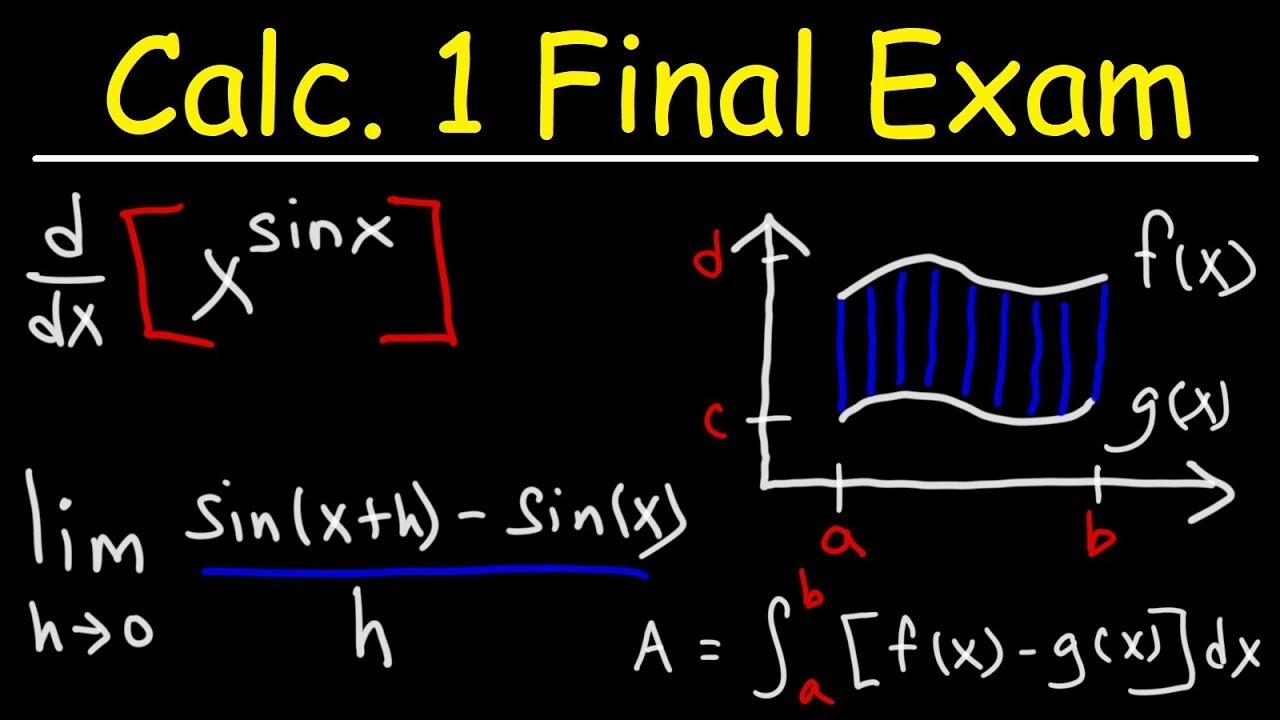
- 📈 Derivatives were introduced using the difference quotient and limits, with the formal definition involving the limit as h approaches 0.
- ✏️ Techniques for finding derivatives include the product rule, quotient rule, chain rule, and understanding the derivatives of exponential and logarithmic functions.
- 🔍 Implicit differentiation is used when the function is given implicitly, treating y as a function of x and applying the chain rule.
- 📌 Critical numbers are found by setting the derivative equal to zero and are used to determine where a function is increasing or decreasing.
- 📉 The second derivative can be used to find inflection points and determine the concavity of a function.
- 🧮 Integration techniques include finding antiderivatives, using substitution, and handling definite integrals with bounds.
- 🔑 Special integration formulas were discussed, such as the integral of e^(ax) and the integral of 1/x, which is a natural logarithm.
- ⌛️ The importance of practicing different methods of integration and differentiation was emphasized for understanding calculus concepts.
Q & A
What is the first topic covered in the course on Business Calculus for Social Sciences?
-The first topic covered in the course is limits, and students should be able to deal with limits both algebraically and graphically.
How do you algebraically evaluate the limit as X approaches a certain value?
-You substitute the value of X into the function and drop the limit sign. If the result is a determinate value, then that's the value of the limit.
What is an indeterminate form when evaluating limits?
-An indeterminate form, such as 0/0, occurs when direct substitution of the limit's value results in a form that does not provide information about the limit, and further manipulation of the function is required.
How can you deal with an indeterminate form like 0/0 when evaluating a limit?
-You can deal with an indeterminate form by factoring out common terms, applying special limit rules, or using L'Hôpital's rule to simplify the expression into a determinate form.
What is the process to find the limit of a function as X approaches a certain value, graphically?
-You look at the graph and follow the path of the function as it approaches the value from the left and right. The limit exists if the left and right values are the same and equal to the function's value at that point if it exists.
How do you find the equation of the tangent line to a curve at a given point using derivatives?
-You find the derivative of the function to get the slope of the tangent line, then plug the given x-value into the original function to find the y-value, which gives you a point on the tangent line. Using the point-slope form of a line, you can then write the equation of the tangent line.
What is the derivative of a function and how is it used?
-The derivative of a function gives the rate of change of the function with respect to its variable. It is used to find the slope of the tangent line to the function at a given point, to analyze the critical points of the function, and to determine the intervals of increase and decrease.
What is the method to find the critical points of a function?
-To find the critical points of a function, you take the derivative of the function and set it equal to zero. The solutions to this equation are the critical points.
How do you determine the intervals of increase and decrease for a function?
-After finding the critical points and the endpoints of the domain, you create a number line and test intervals between these points by plugging in values into the first derivative. If the derivative is positive, the function is increasing; if negative, it is decreasing.
What is the difference between a local maximum/minimum and an absolute maximum/minimum?
-A local maximum or minimum is a point where the function's value is higher or lower than all other points in an immediate neighborhood. An absolute maximum or minimum is a point where the function's value is the highest or lowest over its entire domain.
How do you find the inflection points of a function?
-To find the inflection points, you take the second derivative of the function and set it equal to zero. The solutions to this equation are the potential inflection points. You then test the intervals around these points by plugging in values into the second derivative. If the second derivative changes sign, an inflection point exists.
What is the process to solve an indefinite integral?
-To solve an indefinite integral, you find the antiderivative of the integrand, which is the function that, when differentiated, yields the integrand. You add a constant of integration (C) at the end since the antiderivative is only determined up to a constant.
How do you evaluate a definite integral?
-To evaluate a definite integral, you find the antiderivative of the integrand and then evaluate it at the upper and lower bounds of the integral. The value of the definite integral is the difference between these two evaluations, without adding a constant of integration.
What is the method used for integration by substitution, particularly when dealing with integrals of the form involving exponential functions?
-Integration by substitution is used to transform an integral that is difficult to compute directly into a simpler form. For exponential functions, you often choose the argument of the exponential as the substitution variable (u), then rewrite the integral in terms of u, and finally change the bounds of integration to match the new variable.
How do you integrate a function involving a radical in the denominator?
-To integrate a function with a radical in the denominator, you can use substitution to transform the integral into a more manageable form. You choose a substitution that simplifies the radical, transform the integral, and then solve the resulting integral using standard techniques.
Outlines
📚 Understanding Limits in Business Calculus
The first paragraph introduces the topic of limits within the context of business calculus. It emphasizes the importance of understanding limits both algebraically and graphically. The instructor provides an example of evaluating a limit algebraically by substituting the value of X into the function and dropping the limit sign. The process involves simplifying the expression using properties of exponents and radicals. The paragraph also touches on indeterminate forms, which require further manipulation of the function to evaluate the limit.
📝 Factoring and Simplifying Limits
The second paragraph delves into techniques for addressing indeterminate forms that arise when evaluating limits. It discusses factoring out the greatest common factor and canceling like terms to simplify the expression. The paragraph also covers handling complex fractions by multiplying by the least common denominator (LCD) to eliminate them. An example is provided to illustrate the process of simplifying a limit involving division by zero and how to resolve it through factorization and direct substitution.
📈 Analyzing Graphs to Determine Limits
The third paragraph shifts the focus to understanding limits by analyzing the graph of a function. It describes how to find limits as X approaches a certain value from the left or right and how to identify points on the graph, such as vertical asymptotes and solid/open circles. The instructor demonstrates how to determine the limit at a given point by observing the behavior of the graph as it approaches that point, including the use of arrows to indicate the direction of无限大 (infinity).
🔍 Examining Continuity and End Behaviors
The fourth paragraph discusses the concept of continuity in functions and how to determine if a function is continuous at a particular point using limits. It also addresses how to find the value of the function at a specific point and the existence of limits from both the left and right. Additionally, the paragraph explores the end behaviors of a graph, explaining how to identify horizontal asymptotes and the general trend of the function as X approaches positive or negative infinity.
🧮 Derivatives and Their Applications
The fifth paragraph introduces the concept of derivatives, starting with the difference quotient and the formal definition involving limits. It illustrates how to find the derivative of a function and manipulate expressions to avoid complex fractions. The paragraph also explains how to find the equation of a tangent line to a curve at a specific point by using the derivative to determine the slope and plugging in the point to find the y-value.
🔢 Techniques for Calculating Derivatives
The sixth paragraph covers various techniques and rules for calculating derivatives, such as the product rule, quotient rule, and chain rule. It also discusses the process of taking derivatives of exponential and logarithmic functions. The paragraph provides examples of how to apply these rules to simplify the process of finding derivatives, including the use of properties of exponents and logarithms to streamline calculations.
📉 Using Derivatives to Investigate Function Behavior
The seventh paragraph explains how to use derivatives to analyze the behavior of a function, such as determining intervals of increase and decrease, as well as locating local maxima and minima. It describes the process of finding critical numbers by setting the derivative equal to zero and analyzing the sign of the derivative to understand the slope of the function. The paragraph also demonstrates how to calculate the second derivative to find inflection points and determine concavity.
🧹 Integration by Distribution and Substitution
The eighth paragraph focuses on integration techniques, such as distributing the integrand and using substitution methods. It provides examples of integrating functions involving polynomials and exponentials, as well as handling cases where the integrand includes a radical. The paragraph explains how to apply u-substitution to transform the integral into a more manageable form and how to reverse the substitution to find the final answer.
📌 Evaluating Definite Integrals
The ninth paragraph discusses the evaluation of definite integrals, which involve integrating over a specified interval. It demonstrates how to find the antiderivative of the integrand and then apply the fundamental theorem of calculus by evaluating the antiderivative at the upper and lower limits of the interval. The paragraph also shows how to handle cases where substitution is required during the integration process.
📝 Summary of the Course and Final Exam Information
The final paragraph summarizes the topics covered in the course, including limits, derivatives, and integration, and provides information about the final exam. It emphasizes the importance of understanding these concepts and offers guidance on what to expect in the final assessment. The instructor also provides instructions for students who may have scheduling conflicts with the final exam and expresses gratitude for the semester.
Mindmap
Keywords
💡Limits
💡Derivatives
💡Integrals
💡Continuous Functions
💡Tangent Line
💡Chain Rule
💡Product and Quotient Rule
💡Exponential and Logarithmic Functions
💡Critical Points
💡Concavity
💡Definite Integrals
Highlights
Review of limits, both algebraically and graphically, is essential for understanding business calculus for social sciences.
Direct substitution into the function is the first method to evaluate limits, followed by manipulation if the direct approach results in an indeterminate form.
Factoring is a useful technique when dealing with polynomials that result in indeterminate forms like zero over zero.
The concept of limits at infinity, such as the end behavior of a graph, is important for understanding the long-term trends of a function.
Derivatives are introduced as slopes of tangent lines to a curve at a particular point, using the difference quotient and limits.
The product rule, quotient rule, and chain rule are essential for finding derivatives of more complex functions.
Derivatives can also be used to analyze the behavior of a function, such as determining intervals of increase or decrease.
Critical points, local maxima and minima, and inflection points are identified using derivatives to understand the graph's features.
Integration techniques, including substitution and integration by parts, are covered for finding antiderivatives.
Definite integrals are used to calculate the area under a curve between two bounds, which has practical applications in various fields.
The natural logarithm function and its derivative properties are discussed in the context of integration.
Integration by substitution is demonstrated using the example of integrating a function involving a radical.
The antiderivative method is applied to various functions, including polynomials and exponentials, to find their integrals.
The importance of understanding the difference between indefinite and definite integrals is emphasized for proper application.
The use of limits and derivatives in graphing functions is a key takeaway for visualizing and analyzing mathematical behavior.
The class concludes with a reminder of the final exam and the importance of reaching out for special accommodations if needed.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: