Particle Motion - Speeding Up and Slowing Down (Example, AP Calculus)

James Elliott
4 Feb 202111:42
EducationalLearning
32 Likes 10 Comments

TLDRThis script explores the motion of a particle along the x-axis, using position, velocity, and acceleration functions. It identifies critical points where velocity and acceleration are zero, determines the intervals of speeding up and slowing down by comparing the signs of these functions, and visually represents this analysis on a number line.

Takeaways
  • 📚 The script discusses a physics problem involving a particle moving along the x-axis with its position modeled by a function x(t).
  • ⏱ The time variable t is measured in seconds, and the problem focuses on the interval from t=0 to t=4.
  • 🚀 To determine if the particle is speeding up or slowing down, one must consider its velocity and acceleration.
  • 📈 The velocity function v(t) is derived by differentiating the position function x(t), resulting in a polynomial expression.
  • 🔍 The acceleration function a(t) is found by differentiating the velocity function, yielding another polynomial.
  • 🔑 Critical points are identified by setting v(t) and a(t) equal to zero, which helps in understanding changes in the particle's motion.
  • 📊 A number line is used to visualize the intervals where the particle's velocity and acceleration are positive or negative.
  • 📌 The particle speeds up when the velocity and acceleration have the same sign, indicating consistent movement in the direction of motion.
  • 🔀 The particle slows down when the signs of velocity and acceleration are opposite, suggesting a deceleration in the direction of motion.
  • 📝 Test points are used to determine the sign of the velocity and acceleration functions between the critical points.
  • 🔮 The final step is to compare the signs of velocity and acceleration to conclude the intervals of speeding up and slowing down for the particle.
Q & A

  • What is the primary function of the function x(t) in the context of this problem?

    -The function x(t) models the position of a particle on the x-axis at any given time t, where t is measured in seconds.

  • What is the time interval considered for this problem?

    -The time interval considered for this problem is from t = 0 to t = 4 seconds.

  • How can we determine if a particle is speeding up?

    -A particle is speeding up if its velocity is positive and its acceleration is also positive, indicating it is being pushed to the right.

  • What is the velocity function v(t), and how is it derived?

    -The velocity function v(t) is derived by differentiating the position function x(t). For the given problem, v(t) = 3t^2 - 14t + 15.

  • What is the acceleration function a(t), and how is it derived?

    -The acceleration function a(t) is derived by differentiating the velocity function v(t). For the given problem, a(t) = 6t - 14.

  • Why are critical points important in analyzing the motion of a particle?

    -Critical points are important because they indicate where the velocity or acceleration is zero. These points help determine the intervals where the particle's motion changes direction or speed.

  • What are the critical points for the velocity function v(t) in this problem?

    -The critical points for the velocity function v(t) are t = 5/3 and t = 3, where the velocity is zero.

  • What are the critical points for the acceleration function a(t) in this problem?

    -The critical point for the acceleration function a(t) is t = 7/3, where the acceleration is zero.

  • How can we determine the intervals where the particle is speeding up or slowing down?

    -We determine the intervals by comparing the signs of the velocity and acceleration functions. If both are positive, the particle is speeding up. If both are negative, the particle is slowing down.

  • What are the intervals where the particle is speeding up according to the analysis?

    -The particle is speeding up in the intervals from t = 5/3 to t = 7/3 and from t = 3 to t = 4.

  • What are the intervals where the particle is slowing down according to the analysis?

    -The particle is slowing down in the intervals from t = 0 to t = 5/3 and from t = 7/3 to t = 3.

Outlines
00:00
🚀 Particle Motion Analysis

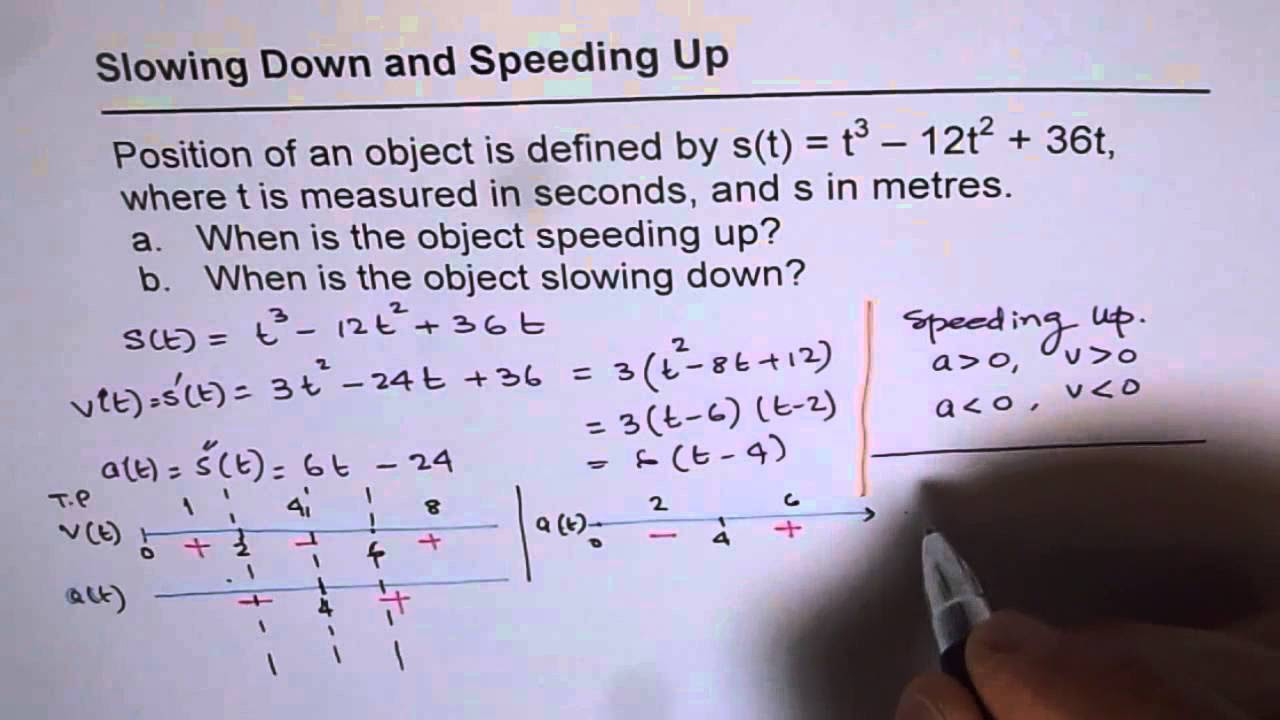
This paragraph introduces a physics problem involving a particle moving along the x-axis. The function x(t) models the particle's position over time, with time t measured in seconds from 0 to 4. The goal is to determine intervals of acceleration and deceleration. The concepts of velocity and acceleration are explained, with velocity indicating direction (right if positive) and acceleration showing the force applied (rightward push if positive). The velocity function v(t) is derived from the position function, and the acceleration function a(t) is the derivative of velocity. Critical points are identified by setting v(t) and a(t) to zero, which helps in understanding changes in the particle's motion.

05:02
📊 Critical Points and Motion Intervals

The second paragraph delves into identifying critical points for velocity and acceleration by solving the equations 3t^2 - 14t + 15 = 0 and 6t - 14 = 0, yielding t values of 5/3, 3, and 7/3, respectively. A visual representation is created using a number line divided into regions based on these critical points. Test points within these regions are used to determine the sign of velocity and acceleration, which indicates the direction of motion and force applied. The regions are analyzed to find where the particle is speeding up (same sign for velocity and acceleration) and slowing down (opposite signs), resulting in specific intervals for each motion type.

10:05
🔍 Conclusion of Particle's Motion Behavior

The final paragraph summarizes the process and findings. Starting with the position function of the particle, the paragraph outlines the steps taken to find velocity and acceleration functions, identify critical points, and plot them on a number line. Using test points, the signs of velocity and acceleration were determined to analyze the particle's motion between critical points. The conclusion identifies specific intervals where the particle speeds up and slows down, providing a comprehensive understanding of the particle's behavior from t=0 to t=4.

Mindmap
Keywords
💡Particle
A particle in physics is an object with mass that can be treated as a point without dimensions. In the video script, the term 'particle' refers to an object moving along the x-axis, whose position, velocity, and acceleration are being analyzed over time. The particle serves as the central subject of the video's theme, which is to understand its motion dynamics.
💡Position Function
In the context of the video, a position function, denoted as 'x of t', models the position of the particle on the x-axis at any given time 't'. It is a mathematical representation of the particle's location as a function of time and is essential for determining the particle's motion characteristics throughout the interval from 0 to 4 seconds.
💡Velocity
Velocity is the rate of change of an object's position with respect to time. In the video, the velocity function 'v of t' is derived from the position function by differentiation. It indicates the speed and direction of the particle's movement at any instant, with positive values indicating movement to the right and negative values indicating movement to the left.
💡Acceleration
Acceleration is the rate of change of velocity with respect to time. The script describes how the acceleration function 'a of t' is obtained by differentiating the velocity function. It represents the force applied to the particle, with positive acceleration indicating a push to the right and negative acceleration indicating a push to the left.
💡Critical Points
Critical points in the context of the video are the values of time 't' where either the velocity or acceleration equals zero. These points are significant as they indicate changes in the motion of the particle, such as when it starts or stops moving, or when it changes direction. The script identifies critical points by setting the velocity and acceleration functions to zero and solving for 't'.
💡Differentiation
Differentiation is a mathematical process used to find the derivative of a function, which represents the rate at which the function is changing at any point. In the video, differentiation is used to find the velocity and acceleration functions from the given position function, which helps in understanding how the particle's speed and direction of motion change over time.
💡Direction
Direction in the script refers to the orientation of the particle's movement along the x-axis. A positive velocity indicates movement in the positive direction (to the right), while a negative velocity indicates movement in the negative direction (to the left). The direction is crucial for determining whether the particle is speeding up or slowing down.
💡Speeding Up
Speeding up occurs when the particle's velocity and acceleration have the same sign, indicating that the particle is moving in the direction of the applied force. The script explains that this happens in intervals where the velocity and acceleration functions have the same sign, such as from five-thirds to seven-thirds and from three to four seconds.
💡Slowing Down
Slowing down is the opposite of speeding up and happens when the particle's velocity and acceleration have opposite signs. This means the particle is moving in one direction while being pushed in the opposite direction, causing its speed to decrease. The script identifies intervals of slowing down as from zero to five-thirds seconds and from seven-thirds to three seconds.
💡Test Points
Test points are specific values of time 't' chosen to evaluate the signs of the velocity and acceleration functions between critical points. By plugging these test points into the functions, one can determine the nature of the particle's motion (speeding up, slowing down, or at rest) within different intervals of time. The script uses test points like 1, 2, and seven-halves seconds to analyze the particle's behavior.
Highlights

A particle's motion along the x-axis is modeled by a position function x(t).

Time t is measured in seconds, with the interval from 0 to 4 seconds.

The problem aims to determine intervals of speeding up and slowing down for the particle.

Velocity and acceleration are key to understanding the particle's motion.

Velocity function v(t) is derived by differentiating the position function x(t).

Acceleration function a(t) is the derivative of the velocity function v(t).

Critical points are found by setting v(t) and a(t) to zero.

The velocity function is factored to find critical points at t = 5/3 and t = 3.

The acceleration function is solved to find the critical point at t = 7/3.

A number line is used to visualize the intervals between critical points.

Test points are used to determine the sign of velocity and acceleration functions.

The particle speeds up when the velocity and acceleration have the same sign.

The particle slows down when the velocity and acceleration have opposite signs.

Intervals of speeding up are identified as (5/3, 7/3) and (3, 4).

Intervals of slowing down are identified as (0, 5/3) and (7/3, 3).

The method involves a comprehensive analysis of position, velocity, and acceleration functions.

The approach provides a clear understanding of the particle's motion dynamics.

The process concludes with a summary of the particle's motion from t = 0 to t = 4.

Transcripts

Browse More Related Video

Slowing Down Speeding Up Derivatives Application Calculus MCV4U

Particle MotionDay1

Motion problems: when a particle is speeding up | AP Calculus AB | Khan Academy

2011 AP Calculus AB Free Response #1

How to Match Motion Graphs in Physics

when does a particle speed up or slow down?

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Particle MotionVelocity AnalysisAccelerationPhysics ConceptsCritical PointsMotion DirectionForce ApplicationTime IntervalMathematical ModelingEducational Content