9. Rotations, Part I: Dynamics of Rigid Bodies
TLDRIn this comprehensive lecture, Professor Ramamurti Shankar delves into the dynamics of rigid bodies, a topic of significant importance in physics. He begins by defining a rigid body as an object that doesn't change shape when forces are applied, using a coin or a meter stick as examples. The professor then introduces the concept of motion in three dimensions, emphasizing the complexity of translational and rotational movements. Through a step-by-step explanation, he guides students to understand how any motion of a body can be achieved by a combination of translation and rotation. Shankar further explores the technical definition of a rigid body in terms of the constancy of distances between points during motion. He also discusses the challenges in visualizing three-dimensional rotations and simplifies the problem by focusing on two-dimensional rotations first. The lecture progresses to cover the measurement of angles in radians as opposed to degrees, highlighting the naturalness of radians in physics and their connection to the universal constants π and 2. The professor also touches on the kinetic energy of rotating bodies, the concept of angular momentum, and the role of torque in causing angular acceleration. He illustrates these concepts with analogies to one-dimensional motion, making the subject more accessible. The lecture concludes with a discussion on calculating the moment of inertia for various objects, including continuous bodies like rods and disks, and emphasizes the importance of the point around which the moment of inertia is computed. The summary provides a clear and engaging overview of the dynamics of rigid bodies, sparking interest in the subject.
Takeaways
- 📚 A rigid body is an object that does not change shape when forces are applied to it, such as a coin or a meter stick, although in reality, no object is perfectly rigid.
- 🔍 The technical definition of a rigid body is that the distance between any two points on the body remains constant during motion.
- 🌀 The dynamics of a rigid body in three dimensions involves both translation (movement from one place to another) and rotation (turning around an axis).
- ⚙️ The simplest case to study is a two-dimensional rigid body, like a shape cut out from a metal sheet, which can rotate around a fixed point on a plane.
- 📏 The orientation of a rotating body can be described by an angle (θ) relative to a reference direction, such as the x-axis.
- 🎓 Radians are the preferred unit for measuring angles in advanced dynamics because they provide a natural connection between angular measure and the arc length of a circle.
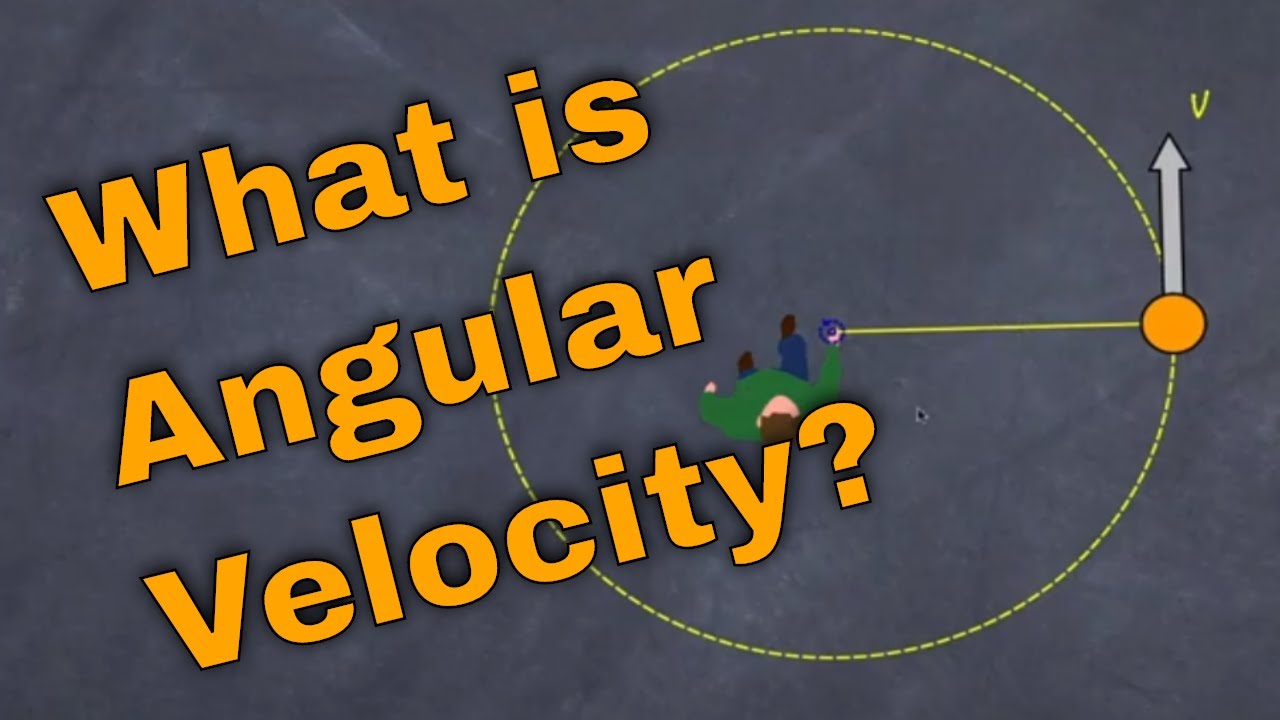
- ⏱️ Angular velocity (ω) is the rate of change of the angle with respect to time and is measured in radians per second.
- 🔄 The kinetic energy of a rotating rigid body can be calculated using the formula K = ½ Iω^(2), where I is the moment of inertia of the body.
- 📊 The moment of inertia (I) is a measure of the mass distribution of an object and depends on the point around which the body is rotating.
- 🤔 The moment of inertia is not a fixed property of a body; it changes depending on the axis of rotation, and calculating it requires integration for continuous bodies.
- 🛠️ Torque (τ) is the rotational equivalent of force and is given by τ = F_r sin θ_i, where F is the force, r is the distance from the pivot, and θ_i is the angle between the force and the lever arm.
- ⚖️ The net torque acting on a rigid body results in an angular acceleration (α), which can be found by dividing the net torque by the moment of inertia of the body.
Q & A
What is a rigid body in the context of physics?
-A rigid body is an object that does not change its shape or bend when forces are applied to it. It is an idealization where the distance between any two points on the body remains constant during motion.
How does the motion of a rigid body in three dimensions differ from a point mass?
-While a point mass only exhibits translations (moving from one place to another, either in a straight line or a curve), a rigid body also exhibits rotations. This means a rigid body can translate and rotate, which makes its dynamics more complex.
What is the technical definition of a rigid body in terms of the distance between two points on the body during motion?
-The technical definition of a rigid body is that the distance between any two points on the body remains constant regardless of the motion of the body.
What is the simplest way to describe the orientation of a two-dimensional rigid body that is rotating around a fixed point?
-The orientation of a two-dimensional rigid body rotating around a fixed point can be described by the angle (θ) that a chosen line on the body makes with a standard reference direction, usually the x-axis.
Why do physicists prefer to measure angles in radians instead of degrees?
-Radians are preferred in physics because they provide a more natural and consistent way to relate angular measurements to the length of an arc on a circle. This is particularly useful in advanced dynamics and when considering the behavior of particles like fermions and bosons.
What is the relationship between the linear distance traveled on the circumference of a circle and the angle through which a point on a rigid body rotates?
-The linear distance traveled (arc length) is directly proportional to the radius of the circle (r) and the angle through which the point rotates (θ), where θ is measured in radians.
How is angular velocity (ω) defined and what are its units?
-Angular velocity (ω) is defined as the rate of change of the angle with respect to time. It is measured in radians per second (rad/s).
What is the relationship between the angular velocity of a rotating rigid body and the tangential velocity of a point on the body?
-The tangential velocity of a point on a rotating rigid body is equal to the product of the angular velocity (ω) and the distance (r) of that point from the center of rotation.
What is the term used to describe the rate of change of angular velocity and what is its symbol?
-The rate of change of angular velocity is called angular acceleration, and it is denoted by the symbol α.
What is the moment of inertia and how does it relate to the kinetic energy of a rotating rigid body?
-The moment of inertia (I) is a measure of the distribution of mass in a rotating rigid body around a specific axis. It relates to the kinetic energy of the rotating body through the equation K = ½ Iω^(2), where K is the kinetic energy, I is the moment of inertia, and ω is the angular velocity.
What is torque and how is it calculated when a force is applied to a rigid body?
-Torque is the rotational equivalent of force and is responsible for causing angular acceleration. It is calculated as the product of the force (F), the distance from the point of rotation (r), and the sine of the angle between the force and the line of separation (sin θ_i), which is F_r * sin θ_i.
Outlines
📚 Introduction to Rigid Body Dynamics
Professor Shankar introduces the concept of rigid bodies, which are objects that do not change shape when forces are applied. He uses examples like a dime or a meter stick to illustrate this concept. The lecture emphasizes that in three dimensions, the dynamics of rigid bodies involve both translation and rotation. The professor explains that any motion of a rigid body can be broken down into a translation followed by a rotation. He also discusses the importance of orientation and how it's necessary to define both position and rotation to fully describe the motion of a rigid body.
🌀 Rotation in Two Dimensions
The professor delves into the example of a two-dimensional rigid body, like a metal shape on a blackboard plane. He explains that such a body can only rotate around an axis passing through the fixed point. To describe the orientation of the body, an angle θ is used, which is the angle a chosen line on the body makes with a reference direction, typically the x-axis. The analogy between one-dimensional translation and two-dimensional rotation is highlighted, and the professor introduces radians as the preferred unit for measuring angles in advanced dynamics, contrasting with the more common degrees.
🔵 Particle Spin and Radian Explanation
The discussion shifts to the nature of particles, specifically bosons and fermions, and their behavior under rotation. The professor explains that fermions, like electrons, require a 720-degree rotation to return to their original state, while bosons, like photons, return to their state after a 360-degree rotation. The preference for radians in physics is linked to the naturalness of the unit across different scales and civilizations. The relationship between radians, the circumference of a circle, and the linear distance traveled during rotation is also explored.
📐 Angular Velocity and Acceleration
The concept of angular velocity (ω) is introduced as the rate of change of the angle θ with respect to time. The professor explains that angular velocity is measured in radians per second and is constant for a rigid body. He also touches on the idea of angular acceleration (α), which is the rate of change of angular velocity. The lecture covers how to calculate the tangential speed of a point on a rotating body and how to relate it to the angular velocity and the radius of rotation. The analogy between linear velocity and angular velocity is emphasized, along with the importance of understanding the components of acceleration on a rotating body.
🚧 Work, Energy, and Torque
The professor discusses the work-energy theorem in the context of rotational motion, leading to the concept of torque. He explains that torque is the rotational equivalent of force and is calculated as the product of the force applied, the radius of rotation, and the sine of the angle between the force and the radius. The importance of the direction of the force in producing torque is highlighted, with the professor using the example of opening a door to illustrate the principle that the most effective force is applied farthest from the hinges and perpendicular to the direction of resistance.
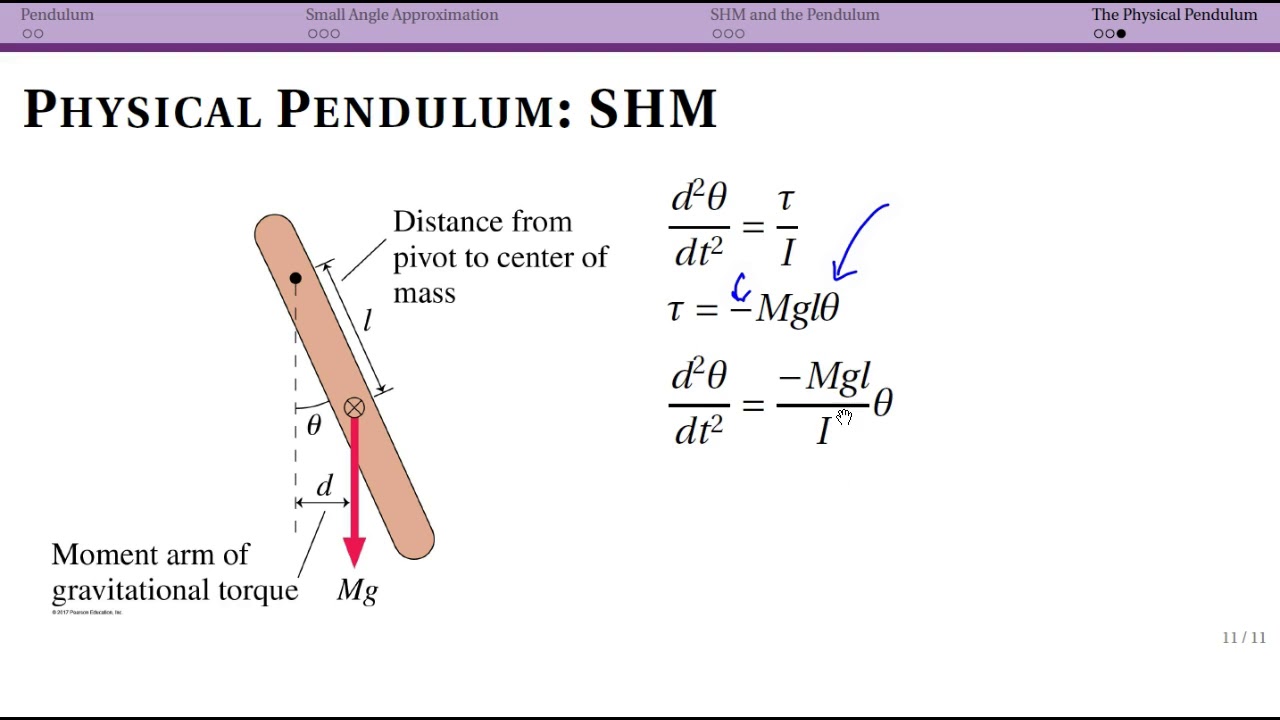
🔄 Moment of Inertia Calculations
The lecture concludes with the calculation of the moment of inertia for various objects, starting with simple cases like point masses and then moving to continuous bodies like rings, disks, and rods. The professor emphasizes that the moment of inertia depends on the distribution of mass relative to the axis of rotation. For a ring, the moment of inertia is found to be Mr^2, where M is the mass and r is the radius. The calculation for a disk results in MR^2/2, and for a rod rotating around one end, it is ML^2/3, where L is the length. The professor also touches on the concept that the moment of inertia around the center of mass is the smallest and can be used to find the moment of inertia around any other point by adding the mass times the square of the distance from the center of mass.
Mindmap
Keywords
💡Rigid Body
💡Translation
💡Rotation
💡Moment of Inertia
💡Angular Velocity
💡Torque
💡Kinetic Energy
💡Angular Momentum
💡Work-Energy Theorem
💡Integration
💡Center of Mass
Highlights
A rigid body is defined as an object that does not bend or change shape when forces are applied to it.
The technical definition of a rigid body involves the constancy of the distance between any two points during its motion.
The dynamics of rigid bodies in three dimensions is complex, involving both translation and rotation.
The concept of angular velocity (ω) is introduced as the rate of change of the angle θ with respect to time.
Angular velocity ω is measured in radians per second, distinguishing it from rotational speed in revolutions per minute (rpm).
The moment of inertia (I) is a measure of an object's resistance to rotational motion, depending on both mass distribution and the axis of rotation.
The kinetic energy of a rotating rigid body is given by the equation K = ½ Iω^(2), analogous to the kinetic energy of translational motion.
Torque (τ) is the rotational equivalent of force and is defined as the product of the force applied, the radius of rotation, and the sine of the angle between them.
The net torque acting on a body results in an angular acceleration (α), following the rotational analog of Newton's second law, τ = Iα.
The Work-Energy Theorem is applied to rotational motion to calculate the work done by a torque causing a rotation.
The moment of inertia for a continuous rigid body, such as a rod or disk, is calculated using integration techniques.
The moment of inertia for a ring is calculated to be Mr^(2), where M is the mass and r is the radius.
For a solid disk, the moment of inertia is found to be MR^(2)/2, reflecting the mass distribution away from the center.
The moment of inertia for a rod rotating around one end is ML^(2)/3, where M is the mass and L is the length.
When calculating the moment of inertia around the center of mass, the value is smaller than that for rotation around an end.
A theorem is introduced stating that the moment of inertia about any axis is equal to the moment of inertia about the center of mass plus the mass times the square of the distance from the center of mass to the axis.
The importance of understanding the concept of radians in physics, as it is a natural unit derived from the fundamental constants π and 2.
The distinction between the angular acceleration when the angular velocity is changing and the centripetal acceleration experienced by points on a rotating body.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: