Russell's Paradox - a simple explanation of a profound problem
TLDRThe video script explores the paradox discovered by Bertrand Russell in set theory, a foundational branch of mathematics. It begins by questioning the nature of numbers and introduces logicism, the idea that mathematics is a branch of logic. The script explains naive set theory, which studies collections of objects, and highlights the development of set theory by Georg Cantor, including his discovery that some infinities are larger than others. The paradox arises from considering sets that contain themselves and sets that do not, leading to a contradiction. Russell and other mathematicians attempted to resolve this by modifying set theory rules, particularly by disallowing sets to contain themselves. However, the script argues that the paradox is not just a mathematical issue but a deeper problem with language and thought itself, as it can be regenerated with the concept of predication, the practice of attributing characteristics to things, which is fundamental to human communication and cognition.
Takeaways
- 📚 Bertrand Russell discovered a paradox at the heart of mathematics and science, specifically concerning set theory.
- 🔢 The nature of numbers was a central question that led to the development of logicism, which aimed to reduce arithmetic to logic and set theory.
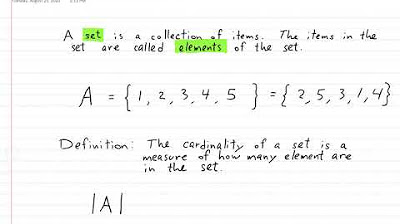
- 🛍️ A set is a collection of objects, which can be anything we can refer to, and does not require the objects to be related or collected together.
- 🌐 Georg Cantor invented set theory in the 1870s, proving that some infinities are larger than others, which was a groundbreaking concept.
- 🚫 Russell's paradox arises from considering the set of all sets that do not contain themselves, leading to a contradiction whether the set is included within itself or not.
- ⛔ The paradox challenges the foundational rules of set theory, particularly the rule that allows sets to contain themselves (Rule 11).
- 🔄 Russell and other mathematicians attempted to resolve the paradox by modifying the rules of set theory, but this was not entirely satisfactory.
- 🔑 The script argues that the rules of set theory are not arbitrary but reflect objective principles governing human thought and language, specifically predication.
- 💬 Predication, the act of attributing characteristics to subjects, mirrors the rules of set theory and also leads to a similar paradox when considering predicates true of themselves.
- 🧐 The paradox is not just a mathematical issue but extends to all of language and thought, as it pertains to the nature of self-reference and truth.
- ❌ The paradox reveals a fundamental contradiction that cannot be easily dismissed or resolved by changing the rules, as it touches on the core of how we understand and describe the world.
Q & A
What is the significance of Bertrand Russell's paradox in the context of mathematics and science?
-Bertrand Russell's paradox is significant because it challenges the foundational principles of set theory, which is a fundamental branch of mathematics. The paradox raises questions about the nature of mathematical truth and objectivity, and it has led to extensive revisions in the understanding and formalization of set theory.
What is Logicism, and how does it relate to the concept of numbers?
-Logicism is a philosophical view developed by mathematicians like Bertrand Russell and Gottlob Frege. It posits that mathematics is a branch of logic and that arithmetic can be reduced to first-order logic and set theory. According to logicism, numbers are essentially sets, which provides a way to define numbers in a logical framework.
What is Georg Cantor's contribution to set theory?
-Georg Cantor, a German-Russian mathematician, is credited with the invention of set theory in the 1870s. He introduced the concept of sets as collections of objects and proved that some infinities are larger than others, a groundbreaking idea that expanded the understanding of infinity in mathematics.
What is the concept of the empty set or null set in set theory?
-The empty set, or null set, is a set with no members or elements. It is denoted by curly brackets with nothing in between or by a zero with a line through it. The existence of the empty set is a fundamental aspect of set theory, and it is defined by the fact that it contains nothing.
How does Russell's paradox challenge the unrestricted composition rule of set theory?
-Russell's paradox challenges the unrestricted composition rule by demonstrating a situation where a set can neither consistently contain itself nor consistently exclude itself. This leads to a contradiction, suggesting that the unrestricted composition rule, which allows for the formation of any conceivable set, may not be a coherent principle.
What is the role of predication in generating Russell's paradox, and why is it significant?
-Predication is the linguistic practice of attributing characteristics to subjects. The paradox arises when predication is used to create a predicate that is true of all predicates that are not true of themselves. This leads to a contradiction similar to Russell's paradox in set theory, suggesting that the issue may be inherent in the nature of language and thought itself, not just in set theory.
How did Russell's paradox impact the development of set theory?
-Russell's paradox had a profound impact on set theory, leading to a crisis in the foundations of mathematics. It prompted mathematicians to revise and restrict the axioms of set theory to avoid such paradoxes. Different formal systems, such as Zermelo-Fraenkel set theory, were developed to address the issues raised by the paradox.
What is the axiom of extensionality in set theory, and how does it relate to set identity?
-The axiom of extensionality states that two sets are identical if and only if they have the same members. It is fundamental to set theory as it determines set identity based solely on the elements contained within the sets, regardless of the order of those elements or how the sets are described.
Can you explain the concept of a singleton set and how it is different from the element it contains?
-A singleton set is a set that contains exactly one element. It is different from the element it contains because the set is a collection, an abstract mathematical entity, while the element is an individual object that the set includes. For example, the singleton set containing LeBron James is not the same as LeBron James himself; the set represents a collection with one member, whereas LeBron James is an individual person.
What is the concept of predication, and how does it relate to the paradox discussed in the script?
-Predication is the act of attributing a property or characteristic to a subject in a sentence or proposition. It is a fundamental aspect of language and thought. In the context of the paradox, predication is used to create a predicate ('is not true of itself') that leads to a contradiction, similar to Russell's paradox, when applied to itself. This suggests that the paradox may be a deeper issue related to the nature of language and logic.
How does the script suggest that the rules of set theory are not arbitrary but have real, objective existence?
-The script argues that the rules of set theory, particularly the principles that led to Russell's paradox, are not just arbitrary constructs but reflect objective aspects of human thought and language, specifically predication. The paradox arises naturally from these objective rules when applied to the concept of self-reference, suggesting that the issue may be more profound than a problem with set theory alone.
What is the set builder notation, and how is it used in set theory?
-The set builder notation is a way to describe a set by specifying a property that its members must satisfy. It is used in set theory to define sets more concisely, especially when the set contains a large number of elements or when the elements are not all known. For example, the set of all cats can be described using set builder notation as {x | x is a cat}, where 'x' represents the elements of the set.
Outlines
🔢 Introduction to Set Theory and Russell's Paradox
This paragraph introduces Bertrand Russell's discovery of a paradox within set theory, a foundational branch of mathematics. It discusses the importance of understanding what a number is and how set theory, developed by Georg Cantor, plays a role in defining numbers as sets. The paradox is said to undermine not just set theory, but all of mathematics and science. The paragraph also touches on the philosophical debate about the nature of mathematical truth, contrasting the subjective view of Immanuel Kant with the objective view of Frege and Russell, known as logicism.
📚 Basic Concepts and Notation of Set Theory
The second paragraph delves into the basic concepts and notation of set theory. It explains that a set is a collection of objects, which need not be related or located in the same place. The notation used to describe sets is introduced, including the set builder notation for describing large or infinite sets. The concept of 'contains' is also defined, emphasizing that the order of elements in a set and the description of items do not affect the identity of the set. The paragraph ends with an introduction to the union of sets and the concept of subsets.
🅰️ Set Theory Rules Leading to Russell's Paradox
This paragraph outlines several fundamental rules of set theory that lead up to Russell's paradox. It discusses unrestricted composition, set identity determined by membership, the irrelevance of element order, the insignificance of duplicates within a set, the independence of item description, the union of sets, the existence of subsets, the possibility of singleton sets, the concept of the empty set, and the allowance of sets containing other sets. The paragraph builds up to the concept that sets can contain themselves, which is the crux of Russell's paradox.
🔥 Russell's Paradox and the Crisis in Set Theory
The fourth paragraph presents Russell's paradox in full. It describes the thought process behind the paradox, which involves considering sets that contain themselves and those that do not. The paradox is articulated through the question of whether the set of all sets that do not contain themselves contains itself. This leads to a contradiction, as the set either contains itself and therefore does not, or does not contain itself and therefore must. The paragraph also discusses the attempts to resolve the paradox by revising the rules of set theory, particularly by disallowing sets to contain themselves.
📝 The Intrinsic Rules of Predication and a Broader Paradox
The final paragraph argues that the rules of set theory are not arbitrary but reflect real, objective principles governing human practices, specifically predication—the act of attributing characteristics to things. The paragraph draws parallels between the rules of set theory and predication, noting that predicates, like sets, can apply to themselves. It then constructs a predicate that refers to all predicates that do not apply to themselves, leading to a paradox similar to Russell's. The paragraph concludes that this is not just a paradox for set theory, but for language and thought as a whole, as it shows that predicates can be both true and false of themselves.
Mindmap
Keywords
💡Set Theory
💡Russell's Paradox
💡Logicism
💡Naive Set Theory
💡Arithmetic
💡Immanuel Kant
💡Georg Cantor
💡Empty Set
💡Singleton Set
💡Predicate
💡Unrestricted Composition
Highlights
Bertrand Russell discovered a paradox at the heart of mathematics and science concerning set theory in 1901.
Russell and other mathematicians initially believed they could solve the paradox but later realized the complexity involved.
Numbers are considered essential to science, technology, and human life, yet their nature is abstract and not physically tangible.
Immanuel Kant proposed that mathematics is a construction of the human mind, implying mathematical truths could be subjective.
Logicism, developed by Frege and Russell, posits that mathematics is a branch of logic and arithmetic can be reduced to logic and set theory.
Set theory, created by Georg Cantor in the 1870s, is the study of collections of objects and is foundational to modern mathematics.
Cantor's work showed that some infinities are larger than others, a revolutionary concept in mathematics.
Naive set theory is the ordinary set theory that can be formulated in natural language, contrasting with formal or axiomatic set theory.
A set is defined by its members, regardless of the order or description of its elements, leading to the concept of set identity.
The empty set, or null set, is a set with no members and is a fundamental concept in set theory.
Sets can contain other sets, leading to complex hierarchical structures of sets within sets.
Russell's paradox arises from considering the set of all sets that do not contain themselves, resulting in a logical contradiction.
The paradox challenges the basic rules of set theory, particularly the unrestricted composition and the ability for sets to contain themselves.
Attempts to resolve the paradox by changing the rules of set theory are not trivial and have far-reaching implications for mathematical foundations.
The paradox is not just a problem for set theory but extends to the practice of predication and the nature of language and thought itself.
The video argues that the rules of set theory are not arbitrary but reflect objective, pre-existing structures governing human practices such as predication.
The paradox generated from predication, similar to Russell's paradox in set theory, shows that the issue extends beyond mathematics into the core of how we use language.
Transcripts
Browse More Related Video

Russell's Paradox - A Ripple in the Foundations of Mathematics

PLT: Type Theory 1 - ZFC set theory (part 1)

13-1 Pure Mathematics Set Theory, Logic, Recursion, Computability, Model Theory

Classification of sets - Lec 03 - Frederic Schuller

Navigating an Infinitely Dense Minefield | Why Measure Infinity?
Sets Theory and Logic Lecture 1 Sets
5.0 / 5 (0 votes)
Thanks for rating: