Navigating an Infinitely Dense Minefield | Why Measure Infinity?
TLDRThe video script explores the concept of different 'sizes' of infinity and their practical applications in mathematics. It introduces the idea that some infinities are larger than others, using the example of countably infinite sets like natural numbers, integers, and rational numbers, versus the uncountably infinite set of real numbers. The script then presents a thought experiment involving navigating an island densely packed with infinitely many landmines, all located at points with rational number coordinates. By comparing the countably infinite number of mines to the uncountably infinite number of possible paths, the video demonstrates that one can find a safe path through the minefield by choosing a random direction, highlighting the power of understanding infinity's sizes. The script concludes by emphasizing the importance of cardinality in solving problems and the value of abstract mathematical structures in simplifying complex issues.
Takeaways
- 🧐 The concept of different 'sizes' of infinity is not just a theoretical abstraction but has practical applications in various branches of mathematics.
- 📏 To measure infinity, mathematicians use the set of natural numbers as a 'standard ruler' and compare other infinite sets to it through a one-to-one correspondence.
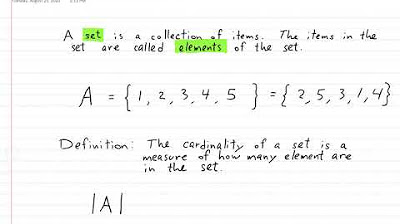
- 📋 The term 'cardinality' is used to describe the number of objects in an infinite set, and 'countably infinite' refers to sets that can be paired with natural numbers without leftovers.
- 🔢 It's possible to arrange sets of negative whole numbers, integers, and rational numbers into infinitely long lists, indicating they are countably infinite.
- 📉 The set of all real numbers, including irrational numbers, is uncountable, meaning it cannot be paired with natural numbers without leaving leftovers, making its cardinality larger than that of natural numbers.
- 💡 The script uses the idea of different infinities to solve a hypothetical problem involving navigating through an infinitely dense minefield, demonstrating a practical application of the concept.
- 🕵️♂️ The solution to the minefield problem hinges on the observation that there are only countably infinitely many mines (as they are located at points with rational number coordinates), contrasting with the uncountably infinite number of possible paths.
- 🛤️ By choosing a path that does not coincide with any of the countably infinite number of mine paths, it's possible to navigate through the minefield safely.
- 🎯 The problem-solving approach emphasizes the importance of understanding the broad structural categories in mathematics, such as cardinality, which can simplify complex problems.
- 🧬 The script illustrates that sometimes a high-level understanding of mathematical structures can eliminate the need for detailed, specific information, streamlining the problem-solving process.
Q & A
What is the concept of different sizes of infinity?
-The concept of different sizes of infinity suggests that not all infinities are the same. Some infinities can be larger or have more elements than others. This is a fundamental idea in set theory and is often counterintuitive to those first encountering it.
Why might some people consider the idea of different sizes of infinity as impractical?
-Some people might consider the idea of different sizes of infinity as impractical because we live in a finite world, and it might seem like a purely theoretical concept that doesn't have tangible applications. However, the script points out that this idea is actually quite widespread in various branches of mathematics.
In which areas of mathematics do the concepts of different sizes of infinity appear?
-The concepts of different sizes of infinity appear in areas such as topology, analysis, and measure theory, where the sizes of infinity and related concepts are directly or indirectly involved.
What is the standard unit for measuring infinity in mathematics?
-The standard unit for measuring infinity in mathematics is the set of positive whole numbers, also known as natural numbers (1, 2, 3, 4, 5, and so on).
What is meant by a 'one-to-one correspondence' in the context of comparing infinite sets?
-A one-to-one correspondence is a way to compare two sets by pairing each element of one set with a unique element of the other set without any leftovers. If such a pairing is possible, the sets are said to have the same size or cardinality.
What is the technical term for the number of objects in an infinite set?
-The technical term for the number of objects in an infinite set is cardinality.
What is a countably infinite set?
-A countably infinite set is an infinite set that can be arranged into an infinitely long list, meaning it can be paired with the set of natural numbers through a one-to-one correspondence.
Why are all rational numbers considered to form a countably infinite set?
-All rational numbers can be arranged into a single infinitely long list by using a method that involves arranging them into an infinitely large table and then weaving a zigzagging string through the table, which ensures that every rational number is included exactly once.
What is the cardinality of the set of real numbers compared to the set of natural numbers?
-The cardinality of the set of real numbers is strictly greater than that of the natural numbers. This means there are more real numbers than there are natural numbers, making the set of real numbers uncountable.
How does the concept of different sizes of infinity help solve the infinitely dense minefield problem presented in the script?
-The concept of different sizes of infinity helps solve the minefield problem by recognizing that there are only countably infinitely many mines (since they are located at points with rational coordinates), whereas there are uncountably many possible paths one could take. This means that there must be many paths that do not intersect any mines, allowing one to navigate the minefield successfully.
What is the significance of the fact that there are uncountably many linear paths that do not hit any mines in the minefield problem?
-The significance is that it provides a strategy to navigate the minefield safely. Since there are uncountably many mine-free paths, one can almost surely avoid all mines by choosing a path at random, which highlights the power of understanding different sizes of infinity.
Why does the arrangement of the mines in the minefield problem not matter when it comes to finding a path to avoid all of them?
-The arrangement of the mines does not matter because as long as there are only countably many mines, it is guaranteed that there will be uncountably many paths that do not intersect any mines. This is due to the fundamental properties of countable and uncountable infinities.
What broader lesson does the minefield problem teach us about the importance of abstract mathematical structures?
-The minefield problem teaches us that understanding abstract mathematical structures, such as cardinality, can be crucial in solving complex problems. Even though the specific arrangement of the mines does not matter, the broader category of cardinality is enough to devise a solution, illustrating the value of abstract thinking in mathematics.
Outlines
🧠 The Concept of Infinite Sizes in Mathematics
This paragraph introduces the abstract concept of different sizes of infinity and challenges the perception that it's merely a theoretical curiosity without practical applications. It emphasizes that the concept is surprisingly widespread across various mathematical disciplines, including topology, analysis, and measure theory, and sets the stage for exploring its significance and usefulness in the foundation of mathematics.
📏 Measuring Infinity: Countable and Uncountable Sets
The script delves into the measurement of infinity, explaining the concept of cardinality and how it's used to determine the size of infinite sets. It introduces the standard unit of measurement for infinity—the set of natural numbers—and discusses how to establish a one-to-one correspondence to compare the sizes of different infinite sets. The paragraph explains the difference between countably infinite sets, like integers and rational numbers, and uncountable sets, using the set of all real numbers as an example to illustrate the larger size of infinity found in the continuum of real numbers.
🏝️ Navigating an Infinitely Dense Minefield
The video script presents a hypothetical scenario where one must navigate an island filled with infinitely many landmines, each located at points with rational coordinates. It explores the idea of different sizes of infinity, specifically countable and uncountable infinities, to solve the problem of finding a path to freedom without stepping on any mines. The scenario is used as a practical example to demonstrate the application of the concept of infinity in a seemingly impossible situation.
🛤️ Escaping the Minefield: The Power of Cardinality
This paragraph reveals the solution to the minefield problem by focusing on the cardinality of the set of mines. It explains that despite the mines being densely packed, there are only countably infinitely many of them, which allows for the existence of uncountably many safe paths. The solution involves choosing a path that does not intersect with any of the mines and constructing a route to the exit by combining this path with others that also avoid the mines. The paragraph concludes by highlighting the importance of understanding different mathematical structures and how cardinality, in this case, simplifies the problem-solving process by ignoring the specific distribution of the mines.
Mindmap
Keywords
💡Infinity
💡Cardinality
💡Countably Infinite
💡Uncountable
💡Rational Numbers
💡Real Numbers
💡Irrational Numbers
💡Topology
💡Measure Theory
💡Minefield Problem
Highlights
The concept of different sizes of infinity is explored, challenging the traditional understanding of infinity as a singular entity.
The practical applications of the sizes of infinity in various branches of mathematics, such as topology, analysis, and measure theory, are discussed.
The video aims to demonstrate the significance and usefulness of the sizes of infinity in solving real-world problems.
A brief review of the concept of sizes of infinity and how one infinity can be larger than another is provided.
The standard unit for measuring infinity is the set of positive whole numbers, or natural numbers.
The concept of cardinality is introduced as the technical term for the number of objects in an infinite set.
Countably infinite sets can be arranged into an infinitely long list, like natural numbers, integers, and rational numbers.
The set of all real numbers, including irrational numbers, is shown to be uncountable and thus a larger infinity than that of natural numbers.
Sets can be broadly classified into finite, countably infinite, and uncountable based on their cardinality.
A thought experiment involving navigating through an infinitely dense minefield using the concept of different infinities is presented.
The problem of the minefield is used to illustrate how the size of infinity (countably vs. uncountably infinite) can provide a solution.
The concept that there are only countably infinitely many mines on the island is explained through the arrangement of rational numbers.
The realization that there are uncountably many linear paths that do not hit any mines, making it possible to navigate the minefield.
The solution to the minefield problem does not require knowledge of the specific arrangement of mines, only their countability.
The importance of cardinality and abstract mathematical structures in solving problems without needing to consider finer details is emphasized.
The video concludes by highlighting the value of learning about abstract mathematical structures for solving complex problems.
Transcripts
Browse More Related Video

Mathematician Explains Infinity in 5 Levels of Difficulty | WIRED

Classification of sets - Lec 03 - Frederic Schuller

The Infinite Hotel Paradox - Jeff Dekofsky

The Beauty of Math and Music | Marcus Miller | TEDxOttawa

Unit VII: Lec 1 | MIT Calculus Revisited: Single Variable Calculus
Sets Theory and Logic Lecture 1 Sets
5.0 / 5 (0 votes)
Thanks for rating: