Classification of sets - Lec 03 - Frederic Schuller
TLDRThe transcript delves into the foundational concepts of set theory and its pivotal role in mathematics, emphasizing the classification of sets and spaces. It outlines how mathematicians use structure-preserving maps to classify spaces, a method applicable to various mathematical structures like topology and differentiable structures. The video explains the concept of a map in set theory and introduces key terms such as domain, target, image, and bijections. It also distinguishes between injective, surjective, and bijective maps, leading to the definition of set theoretical isomorphism. The classification extends to infinite sets, countably infinite, and uncountably infinite, contrasting with finite sets. The script further explores the composition of maps, the existence of inverse maps, and the concept of equivalence relations, which are used to identify elements in a set based on specific criteria. The video also touches on the construction of integers, rational numbers, and real numbers from axiomatic set theory, highlighting the meticulous process of defining mathematical operations like addition and multiplication on these sets. The summary concludes with a look ahead to the study of topology, which will build on these foundational concepts to explore continuity and convergence.
Takeaways
- 📚 The process of classification in mathematics involves categorizing sets and understanding their structure, which is not limited to set theory but is a recurrent theme across the discipline.
- 🔍 To classify spaces, mathematicians use structure-preserving maps, which require a clear definition of both 'spaces' and 'maps', with spaces being sets equipped with some structure.
- 🔗 A map, in set theory, is a relation that assigns to every element from one set a unique element in another set, and this assignment is often notated as a special relation.
- 🎯 The concept of 'injective', 'surjective', and 'bijective' maps are central to understanding how sets are related through mappings, with bijective maps being particularly important as they indicate a one-to-one correspondence between sets.
- 🧵 The composition of maps allows for the creation of new maps from existing ones, which is a fundamental operation in understanding how different spaces can be interconnected.
- 🔄 Equivalence relations are used to identify elements in a set based on specific criteria, leading to the creation of equivalence classes, which are subsets of the original set that share the defined property.
- ⚖️ Equivalence classes have the property that any element can represent the entire class, and either two classes are identical or they have no elements in common.
- 🌐 The construction of integers, rational numbers, and real numbers can be defined using set theory, with integers being created from pairs of natural numbers under an equivalence relation.
- ⛓ The integers can be embedded into the rational numbers by representing an integer as a fraction with a denominator of one.
- 🔢 Rational numbers are defined as pairs of integers under an equivalence relation that identifies simplified forms of fractions, which leads to the construction of the set of rational numbers, Q.
- 📈 Real numbers can be approached through various constructions, one of which involves the set of almost homomorphisms on Z, leading to the inclusion of numbers like the square root of two.
- 🏭 The axioms of set theory are powerful enough to construct the integers, and with further work, the rational and real numbers, showcasing the foundational role of set theory in mathematics.
Q & A
What is the recurrent theme in mathematics that the speaker introduces?
-The recurrent theme in mathematics introduced by the speaker is the classification of spaces by means of structure-preserving maps between those spaces.
What does the speaker mean by 'structure' in the context of set theory?
-In the context of set theory, 'structure' refers to additional properties or operations that can be defined on a set, such as a topological structure, differentiable structure, or group structure.
How is a map defined in the context of set theory?
-A map, or function, f from a set A to a set B is defined as a relation such that for every element a in A, there exists exactly one element b in B such that the relation f(a, b) holds true.
What are the three key properties of a map that the speaker discusses?
-The three key properties discussed are: a map being injective (one-to-one), surjective (onto), and bijective (both injective and surjective).
What is the definition of set theoretical isomorphism?
-Two sets A and B are called set theoretically isomorphic if there exists a bijective map from A to B. This is denoted as A ~_B, with the tilde and 'set' as subscript.
How does the speaker define an infinite set?
-An infinite set is defined as a set that has a proper subset which is isomorphic to the original set. This means there exists a proper subset that can be paired with the original set element by element.
What is the difference between countably infinite and uncountably infinite sets?
-A set is countably infinite if it is isomorphic to the set of natural numbers, meaning its elements can be labeled by natural numbers. A set is uncountably infinite if it is not countably infinite, implying there is no way to label its elements with natural numbers.
What is the concept of composition of maps in the context of set theory?
-The composition of maps is a process where given two maps, one from set A to set B and another from set B to set C, a third map can be constructed that goes directly from set A to set C. This new map is defined by applying the first map to an element of A and then applying the second map to the result.
How does the speaker define the equivalence relation?
-An equivalence relation is defined on a set M by a relation that satisfies three axioms: reflexivity (each element is equivalent to itself), symmetry (if one element is equivalent to a second, then the second is equivalent to the first), and transitivity (if the first is equivalent to the second and the second to the third, then the first is equivalent to the third).
What is the concept of an equivalence class?
-An equivalence class is a subset of a set M that contains all elements of M that are equivalent to a given element n, under a specified equivalence relation.
How does the speaker describe the construction of integers from natural numbers?
-The speaker describes constructing integers from natural numbers by defining an equivalence relation on the Cartesian product of natural numbers with themselves, where a pair (M, N) is equivalent to a pair (P, Q) if M + Q = P + N. The set of integers is then defined as the quotient set of these pairs under this equivalence relation.
Outlines
😀 Introduction to Set Classification in Mathematics
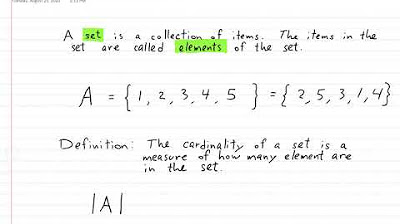
The paragraph introduces the concept of set classification in mathematics, emphasizing its importance in gaining an overview of the structure of sets. It discusses the classification of spaces by means of structure-preserving maps and the need to define spaces and maps. A space is described as a set equipped with some structure, which could be a topology, a differentiable structure, or a group structure. The paragraph also explains the definition of a map as a relation that assigns to every element in one set a unique element in another set.
📚 Terminology and Properties of Maps
This paragraph delves into the standard notation for maps and the structural understanding of maps as relations. It introduces the terminology of domain and target of a map and defines the image of a set under a map. The concepts of injective, surjective, and bijective maps are explained, highlighting the importance of bijective maps in set theory. The paragraph also discusses the concept of set theoretical isomorphism.
🔢 Classification of Sets by Infinity and Countability
The paragraph explores the classification of sets as finite or infinite. It defines a set as infinite if there exists a proper subset that is isomorphic to the set itself. The infinite sets are further categorized into countably infinite, where elements can be put into one-to-one correspondence with natural numbers, and uncountably infinite, which cannot be labeled in such a manner. The paragraph also provides examples of countably infinite sets and discusses the challenges in defining infinite sets.
🔄 Composition of Maps and the Concept of Equivalence Relations
This section discusses the composition of maps, which is the process of combining two maps to create a third. It emphasizes the associative property of map composition and introduces the concept of an inverse map. The paragraph also touches on the idea of pre-images under a map and the importance of well-defined operations on quotient sets. It concludes with an introduction to equivalence relations and their role in classifying elements of a set based on certain properties.
🔗 Equivalence Relations and Their Properties
The paragraph focuses on the properties of equivalence relations, which are relations that satisfy reflexivity, symmetry, and transitivity. It provides examples of relations that are and are not equivalence relations, and explains the construction of equivalence classes. The paragraph also discusses the key properties of equivalence classes, including the ability for any element of an equivalence class to represent the whole class and the mutual exclusivity of different equivalence classes.
🤔 Equivalence Classes and Quotient Sets
This paragraph explains the concept of a quotient set, which is a set of equivalence classes resulting from an equivalence relation on a larger set. It discusses the formal definition of a quotient set and the need to ensure that the construction of such a set is valid within the axioms of set theory. The paragraph also touches on the axiom of choice and its implications for selecting representatives from each equivalence class.
🧮 Construction of Integers from Set Theory
The paragraph outlines the construction of integers using set theory. It discusses the definition of a suitable equivalence relation on pairs of natural numbers and how this leads to the creation of the set of integers. The paragraph also explains the embedding of natural numbers into the set of integers and the definition of the inverse of a natural number within this context. It concludes with an example of how to perform addition with integers constructed in this manner.
📐 Constructing Rational Numbers and Real Numbers
This section discusses the construction of rational numbers as a quotient set of pairs of integers, modulo an equivalence relation that identifies fractions that are equivalent after factorization. The paragraph explains the embedding of integers into rational numbers and the definition of addition and multiplication for rational numbers. It also mentions the construction of real numbers using Dedekind cuts or Cauchy sequences, although it does not provide a detailed explanation of these methods.
🌐 Continuation to Real Numbers and Upcoming Topics
The final paragraph briefly mentions the continuation of the discussion to real numbers and acknowledges a typo regarding the mention of real numbers. It concludes with a transition to the topic of topology, which will be covered in the next lecture. The paragraph emphasizes the progression from set theory to high school mathematics and the upcoming exploration of continuity and convergence within the framework of topology.
Mindmap
Keywords
💡Set Theory
💡Axioms
💡Bijection
💡Injective, Surjective, and Bijective Maps
💡Isomorphism
💡Countably Infinite
💡Uncountably Infinite
💡Equivalence Relation
💡Quotient Set
💡Natural Numbers
💡Integers
Highlights
The importance of classifying mathematical objects and gaining an overview of structures in set theory.
The recurrent theme in mathematics of classifying spaces using structure preserving maps.
Clarification of the concept of a 'space' as a set equipped with some structure.
Introduction to the concept of a map in set theory as a relation that assigns a unique element from set B to each element in set A.
Explanation of the standard notation for maps and the concept of assigning a value in the context of relations.
Terminology associated with maps, including domain, target, and image of a set under a map.
Definitions of injective, surjective, and bijective maps and their importance in set theory.
The key definition of set theoretical isomorphism between two sets A and B.
The intuition behind isomorphism as pairing elements of two sets, such as boys and girls in classrooms.
The classification of sets into finite, countably infinite, and uncountably infinite.
The concept of a proper subset being isomorphic to the original set as a characteristic of infinite sets.
The composition of maps and its associative property in the context of set theory.
The definition and properties of the inverse of a map, specifically for bijective maps.
The concept of a pre-image under a map and its relation to the image of the inverse of a map.
The construction of integers, rational numbers, and real numbers using axiomatic set theory.
The embedding of natural numbers into the set of integers and the definition of negative integers.
The construction of rational numbers as equivalence classes of pairs of integers under a suitable relation.
The definition of addition and multiplication for rational numbers within the set theoretic framework.
The caution required when defining maps with a quotient set as the domain, especially when using representatives.
Transcripts
Browse More Related Video

Topological spaces - construction and purpose - Lec 04 - Frederic Schuller

Navigating an Infinitely Dense Minefield | Why Measure Infinity?
Sets Theory and Logic Lecture 1 Sets

Russell's Paradox - a simple explanation of a profound problem

Understanding Vector Spaces

Python Hash Sets Explained & Demonstrated - Computerphile
5.0 / 5 (0 votes)
Thanks for rating: